Barber J.R. Intermediate Mechanics of Materials
Подождите немного. Документ загружается.


5
Non-linear and Elastic-Plastic Bending
In this chapter, we shall consider the question of determining the stress distribution
and curvature when a beam is subjected to bending moments sufficient to cause plas-
tic deformation. The same analytical procedure can also be used for the bending of
beams made of materials such as rubber, which have a non-linear elastic constitutive
behaviour.
Engineering components are generally not expected to experience plastic defor-
mation in normal service, but a calculation of the maximum load that can be carried
in a single exceptional loading experience is often of interest from a safety viewpoint.
A dramatic example is provided by studies of the crash behaviour of automobiles,
which are based on elastic-plastic analysis of the structural elements of the vehicle.
We shall also find that when a beam is loaded into the plastic range and then
released, it does not return to its original configuration and there are generally resid-
ual stresses remaining. This information forms the basis of analyses of simple metal
forming processes, such as the forming of a curved bar by plastic bending of a bar
that is initially straight.
5.1 Kinematics of bending
In the elementary theory of bending, it is customary to assume that initially plane
sections remain plane. This assumption seems plausible, but the background to it is
seldom discussed in any detail.
To fix ideas, suppose we have a very long beam, subjected to pure bending by the
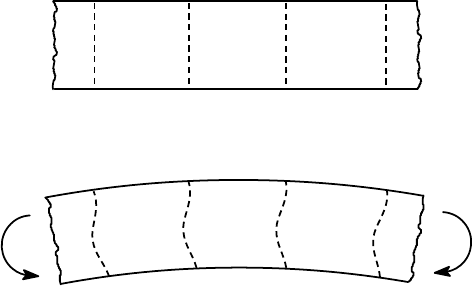
application of equal and opposite moments at the end. We imagine the beam as made
up of a large number of identical slices, with initially parallel plane faces, as shown
in Figure 5.1 (a). The stress distribution near the ends of the beam will be affected
by the precise way in which the moment is applied — i.e. by the traction distribution
on the two end faces, but it is reasonable to assume that this end effect will decay
as we get further from the ends and that there is some ‘preferred’ way in which the
moment will be transmitted along the beam. This idea is known as Saint-Venant’s
J.R. Barber, Intermediate Mechanics of Materials, Solid Mechanics and Its Applications 175,
2nd ed., DOI 10.1007/978-94-007-0295-0_5, © Springer Science+Business Media B.V. 2011
236 5 Non-linear and Elastic-Plastic Bending
principle. Thus, sufficiently far from the ends of the beam, the stress distribution on
all cross-sectional planes will be the same.
A
A
B
B
C
C
D
D
E
E
F
F
M
M
(a)
(b)
Figure 5.1: Deformation of the beam: (a) the undeformed configuration; (b) after
deformation
In particular, the stress distribution across AB in Figure 5.1 (a) will be the same
as that across CD and EF. It follows that the element ABCD is loaded in exactly the
same way as the element CDEF and hence the deformed shapes of the two elements
must be the same. This implies that the deformed shape of the initially straight line
AB must be the same as that of CD.
Now the deformation of the elements must be one which preserves compatibil-
ity — in other words, adjacent slices must still be capable of stacking against each
other without generating any gaps. We illustrate this in Figure 5.1 (b). Each of the
initially straight vertical lines must deform to the same shape and the most general
deformation permitted in the beam is one in which (for example) AB deforms to an
arbitrary new shape and CD deforms to the same shape, but with an arbitrary (small)
rigid-body translation and rotation. The translation of CD to the right relative to AB
will lead to a uniform tensile strain across the element ABCD and the rotation will
lead to a tensile strain that varies linearly with y, regardless of the common deformed
shape of AB and CD. To convince yourself of this, draw an arbitrary curve on a sheet
of paper, copy the same curve onto a transparent sheet and experiment with various
small rigid body displacements of one curve with respect to the other. You will find
that the most general change in the distances between corresponding points on the
curves due to the relative motion is a general linear function of y and furthermore,
that the multiplier on y is proportional to the rigid-body relative rotation of the two
curves in radians.
Similar considerations apply to the more general three-dimensional case and lead
to the conclusion that regardless of the common deformed shape adopted by the var-
ious initially plane sections, the axial strain e
zz
must be a linear function of position
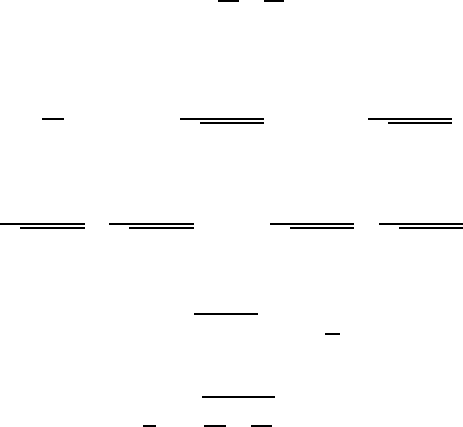
5.2 Elastic-plastic constitutive behaviour 237
in the section — i.e.
e
zz
= C
0
+C
1
x +C
2
y , (5.1)
where C
0
,C
1
,C
2
are unknown constants. This is all that is necessary to establish the
bending theory. It is not necessary to make the more restrictive assumption that plane
sections remain plane. To verify this, go back and review §4.1. You will find that we
only used the assumption to arrive at equation (4.1), which is a reduced form of (5.1).
Notice that this kinematic argument makes no appeal to linearity in the stress-
strain law, since it makes no reference to stress at all. Thus, it applies equally to any
kind of material behaviour, including an elastic-plastic material loaded beyond the
yield stress, a non-linear elastic material, or a generally anisotropic material.
As in §4.1, we can establish a relation between the constants C
1
,C
2
and the radii
of curvature of the beam, leading to the alternative expression
e
zz
= C
0
−
x
R
y
+
y
R
x
. (5.2)
It is sometimes convenient to define a Cartesian coordinate system aligned with the
resultant bending axis. If x
′
,y
′
is rotated through
θ
anticlockwise from x,y, where
tan
θ
= −
C
1
C
2
; sin
θ
= −
C
1
q
C
2
1
+C
2
2
; cos
θ
=
C
2
q
C
2
1
+C
2
2
, (5.3)
we have
x
′
=
C
2
x
q
C
2
1
+C
2
2
−
C
1
y
q
C
2
1
+C
2
2
; y
′
=
C
1
x
q
C
2
1
+C
2
2
+
C
2
y
q
C
2
1
+C
2
2
(5.4)
from (4.40, 4.41). It then follows from (5.1) and the second of (5.4) that
e
zz
= C
0
+ y
′
q
C
2
1
+C
2
2
= C
0
+
y
′
R
, (5.5)
where the resultant curvature
1
R
=
s
1
R
2
x
+
1
R
2
y
. (5.6)
5.2 Elastic-plastic constitutive behaviour
The uniaxial stress strain curve for a typical ductile material is shown in Figure 5.2. It
exhibits a linear elastic region OA, beyond which the curve may be of quite complex
shape. The yield stress S
Y
is defined as that stress beyond which some permanent
deformation is produced — i.e. the stress-strain curve does not return to the point O
on unloading. Experimentally, we need to see a measurable level of permanent strain
to be sure some yield has occurred, so the yield point B is conventionally identified
as the stress sufficient to leave a permanent tensile strain of 0.2% on unloading. The
yield point B is not necessarily identified with the limit of proportionality A, though
for metals they are generally very close.
238 5 Non-linear and Elastic-Plastic Bending
C
D
E
B
.
A
.
O
σ
zz
S
Y
e
zz
Figure 5.2: Uniaxial stress strain curve for a typical ductile material
Beyond the yield point, the stress continues to increase with strain, but the rate of
increase (the slope of the curve) is orders of magnitude lower than that in the linear
elastic range OA.
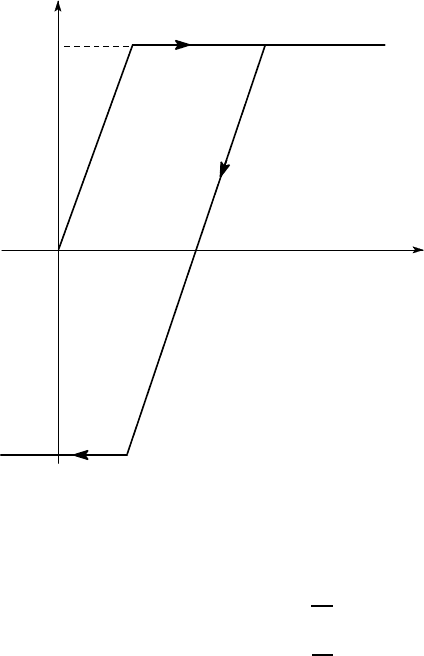
5.2.1 Unloading and reloading
If the stress is increased into the plastic range and then reduced, the material will
generally unload along a straight line (CD in Figure 5.2) with the same slope as
OA. Reloading from D will follow the same line DC, showing that the unload-
ing/reloading process is elastic until the original maximum stress
σ
(C) is exceeded.
Thus a specimen which has been loaded and unloaded along the path OABCD be-
haves as a material with an increased yield stress S
′
Y
=
σ
(C). In effect, it has become
a new ‘work-hardened’ material. Remember that when we machine a specimen of
a ductile material to perform a tensile test, we have no knowledge of the previous
plastic deformation of the material, for example during the rolling process. We es-
sentially measure just the current properties of the material.
During the loading process, work is done by the applied loads and the specific
work (i.e. the work done per unit volume of material) is equal to the area under the
stress-strain curve. In the elastic range, this work is stored in the material as strain
energy (see §2.2.3) and is recovered on unloading. Beyond the yield point B, some
of the work done is dissipated as heat and is not recoverable. For the loading path
OABCD, the specific work done during loading is defined by the area OABCE and
the work recovered during unloading is equal to the triangular area CDE. Thus, the
shaded area OABCD in Figure 5.2 represents the energy dissipated as heat and CDE
represents the elastic strain energy at point C. Notice that the elastic strain energy
increases as a result of work hardening, so not all of the work done between B and C
is dissipated as heat, but for most materials the rate of work hardening is sufficiently
slow for this difference to be negligible.
5.2 Elastic-plastic constitutive behaviour 239
5.2.2 Yield during reversed loading
So far we have discussed only those loading scenarios leading to positive (tensile)
stresses, but many engineering applications involve alternating tensile and compres-
sive stresses (see for example §2.3). For isotropic ductile materials, yielding in com-
pression occurs at
σ
zz
= −S
Y
— i.e. at the same stress magnitude as in tensile load-
ing. This result is implicit in both the Tresca and von Mises theories of ductile failure
(§2.2.3).
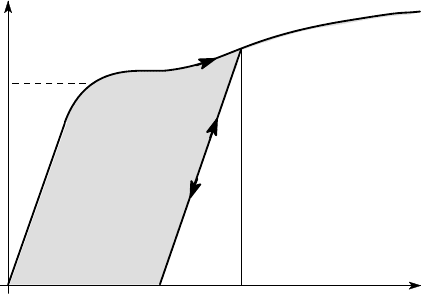
C
D
B
.
A
.
O
σ
zz
S
Y
e
zz
F
Figure 5.3: Yield during reversed loading
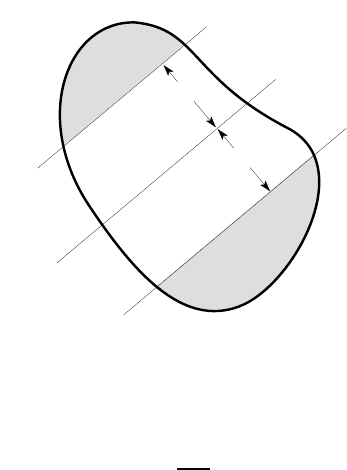
If the material has work hardened, this will generally imply a corresponding in-
crease in compressive yield stress, so that negative loading following the scenario
OABCDF in Figure 5.3 would be expected to involve compressive yield at F, with
CD = DF. This is known as ‘isotropic hardening’, since it assumes that the material
remains isotropic even after plastic deformation. However, significant anisotropic ef-
fects are often observed during plastic deformation, since the shape of the grains and
the distribution of dislocations is influenced by the particular loading scenario. Also,
a drop in the stress for reversed yield is often observed under uniaxial conditions.
This is known as the Bauschinger effect.
1
The conditions at yield under complex
1
See for example, F.A. McClintock and A.S. Argon (1966), Mechanical Behaviour of Ma-
terials, Addison-Wesley, Reading MA, §5.8
240 5 Non-linear and Elastic-Plastic Bending
loading scenarios remain a subject of active research, since finite element methods
now permit complex forming operations to be analysed and the constitutive law for
the material in the plastic r´egime can place limits on the accuracy achievable.
5.2.3 Elastic-perfectly plastic material
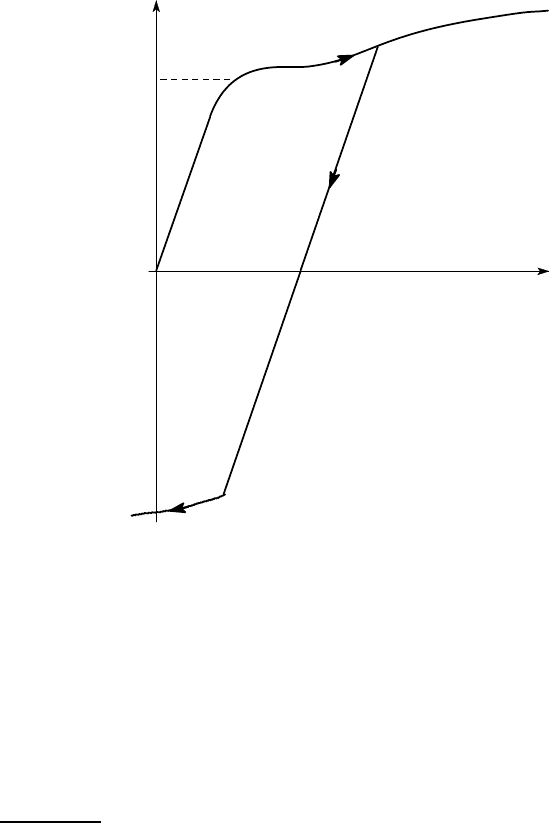
In many applications, it is sufficient to use a simplified constitutive law with no
work hardening — i.e. to assume that the stress remains at a constant yield stress S
Y
during plastic deformation, as shown in Figure 5.4, which also shows the assumed
behaviour for unloading (CD) and for yield under reversed loading (CF). A material
which behaves in this way is described as elastic-perfectly plastic. It represents quite
a good approximation to Figures 5.2, 5.3 as long as the plastic strains are not too
large and this in turn is likely to be true when the plastic zone is adjacent to material
that is still elastic. For example, in the bending of beams, plastic strains sufficient to
cause significant work hardening typically require the beam to be bent to a radius
less than about five times the beam thickness.
C
D
A
O
σ
zz
S
Y
e
zz
F
-
S
Y
Figure 5.4: Constitutive behaviour for an elastic-perfectly plastic material
The loading portion OAC of Figure 5.4 can be described by the equations
σ
zz
= Ee
zz
; |e
zz
| <
S
Y
E
(5.7)
= S
Y
sgn(e
zz
) ; |e
zz
| >
S
Y
E
, (5.8)

5.3 Stress fields in non-linear and inelastic bending 241
where the expression sgn(e
zz
) takes the value +1 if e
zz
> 0 and −1 if e
zz
< 0, and is
included to allow for the possibility of compressive loading
σ
zz
< 0, for which the
corresponding strain will be negative.
5.3 Stress fields in non-linear and inelastic bending
We saw in §5.1 that the tensile strain e
zz
due to bending must be a linear function of
x,y, as defined by equations (5.1, 5.2, 5.5). If the monotonic stress-strain relation is
σ
zz
= f (e
zz
) , (5.9)
the stress will be given by
σ
zz
= f (C
0
+C
1
x +C
2
y) = f
C
0
+
y
′
R
. (5.10)
It follows that, as in elastic bending, there will be a neutral axis defined by the straight
line
C
0
+C
1
x +C
2
y = C
0
+
y
′
R
= 0 (5.11)
on which the stress
σ
zz
= 0. Also, the stress is constant along any line parallel to the
neutral axis. Indeed, a plot of the stress distribution along y
′
, perpendicular to the
neutral axis, has the same form as the constitutive law except for a linear scaling of
the axis.
These results apply for any function f (e
zz
) in equation (5.9) and can therefore
be used for beams made of non-linear elastic materials as well as for elastic-plastic
bending.
For the special case of an elastic-perfectly plastic material, substitution of (5.5)
into (5.7, 5.8) gives
σ
zz
= EC
0
+
Ey
′
R
;
EC
0
+
Ey
′
R
< S
Y
(5.12)
= S
Y
sgn
C
0
+
Ey
′
R
;
EC
0
+
Ey
′
R
> S
Y
. (5.13)
Thus, there will be an elastic region bounded by the two parallel lines
EC
0
+
Ey
′
R
= ±S
Y
, (5.14)
which are also parallel to and equidistant from the neutral axis, as shown in Figure
5.5.
242 5 Non-linear and Elastic-Plastic Bending
plastic
elastic
neutral
axis
d
plastic
d
zz
σ = S
Y
Y
σ = −S
zz
Figure 5.5: Elastic and plastic zones in a beam cross section
The perpendicular distance between each line and the neutral axis is
d =
S
Y
R
E
. (5.15)
Outside these lines, the material will have yielded and the stress will be equal
to ±S
Y
, the positive sign being taken on one side and the negative on the other. At
sufficiently small applied moments, R will be large and the two bounding lines will
lie outside the beam cross section, all of which will therefore lie in the linear elastic
range. However, at some critical bending moment, denoted by M
Y
, one or both of
the bounding lines will touch the section and yielding will start. We refer to M
Y
as
the moment for first yield. Further increase in moment will cause the bounding lines
to move closer together, increasing the size of the plastic zone at the expense of the
elastic zone.
The maximum moment that can be carried, M
P
, occurs when the elastic zone
has shrunk to zero. Equation (5.15) shows that this requires that the curvature be
unbounded (i.e. that the radius of curvature R be zero) so it represents local collapse
of the beam. A point in a beam where this has happened is known as a plastic hinge
(see §5.8 below). The moment M
P
is known as the fully-plastic moment for the beam
section.
5.3.1 Force and moment resultants
If the constants C
0
,C
1
,C
2
defining the strain field are known, we can compute the
force and moment resultants F,M
x
,M
y
by substituting (5.10) into (4.9, 4.13, 4.14) —
i.e.
F =
ZZ
A
σ
zz
dA ; M
x
=
ZZ
A
σ
zz
ydA ; M
y
= −
ZZ
A
σ
zz
xdA (5.16)
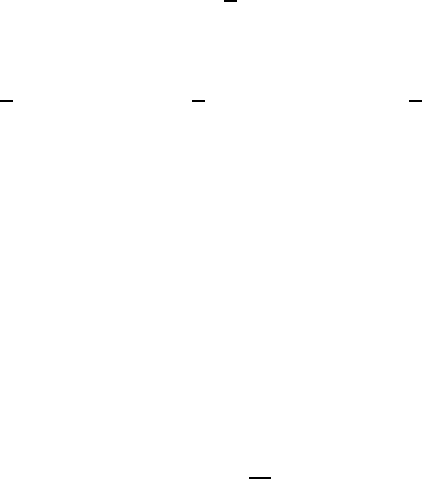
5.4 Pure bending about an axis of symmetry 243
and evaluating the integrals. In the more usual case where F,M
x
,M
y
are specified,
(5.16) provides three simultaneous equations which can be solved for the three un-
knowns C
0
,C
1
,C
2
, after which substitution in (5.10) defines the complete stress dis-
tribution in the beam.
However, the problem is non-trivial because the non-linearity of the constitu-
tive law (5.9) causes the resulting equations to be non-linear. In addition, we can no
longer treat the three resultants separately and use superposition. For a general un-
symmetrical section or a beam loaded by both an axial force and a bending moment,
the neutral axis is likely to move and rotate as the loads increase and most cases are
sufficiently complex to require a numerical solution.
5.4 Pure bending about an axis of symmetry
The solution is considerably simplified if:-
(i) there is no axial force,
(ii) the beam cross section is symmetric about the axis of the applied bending mo-
ment, and
(iii) the relation between stress and strain is the same in tension and compression,
since the neutral axis must then coincide with the axis of symmetry.
To prove this result, we first adopt it as a tentative hypothesis, in which case
e
zz
=
y
R
. (5.17)
Substituting for
σ
zz
from (5.10) into (5.16), we then obtain
F =
ZZ
A
f
y
R
dA ; M
x
=
ZZ
A
f
y
R
ydA ; M
y
= −
ZZ
A
f
y
R
xdA . (5.18)
In view of the symmetry of the beam cross section, symmetrically disposed el-
ements dA with equal and opposite values of y will make equal and opposite con-
tributions to the first and third of these integrals as long as f (y/R) = −f (−y/R) as
required by condition (iii) above. Thus, the conditions F = 0, M
y
= 0 are satisfied
identically by the choice of the symmetry axis as neutral axis, justifying this ini-
tial assumption. The second of (5.18) then defines the relation between the bending
moment M
x
and the radius of curvature of the beam R.
Example 5. 1
A rectangular beam of (horizontal) width b and height h is made of a rubber for
which the uniaxial constitutive law is approximated by the equation
σ
zz
= E
e
zz
+
e
3
zz
e
2
0
,
244 5 Non-linear and Elastic-Plastic Bending
where E, e
0
are material constants. Find the relation between the bending moment
M and the radius of curvature R for bending about a horizontal axis.
If the material fails at a tensile stress
σ
= Ee
0
, find the largest moment that can
be transmitted by the beam.
Using equation (5.17) and the given constitutive law, we have
σ
zz
= E
y
R
+
y
3
R
3
e
2
0
.
The second of (5.16) or (5.18) gives
M = E
Z
h/2
−h/2
Z
b/2
−b/2
y
R
+
y
3
R
3
e
2
0
ydxdy = Eb
Z
h/2
−h/2
y
R
+
y
3
R
3
e
2
0
ydy
= Eb
h
3
12R
+
h
5
160R
3
e
2
0
,
which defines the required relation between M and R.
The maximum tensile stress occurs at y= h/2 and is
σ
max
= E
h
2R
+
h
3
8R
3
e
2
0
.
Thus, failure will occur when
h
2R
+
h
3
8R
3
e
2
0
= e
0
or
h
2Re
0
+
h
2Re
0
3
= 1 ,
which has the solution
h
2Re
0
= 0.682 or R = 0.733
h
e
0
.
The bending moment at this limiting condition is
M
max
= Eb
h
2
e
0
12 ×0.733
+
h
2
e
0
160 ×(0.733)
3
= 0.1296Ebh
3
e
0
.
5.4.1 Symmetric problems for elastic-perfectly plastic materials
The elastic-perfectly plastic constitutive law of equations (5.7, 5.8) has the same be-
haviour in tension and compression and hence satisfies condition (iii) above. As in
Figure 5.5, there will be a central elastic region and symmetrically disposed plas-
tic regions yielded in tension and compression respectively. The bending moment-
curvature relation can be determined exactly as in the preceding example, but the
discontinuity at
σ
zz
=S
Y
necessitates breaking the integral into three parts — one for
the central elastic region and one for each of the plastic regions.
