Appel W. Mathematics for Physics and Physicists

Подождите немного. Документ загружается.

Relations with vector calculus: gradient, divergence, curl 477

or, putting f = ( f

, f

) and using the fact that the components of dω are

the components of the curl curl f ,

curl f d

σ =

f ·dℓ, (17.1)

or in other words still, th e ﬂ ux of the curl of f through a surface is equal to

the circulation of f along the boundary of this surface (Green-Ostrogradski

formula).

Similarly, if ω is a differential 2-form given by

def

= f

dy ∧ dz + f

dz ∧dx + f

dx ∧dy,

its e xterior derivative is then

dω =



∂ f

∂ x

∂ f

∂ y

∂ f

∂ z



dx ∧dy ∧dz = (div f ) dx ∧dy ∧dz,

and if S is a closed surface bounding a volume V such that S = ∂V , we

obtain

ω =

dω, that is,

f ·d

σ =

ZZZ

div f d

Finally, if ω = f is a differential 0-form, that is, a function, since the

differential d f satisﬁes d f ( n) = grad f · n, the Stokes formula expresses that

grad f ·dℓ = f



γ(1)



− f



γ(0)



Note that the relations just stated between exterior derivatives and the

gradient, divergence and curl operators show that the identity d

≡ 0 may

simply be expressed, in the language of the man in the street, as

div curl ≡ 0 and curl grad ≡ 0.

17.5.b Existence of the scalar e le ctrostatic potential

Let f = ( f

, f

) be a vector ﬁeld on R

, and as sume it satisﬁes the condi-

tion

∇∧ f = curl f ≡ 0 (17.2)

at every point of a domain Ω ⊂ R

which is simply connected. Deﬁ ne a

differential 1-form on Ω by

ω = f

= f

dx + f

dy + f

dz.

As we have seen, the exterior derivative of ω is

dω =



∂ f

∂ x

−

∂ f

∂ y



dx∧dy+



∂ f

∂ y

−

∂ f

∂ z



dy∧dz+



∂ f

∂ z

−

∂ f

∂ x



dz∧dx,

478 Differential forms

hence condition (17.2) can be expressed more simply by stating th at

dω ≡ 0. (17.2

′

)

Poincaré’s theorem (the second version, Theorem 17.45) ensures that there

exists a differential 0-form, that is, a function ϕ : R → R, such that

ω = dϕ =

∂ϕ

∂ x

dx +

∂ϕ

∂ y

dy +

∂ϕ

∂ z

dz.

Comparing with the d eﬁnition ω = f

dx + f

dy + f

dz, it follows that

f = ∇ϕ.

This proves the following theorem:

THEOREM 17.48 (Existence of the scalar potential) Let Ω ⊂ R

be a simply

connected open set in R

, and let f : Ω → R

be an irrotational vector ﬁeld on Ω,

that is, su ch that cu rl f ≡ 0. Then there exists a potential ϕ : Ω → R such that

f = ∇ϕ.

Application to electrostatic

Since th e electrostatic ﬁeld satisﬁes ∇∧ E ≡ 0 on R

, both with and without

charges

it follows that it derives from a potential:

E = −∇ϕ.

It is important to remark that the scalar potential only exists because

is simply connected. I f the equation were only satisﬁed in a non-simply

connected subset Ω ⊂ R

, there would be no reason for the potential to exist

globally (its local existence being of course always true).

Too many physics books do not hesitate to state things in roughly the

following way: “since we know that curl grad ≡ 0, it follows that: if curl E ≡ 0,

then E = −grad ϕ.” Th is, we just saw, is a mathematical absurdity and, worse,

a dreadful logical mistake.

Moreover this may discredit physics in the eyes of

students who, in general, are perfectly aware that th ey are being hoodwinked,

even if they may not know the necessary tools and terminology to explain

the problem.

It is perfectly possible to remain rigorous in physics; students

should be made aware of this.

Should you, reader, teach physics one d ay, do not make this mist ake!

This is one of the homogeneous Maxwell equations, giving the global structure of ﬁelds in

the setting of el ectrostatics. Hence all magnetic phenomena have been neglected.

This amounts to mixing up the logical statements (P ⇒ Q) and (Q ⇒ P )!

And who, moreover, would be quite incapable of ﬁnding a counterexample: it is tru e that

E c omes from a potential, but for a very different reason.

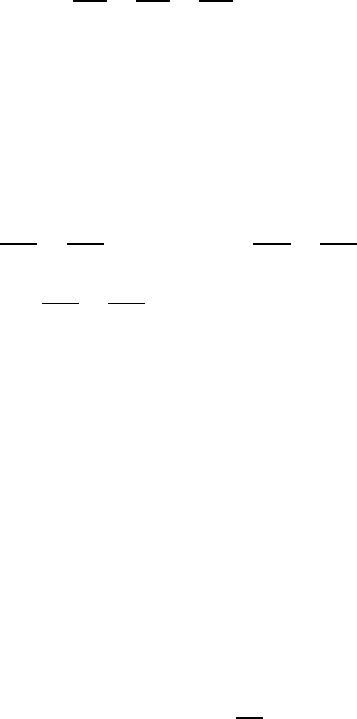
Relations with vector calculus: gradient, divergence, curl 479

17.5.c Existence of the vector potential

Let now f : Ω → R

be a vector ﬁeld with vanishing divergence:

div f = ∇ · f ≡ 0 on Ω. (17.3)

Deﬁne a differential 2-form

def

= f

∧dx

+ f

∧dx

+ f

∧dx

Then the exterior d erivative of ω is simply

dω =



∂ f

∂ x

∂ f

∂ x

∂ f

∂ x



∧dx

and the condition (17.3) can be expressed again simply as

dω = 0.

Poincaré’s theorem (Theorem 17.4 4 now) ensures that if Ω is contractible, there

exists a differential 1-form A = A

+ A

such that

ω = dA



∂ A

∂ x

−

∂ A

∂ x



∧dx



∂ A

∂ x

−

∂ A

∂ x



∧dx



∂ A

∂ x

−

∂ A

∂ x



∧dx

which, putting A = (A

, A

) and comparing with the deﬁnition of ω,

shows t hat

f = ∇ ∧ A = curl A.

THEOREM 17.49 (Existence of the vector potential ) Let Ω ⊂ R

be a contrac-

tible open subset and let f : Ω → R

be a vector ﬁeld with zero divergence on Ω:

div f ≡ 0. Then there exists a vector potential A : Ω → R

such that f = ∇ ∧ A.

In the space R

, the magnetic ﬁeld satisﬁ es the relation div B ≡ 0, a nd

this independently of the distribution of charges. It follows that the magnetic

ﬁeld, on R

which is contractible, comes from a vector potential A.

Remark 17.50 In the general setting of electromagnetism, one ﬁrst proves the existence of the

vector potential A; then, from the equation curl E = −∂ B/∂ t, it follows that curl(E +

∂ A/∂ t) = 0 and hence the existence of the scalar potential ϕ such that

E = −∇ϕ −

∂ A

∂ t

on any contractible open set.

480 Differential forms

17.5.d Magnetic monopoles

The magnetic ﬁeld B satisﬁes div B ≡ 0, and so it comes from a vector poten-

tial; or, more precisely, this is ensured by Poincaré’s theorem when considering

a contractible set (in particular it is not sufﬁcient to have a simply connected

set).

What happens if we consider a magnetic monopole, th at is, a point-like particle

which transforms one t he Maxwell equations to be

div B( r, t) = q

δ( r) ? (17.4)

The relation div B ≡ 0 is satisﬁed now only on R

\{0}, which is not contractible.

It is possible to show then that there does not exist a vector potential A deﬁned

on R

\{0} such tha t we have B = ∇ ∧ A at every point of R

\{0}. But

since a local description of the magnetic ﬁeld using a vector potential remains

possible, it is possible to show that one may deﬁned two potentials A

and

deﬁned on a neighborhood of th e upper half-space (resp. lower half-space)

such that the relations ∇∧A

= B for i = 1, 2 hold on the respective domains

where the vectors are deﬁned. For instance, A

and A

may be deﬁ ned on

the following grayed domains:

0 A

Hence the existence of magnetic monopoles would have as a consequence

that the vector potential does not exist. Experiences have been attempted

to discover mag net ic monopoles (which have amusing theoretical properties;

for instance, their existence automatically implies the quantiﬁcation of the

elementary electric charge), but without success.

17.6

Electromagnetism in the l anguage

of differential forms

Physicists see the vector potential usually as a vector, but it should rather

be seen as a differential form. Indeed, if A itself does not have an intrinsic

meaning, the integral of A along a path has one: for a closed path γ, the

integral

A ·dℓ is equal to the ﬂux of the magnetic ﬁeld through a surface

S bounded by γ (see formula (17.1)).

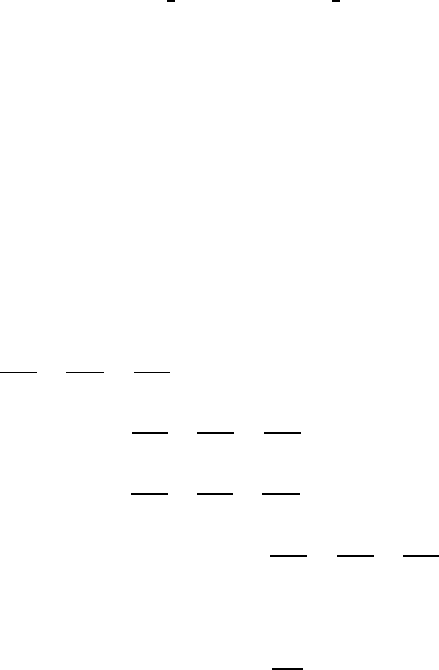
Electromagnetism in the language of differential forms 481

This is why, in special relativity, the components of the potential four-

vector (vector potential + scalar potential) are denoted in contravariant nota-

tion:

A = A

The Faraday tensor (which is a differential 2-form or equivalently a ﬁeld





-tensors) is d eﬁned as the exterior derivative of A:

F = dA, namely, F =

µν

∧dx



∂

−∂



∧dx

where complete summation on repeated indices is performed. Denoting the

potential four-vector A = (A

, A), E = grad A

− ∂ A/∂ t and B = curl A,

the coordinates of F are given by

= E

, F

= −B

= E

, F

= B

= E

, F

= −B

that is, we have

F = E

dx ∧dt + E

dy∧dt +E

dz ∧dt −B

dx ∧dy −B

dy∧dz + B

dz ∧dx.

Since F = dA, its exterior derivative dF is zero, wh ich means that

0 =



∂ B

∂ x

∂ B

∂ y

∂ B

∂ z



dx ∧dy ∧dz



∂ E

∂ y

−

∂ E

∂ z

∂ B

∂ t



dt ∧dy ∧dz



∂ E

∂ z

−

∂ E

∂ x

∂ B

∂ t



dt ∧dz ∧dx



∂ E

∂ x

−

∂ E

∂ y

∂ B

∂ t



dt ∧dx ∧dy.

If we reorder the terms, we obtain t he Maxwell equations without source:

dF = 0 ⇐⇒







curl E = −

∂ B

∂ t

div B = 0

Maxwell equations

without source.

How is it possible to ob tain the Maxwell equations with source? We need

to involve derivatives of E and B, but we cannot get this from dF (which is

identically zero). Another differential form is needed, constructed using E

and B and involving derivatives of the ﬁrst order.

Now notice that the space of exterior 1-forms is a vector s pace of dimen-

sion





= 4 (generated by dt, dx, dy, and dz), and the the space of exterior

482 Differential forms

3-forms is of dimension





= 4 also. Using the Hodge operator, an isomor-

phism between both spaces is constructed:

DEFINITION 17.51 (Hodge operator) The Hodge operator, denoted “⋆”, is a

linear map deﬁned on the spaces of exterior forms on R

⋆ : Λ

∗k

) −→ Λ

∗(n−k )

∧···∧dx

7−→

(n −k)!

,...,i

k+1

,...,i

k+1

∧···∧ dx

with complete summation on repeated indices. For any k ∈ [[0, n]], the Hodge

operator is an isomorphism between th e space of k-forms and the space of

(n −k)-forms.

Example 17.52 Consider the case n = 3. Then

⋆

dx =

(3−1)!



dy ∧ dz − dz ∧ dy



= dy ∧ dz.

Similarly

⋆

(dx ∧dy) =

(3 −2)!

dz = dz.

The Hodge dual of a k-form is thus a (3 −k)-form.

In the case n = 4, we ﬁnd for instance that

⋆

dx = dy ∧dz∧dt and that

⋆

(dx ∧dy) = dz ∧dt.

A k-form is mapped to a (4 −k)-form.

From the differential 2-form F , we can thus construct another 2-form,

namely,

⋆

F . It is given by

⋆

F = −B

dx∧dt−B

dy∧dt−B

dz∧dt+E

dx∧dy+E

dy∧dz+E

dz∧dx.

Let J be the differential 1-form J = ρ dt + j

dx + j

dy + j

dz, and consider

its dual (image) by the Hodge operator

⋆

J = ρ dx ∧dy ∧ dz − j

dt ∧dy ∧dz − j

dt ∧dz ∧dx − j

dt ∧dx ∧dy.

The equation

⋆

F =

⋆

J (17.5)

is then equivalent to

curl B −

∂ E

∂ t

= − j

div E = ρ

Maxwell equations

with source.

Before concluding, a last remark about the differential form

⋆

J . The

equation (17.5) means that t his form is ex act (being the exterior derivative

⋆

F ). Hence its exterior derivative is identically zero, that is, we have

⋆

J =



∂ρ

∂ t

∂ j

∂ x

∂ j

∂ y

∂ j

∂ z



dt ∧dx ∧dy ∧dz ≡ 0,

Electromagnetism in the language of differential forms 483

which is simply the equation of conservation of charge, which is indeed known

to follow from the Maxwell equations.

Note that the components of F and

⋆

F may be written down in matrix

form. They are

F = (F

µν

) =







0 E

−E

0 −B

−E

0 −B

−E

−B







⋆

F = (

⋆

µν

) =







0 −B

−B

0 E

−E

0 E

−E







The coordinates of F and

⋆

F satisfy also

⋆

αβ

αβµν

µν

where ǫ is the totally antisymmetric tensor deﬁned by ǫ

1234

= 1, which is

called the Levi-Civita tensor. It satisﬁes ǫ

αβµν

= 1 if (α, β, µ, ν) is an

even permutation of (1, 2, 3, 4), ǫ

αβµν

= −1 if it is an odd permutation,

and ǫ

αβµν

= 0 if any index is repeated more than once.

Remark 17.53 What are the laws of electromagnetism in two dimensions? If we want to keep

the local nature of th e fundamental laws of the universe, it is their dif ferential expression whic h

should be preserved in o rder to extend them to such imaginary universes. Thus, one should

keep the structure of the Faraday tensor for the electromagnetic ﬁeld. This may be written here

µν







0 E

−E

0 B

−E

B 0







which shows that the electric ﬁeld has two components, whereas the magnetic ﬁeld is a scalar

ﬁeld (or rather a pseudo-scalar, which changes sign during a change of basis that reverses the

orientation).

484 Differential forms

PROBLEM

 Problem 6 (Proca lagrangian and Cavendish experiment) We want to change the Maxwell

equations in order to describe a “photon with nonzero mass.” For this purpose, we start

from the lagrangian description of the ele ctromagnetic ﬁeld, and add a “mass term” to the

lagrangian. Then we look for detectable effects of the resulting equations.

I. Recall that a lagrangian density describing the laws

of electromagnetism is given by

def

= −

αβ

− J

/ǫ

with units where }h = c = 1 [49, 50, 57], where the F

αβ

are the covariant coordinates

of the Faraday tensor, related to the potential four-vector by F

αβ

= ∂

−∂

. We

have denoted here ∂

= ∂/∂ x

and used the Einstein convention for the summation

of repeated indices (see Chapter 16 concerning tensors).

1. Show that the equations of movement, given by t he Euler-Lagrange equations

∂L

∂ A

= ∂

∂L

∂(∂

)

provide the Maxwell equations with source. Write them in Lorenz gauge, in which

the condition ∂ ·A = ∂

= 0 is i mposed.

2. A mass term of the type

is added to the classical lagrangian to obtain the Proca lagrangian

def

= −

αβ

− J

/ǫ

Write the equations of movement associated with this lagrangian. Show that, in

Lorenz gauge, they are given by

( + µ

= J

/ǫ

3. Express the relation between the frequency of an electromagnetic wave and its wave

number. Show that µ has the dimension of mass (still with }h = c = 1). Using the

de Broglie relations, show that th e waves thus described are massive.

4. Consider the ﬁeld created by a single, immobile, point-like particle situated at the

origin. Show that only the component A

= ϕ is nonzero. Show th at this scalar

potential is of the “Yukawa potential” type and is equal to

ϕ( r ) =

4πǫ

−µr

What is the typical decay distance for this potential?

II. In 1772, Cavendish realized an experiment to check the 1/r decay of the Coulomb

potential [50].

Consider two hollow spheres, perfectly conducting, concentric with respective radii R

and R

, wit h R

< R

. At the beginning of the experiment, both spheres are electrically

neutral, and the exterior sphere is given a potential V . Then the spheres are joined

(with a thin metallic wire for instance).

That is, the Maxwell equations for the electromagnetic ﬁeld, and the Newton equation

with Lorentz force for particles.

Solution of the problem 485

1. Show that, if the electrostatic potential created by a poi nt-like charge follows the

Coulomb law in 1/r, the inner sphe re remains electrically neut ral.

2. We assume now that the electromagnetic ﬁeld is described by the Proca lagrangian

and that the electric potential created by a point-like charge follows th e Yukawa

potential. Show that this potential satisﬁes the differential equation

(−△+µ

) ϕ = ρ/ǫ

3. Show that the electric ﬁeld has a discontinuity σ/ǫ

when passing through a surface

density of charge σ.

4. Compute the potential at any point of space after the experiment is ended (both

spheres are at the same potential V ). Deduce what is the charge of the inner sphere.

(One can assume that µ is small compared to 1/R

and 1 /R

and simplify the

expressions accordingly.)

5. Knowing th at C avendish, in his time, could not measure any charge on the inner

sphere, and that he was able to measure charges as small as 10

−9

C, deduce an upper

bound for the mass of a photon. Take V = 10 000 V and R

≈ R

= 30 cm.

Any comments?

SOLUTION

 Solution of problem 6.

I. 1. In Lorenz gauge, one ﬁnds ∂

µν

= J

, whic h i s indeed the covariant form of the

Maxwell equations.

2. Immediate co mputation.

3. Notice ﬁrst that, in analogy w ith the Klein-Gordon equation, the additional term

deserves to be called a “mass term.” If we consider a monochromatic wave with

frequency ω and wave vector k, the equation of movement in vacuum (+µ

) A

0 gives −ω

+ k

+ µ

= 0. The de Broglie relations link frequency and energy on

the one hand, and momentum and wave vector on the other hand. We obtain then

= p

+ µ

, which may be interpreted as the Einstein relation between energy,

momentum, and mass, taking µ as the mass. In fact, it is rather }hµ/c which has

the dimension of a mass.

4. We have J

= ( qδ, 0); the components A

for i = 1, 2, 3 therefore are solutions of

the equation ( + µ

) J

≡ 0 and are zero up to a free term.

The component ϕ = A

, on the other hand, satisﬁes

ϕ −△ϕ + µ

ϕ = q δ( r )/ǫ

which is independent of time. Looking for a st ationary solution, we write therefore

(−△+µ

) ϕ = q δ( r)/ǫ

which (see Problem 5, page 325) can be solved using the Fourier transform and a

residue computation, and yields the formula stated. The typical decay distance is

therefore µ

−1

II. 1. When the exterior sphere i s charged with the potential V , the potential at any point

exterior to the two spheres is (using for instance Gauss’s theorem on th e electric

ﬁeld)

ϕ( r ) ≡ V

for all r > R

486 Differen tial forms

The inner potential, on the other hand, is uniform and equal to V (same method).

In particular, the inner sphere is already at the same potential V . The electric wire

is also itself entirely at the potential V . Hence there is no electric ﬁeld, no current

is formed, and the inner sph ere remains neutral.

2. If elec tromagnetism follows the Proca lagrangian, then the Poisson law is not valid

anymore, and consequently, Gauss’s theorem is not either. The potential created by

a point-like charge being

Φ( r) =

4πǫ

−µr

it satisﬁes the differential equation (µ

−△)Φ = δ/ǫ

(which is indeed the differ-

ential equation of Qu estion I.2 in stationary regime); by convolution, the potential

created by a distribution of charge ρ therefore satisﬁes (µ

− △)ϕ = ρ/ǫ

(recall

that △(Φ ∗ρ) = (△Φ) ∗ρ).

3. Gauss’s theorem is not valid anymore, since we have

div E = −△ϕ = ρ/ǫ

−µ

ϕ.

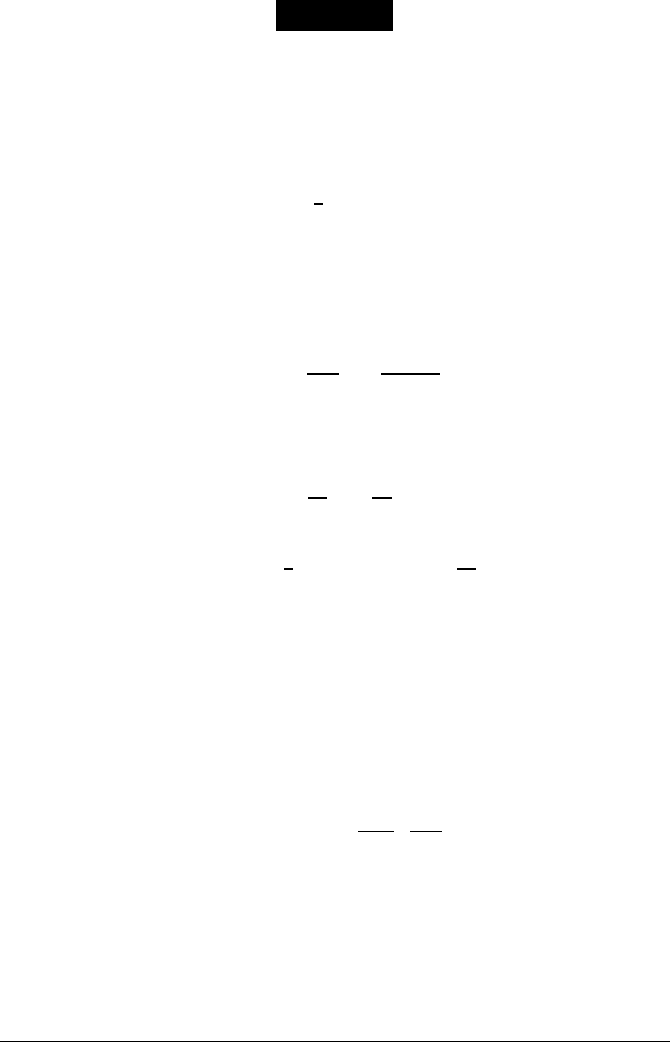
Consider a charged surface element, surrounded by a surface S .

Then we have

E ·dS =

ZZZ

△ϕ d

r =

ZZZ



−µ



But, close to σ, the Yukawa potential is regular (i.e., behaves like the Coulomb

potential), and hence

RRR

ϕ d

r tends to 0 when the volume tends to 0. The

distribution ρ is a Dirac surface distribution surfacique. It follows that the discon-

tinuity of the normal component of the electric ﬁeld is σ/ǫ

4. Since the potential has radial symmetry, it satisﬁes the equation

∂

∂ r

(rϕ) −µ

ϕ = 0

at every point in space except where r = R

or r = R

. The general form of the

potential is therefore

ϕ(r) =

cosh µr +

sinh µr.

Denote by ϕ, χ , and ψ the functions giving the potential (respectively) inside the

inner sphere, between the two spheres, and outside the exterior sphere.

The potential ϕ is bounded in the neighborhood of the origin, and t h erefore may

be writ ten

ϕ(r) = A

sinh(µr)

which, together wit h t h e condition of conti nuity of the potential ϕ(R

) = V , gives

ϕ(r) = V

sinh(µr)

sinh(µR

)