Zehnder E. Lectures on Dynamical Systems: Hamiltonian Vector Fields and Symplectic Capacities

Подождите немного. Документ загружается.

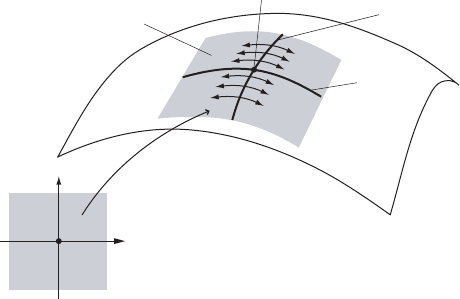
V.10. Integrable systems, action–angle variables 231

(3) We return to our chosen point p 2 N

and to the Liouville chart .W; /

of Lemma V.42, where maps the open neighborhood W of p onto the open

neighborhood V of 0 2 R

and satisﬁes .p/ D 0. To extend the inverse map

1

W V ! W , we introduce the map

# W R

 D

! M; #.x; y/ ´ '

1

.0; y/;

where D

is a small neighborhood of 0 2 R

and '

for x 2 R

is, as in the

ﬁrst step, the ﬂow of the Hamiltonian vector ﬁelds X

.Ify D 0, we obtain

#.x;0/ D '

.p/ and the ﬂow does exist for all x 2 R

, as we have already seen.

We shall show that also for small y 2 D

the map #.x;y/is deﬁned for all x 2 R

In view of Corollary V.43,

#.x;y/ D

1

.x; y/;

for small x 2 R

. Geometrically we apply the ﬂow maps '

to the section S D

1

.0; y/ 2 M j y 2 D

g which, in view of Corollary V.43 is transversal to the

vector ﬁelds X

1

S D

1

.0; D

#..1; 1/  D

Figure V.3. The geometrical meaning of the map #, ﬂowing out from the transversal section

S of N

From the invariance F B '

D F we conclude by means of Lemma V.42 that

F.#.x;y// D F.'

1

.0; y// D F.

1

.0; y// D y;

so that on its domain of deﬁnition the map # satisﬁes

# W R

fyg!N

D F

1

.y/

232 Chapter V. Hamiltonian vector ﬁelds and symplectic diffeomorphisms

for y 2 D

. In the special case y D 0 the map #.x;0/ D '

.p/ is, due to step (1),

deﬁned for all x 2 R

and maps R

f0g onto the submanifolds N

D F

1

.0/.

From this it follows for D

sufﬁciently small that the map # is deﬁned on K D

where K  R

is a compact set containing the generators 

2  of the lattice.

We show that # is deﬁned on R

 D

.Ify D 0, then #.x C ; 0/ D #.x;0/

for all x 2 R

and all  2 . We construct for y in a small neighborhood of

0 an n-dimensional lattice .y/  R

which is close to .0/ D  and satisﬁes



x C .y/;y



D #.x;y/ for all x 2 R

and for all .y/ 2 .y/.

If  D 

2  is a generator of the lattice, then '



.p/ D p and .p/ D 0.In

the Liouville chart V  R

the map

./ B '



1

W .x; y/ 7! .; /

is a local symplectic diffeomorphism near the ﬁxed point 0 in R

Lemma V.44. The symplectic map ./ has the form

 D x  v.y/; v.y/ D

Q.y/;  D y

for a smooth real-valued function Q which satisﬁes Q.0/ D 0. Moreover, v.0/ D 0.

Proof. By deﬁnition '



1

.x; y/ D

1

.; / and from F B'

D F it follows

using Lemma V.42 that

y D F B

1

.x; y/ D F B '



1

.x; y/ D F B

1

.; / D :

In Section V.9. we have seen that every symplectic map which extends the relation

 D y is necessarily of the desired form. The statement v.0/ D 0 follows from

B '



1

.0/ D 0, and the lemma is proved. 

By deﬁnition, '



1

.x; y/ D

1

.; /, and hence in view of Lemma V.44

and Corollary V.43,

./

1

.x; y/ D '

Cv.y/

1

.x; y/

for .x; y/ 2 V

 V . Carrying out this construction for every generator 

of  we

obtain the maps v

.y/ D

.y/, which satisfy the identity ./ with 

.y/

replacing  C v.y/. Deﬁning 

.y/ D 

C v

.y/, we therefore introduce the

lattice .y/  R

.y/ D

.y/ D

j D1



.y/ j n

2 Z



In view of Lemma V.44,



.y/ D 

C v

.y/ D

.y/;

V.10. Integrable systems, action–angle variables 233

with the real-valued functions W

.y/ D Q

.y/ Chy;

i satisfying W

.0/ D 0 for

all 1  j  n. From v

.0/ D 0 we deduce 

.0/ D 

and so

.0/ D 

if y D 0. We conclude for small y that the lattice vectors 

.y/ are linearly

independent and satisfy, due to ./, the equations

.]/

1

.x; y/ D '



.y/

1

.x; y/

for all .x; y/ 2 V

 V and for all 1  j  n.

Lemma V.45. The map #.x;y/ D '

1

.0; y/ is deﬁned on R

 D

and

satisﬁes

(i) #



! D !

(ii) #



x C .y/;y



D #.x;y/ for all .y/ 2 .y/ and all .x; y/ 2 R

 D

Proof. The map # is deﬁned for .x; y/ 2 K D

where K  R

is a compact set

containing the generators of  D .0/ in its interior, if D

 R

is a sufﬁciently

small neighborhood of 0 2 R

. For small x we ﬁnd in view of the identity .]/,

#.x C 

.y/; y/ D '

xC

.y/

1

.0; y/

D '

B '



.y/

1

.0; y/

D '

1

.0; y/

D #.x;y/

for every generator 

.y/ of . Therefore, we can indeed continue the ﬂow x 7!

#.x;y/ D '

1

.0; y/ to all x 2 R

and it remains to prove that #



! D !

.If

.x; y/ 2 R

D

, we choose x

2 R

near x such that .x x

;y/lies in V . This

way we can represent # locally as a composition of symplectic maps, since in view

of Corollary V.43 we obtain

#.x;y/ D '

C.xx

1

.0; y/

D '

1

.x  x

;y/

D '

1

B 

.x; y/;

with the translation 

.x; y/ D .x x

;y/in R

. As a composition of symplectic

maps, the map # is symplectic and the lemma is proved. 

We have already seen that #.R

fyg/  F

1

.y/. Next we show that # induces

a diffeomorphism

# W R

=.y/ ! #.R

fyg/  F

1

.y/

234 Chapter V. Hamiltonian vector ﬁelds and symplectic diffeomorphisms

for every small y 2 D

. It then follows in particular that the image is diffeomorphic

to the torus T

. Since #.;y/is a local diffeomorphism, it only remains to show that

# is injective, so that .y/ is indeed the isotropy group of #.;y/. By construction

the lattice .y/ is n-dimensional and we have to show that the periods 

.y/ are

in fact minimal. Arguing by contradiction we assume that there exist bounded

sequences .x

/ and .x

/ satisfying y

! 0 and having the properties

#.x

/ D #.x

/ and x

 x

… .y

Going over to subsequences we may assume that x

! x



and x

! y



so that

#.x



;0/D #.y



;0/and hence x



y



2 .0/ D . Therefore x

x

is near a

lattice point in .y

/. Since the lattice is discrete, x

x

2 .y

/ must hold true

for large j , in contradiction to the assumption. Consequently, we do, indeed, have

a diffeomorphism R

=.y/ ! #.R

fyg/.

(4) Finally, we normalize all lattices .y/to Z

by a symplectic diffeomorphism

 W R

 D

! R

 D

;.;/7! .x; y/:

The lattice .y/ is generated by the lattice vectors



.y/ D 

C v

.y/ D

.y/:

The diffeomorphism  should satisfy

.Z

fg/ D .y/ fyg;D .y/

where we still have to deﬁne the diffeomorphism . We deﬁne the map  implicitly

by means of the generating function

S.;y/ D

j D1



.y/;

so that



@

S.;y/ D W

.y/ and x

S.;y/ D

sD1



.y/:

The ﬁrst line deﬁnes the map  D .y/ D .W

.y/;:::;W

.y//. It is a local

diffeomorphism in the neighborhood of 0 D .0/, in the statement of the theorem

denoted by W D

! D

. This follows because the Jacobi matrix of  is equal to

d.y/ D





.y/;:::;

.y/



V.10. Integrable systems, action–angle variables 235

and the column vectors 

.y/ are linearly independent. Since the matrix S

y

.; y/

is equal to d.y/ and therefore is invertible for all  if y is small, the function S

generates a symplectic map  W R

 D

! R

 D

. It satisﬁes

.e

;/D .

.y/; y/;  D .y/:

Hence .Z

fg/ D .y/ fyg for  D .y/ as desired. The composition

ˆ D # B  W .R

 D

/ ! .M; !/

is symplectic, satisﬁes ˆ. Cj; / D ˆ.; / for all j 2 Z

, and therefore induces

a symplectic diffeomorphism

ˆW R

 D

! U  M

onto an open neighborhood of N

. The map ˆ deﬁnes the desired action- angle

variables .; / 2 T

D

. Indeed, ˆ.T

fg/ D F

1

.y/ \U where  D .y/

and

 B F B ˆ.; / D  B F B # B .;/

D  B F B #.x;y/

D  B F B '

1

.0; y/

D  B F B

1

.0; y/

D .y/

D :

This completes the proof of the theorem of Arnold and Jost.

Outlook. Integrable systems are extremely rare and therefore attract special atten-

tion and we refer to J. Moser’s lecture [72] given at the ICM 1998 in Berlin. On the

other hand, there are many systems in nature, which are not integrable, but which

are near an integrable system. The most prominent is our planetary system. We

therefore wonder how such an integrable system behaves under perturbation. We

recall that an integrable system X

on the phase space T

 D of the angle and

action variables .x; y/ can be solved immediately. The Hamiltonian function h.y/

depends only on the action variable y 2 D, so that the ﬂow leaves every single torus

fyg invariant. On the torus the ﬂow is the Kronecker ﬂow x 7! x C t!.y/,

where !.y/ D

h.y/ 2 R

are the frequencies associated with the torus T

fyg.

If the frequencies !.y/ D .!

;:::;!

/ are rationally independent or, more

explicitly, if h!;ji…Z for all j 2 Z

nf0g, then every orbit on this torus is

dense, in view of the proposition by Kronecker (Corollary I.7). Otherwise, there

exist non-trivial relations of the form h!;ji2Z for integer vectors j 2 Z nf0g,

so-called resonance conditions. In this case, the closure of every orbit lies densely

236 Chapter V. Hamiltonian vector ﬁelds and symplectic diffeomorphisms

in a torus of lower dimension. If there exist n1 independent resonance conditions,

then every orbit is periodic on the corresponding torus. In the nondegenerate case

deﬁned by the condition

det

!.y/ D det

h.y/ ¤ 0; y 2 D

the frequencies !.y/ actually do depend on the action variables y and all the differ-

ent types of tori are mixed together. It turns out that these tori T

fyg, depending

on their frequency vectors !.y/, react completely differently to a Hamiltonian per-

turbation H of h of the form

H.x;y/ D h.y/ C h

.x; y/;

where the perturbation term h

is small and periodic in the angle variable, so that

.x Cj; y/ D h

.x; y/ for all integer vectors j 2 Z. As an example we consider

an irrational torus T

fyg, whose frequency vector is strongly irrational in the

sense that it satisﬁes the Diophantine inequalities

jh!.y/; j ij  jj j



for all j 2 Z

nf0g, with two constants >0and >n 1. One can prove that

such a torus survives under the perturbation h

as an invariant parametrized torus,

on which the ﬂow is conjugated to the unperturbed ﬂow, if only the perturbation h

is sufﬁciently small and sufﬁciently smooth. Therefore, the torus is only slightly

deformed. For this purpose, one constructs an embedding

' W T

fyg!T

 D

of the unperturbed torus into the phase space, which is in the neighborhood of the

inclusion map of the torus and solves the partial differential equation

d'.x/! D J rH.'.x// for all x 2 T

where ! D !.y/ are the frequencies of the unperturbed system,

Such an embedding ' maps the solution .t/ D  Ct! on the unperturbed torus

fyg onto the solution x.t/ ´ '..t// on the perturbed torus '.T

fyg/,

since using the above partial differential equation,

Px.t/ D d'..t//

.t/

D d'..t // !

D J rH.'..t///

D J rH.x.t//:

V.10. Integrable systems, action–angle variables 237

The partial differential equation is an analytically very subtle boundary value prob-

lem for periodic boundary condition. Solutions can be constructed by means of

powerful iteration methods, called KAM theory. The acronym KAM stands for

Kolmogorov–Arnold–Moser. The KAM theory guarantees not only one, but an

abundance of such invariant tori for the vector ﬁeld X

, under suitable conditions

on the smoothness of H and the smallness of the perturbation h

. The set of all

these invariant tori form a set of positive Lebesgue measure in T

D which, how-

ever, is nowhere dense in T

D, so that the complement of this set of stable orbits

for n  3 is connected. It immediately follows that perturbed integrable systems

cannot be ergodic, as the famous physicist E. Fermi has claimed. So far, the system

still looks like an integrable system, at least as far as the measure is concerned.

However, in the complement of the set of invariant KAM tori something com-

pletely different happens under the perturbation. Here the invariants and stability

properties break down and unstable phenomena show up generically. They can

give rise to a slow so-called Arnold diffusion through the phase space and even to

escaping solutions.

That in the Hamiltonian systems near integrable systems stable and unstable

orbits coexist and cannot be separated from each other is best illustrated by the

celebrated sketch due to V. I. Arnold and A. Avez in their book [8]. It demonstrates

vividly the complexity of the orbit structure of a smooth nonlinear area preserving

diffeomorphism ' near an elliptic ﬁxed point in the plane R

. As explained in

Section VIII.5 such a map arises, for example, as a transversal section map of a

stable periodic orbit on the 3-dimensional energy surface of a Hamiltonian system.

Instead of studying nearby solutions for all times one can, just as well, study all the

iterates of the section map.

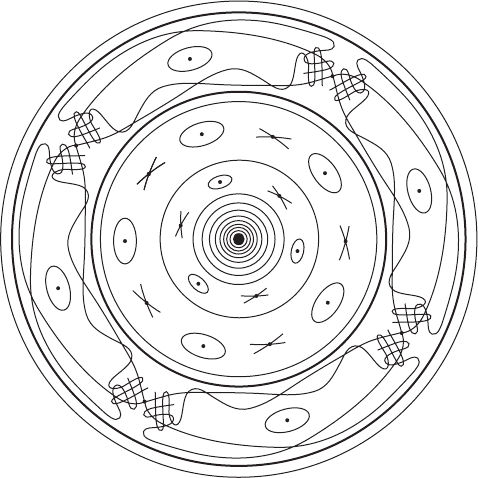
The elliptic ﬁxed point 0 at the center of Figure V.4 is surrounded by smooth

closed curves which are close to circles and which are invariant under the map '.

Their existence was established by J. Moser, 1962, in [70]. For a recent proof in

the analytic case we point out [62] by M. Levi and J. Moser. On every invariant

curve the map is conjugated to the rigid rotation of a circle by an irrational rotation

which depends on the curve. These invariant curves represent the stable part of

the map ' and ﬁll out a Cantor set of relatively large measure and reﬂect the fact

that in a neighborhood of the elliptic ﬁxed point the map ' is close to an integrable

map. One concludes, in particular, that the ﬁxed point is topologically stable.

However, between these invariant curves one sees generically orbits of elliptic and

hyperbolic periodic points. Taking a closer look, one discovers that the stable and

unstable invariant manifolds issuing from the hyperbolic periodic points intersect

transversally in homoclinic points. Recalling the discussion in Chapter III, the

homoclinic points give rise to invariant hyperbolic sets for higher iterates of the

map ', near which the unstable and unpredictable orbit structure is described by

means of topological Bernoulli systems. The elliptic ﬁxed point is a cluster point

of homoclinic points. The picture is repeated near the elliptic periodic orbits for

238 Chapter V. Hamiltonian vector ﬁelds and symplectic diffeomorphisms

Figure V.4. The sketch of Arnold and Avez, after [8].

higher iterates of the map ', and so on. The existence of the homoclinic orbits in

the picture was established in 1973 by E. Zehnder in[120]. A modern version is due

to C. Genecand [39]; further references are A. Chenciner [17] and J. Moser [73].

Literature. The monographs [2] by R. Abraham and J. Marsden, [45] by C. God-

billon, [66] by K. Meyer and G. Hall, [107] by C. L. Siegel and J. Moser and

[73] by Moser are classics in Hamiltonian mechanics and present also some of

the interesting history of the topics. Examples of integrable systems and applica-

tions to celestial mechanics are contained in the lecture notes [74] by J. Moser and

E. Zehnder. An efﬁcient introduction into the Hamiltonian formalism on symplec-

tic manifolds is in Chapter 3 of [30] by J. J. Duistermaat. The monograph [65]by

D. McDuff and D. Salamon goes beyond the formalism and introduces symplectic

topology. Introductions into the KAM theory containing the references to the old

masters are the lectures [61]byR.delaLlaveand[86] by J. Pöschel and the paper

[97] by D. Salamon. The KAM theory in the conﬁguration space is treated in [98]

by D. Salamon and E. Zehnder.

Chapter VI

Questions, phenomena, results

So far we have introduced the concepts of symplectic manifolds and symplectic

diffeomorphisms and have presented the Hamiltonian formalism which was used

and taught in physics and mathematics over centuries. We now turn to more recent

developments which are at the beginning of the new ﬁeld of symplectic topology. In

the present, very short, Chapter VI we shall raise some questions and present some

phenomena and results, in order to motivate the detailed construction of symplectic

invariants later on in Chapter VII.

VI.1 Geometric questions

We consider two compact domains K

and K

in R

which possess smooth bound-

aries and which are diffeomorphic by an orientation preserving diffeomorphism

uW K

! K

. We look for conditions on these two domains which allow us to con-

clude that there exists a diffeomorphism W K

! K

that is volume preserving

and hence satisﬁes det d .x/ D 1 for all x 2 K

, or equivalently,



 D  where  D dx

^^dx

is the volume form on R

. In order to derive necessary conditions we assume that

W K

! K

is a volume preserving diffeomorphism. Then it follows from the

variable transformation formula for every integrable function f W K

! R that

f . .x// dx D

f . .x// jdet d .x/jdx D

D .K

f.x/ dx:

If f D 

is the characteristic function of the domain K

, then f B D 

and

we obtain

vol.K

/ D vol.K

so that the domains must have the same volume. We see that the total volume is an

invariant of volume preserving diffeomorphisms. It turns out that this condition is

also sufﬁcient, as the following statement shows.

Theorem VI.1 (Dacorogna–Moser, 1990). If K

and K

are two compact domains

in R

which possess smooth boundaries, are diffeomorphic and have the same

volume, vol.K

/ D vol.K

/, then there exists a volume preserving diffeomorphism

W K

! K

, hence satisfying



 D .

240 Chapter VI. Questions, phenomena, results

For the proof of a more general theorem with precise regularity conditions we

refer to Dacorogna and Moser [25]. That the total volume is the only invariant of

volume preserving diffeomorphisms is also demonstrated by the following result

for compact manifolds without boundaries.

Theorem VI.2 (Moser). If M is an oriented, compact and connected manifold

(without boundary) and if 

and 

are two volume forms on M having the same

total volume such that



;

then there exists a volume preserving diffeomorphism u of M such that



D u





In other words, the only invariant of volume forms is their total volume.

Proof. We proceed as in the proof of Darboux’s theorem and deform the volume

form 

into 

deﬁning



D .1  t/

C t

;0 t  1:

These forms are volume forms since locally 

and 

are represented by 

.x/ D

a.x/dx

^^dx

and 

.x/ D b.x/dx

^^dx

with non-vanishing smooth

functions a and b which, by the assumption on the total volume, must have the same

sign. We shall construct a family '

of diffeomorphisms satisfying

./.'





D 

D Id

for 0  t  1, so that the diffeomorphism u D '

will solve our problem. Since

M is compact, connected and oriented we conclude from

.

 

/ D 0 that



 

D d

for some .m  1/-form  on M . This is a special case of the de Rham theorem.

Since 

are volume forms, we ﬁnd a unique time dependent vector ﬁeld X

on M

solving the linear equation



D

for 0  t  1. Denote by '

the ﬂow of the vector ﬁeld X

satisfying '

D Id.

Since d˛

D 0 for volume forms we ﬁnd, again using Cartan’s formula,

Œ.'



 D .'







D .'



Œd.i

/ C 

 



which vanishes, because d.i

/ C



D d.i

C/ D 0 by our choice

of the vector ﬁeld X

. Therefore, ./ holds true and the proof is ﬁnished. 