Zee A. Quantum Field Theory in a Nutshell

Подождите немного. Документ загружается.

312 | V. Field Theory and Collective Phenomena

V.7.3 Consider the vortex configuration in which ϕ −→

r→∞

iνθ

, with ν an integer. Calculate the magnetic flux.

Show that the magnetic flux coming out of an antivortex (for which ν =−1) is opposite to the magnetic

flux coming out of a vortex.

V.7.4 Mathematically, since g(θ) ≡ e

iνθ

may be regarded as an element of the group U(1), we can speak of

a map of S

, the circle at spatial infinity, onto the group U(1). Calculate (i/2π)



gdg

†

, thus showing

that the winding number is given by this integral of a 1-form.

V.7.5 Show that within a region in which ϕ

is constant, F

μν

as defined in the text is the electromagnetic field

strength. Compute



B far from the center of a magnetic monopole and show that Dirac quantization

holds.

V.7.6 Display explicitly the map S

→S

, which wraps one sphere around the other twice. Verify that this map

corresponds to a magnetic monopole with magnetic charge 2.

V.7.7 Write down the variational equations that minimize (8).

V.7.8 Find the BPS solution explicitly.

V.7.9 Discuss the dyon solution. Work it out in the BPS limit.

V.7.10 Verify explicitly that the magnetic monopole is rotation invariant in spite of appearances. By this is

meant that all physical gauge invariant quantities such as



B are covariant under rotation. Gauge variant

quantities such as A

can and do vary under rotation. Write down the generators of rotation.

V.7.11 Show that the mass of the magnetic monopole is about 137M

V.7.12 Evaluate n ≡−(1/24π

)



tr(gdg

†

)

for the map g = e



σ

. [Hint: By symmetry, you need calculate the

integrand only in a small neighborhood of the identity element of the group or equivalently the north

pole of S

. Next, consider g = e

i(θ

+θ

+mθ

)

for m an integer and convince yourself that m measures

the number of times S

wraps around S

.] Compare with exercise V.7.4 and admire the elegance of

mathematics.

V.7.13 Prove that higher order corrections do not change the chiral anomaly ∂

= [1/(4π)

]ε

μνλσ

tr F

μν

λσ

(I have rescaled A → (1/g)A). [Hint: Integrate over spacetime and show that the left hand side is given

by the number of right-handed fermion quanta minus the number of left-handed fermion quanta, so

that both sides are given by integers.]

Part VI Field Theory and Condensed Matter

This page intentionally left blank

VI.1

Fractional Statistics, Chern-Simons Term, and

Topological Field Theory

Fractional statistics

The existence of bosons and fermions represents one of the most profound features

of quantum physics. When we interchange two identical quantum particles, the wave

function acquires a factor of either +1or−1. Leinaas and Myrheim, and later Wilczek

independently, had the insight to recognize that in (2 +1)-dimensional spacetime particles

can also obey statistics other than Bose or Fermi statistics, a statistics now known as

fractional or anyon statistics. These particles are now known as anyons.

To interchange two particles, we can move one of them half-way around the other and

then translate both of them appropriately. When you take one anyon half-way around

another anyon going anticlockwise, the wave function acquires a factor of e

iθ

where θ

is a real number characteristic of the particle. For θ = 0, we have bosons and for θ = π ,

fermions. Particles half-way between bosons and fermions, with θ = π/2, are known as

semions.

After Wilczek’s paper came out, a number of distinguished senior physicists were thor-

oughly confused. Thinking in terms of Schr

odinger’s wave function, they got into endless

arguments about whether the wave function must be single valued. Indeed, anyon statis-

tics provides a striking example of the fact that the path integral formalism is sometimes

significantly more transparent. The concept of anyon statistics can be formulated in terms

of wave functions but it requires thinking clearly about the configuration space over which

the wave function is defined.

Consider two indistinguishable particles at positions x

and x

at some initial time

that end up at positions x

and x

a time T later. In the path integral representation

for x

, x

−iHT

, x

 we have to sum over all paths. In spacetime, the worldlines of

the two particles braid around each other (see fig. VI.1.1). (We are implicitly assuming

that the particles cannot go through each other, which is the case if there is a hard core

repulsion between them.) Clearly, the paths can be divided into topologically distinct

classes, characterized by an integer n equal to the number of times the worldlines of the

316 | VI. Field Theory and Condensed Matter

Figure VI.1.1

two particles braid around each other. Since the classes cannot be deformed into each

other, the corresponding amplitudes cannot interfere quantum mechanically, and with

the amplitudes in each class we are allowed to associate an additional phase factor e

iα

beyond the usual factor coming from the action.

The dependence of α

on n is determined by how quantum amplitudes are to be

combined. Suppose one particle goes around the other through an angle ϕ

, a history to

which we assign an additional phase factor e

if (ϕ

)

with f some as yet unknown function.

Suppose this history is followed by another history in which our particle goes around the

other by an additional angle ϕ

. The phase factor e

if (ϕ

+ϕ

)

we assign to the combined

history clearly has to satisfy the composition law e

if (ϕ

+ϕ

)

= e

if (ϕ

)

if (ϕ

)

. In other

words, f (ϕ) has to be a linear function of its argument.

We conclude that in (2 + 1)-dimensional spacetime we can associate with the quantum

amplitude corresponding to paths in which one particle goes around the other anti-

clockwise through an angle ϕ a phase factor e

i(θ/π)ϕ

, with θ an arbitrary real parameter.

Note that when one particle goes around the other clockwise through an angle ϕ the

quantum amplitude acquires a phase factor e

−i

ϕ

When we interchange two anyons, we have to be careful to specify whether we do it

“anticlockwise” or “clockwise,” producing factors e

iθ

and e

−iθ

, respectively. This indicates

immediately that parity P and time reversal invariance T are violated.

Chern-Simons theory

The next important question is whether all this can be incorporated in a local quantum field

theory. The answer was given by Wilczek and Zee, who showed that the notion of fractional

statistics can result from the effect of coupling to a gauge potential. The significance of

VI.1. Topological Field Theory | 317

a field theoretic formulation is that it demonstrates conclusively that the idea of anyon

statistics is fully compatible with the cherished principles that we hold dear and that go

into the construction of quantum field theory.

Given a Lagrangian L

with a conserved current j

, construct the Lagrangian

L = L

+ γε

μνλ

∂

+ a

(1)

Here ε

μνλ

denotes the totally antisymmetric symbol in (2 + 1)-dimensional spacetime and

γ is an arbitrary real parameter. Under a gauge transformation a

→ a

+ ∂

, the term

μνλ

∂

, known as the Chern-Simons term, changes by ε

μνλ

∂

→ ε

μνλ

∂

μνλ

∂

∂

. The action changes by δS = γ



xε

μνλ

∂

(∂

) and thus, if we are

allowed to drop boundary terms, as we assume to be the case here, the Chern-Simons

action is gauge invariant. Note incidentally that in the language of differential form you

learned in chapters IV.5 and IV.6 the Chern-Simons term can be written compactly as ada.

Let us solve the equation of motion derived from (1):

2γε

μνλ

∂

=−j

(2)

for a particle sitting at rest (so that j

= 0). Integrating the μ = 0 component of (2), we

obtain



x(∂

− ∂

) =−

2γ



(3)

Thus, the Chern-Simons term has the effect of endowing the charged particles in the

theory with flux. (Here the term charged particles simply means particles that couple to

the gauge potential a

. In this context, when we refer to charge and flux, we are obviously

not referring to the charge and flux associated with the ordinary electromagnetic field. We

are simply borrowing a useful terminology.)

By the Aharonov-Bohm effect (chapter IV.4), when one of our particles moves around

another, the wave function acquires a phase, thus endowing the particles with anyon

statistics with angle θ =1/4γ (see exercise VI.1.5).

Strictly speaking, the term “fractional statistics” is somewhat misleading. First, a trivial

remark: The statistics parameter θ does not have to be a fraction. Second, statistics is

not directly related to counting how many particles we can put into a state. The statistics

between anyons is perhaps better thought of as a long ranged phase interaction between

them, mediated by the gauge potential a.

The appearance of ε

μνλ

in (1) signals the violation of parity P and time reversal invari-

ance T , something we already know.

Hopf term

An alternative treatment is to integrate out a in (1). As explained in chapter III.4, and as

in any gauge theory, the inverse of the differential operator ε∂ is not defined: It has a zero

mode since (ε

μνλ

∂

)(∂

F(x))= 0 for any smooth function F(x). Let us choose the Lorenz

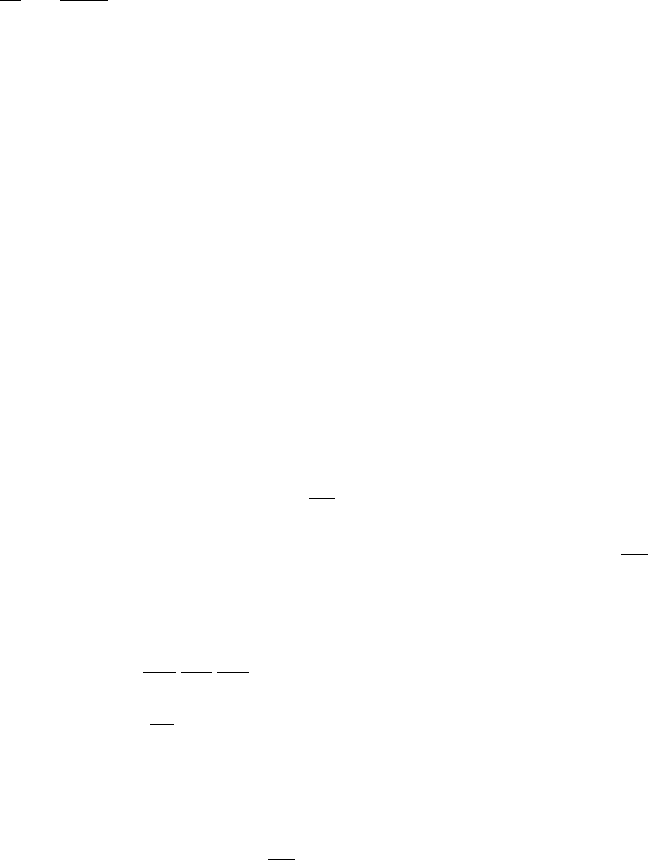
318 | VI. Field Theory and Condensed Matter

gauge ∂

=0. Then, using the fundamental identity of field theory (see appendix A) we

obtain the nonlocal Lagrangian

Hopf

4γ



μνλ

∂



(4)

known as the Hopf term.

To determine the statistics parameter θ consider a history in which one particle moves

half-way around another sitting at rest. The current j is then equal to the sum of two

terms describing the two particles. Plugging into (4) we evaluate the quantum phase

= e



Hopf

and obtain θ =1/(4γ ).

Topological field theory

There is something conceptually new about the pure Chern-Simons theory

S = γ



xε

μνλ

∂

(5)

It is topological.

Recall from chapter I.11 that a field theory written in flat spacetime can be immediately

promoted to a field theory in curved spacetime by replacing the Minkowski metric η

μν

the Einstein metric g

μν

and including a factor

√

−g in the spacetime integration measure.

But in the Chern-Simons theory η

μν

does not appear! Lorentz indices are contracted

with the totally antisymmetric symbol ε

μνλ

. Furthermore, we don’t need the factor

√

−g,

as I will now show. Recall also from chapter I.11 that a vector field transforms as a

(x) =

(∂x

λ

/∂x



) and so for three vector fields

μνλ

(x)b

(x)c

(x) = ε

μνλ

∂x

σ

∂x

τ

∂x

ρ

∂x



)

= det



∂x



∂x



στρ



)

On the other hand, d



= d

x det(∂x



/∂x). Observe, then,

xε

μνλ

(x)b

(x)c

(x) = d



στρ



)

which is invariant without the benefit of

√

−g.

So, the Chern-Simons action in (5) is invariant under general coordinate transforma-

tion—it is already written for curved spacetime. The metric g

μν

does not enter anywhere.

The Chern-Simons theory does not know about clocks and rulers! It only knows about the

topology of spacetime and is rightly known as a topological field theory. In other words,

when the integral in (5) is evaluated over a closed manifold M the property of the field

theory



Dae

iS(a)

depends only on the topology of the manifold, and not on whatever

metric we might put on the manifold.

VI.1. Topological Field Theory | 319

Ground state degeneracy

Recall from chapter I.11 the fundamental definition of energy and momentum. The

energy-momentum tensor is defined by the variation of the action with respect to g

μν

, but

hey, the action here does not depend on g

μν

. The energy-momentum tensor and hence the

Hamiltonian is identically zero! One way of saying this is that to define the Hamiltonian

we need clocks and rulers.

What does it mean for a quantum system to have a Hamiltonian H = 0? Well, when we

took a course on quantum mechanics, if the professor assigned an exam problem to find

the spectrum of the Hamiltonian 0, we could do it easily! All states have energy E =0. We

are ready to hand it in.

But the nontrivial problem is to find how many states there are. This number is known

as the ground state degeneracy and depends only on the topology of the manifold M.

Massive Dirac fermions and the Chern-Simons term

Consider a gauge potential a

coupled to a massive Dirac fermion in (2 + 1)-dimensional

spacetime: L =

ψ(i∂ + a − m)ψ . You did an exercise way back in chapter II.1 discovering

the rather surprising phenomenon that in (2 + 1)-dimensional spacetime the Dirac mass

term violates P and T . (What? You didn’t do it? You have to go back.) Thus, we would expect

to generate the P and T violating the Chern-Simons term ε

μνλ

∂

if we integrate out the

fermion to get the term tr log(i∂ + a −m) in the effective action, along the lines discussed

in chapter IV.3.

In one-loop order we have the vacuum polarization diagram (diagrammatically exactly

the same as in chapter III.7 but in a spacetime with one less dimension) with a Feynman

integral proportional to



(2π)



p + q −m

p − m



(6)

As we will see, the change 4 → 3 makes all the difference in the world. I leave it to you

to evaluate (6) in detail (exercise VI.1.7) but let me point out the salient features here.

Since the ∂

in the Chern-Simons term corresponds to q

in momentum space, in order

to identify the coefficient of the Chern-Simons term we need only differentiate (6) with

respect to q

and set q → 0:



(2π)

tr(γ

p − m

)



(2π)

tr [γ

( p + m)γ

( p + m)]

− m

)

(7)

320 | VI. Field Theory and Condensed Matter

I will simply focus on one piece of the integral, the piece coming from the term in the

trace proportional to m

μνλ



− m

)

(8)

As I remarked in exercise II.1.12, in (2 + 1)-dimensional spacetime the γ

’s are just the

three Pauli matrices and thus tr(γ

) is proportional to ε

μνλ

: The antisymmetric

symbol appears as we expect from P and T violation.

By dimensional analysis, we see that the integral in (8) is up to a numerical constant

equal to 1/m

and so m cancels.

But be careful! The integral depends only on m

and doesn’t know about the sign of m.

The correct answer is proportional to 1/|m|

, not 1/m

. Thus, the coefficient of the Chern-

Simons term is equal to m

/|m|

= m/|m|=sign of m, up to a numerical constant. An

instructive example of an important sign! This makes sense since under P (or T)a Dirac

field with mass m is transformed into a Dirac field with mass −m. In a parity-invariant

theory, with a doublet of Dirac fields with masses m and −m a Chern-Simons term should

not be generated.

Exercises

VI.1.1 In a nonrelativistic theory you might think that there are two separate Chern-Simons terms, ε

∂

and

∂

. Show that gauge invariance forces the two terms to combine into a single Chern-Simons term

μνλ

∂

. For the Chern-Simons term, gauge invariance implies Lorentz invariance. In contrast, the

Maxwell term would in general be nonrelativistic, consisting of two terms, f

and f

, with an arbitrary

relative coefficient between them (with f

μν

= ∂

− ∂

as usual).

VI.1.2 By thinking about mass dimensions, convince yourself that the Chern-Simons term dominates the

Maxwell term at long distances. This is one reason that relativistic field theorists find anyon fluids so

appealing. As long as they are interested only in long distance physics they can ignore the Maxwell

term and play with a relativistic theory (see exercise VI.1.1). Note that this picks out (2 +1)-dimensional

spacetime as special. In (3 + 1)-dimensional spacetime the generalization of the Chern-Simons term

μνλσ

μν

λσ

has the same mass dimension as the Maxwell term f

.In(4 + 1)-dimensional space the

term ε

ρμνλσ

μν

λσ

is less important at long distances than the Maxwell term f

VI.1.3 There is a generalization of the Chern-Simons term to higher dimensional spacetime different from

that given in exercise IV.1.2. We can introduce a p-form gauge potential (see chapter IV.4). Write the

generalized Chern-Simons term in (2p + 1)-dimensional spacetime and discuss the resulting theory.

VI.1.4 Consider L = γaε∂a − (1/4g

. Calculate the propagator and show that the gauge boson is massive.

Some physicists puzzled by fractional statistics have reasoned that since in the presence of the Maxwell

term the gauge boson is massive and hence short ranged, it can’t possibly generate fractional statistics,

which is manifestly an infinite ranged interaction. (No matter how far apart the two particles we are

interchanging are, the wave function still acquires a phase.) The resolution is that the information is

in fact propagated over an infinite range by a q = 0 pole associated with a gauge degree of freedom.

This apparent paradox is intimately connected with the puzzlement many physicists felt when they first

heard of the Aharonov-Bohm effect. How can a particle in a region with no magnetic field whatsoever

and arbitrarily far from the magnetic flux know about the existence of the magnetic flux?

VI.1. Topological Field Theory | 321

VI.1.5 Show that θ = 1/4γ . There is a somewhat tricky factor

of 2. So if you are off by a factor of 2, don’t despair.

Try again.

VI.1.6 Find the nonabelian version of the Chern-Simons term ada. [Hint: As in chapter IV.6 it might be easier

to use differential forms.]

VI.1.7 Using the canonical formalism of chapter I.8 show that the Chern-Simons Lagrangian leads to the

Hamiltonian H = 0.

VI.1.8 Evaluate (6).

X.G. Wen and A. Zee, J. de Physique, 50: 1623, 1989.