Zee A. Quantum Field Theory in a Nutshell
Подождите немного. Документ загружается.


232 | IV. Symmetry and Symmetry Breaking
and π
−
→ π
0
+ e
−
+ ν had been measured. Neutron β decay n → p + e
−
+ ν was of
course the process for which Fermi invented his theory, which by that time had assumed
the form L = G[
eγ
μ
(1 − γ
5
)ν][pγ
μ
(1 − γ
5
)n], where n is a neutron field annihilating a
neutron, p a proton field annihilating a proton, ν a neutrino field annihilating a neutrino
(or creating an antineutrino as in β decay), and e an electron field annihilating an electron.
It became clear that to write down a field for each hadron and a Lagrangian for each
decay process, as theorists were in fact doing for a while, was a losing battle. Instead, we
should write
L = G[eγ
μ
(1 − γ
5
)ν](J
μ
− J
5μ
) (1)
with J
μ
and J
5μ
two currents transforming as a Lorentz vector, and axial vector respectively.
We think of J
μ
and J
5μ
as quantum operators in a canonical formulation of field theory.
Our task would then be to calculate the matrix elements between hadron states, p|(J
μ
−
J
5μ
) |n, 0|(J
μ
− J
5μ
) |π
−
, π
0
|(J
μ
− J
5μ
) |π
−
, and so on, corresponding to the three
decay processes I listed above. (I should make clear that although I am talking about weak
decays, the calculation of these matrix elements is a problem in the strong interaction. In
other words, in understanding these decays, we have to treat the strong interaction to all
orders in the strong coupling, but it suffices to treat the weak interaction to lowest order in
the weak coupling G.) Actually, there is a precedent for the attitude we are adopting here.
To account for nuclear β decay (Z, A) → (Z + 1, A) + e
−
+ ν , Fermi certainly did not
write a separate Lagrangian for each nucleus. Rather, it was the task of the nuclear theorist
to calculate the matrix element Z + 1, A|[
pγ
μ
(1 −γ
5
)n]|Z, A. Similarly, it is the task of
the strong interaction theorist to calculate matrix elements such as p|(J
μ
− J
5μ
) |n.
For the story I am telling, let me focus on trying to calculate the matrix element of
the axial vector current J
μ
5
between a neutron and a proton. Here we make a trivial
change in notation: We no longer indicate that we have a neutron in the initial state and a
proton in the final state, but instead we specify the momentum p of the neutron and the
momentum p
of the proton. Incidentally, in (1) the fields and the currents are of course
all functions of the spacetime coordinates x. Thus, we want to calculate p
|J
μ
5
(x) |p,
but by translation invariance this is equal to p
|J
μ
5
(0) |pe
−i(p
−p)
.
x
. Henceforth, we
simply calculate p
|J
μ
5
(0) |p and suppress the 0. Note that spin labels have already been
suppressed.
Lorentz invariance and parity can take us some distance: They imply that
2
p
|J
μ
5
|p=¯u(p
)[γ
μ
γ
5
F(q
2
) + q
μ
γ
5
G(q
2
)]u(p) (2)
with q ≡p
− p [compare with (III.6.7)]. But Lorentz invariance and parity can only take
us so far: We know nothing about the “form factors” F(q
2
) and G(q
2
).
2
Another possible term of the form (p
+ p)
μ
γ
5
can be shown to vanish by charge conjugation and isospin
symmetries.
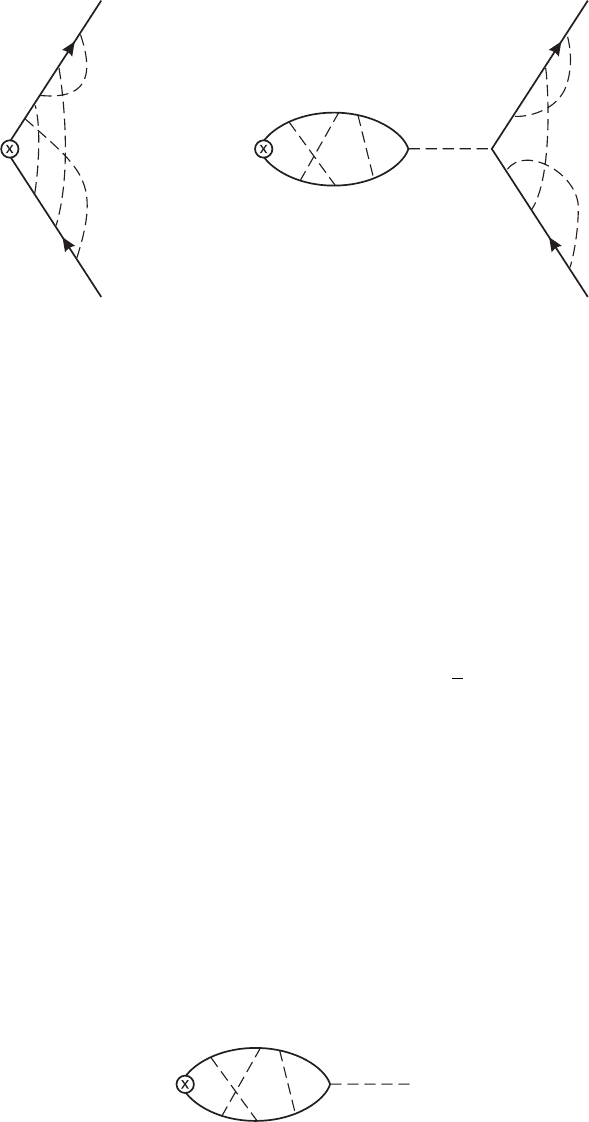
IV.2. Pion as Nambu-Goldstone Boson | 233
(a) (b)
Figure IV.2.1
Similarly, for the matrix element 0|J
μ
5
|π
−
Lorentz invariance tells us that
0|J
μ
5
|k=fk
μ
(3)
I have again labeled the initial state by the momentum k of the pion. The right-hand side
of (3) has to be a vector but since k is the only vector available it has to be proportional
to k. Just like F(q
2
) and G(q
2
), the constant f is a strong interaction quantity that we
don’t know how to calculate. On the other hand, F(q
2
), G(q
2
), and f can all be measured
experimentally. For instance, the rate for the decay π
−
→ e
−
+ ν clearly depends on f
2
.
Too many diagrams
Let us look over the shoulder of a field theorist trying to calculate p
|J
μ
5
|p and 0|J
μ
5
|k
in (2) in the late 1950s. He would draw Feynman diagrams such as the ones in figures IV.2.1
and IV.2.2 and soon realize that it would be hopeless. Because of the strong coupling, he
would have to calculate an infinite number of diagrams, even if the strong interaction were
described by a field theory, a notion already rejected by many luminaries of the time.
Figure IV.2.2
234 | IV. Symmetry and Symmetry Breaking
In telling the story of the breakthrough I am not going to follow the absolutely fascinating
history of the subject, full of total confusion and blind alleys. Instead, with the benefit of
hindsight, I am going to tell the story using what I regard as the best pedagogical approach.
The pion is very light
The breakthrough originated in the observation that the mass of the π
−
at 139 Mev was
considerably less than the mass of the proton at 938 Mev. For a long time this was simply
taken as a fact not in any particular need of an explanation. But eventually some theorists
wondered why one hadron should be so much lighter than another.
Finally, some theorists took the bold step of imagining an “ideal world” in which the
π
−
is massless. The idea was that this ideal world would be a good approximation of our
world, to an accuracy of about 15% (∼139/938).
Do you remember one circumstance in which a massless spinless particle would emerge
naturally? Yes, spontaneous symmetry breaking! In one of the blinding insights that have
characterized the history of particle physics, some theorists proposed that the π mesons
are the Nambu-Goldstone bosons of some spontaneous broken symmetry.
Indeed, let’s multiply (3) by k
μ
:
k
μ
0|J
μ
5
|k=fk
2
= fm
2
π
(4)
which is equal to zero in the ideal world. Recall from our earlier discussion on translation
invariance that
0|J
μ
5
(x) |k=0|J
μ
5
(0) |ke
−ik
.
x
and hence
0|∂
μ
J
μ
5
(x) |k=−ik
μ
0|J
μ
5
(0) |ke
−ik
.
x
Thus, if the axial current is conserved, ∂
μ
J
μ
5
(x) = 0, in the ideal world, k
μ
0|J
μ
5
|k=0
and (4) would indeed imply m
2
π
= 0.
The ideal world we are discussing enjoys a symmetry known as the chiral symmetry
of the strong interaction. The symmetry is spontaneously broken in the ground state we
inhabit, with the π meson as the Nambu-Goldstone boson. The Noether current associated
with this symmetry is the conserved J
μ
5
.
In fact, you should recognize that the manipulation here is closely related to the proof
of the Nambu-Goldstone theorem given in chapter IV.1.
Goldberger-Treiman relation
Now comes the punchline. Multiply (2) by (p
− p)
μ
. By the same translation invariance
argument we just used,
(p
− p)
μ
p
|J
μ
5
(0) |p=ip
|∂
μ
J
μ
5
(x) |pe
i(p
−p)
.
x

IV.2. Pion as Nambu-Goldstone Boson | 235
and hence vanishes if ∂
μ
J
μ
5
= 0. On the other hand, multiplying the right-hand side of
(2) by (p
− p)
μ
we obtain ¯u(p
)[(p
− p)γ
5
F(q
2
) + q
2
γ
5
G(q
2
)]u(p). Using the Dirac
equation (do it!) we conclude that
0 = 2m
N
F(q
2
) + q
2
G(q
2
) (5)
with m
N
the nucleon mass.
The form factors F(q
2
) and G(q
2
) are each determined by an infinite number of
Feynman diagrams we have no hope of calculating, but yet we have managed to relate
them! This represents a common strategy in many areas of physics: When faced with
various quantities we don’t know how to calculate, we can nevertheless try to relate them.
We can go farther by letting q → 0 in (5). Referring to (2) we see that F(0) is measured
experimentally in n → p + e
−
+ ν (the momentum transfer is negligible on the scale of
the strong interaction). But oops, we seem to have a problem: We predict the nucleon mass
m
N
= 0!
In fact, we are saved by examining figure IV.2.1b: There are an infinite number of
diagrams exhibiting a pole due to none other than the massless π meson, which you can
see gives
fq
μ
1
q
2
g
πNN
¯u(p
)γ
5
u(p) (6)
When the π propagator joins onto the nucleon line, an infinite number of diagrams
summed together gives the experimentally measured pion-nucleon coupling constant
g
πNN
. Thus, referring to (2), we see that for q ∼ 0 the form factor G(q
2
) ∼ f(1/q
2
)g
πNN
.
Plugging into (5), we obtain the celebrated Goldberger-Treiman relation
2m
N
F(0) + fg
πNN
= 0 (7)
relating four experimentally measured quantities. As might be expected, it holds with about
a 15% error, consistent with our not living in a world with an exactly massless π meson.
Toward a theory of the strong interaction
The art of relating infinite sets of Feynman diagrams without calculating them, and it is an
art form involving a great deal of cleverness, was developed into a subject called dispersion
relations and S-matrix theory, which we mentioned briefly in chapter III.8. Our present
understanding of the strong interaction was built on this foundation. You could see from
this example that an important component of dispersion relations was the study of the
analyticity properties of Feynman diagrams as described in chapter III.8. The essence of
the Goldberger-Treiman argument is separating the infinite number of diagrams into those
with a pole in the complex q
2
-plane and those without a pole (but with a cut.)
The discovery that the strong interaction contains a spontaneously broken symmetry
provided a crucial clue to the underlying theory of the strong interaction and ultimately
led to the concepts of quarks and gluons.
236 | IV. Symmetry and Symmetry Breaking
A note for the historian of science: Whether theoretical physicists regard a quantity as
small or large depends (obviously) on the cultural and mental framework they grew up
in. Treiman once told me that the notion of setting 138 Mev to zero, when the energy
released per nucleon in nuclear fission is of order 10 Mev, struck the generation that grew
up with the atomic bomb (as Treiman did—he was with the armed forces in the Pacific) as
surely the height of absurdity. Now of course a new generation of young string theorists
is perfectly comfortable in regarding anything less than the Planck energy 10
19
Gev as
essentially zero.

IV.3 Effective Potential
Quantum fluctuations and symmetry breaking
The important phenomenon of spontaneous symmetry breaking was based on minimizing
the classical potential energy V(ϕ)of a quantum field theory. It is natural to wonder how
quantum fluctuations would change this picture.
To motivate the discussion, consider once again (III.3.3)
L =
1
2
(∂ϕ)
2
−
1
2
μ
2
ϕ
2
−
1
4!
λϕ
4
+ A(∂ϕ)
2
+ Bϕ
2
+ Cϕ
4
(1)
(Speaking of quantum fluctuations, we have to include counterterms as indicated.) What
have you learned about this theory? For μ
2
> 0, the action is extremized at ϕ = 0, and
quantizing the small fluctuations around ϕ = 0 we obtain scalar particles that scatter off
each other. For μ
2
< 0, the action is extremized at some ϕ
min
, and the discrete symmetry
ϕ →−ϕ is spontaneously broken, as you learned in chapter IV.1. What happens when
μ = 0? To break or not to break, that is the question.
A quick guess is that quantum fluctuations would break the symmetry. The μ = 0 theory
is posed on the edge of symmetry breaking, and quantum fluctuations ought to push it
over the brink. Think of a classical pencil perfectly balanced on its tip. Then “switch on”
quantum mechanics.
Wisdom of the son-in-law
Let us follow Schwinger and Jona-Lasinio and develop the formalism that enables us to
answer this question. Consider a scalar field theory defined by
Z = e
iW (J )
=
Dϕe
i[S(ϕ)+Jϕ]
(2)
[with the convenient shorthand Jϕ =
d
4
xJ (x)ϕ(x)]. If we can do the functional integral,
we obtain the generating functional W(J). As explained in chapter I.7, by differentiating

238 | IV. Symmetry and Symmetry Breaking
W with respect to the source J(x) repeatedly, we can obtain any Green’s function and
hence any scattering amplitude we want. In particular,
ϕ
c
(x) ≡
δW
δJ (x)
=
1
Z
Dϕe
i[S(ϕ)+Jϕ]
ϕ(x) (3)
The subscript c is used traditionally to remind us (see appendix 2 in chapter I.8) that in a
canonical formalism ϕ
c
(x) is the expectation value 0|ˆϕ |0 of the quantum operator ˆϕ.It
is certainly not to be confused with the integration dummy variable ϕ in (3). The relation
(3) determines ϕ
c
(x) as a functional of J .
Given a functional W of J we can perform a Legendre transform to obtain a functional
of ϕ
c
. Legendre transform is just the fancy term for the simple relation
(ϕ
c
) = W(J)−
d
4
xJ (x)ϕ
c
(x) (4)
The relation is simple, but be careful about what it says: It defines a functional of ϕ
c
(x)
through the implicit dependence of J on ϕ
c
. On the right-hand side of (4) J is to be
eliminated in favor of ϕ
c
by solving (3). We expand the functional (ϕ
c
) in the form
(ϕ
c
) =
d
4
x[−V
eff
(ϕ
c
) + Z(ϕ
c
)(∂ϕ
c
)
2
+
...
] (5)
where (
...
) indicates terms with higher and higher powers of ∂ . We will soon see the
wisdom of the notation V
eff
(ϕ
c
).
The point of the Legendre transform is that the functional derivative of is nice and
simple:
δ(ϕ
c
)
δϕ
c
(y)
=
d
4
x
δJ (x)
δϕ
c
(y)
δW (J )
δJ (x)
−
d
4
x
δJ (x)
δϕ
c
(y)
ϕ
c
(x) − J(y)
=−J(y) (6)
a relation we can think of as the “dual” of δW (J )/δJ (x) = ϕ
c
(x).
If you vaguely feel that you have seen this sort of manipulation before in your physics
eduction, you are quite right! It was in a course on thermodynamics, where you learned
about the Legendre transform relating the free energy to the energy: F = E − TS with
F a function of the temperature T and E a function of the entropy S. Thus J and
ϕ are “conjugate” pairs just like T and S (or even more clearly magnetic field H and
magnetization M). Convince yourself that this is far more than a mere coincidence.
For J and ϕ
c
independent of x we see from (5) that the condition (6) reduces to
V
eff
(ϕ
c
) = J (7)
This relation makes clear what the effective potential V
eff
(ϕ
c
) is good for. Let’s ask what
happens when there is no external source J . The answer is immediate: (7) tells us that
V
eff
(ϕ
c
) = 0, (8)
IV.3. Effective Potential | 239
In other words, the vacuum expectation value of ˆϕ in the absence of an external source is
determined by minimizing V
eff
(ϕ
c
).
First order in quantum fluctuations
All of these formal manipulations are not worth much if we cannot evaluate W(J).In
fact, in most cases we can only evaluate e
iW (J )
=
Dϕe
i[S(ϕ)+Jϕ]
in the steepest descent
approximation (see chapter I.2). Let us turn the crank and find the steepest descent “point”
ϕ
s
(x), namely the solution of (henceforth I will drop the subscript c as there is little risk
of confusion)
δ[S(ϕ) +
d
4
yJ (y)ϕ(y)]
δϕ(x)
ϕ
s
= 0 (9)
or more explicitly,
∂
2
ϕ
s
(x) + V
[ϕ
s
(x)] =J(x) (10)
Write the dummy integration variable in (2) as ϕ = ϕ
s
+ϕ and expand to quadratic order
in ϕ to obtain
Z = e
(i/)W (J )
=
Dϕe
(i/)[S(ϕ)+Jϕ]
e
(i/)[S(ϕ
s
)+Jϕ
s
]
Dϕe
(i/)
d
4
x
1
2
[(∂ϕ)
2
−V
(ϕ
s
)ϕ
2
]
= e
(i/)[S(ϕ
s
)+Jϕ
s
]−
1
2
tr log[∂
2
+V
(ϕ
s
)]
(11)
We have used (II.5.2) to represent the determinant we get upon integrating over ϕ. Note
that I have put back Planck’s constant . Here ϕ
s
, as a solution of (10), is to be regarded as
a function of J .
Now that we have determined
W(J) = [S(ϕ
s
) + Jϕ
s
] +
i
2
tr log[∂
2
+ V
(ϕ
s
)] +O(
2
)
it is straightforward to Legendre transform. I will go painfully slowly here:
ϕ =
δW
δJ
=
δ[S(ϕ
s
) + Jϕ
s
]
δϕ
s
δϕ
s
δJ
+ ϕ
s
+ O() = ϕ
s
+ O()
To leading order in , ϕ (namely the object formerly known as ϕ
c
) is equal to ϕ
s
. Thus,
from (4) we obtain
(ϕ) =S(ϕ) +
i
2
tr log[∂
2
+ V
(ϕ)] + O(
2
) (12)
Nice though this formula looks, in practice it is impossible to evaluate the trace for
arbitrary ϕ(x) : We have to find all the eigenvalues of the operator ∂
2
+ V
(ϕ), take their
log, and sum. Our task simplifies drastically if we are content with studying (ϕ) for
240 | IV. Symmetry and Symmetry Breaking
ϕ independent of x, in which case V
(ϕ) is a constant and the operator ∂
2
+ V
(ϕ) is
translation invariant and easily treated in momentum space:
tr log[∂
2
+ V
(ϕ)] =
d
4
xx| log[∂
2
+ V
(ϕ)]|x
=
d
4
x
d
4
k
(2π)
4
x|kk| log[∂
2
+ V
(ϕ)]|kk|x
=
d
4
x
d
4
k
(2π)
4
log[−k
2
+ V
(ϕ)] (13)
Referring to (5), we obtain
V
eff
(ϕ) = V(ϕ)−
i
2
d
4
k
(2π)
4
log
k
2
− V
(ϕ)
k
2
+ O(
2
) (14)
known as the Coleman-Weinberg effective potential. What we computed is the order
correction to the classical potential V(ϕ). Note that we have added a ϕ independent constant
to make the argument of the logarithm dimensionless.
We can give a nice physical interpretation of (14). Let the universe be suffused with
the scalar field ϕ(x) taking on the value ϕ, a background field so to speak. For V(ϕ)=
1
2
μ
2
ϕ
2
+ (1/4!)λϕ
4
, we have V
(ϕ) = μ
2
+
1
2
λϕ
2
≡ μ(ϕ)
2
, which, as the notation μ(ϕ)
2
suggests, we recognize as the ϕ-dependent effective mass squared of a scalar particle
propagating in the background field ϕ. The mass squared μ
2
in the Lagrangian is corrected
by a term
1
2
λϕ
2
due to the interaction of the particle with the background field ϕ. Now we
see clearly what (14) tells us: The first term V(ϕ)is the classical energy density contained
in the background ϕ, while the second term is the vacuum energy density of a scalar field
with mass squared equal to V
(ϕ) [see (II.5.3) and exercise IV.3.4].
Your renormalization theory at work
The integral in (14) is quadratically divergent, or more correctly, quadratically dependent
on the cutoff. But no sweat, we were instructed to introduce three counterterms (of which
only two are relevant here since ϕ is independent of x). Thus, we actually have
V
eff
(ϕ) = V(ϕ)+
2
d
4
k
E
(2π)
4
log
k
2
E
+ V
(ϕ)
k
2
E
+ Bϕ
2
+ Cϕ
4
+ O(
2
) (15)
where we have Wick rotated to a Euclidean integral (see appendix D). Using (D.9) and
integrating up to k
2
E
=
2
, we obtain (suppressing )
V
eff
(ϕ) = V(ϕ)+
2
32π
2
V
(ϕ) −
[V
(ϕ)]
2
64π
2
log
e
1
2
2
V
(ϕ)
+ Bϕ
2
+ Cϕ
4
(16)
As expected, since the integrand in (15) goes as 1/k
2
E
for large k
2
E
the integral depends
quadratically and logarithmically on the cutoff
2
.

IV.3. Effective Potential | 241
Watch renormalization theory at work! Since V is a quartic polynomial in ϕ , V
(ϕ) is a
quadratic polynomial and [V
(ϕ)]
2
a quartic polynomial. Thus, we have just enough coun-
terterms Bϕ
2
+ Cϕ
4
to absorb the cutoff dependence. This is a particularly transparent
example of how the method of adding counterterms works.
To see how bad things can happen in a nonrenormalizable theory, suppose in contrast
that V is a polynomial of degree 6 in ϕ. Then we are allowed to have three counterterms
Bϕ
2
+ Cϕ
4
+ Dϕ
6
, but that is not enough since [V
(ϕ)]
2
is now a polynomial of degree
8. This means that we should have started out with V a polynomial of degree 8, but then
[V
(ϕ)]
2
would be a polynomial of degree 12. Clearly, the process escalates into an infinite-
degree polynomial. We see the hallmark of a nonrenormalizable theory: its insatiable
appetite for counterterms.
Imposing renormalization conditions
Waking up from the nightmare of an infinite number of counterterms chasing us, let us
go back to the sweetly renormalizable ϕ
4
theory. In chapter III.3 we fix the counterterms
by imposing conditions on various scattering amplitudes. Here we would have to fix the
coefficients B and C by imposing two conditions on V
eff
(ϕ) at appropriate values of ϕ.We
are working in field space, so to speak, rather than momentum space, but the conceptual
framework is the same.
We could proceed with the general quartic polynomial V(ϕ), but instead let us try to
answer the motivating question of this chapter: What happens when μ = 0, that is, when
V(ϕ)= (1/4!)λϕ
4
? The arithmetic is also simpler.
Evaluating (16) we get
V
eff
(ϕ) = (
2
64π
2
λ + B)ϕ
2
+ (
1
4!
λ +
λ
2
(16π)
2
log
ϕ
2
2
+ C)ϕ
4
+ O(λ
3
)
(after absorbing some ϕ-independent constants into C). We see explicitly that the
dependence can be absorbed into B and C.
We started out with a purely quartic V(ϕ). Quantum fluctuations generate a quadrati-
cally divergent ϕ
2
term that we can cancel with the B counterterm. What does μ =0 mean?
It means that (d
2
V/dϕ
2
)|
ϕ=0
vanishes. To say that we have a μ = 0 theory means that we
have to maintain a vanishing renormalized mass squared, defined here as the coefficient
of ϕ
2
. Thus, we impose our first condition
d
2
V
eff
dϕ
2
ϕ=0
= 0 (17)
This is a somewhat long-winded way of saying that we want B =−(
2
/64π
2
)λ to this
order.
Similarly, we might think that the second condition would be to set (d
4
V
eff
/dϕ
4
)|
ϕ=0
equal to some coupling, but differentiating the ϕ
4
log ϕ term in V
eff
four times we are
going to get a term like log ϕ, which is not defined at ϕ = 0. We are forced to impose our
