Zee A. Quantum Field Theory in a Nutshell

Подождите немного. Документ загружается.

212 | III. Renormalization and Gauge Invariance

Decay and distintegration

At this point you might already be flipping back to the expression given in chapter II.6 for

the decay rate of a particle. Earlier we entertained the suspicion that the imaginary part of

(z) corresponds to decay. To confirm our suspicion, let us first go back to elementary

quantum mechanics. The higher energy levels in a hydrogen atom, say, are, strictly

speaking, not eigenstates of the Hamiltonian: an electron in a higher energy level will, in a

finite time, emit a photon and jump to a lower energy level. Phenomenologically, however,

the level could be assigned a complex energy E − i

. The probability of staying in this

level then goes with time like |ψ(t)|

∝|e

−i(E−i

)t

= e

−t

. (Note that in elementary

quantum mechanics, the Coulomb and radiation components of the electromagnetic field

are treated separately: the former is included in the Schr

odinger equation but not the latter.

One of the aims of quantum field theory is to remedy this artificial split.)

We now go back to (5) and field theory: note that (q

) effectively shifts M

→ M

−

(q

). Recall from (III.3.3) that we have counter terms available to, well, counter two

cutoff-dependent pieces of (q

). But we have nothing to counter the imaginary part of

(q

) with, and so it better be cutoff independent. Indeed it is! The cutoff only appears in

the real part in (7).

We conclude that the effect of  going imaginary is to shift the mass of the ϕ meson

by a cutoff-independent amount from M to



− iIm(M

) ≈ M −iIm(M

)/(2M).

Note that to order g

it suffices to evaluate  at the unshifted mass squared M

, since

the shift in mass is itself of order g

. Thus  = Im(M

)/M gives the decay rate, as we

suspected. We obtain (g has dimension of mass and so the dimension is correct)

 =

16πM



− (m + μ)

][M

− (m − μ)

] (9)

precisely what we had in (II.6.7). You and I could both take a bow for getting all the factors

exactly right!

Note that both the treatment given in elementary quantum mechanics and here are in

the spirit of treating the decay as a small perturbation. As the width becomes large, at some

point it no longer makes good sense to talk of the field associated with the particle ϕ.

Taking the imaginary part directly

We ought to be able to take the imaginary part of the Feynman integral in (6) directly, rather

than having to first calculate it as an integral over the Feynman parameter α. I will now

show you how to do this using a trick. For clarity and convenience, change notation from

(6), label the momentum carried by the two internal lines in figure III.8.4a separately, and

restore the momentum conservation delta function, so that

i(q) = (ig)



(2π)

+ k

− q)



− m

+ i

− m

+ i



(10)

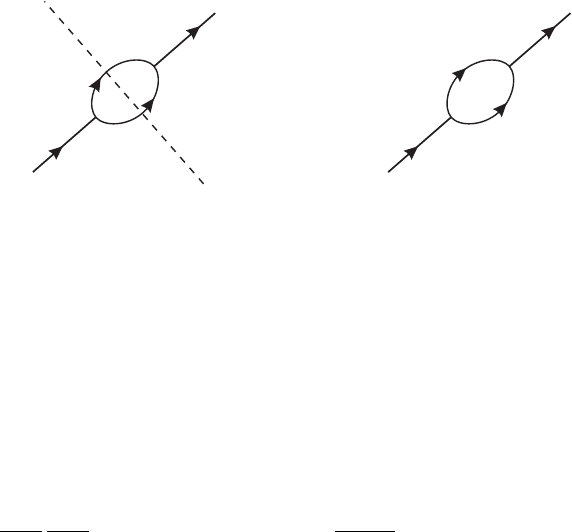
III.8. Becoming Imaginary | 213

␰

␩

␸

␰

␩

(b)(a)

Figure III.8.4

Write the propagator as 1/(k

− m

+ i) = P(1/(k

− m

)) − iπδ(k

− m

) and, noting

an explicit overall factor of i, take the real part of the curly bracket above, thus obtaining

Im(q) =−g



d(P

− 



) (11)

For the sake of compactness, we have introduced the notation

d =

(2π)

+ k

− q), P

= P

− m

, 

= πδ(k

− m

)

and so on.

We welcome the product of two delta functions; they are what we want, restricting the

two particles η and ξ on shell. But yuck, what do we do with the product of the two principal

values? They don’t correspond to anything too physical that we know of.

To get rid of the two principal values, we use a trick.

First, we regress and recall that

we started out with Feynman diagrams as spacetime diagrams (for example, fig. I.7.6) of

the process under study. Here (fig. III.8.4b) a ϕ excitation turns into an η and a ξ with

amplitude ig at some spacetime point, which by translation invariance we could take to be

the origin; the η and the ξ excitations propagate to some point x with amplitude iD

(x)

and iD

(x), respectively, and then recombine into ϕ with amplitude ig (note: not −ig).

Fourier transforming this product of spacetime amplitudes gives

i(q) = (ig)



dxe

−iqx

(x)D

(x) (12)

To see that this is indeed the same as (10), all you have to do is to plug in the expression

(I.3.22) for D

(x) and D

(x).

Incidentally, while many “professors of Feynman diagrams” think almost exclusively

in momentum space, Feynman titled his 1949 paper “Space-Time Approach to Quantum

Electrodynamics,” and on occasions it is useful to think of the spacetime roots of a given

Feynman diagram. Now is one of those occasions.

C. Itzkyson and J.-B. Zuber, Quantum Field Theory, p. 367.

214 | III. Renormalization and Gauge Invariance

Next, go back to exercise I.3.3 and recall that the advanced propagator D

adv

(x) and

retarded propagator D

ret

(x) vanish for x

< 0 and x

> 0, respectively, and thus the product

adv

(k)D

ret

(k) manifestly vanishes for all x. Also recall that the advanced and retarded

propagators D

adv

(k) and D

ret

(k) differ from the Feynman propagator D(k) by simply, but

crucially, having their poles in different half-planes in the complex k

plane. Thus

0 =−ig



dxe

−iqx

η, adv

(x)D

ξ ,ret

(x)



(2π)

+ k

− q)

− m

− iσ



− m

+ iσ



(13)

where we used the shorthand σ

=sgn(k

) and σ

=sgn(k

). (The sign function is defined

by sgn(x) =±1 according to whether x>0or< 0.) Taking the imaginary part of 0, we

obtain [compare (11)]

0 =−g



d(P

+ σ



) (14)

Subtracting (14) from (11) to get rid of the rather unpleasant term P

, we find finally

Im(q) =+g



d(1 + σ

)(



)

= g



(2π)

+ k

− q)θ(k

)δ(k

− m

)θ(k

)δ(k

− m

)(1 + σ

)

(15)

Thus (q) develops an imaginary part only when the three delta functions can be satisfied

simultaneously.

To see what these three conditions imply, we can, since (q) is a function of q

,go

to a frame in which q = (Q,



0) with Q>0 with no loss of generality. Since k

+ k

Q>0 and since (1 + σ

) vanishes unless k

and k

have the same sign, k

and k

must be both positive if Im(q) is to be nonzero, but that is already mandated by the

two step functions. Furthermore, we need to solve the conservation of energy condition

Q =





+ m





+ m

for some 3-vector



k. This is possible only if Q>m

+ m

,in

which case, using the identity (I.8.14)

θ(k

)δ(k

− μ

) = θ(k

)

δ(k

− ε

)

2ε

, (16)

we obtain

Im(q) =



(2π)

2ω

(2π)

2ω

(2π)

+ k

− q) (17)

We see that (and as we will see more generally) Im(q) works out to be a finite integral

over delta functions. Indeed, no counter term is needed.

Some readers might feel that this trick of invoking the advanced and retarded propa-

gators is perhaps a bit “too tricky.” For them, I will show a more brute force method in

appendix 1.

III.8. Becoming Imaginary | 215

Unitarity and the Cutkosky cutting rule

The simple example we just went through in detail illustrates what is known as the

Cutkosky cutting rule, which states that to calculate the imaginary part of a Feynman

amplitude we first “cut” through a diagram (as indicated by the dotted line in figure II.8.4a).

For each internal line cut, replace the propagator 1/(k

− m

+ i) by δ(k

−m

); in other

words, put the virtual excitation propagating through the cut onto the mass shell. Thus,

in our example, we could jump from (10) to (15) directly. This validates our intuition that

Feynman amplitudes go imaginary when virtual particles can “get real.”

For a precise statement of the cutting rule, see below. (The Cutkosky cut is not be

confused with the Cauchy cut in the complex plane, of course.)

The Cutkosky cutting rule in fact follows in all generality from unitarity. A basic postulate

of quantum mechanics is that the time evolution operator e

−iHT

is unitary and hence

preserves probability. Recall from chapter I.8 that it is convenient to split off from the

S matrix S

=f |e

−iHT

|i the piece corresponding to “nothing is happening”: S = I +

iT . Unitarity S

†

S = I then implies 2 Im T = i(T

†

−T)= T

†

T . Sandwiching this between

initial and final states and inserting a complete set of intermediate states (1 =



|nn|)

we have

2ImT



†

(18)

which some readers might recognize as a generalization of the optical theorem from

elementary quantum mechanics.

It is convenient to introduce F =−iM. (We are merely taking out an explicit factor of

i in M: in our simple example, M corresponds to i, F to .) Then the relation (I.8.16)

between T and M becomes T

= (2π)

(4)

)(

1/ρ)F (f ← i), where for the sake

of compactness we have introduced some obvious notations [thus (

) denotes the

product of the normalization factors 1/ρ (see chapter I.8), one for each of the particle in

the state i and in the state f , and P

the sum of the momenta in f minus the sum of the

momenta in i.]

With this notation, the left-hand side of the generalized optical theorem becomes

2ImT

= 2(2π)

(4)

)(

1/ρ)Im F (f ← i) and the right-hand side



†



(2π)

(4)

)(2π)

(4)

)











(F (n ← f))

∗

F (n ← i)

The product of two delta functions δ

(4)

)δ

(4)

) = δ

(4)

)δ

(4)

), and thus we

could cancel off δ

(4)

). Also (

1/ρ)(

1/ρ)/(

1/ρ) = (

1/ρ

), and we happily

recover the more familiar factor ρ

[namely (2π)

2ω for bosons]. Thus finally, the gener-

alized optical theorem tells that

2ImF (f ←i) =



(2π)

(4)

)







(F (n ← f))

∗

F (n ← i), (19)

216 | III. Renormalization and Gauge Invariance

namely, that the imaginary part of the Feynman amplitude F (f ←i) is given by a sum

of (F (n ← f))

∗

F (n ← i) over intermediate states |n. The particles in the intermediate

state are of course physical and on shell. We are to sum over all possible states |n allowed

by quantum numbers and by the kinematics.

According to Cutkosky, given a Feynman diagram, to obtain its imaginary part, we

simply cut through it in the several different ways allowed, corresponding to the different

possible intermediate state |n. Particles in the state |n are manifestly real, not virtual.

Note that unitarity and hence the optical theorem are nonlinear in the transition am-

plitude. This has proved to be enormously useful in actual computation. Suppose we are

perturbing in some coupling g and we know F (n ← i) and F (n ← f)to order g

. The op-

tical theorem gives us Im F (f ← i) to order g

N+1

, and we could then construct F (f ←i)

to order g

N+1

using a dispersion relation.

The application of the Cutkosky rule to the vacuum polarization function discussed in

this chapter is particularly simple: there is only one possible way of cutting the Feynman

diagram for . Here the initial and final states |iand |f both consist of a single ϕ meson,

while the intermediate state |n>consists of an η and a ξ meson.

Referring to the appendix to chapter II.6, we recall that



corresponds to



(2π)

Thus the optical theorem as stated in (19) says that

Im(q) =



(2π)

2ω

(2π)

2ω

(2π)

+ k

− q) (20)

precisely what we obtained in (17). You and I could take another bow, since we even get

the factor of 2 correctly (as we must!).

We should also say, in concluding this chapter, “Vive Cauchy!”

Appendix 1: Taking the imaginary part by brute force

For those readers who like brute force, we will extract the imaginary part of

 =−ig



(2π)

− μ

+ iε

(q − k)

− m

+ iε

(21)

by a more staightforward method, as promised in the text. Since  depends only on q

, we have the luxury

of setting q = (M ,



0). We already know that in the complex M

plane,  has a cut on the axis starting at

= (m + μ)

. Let us verify this by brute force.

We could restrict ourselves to M>0. Factorizing, we find that the denominator of the integrand is a product of

four factors, k

−(ε

−iε), k

+(ε

−iε), k

−(M + E

−iε), and k

−(M − E

+iε), and thus the integrand

has four poles in the complex k

plane. (Evidently, ε





+ μ

, E





+ m

, and if you are perplexed over

the difference between ε

and ε, then you are hopelessly confused.) We now integrate over k

, choosing to close

the contour in the lower half plane and going around picking up poles. Picking up the pole at ε

−iε, we obtain



=−g



k/(2π)

)(1/(2ε

(ε

− M −E

)(ε

− M +E

))). Picking up the pole at M +E

− iε, we obtain



=−g



k/(2π)

)(1/((M + E

− ε

)(M +E

+ ε

)(2E

))). We now regard  = 

+

as a function of

 =−g



(2π)

(M + E

− ε

)



2ε

(M − ε

− E

+ iε)

(M + E

+ ε

− iε)



(22)

III.8. Becoming Imaginary | 217

In spite of appearances, there is no pole at M ≈ ε

−E

. (Since this pole would lead to a cut at μ −m, there better

not be!) For M>0 we only care about the pole at M ≈ε





+ μ





+ m

. When we integrate over



k, this pole gets smeared into a cut starting at m + μ. So far so good.

To calculate the discontinuity across the cut, we use the identity (16) once again Restoring the iε’s and throwing

away the term we don’t care about, we have effectively

 =−g



(2π)

2ε

(ε

− M − E

+ iε)(ε

− M + E

− iε)

=−2πg



(2π)

θ(k

)δ(k

− μ

)

(M − ε

+ E

− iε)(M −(ε

+ E

) + iε)

The discontinuity of  across the cut just specified is determined by applying (I.2.13) to the factor

1/(M − (ε

+ E

) + iε), giving Im = 2π



k/(2π)

)θ(k

)δ(k

− μ

)δ(M −(ε

+ E

))/2E

. Use the

identity (16) again in the form

θ(q

− k

)θ((q − k)

− m

) = θ(q

− k

)

θ((q

− k

) − E

)

(23)

and we obtain

Im = 2π



(2π)

θ(k

)δ(k

− μ

)θ(q

− k

)δ((q −k)

− m

) (24)

Remarkably, as Cutkosky taught us, to obtain the imaginary part we simply replace the propagators in (21) by

delta functions.

Appendix 2: A dispersion representation for the two-point amplitude

I would like to give you a bit more flavor of the dispersion program once active and now being revived (see

part N). Consider the two-point amplitude iD(x) ≡0|T(O(x)O(0)) |0, with O(x) some operator in the canonical

formalism. For example, for O(x) equal to the field ϕ(x), D(x) would be the propagator. In chapter I.8, we were

able to evaluate D(x) for a free field theory, because then we could solve the field equation of motion and expand

ϕ(x) in terms of creation and annihilation operators. But what can we do in a fully interacting field theory? There

is no hope of solving the operator field equation of motion.

The goal of the dispersion program of the 1950s and 1960s is to say as much as possible about D(x) based on

general considerations such as analyticity.

OK, so first write iD(x) = θ(x

)0|e

iPx

O(0)e

−iPx

O(0) |0+θ(−x

)0|O(0)e

iPx

O(0)e

−iPx

|0, where we

used spacetime translation O(x) = e

O(0)e

−iP

. By the way, if you are not totally sure of this relation, dif-

ferentiate it to obtain ∂

O(x) = i[P

, O(x)] which you should recognize as the relativistic version of the usual

Heisenberg equations (I.8.2, 3). Now insert 1=



|nn|, with |na complete set of intermediate states, to obtain

0|e

iPx

O(0)e

−iPx

O(0) |0=0|O(0)e

−iPx

O(0) |0=



0|O(0) |nn|e

−iPx

O(0) |0=



−iP

, where

we used P

|0=0 and P

|n=P

|n and defined O

≡0|O(0) |n. Next, use the integral representations

for the step function θ(t) =−i



(dω/2π)e

iωt

/(ω − iε) and θ(−t) =i



(dω/2π)e

iωt

/(ω + iε). Again, if you are

not sure of this, simply differentiate

θ(t)=−i



(dω/2π)e

iωt

/(ω − iε) =



(dω/2π)e

iωt

, which you recog-

nize from (I.2.12) as indeed the integral representation of the delta function δ(t) =

θ(t). In other words, the

representation used here is the integral of the representation in (I.2.12).

Putting it all together, we obtain

iD(q) =



iD(x) =−i(2π)





(3)

(q −



)

− q

− iε

(3)

(q +



)

+ q

− iε



. (25)

The integral over d

x produced the 3-dimensional delta function, while the integral over dx

=dt picked up the

denominator in the integral representation for the step function.

Now take the imaginary part using Im1/(P

− q

− iε) =πδ(q

− P

). We thus obtain

Im(i



iqx

0|T(O(x)O(0)) |0) = π(2π)



(δ

(4)

(q − P

) + δ

(4)

(q + P

)) (26)

218 | III. Renormalization and Gauge Invariance

with the more pleasing 4-dimensional delta function. Note that for q

> 0 the term involving δ

(4)

(q + P

) drops

out, since the energies of physical states must be positive.

What have we accomplished? Even though we are totally incapable of calculating D(q), we have managed to

represent its imaginary part in terms of physical quantities that are measurable in principle, namely the absolute

square |O

of the matrix element of O(0) between the vacuum state and the state |n. For example, if O(x) is

the meson field ϕ(x) in a ϕ

theory, the state |n would consist of the single-meson state, the three-meson state,

and so on. The general hope during the dispersion era was that by keeping a few states we could obtain a decent

approximation to D(q). Note that the result does not depend on perturbing in some coupling constant.

The contribution of the single meson state |



k has a particularly simple form, as you might expect.

With our normalization of single-particle states (as in chapter I.8), Lorentz invariance implies 



k|O(0) |0=



(2π)

2ω

, with ω





+ m

and Z

an unknown constant, measuring the “strength” with which O is

capable of producing the single meson from the vacuum. Putting this into (25) and recognizing that the sum

over single-meson states is now given by



k |



k



k| [with the normalization 







k=δ

(





−



k)], we find that

the single-meson contribution to iD(q) is given by

−i(2π)



(2π)

2ω



(3)

(q −



− q

− iε

+ (q →−q)



=−i

2ω



− q

− iε

+ (q →−q)



− m

+ iε

(27)

This is a very satisfying result: even though we cannot calculate iD(q), we know that it has a pole at a position

determined by the meson mass with a residue that depends on how O is normalized.

As a check, we can also easily calculate the contribution of the single-meson state to −ImD(q). Plugging

into (26), we find, for q

> 0, πZ



k/2ω

)δ

(q − k) = (π Z/2ω

)δ(q

−ω

) = πZδ(q

−m

), where we used

(I.8.14, 16) in the last step.

Given our experience with the vacuum polarization function, we would expect D(q) (which by Lorentz

invariance is a function of q

) to have a cut starting at q

=(3m)

. To verify this, simply look at (26) and choose q =

0. The contribution of the three-meson state occurs at



“3”





+ m





+ m





+ m

≥

3m. The sum over states is now a triple integration over



, and



, subject to the constraint



= 0.

Knowing the imaginary part of D(q) we can now write a dispersion relation of the kind in (3).

Finally, if you stare at (26) long enough (see exercise III.8.3) you will discover the relation

Im(i



iqx

0|T(O(x)O(0)) |0) =



iqx

0|[O(x), O(0)] |0 (28)

The discussion here is relevant to the discussion of field redefinition in chapter I.8. Suppose our friend uses

η = Z

ϕ + αϕ

instead of ϕ; then the present discussion shows that his propagator



iqx

0|T (η(x)η(0)) |0

still has a pole at q

− m

. The important point is that physics fixes the pole to be at the same location.

Here we have taken O to be a Lorentz scalar. In applications (see chapter VII.3) the role of O is often played

by the electromagnetic current J

(x) (treated as an operator). The same discussion holds except that we have

to keep track of some Lorentz indices. Indeed, we recognize that the vacuum polarization function 

μν

then

corresponds to the function D in this discussion.

Exercises

III.8.1 Evaluate the imaginary part of the vacuum polarization function, and by explicit calculation verify that it

is related to the decay rate of a vector particle into an electron and a positron.

III.8.2 Suppose we add a term gϕ

to our scalar ϕ

theory. Show that to order g

there is a “box diagram”

contributing to meson scattering p

+ p

→ p

+ p

with the amplitude

I =g



(2π)

− m

− iε)((k + p

)

− m

− iε)((k − p

)

− m

− iε)((k + p

− p

)

− m

− iε)

III.8. Becoming Imaginary | 219

Calculate the integral explicitly as a function of s =(p

+ p

)

and t = (p

− p

)

. Study the analyticity

property of I as a function of s for fixed t. Evaluate the discontinuity of I across the cut and verify

Cutkosky’s cutting rule. Check that the optical theorem works. What about the analyticity property of I

as a function of t for fixed s? And as a function of u = (p

− p

)

III.8.3 Prove (28). [Hint: Do unto



iqx

0|[O(x), O(0)]|0 what we did to



iqx

0||T(O(x)O(0)) |0,

namely, insert 1 =



|nn| (with |n a complete set of states) between O(x) and O(0) in the commu-

tator. Now we don’t have to bother with representing the step function.]

This page intentionally left blank

Part IV Symmetry and Symmetry Breaking