Zee A. Quantum Field Theory in a Nutshell
Подождите немного. Документ загружается.


II.8 Photon-Electron Scattering and Crossing
Photon scattering on an electron
We now apply what we just learned to calculating the amplitude for the Compton scattering
of a photon on an electron, namely, the process γ(k)+ e(p) → γ(k
) + e(p
). First step:
draw the Feynman diagrams, and notice that there are two, as indicated in figure II.8.1.
The electron can either absorb the photon carrying momentum k first or emit the photon
carrying momentum k
first. Think back to the spacetime stories we talked about in chapter
I.7. The plot of our biopic here is boringly simple: the electron comes along, absorbs and
then emits a photon, or emits and absorbs a photon, and then continues on its merry way.
Because this is a quantum movie, the two alternate plots are shown superposed.
So, apply the Feynman rules (chapter II.5) to get (just to make the writing a bit easier,
we take the polarization vectors ε and ε
to be real)
M = A(ε
, k
; ε, k) + (ε
↔ ε, k
↔−k) (1)
where
A(ε
, k
; ε, k) = (−ie)
2
¯u(p
) ε
i
p +k − m
εu(p)
=
i(−ie)
2
2pk
¯u(p
) ε
(p +k + m) εu(p)
(2)
In either case, absorb first or emit first, the electron is penalized for not being real, by the
factor of 1/((p + k)
2
− m
2
) = 1/(2pk) in one case, and 1/((p − k
)
2
− m
2
) =−1/(2pk
) in
the other.
At this point, to obtain the differential cross section, you just have take a deep breath and
calculate away. I will show you, however, that we could simplify the calculation considerably
by a clever choice of polarization vectors and of the frame of reference. (The calculation is
still a big mess, though!) For a change, we will be macho guys and not average and sum
over the photon polarizations.
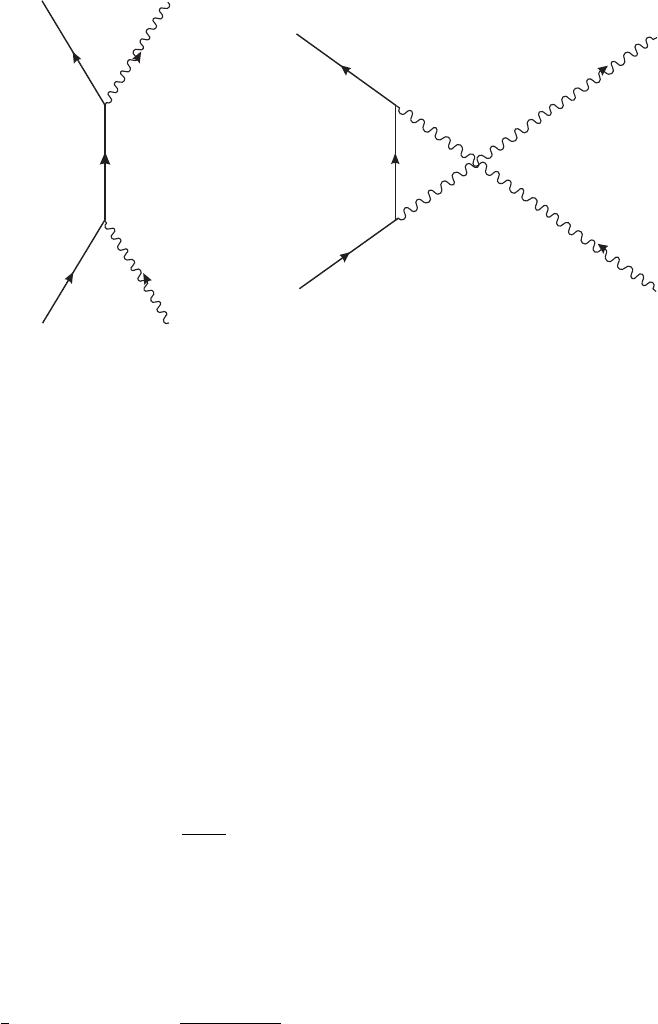
II.8. Photon-Electron Scattering | 153
p + k p − k’
(b)(a)
p’
, k
’, k ’
p
p’
, k
p
’, k ’
Figure II.8.1
In any case, we have εk = 0 and ε
k
= 0. Now choose the transverse gauge introduced
in the preceding chapter, so that ε and ε
have zero time components. Then calculate in
the lab frame. Since p = (m,0,0,0), we have the additional relations
εp = 0 (3)
and
ε
p = 0 (4)
Why is this a shrewd choice? Recall that a b = 2ab −b a. Thus, we could move p past
ε or ε
at the rather small cost of flipping a sign. Notice in (1) that (p +k + m) εu(p) =
ε(−p −k + m)u(p) =−ε ku(p) (where we have used εk = 0.) Thus
A(ε
, k
; ε, k) = ie
2
¯u(p
)
ε
ε k
2pk
u(p) (5)
To obtain the differential cross section, we need |M|
2
. We will wimp out a bit and
suppose, just as in chapter II.6, that the initial electron is unpolarized and the polarization
of the final electron is not measured. Then averaging over initial polarization and summing
over final polarization we have [applying II.2.8]
1
2
|A(ε
, k
; ε, k)|
2
=
e
4
2(2m)
2
(2pk)
2
tr(p
+ m) ε
ε k(p + m) k ε ε
(6)
In evaluating the trace, keep in mind that the trace of an odd number of gamma
matrices vanishes. The term proportional to m
2
contains k k = k
2
= 0 and hence van-
ishes. We are left with tr(p
ε
ε k p k ε ε
) = 2kp tr(p
ε
ε k ε ε
) =−2kp tr(p
ε
ε ε k ε
)
= 2kp tr(p
ε
k ε
) = 8kp[2(kε
)
2
+ k
p].

154 | II. Dirac and the Spinor
Work through the steps as indicated and the strategy should be clear. We anticommute
judiciously to exploit the “zero relations” εp = 0, ε
p = 0, εk = 0, and ε
k
= 0 and the
normalization conditions ε ε = ε
2
=−1 and ε
ε
= ε
2
=−1 as much as possible.
We obtain
1
2
|A(ε
, k
; ε, k)|
2
=
e
4
2(2m)
2
(2pk)
2
8kp[2(kε
)
2
+ k
p] (7)
The other term
1
2
|A(ε, −k; ε
, k
)|
2
=
e
4
2(2m)
2
(2pk)
2
8(−k
p)[2(k
ε)
2
− kp]
follows immediately by inspecting figure II.8.1 and interchanging (ε
↔ ε, k
↔−k).
Just as in chapter II.6, the interference term
1
2
A(ε, −k; ε
, k
)
∗
A(ε
, k
; ε, k) =
e
4
2(2m)
2
(2pk)(−2pk
)
tr(p
+ m) ε
ε k(p + m) k
ε
ε (8)
is the most tedious to evaluate. Call the trace T . Clearly, it would be best to eliminate
p
=p + k − k
, since we could “do more” with p than p
. Divide and conquer: write T =
P + Q
1
+ Q
2
. First, massage P ≡ tr(p + m) ε
ε k(p + m) k
ε
ε = m
2
tr ε
ε k k
ε
ε +
tr p ε
ε k p k
ε
ε. In the second term, we could sail the first p past the ε and ε
(ah, so
nice to work in the rest frame for this problem!) to find the combination p k p =2kp p −
m
2
k. The m
2
term gives a contribution that cancels the first term in P , leaving us with
P = 2kp tr ε
ε p k
ε
ε = 2kp tr p k
ε
(2ε
ε −ε
ε) ε = 8(kp)(k
p)[2(εε
)
2
− 1]. Similarly,
Q
1
=tr k ε
ε k p k
ε
ε =−2kε
tr k p k
ε
=−8(ε
k)
2
k
p and Q
2
=−tr k
ε
ε k p k
ε
ε
= 8(εk
)
2
kp
.
Putting it all together and writing kp
= k
p = mω
and k
p
= kp = mω,wefind
1
2
|M|
2
=
e
4
(2m)
2
ω
ω
+
ω
ω
+ 4(εε
)
2
− 2
(9)
We calculate the differential cross section as in chapter II.6 with some minor differences
since we are in the lab frame, obtaining
dσ =
m
(2π)
2
2ω
d
3
k
2ω
d
3
p
E
p
δ
(4)
(k
+ p
− k − p)
1
2
|M|
2
(10)
As described in the appendix to chapter II.6, we could use (I.8.14) and write
d
3
p
E
p
(
...
) =
d
4
p
θ(p
0
)δ(p
2
−m
2
)(
...
). Doing the integral over d
4
p
to knock out the 4-dimensional
delta function, we are left with a delta function enforcing the mass shell condition 0 =
p
2
−m
2
=(p + k − k
)
2
−m
2
=2p(k − k
) − 2kk
=2m(ω − ω
) − 2ωω
(1 −cos θ), with
θ the scattering angle of the photon. Thus, the frequency of the outgoing photon and of
the incoming photon are related by
ω
=
ω
1 +
2ω
m
sin
2
θ
2
(11)
giving the frequency shift that won Arthur Compton the Nobel Prize. You realize of course
that this formula, though profound at the time, is “merely” relativistic kinematics and has
nothing to do with quantum field theory per se.

II.8. Photon-Electron Scattering | 155
2
, k
2
1
, k
1
2
, k
2
1
, k
1
p
1
⫺ k
2
p
1
⫺ k
1
p
2
p
1
p
2
p
1
(b)(a)
Figure II.8.2
What quantum field theory gives us is the Klein-Nishina formula (1929)
dσ
d
=
1
(2m)
2
(
e
2
4π
)
2
(
ω
ω
)
2
ω
ω
+
ω
ω
+ 4(εε
)
2
− 2
. (12)
You ought to be impressed by the year.
Electron-positron annihilation
Here and in chapter II.6 we calculated the cross sections for some interesting scattering
processes. At the end of that chapter we marvelled at the magic of theoretical physics.
Even more magical is the annihilation of matter and antimatter, a process that occurs
only in relativistic quantum field theory. Specifically, an electron and a positron meet and
annihilate each other, giving rise to two photons: e
−
(p
1
) +e
+
(p
2
) →γ(ε
1
, k
1
) +γ(ε
2
, k
2
).
(Annihilating into one physical, that is, on-shell, photon is kinematically impossible.)
This process, often featured in science fiction, is unknown in nonrelativistic quantum
mechanics. Without quantum field theory, you would be clueless on how to calculate, say,
the angular distribution of the outgoing photons.
But having come this far, you simply apply the Feynman rules to the diagrams in fig-
ure II.8.2, which describe the process to order e
2
. We find the amplitude M =
A(k
1
, ε
1
; k
2
, ε
2
) + A(k
2
, ε
2
; k
1
, ε
1
) (Bose statistics for the two photons!), where
A(k
1
, ε
1
; k
2
, ε
2
) = (ie)(−ie)¯v(p
2
) ε
2
i
p
1
−k
1
− m
ε
1
u(p
1
) (13)
Students of quantum field theory are sometimes confused that while the incoming electron
goes with the spinor u, the incoming positron goes with ¯v, and not with v. You could check
this by inspecting the hermitean conjugate of (II.2.10). Even simpler, note that ¯v(
...
)u
[with (
...
) a bunch of gamma matrices contracted with various momenta] transforms
correctly under the Lorentz group, while v(
...
)u does not (and does not even make sense,
since they are both column spinors.) Or note that the annihilation operator d for the
positron is associated with ¯v, not v.

156 | II. Dirac and the Spinor
I want to emphasize that the positron carries momentum p
2
=(+
p
2
2
+ m
2
, p
2
) on its
way to that fatal rendezvous with the electron. Its energy p
0
2
=+
p
2
2
+ m
2
is manifestly
positive. Nor is any physical particle traveling backward in time. The honest experimental-
ist who arranged for the positron to be produced wouldn’t have it otherwise. Remember
my rant at the end of chapter II.2?
In figure II.8.2a I have labeled the various lines with arrows indicating momentum
flow. The external particles are physical and there would have been serious legal issues
if their energies were not positive. There is no such restriction on the virtual particle
being exchanged, though. Which way we draw the arrow on the virtual particle is purely
up to us. We could reverse the arrow, and then the momentum label would become
p
2
− k
2
= k
1
− p
1
: the time component of this “composite” 4-vector can be either positive
or negative.
To make the point totally clear, we could also label the lines by dotted arrows showing the
flow of (electron) charge. Indeed, on the positron line, momentum and (electron) charge
flow in opposite directions.
Crossing
I now invite you to discover something interesting by staring at the expression in (13) for
a while.
Got it? Does it remind you of some other amplitude?
No? How about looking at the amplitude for Compton scattering in (2)?
Notice that the two amplitudes could be turned into each other (up to an irrelevant sign)
by the exchange
p ↔ p
1
, k ↔−k
1
, p
↔−p
2
, k
↔ k
2
, ε ↔ ε
1
, ε
↔ ε
2
, u(p) ↔ u(p
1
), u(p
) ↔ v(p
2
) (14)
This is known as crossing. Diagrammatically, we are effectively turning the diagrams in
figures II.8.1 and II.8.2 into each other by 90
◦
rotations. Crossing expresses in precise terms
what people who like to mumble something about negative energy traveling backward in
time have in mind.
Once again, it is advantageous to work in the electron rest frame and in the transverse
gauge, so that we have ε
1
p
1
= 0 and ε
2
p
1
= 0 as well as ε
1
k
1
= 0 and ε
2
k
1
= 0. Averaging
over the electron and positron polarizations we obtain
dσ
d
=
α
2
8m
ω
1
|p|
ω
ω
+
ω
ω
− 4(εε
)
2
+ 2
(15)
with ω
1
= m(m + E)/(m + E − p cos θ), ω
2
= (E − m − p cos θ)ω
1
/m, and p =|p| and
E the positron momentum and energy, respectively.

II.8. Photon-Electron Scattering | 157
x
time
y
Figure II.8.3
Special relativity and quantum mechanics require antimatter
The formalism in chapter II.2 makes it totally clear that antimatter is obligatory. For us to
be able to add the operators b and d
†
in (II.2.10) they must carry the same electric charge,
and thus b and d carry opposite charge. No room for argument there. Still, it would be
comforting to have a physical argument that special relativity and quantum mechanics
mandate antimatter.
Compton scattering offers a context for constructing a nice heuristic argument. Think
of the process in spacetime. We have redrawn figure II.8.1a in figure II.8.3: the electron is
hit by the photon at the point x, propagates to the point y, and emits a photon. We have
assumed implicitly that (y
0
− x
0
)>0, since we don’t know what propagating backward
in time means. (If the reader knows how to build a time machine, let me know.) But
special relativity tells us that another observer moving by (along the 1-direction say) would
see the time difference (y
0
− x
0
) = coshϕ(y
0
− x
0
) − sinhϕ(y
1
− x
1
), which could be
negative for large enough boost parameter ϕ, provided that (y
1
− x
1
)>(y
0
− x
0
), that
is, if the separation between the two spacetime points x and y were spacelike. Then this
observer would see the field disturbance propagating from y to x. Since we see negative
electric charge propagating from x to y, the other observer must see positive electric
charge propagating from y to x. Without special relativity, as in nonrelativistic quantum
mechanics, we simply write down the Sch
¨
odinger equation for the electron and that is
that. Special relativity allows different observers to see different time ordering and hence
opposite charges flowing toward the future.
Exercises
II.8.1 Show that averaging and summing over photon polarizations amounts to replacing the square bracket
in (9) by 2[
ω
ω
+
ω
ω
− sin
2
θ]. [Hint: We are working in the transverse gauge.]
II.8.2 Repeat the calculation of Compton scattering for circularly polarized photons.
This page intentionally left blank

Part III Renormalization and Gauge Invariance
This page intentionally left blank

III.1 Cutting Off Our Ignorance
Who is afraid of infinities? Not I, I just cut them off.
—Anonymous
An apparent sleight of hand
The pioneers of quantum field theory were enormously puzzled by the divergent integrals
that they often encountered in their calculations, and they spent much of the 1930s
and 1940s struggling with these infinities. Many leading lights of the day, driven to
desperation, advocated abandoning quantum field theory altogether. Eventually, a so-called
renormalization procedure was developed whereby the infinities were argued away and
finite physical results were obtained. But for many years, well into the late 1960s and even
the 1970s many physicists looked upon renormalization theory suspiciously as a sleight of
hand. Jokes circulated that in quantum field theory infinity is equal to zero and that under
the rug in a field theorist’s office had been swept many infinities.
Eventually, starting in the 1970s a better understanding of quantum field theory was
developed through the efforts of Ken Wilson and many others. Field theorists gradually
came to realize that there is no problem of divergences in quantum field theory at all. We
now understand quantum field theory as an effective low energy theory in a sense I will
explain briefly here and in more detail in chapter VIII.3.
Field theory blowing up
We have to see an infinity before we can talk about how to deal with infinities. Well, we
saw one in chapter I.7. Recall that the order λ
2
correction (I.7.23) to the meson-meson
scattering amplitude diverges. With K ≡k
1
+ k
2
, we have
M =
1
2
(−iλ)
2
i
2
d
4
k
(2π)
4
1
k
2
− m
2
+ iε
1
(K − k)
2
− m
2
+ iε
(1)
As I remarked back in chapter I.7, even without doing any calculations we can see the
problem that confounded the pioneers of quantum field theory. The integrand goes as 1/k
4
