Yi Lin. General Systems Theory: A Mathematical Approach

Подождите немного. Документ загружается.

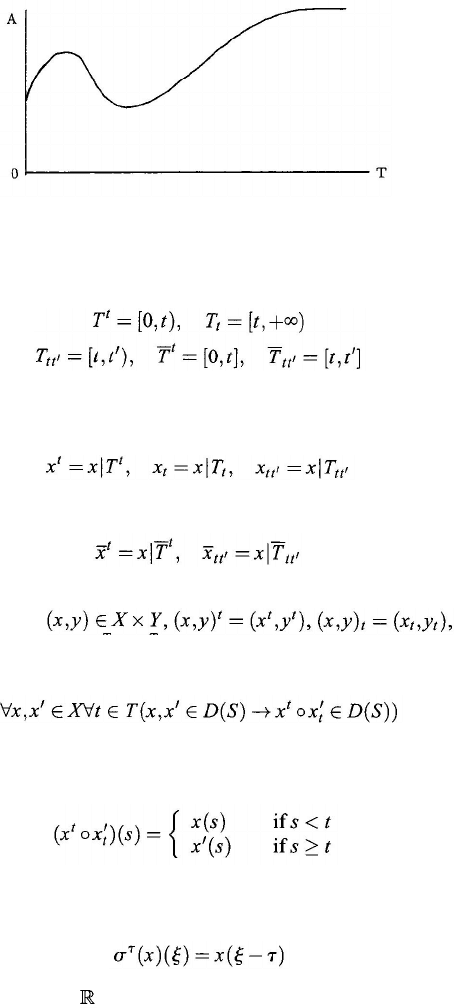
274

Chapter 10

Figure 10.7. The graph of x in the plane T

For any t, t' ∈ T with t < t', let

(10.60)

(10.61)

Then the restrictions of a time function x

∈ X with respect to these time intervals

are denoted by

(10.62)

and

(10.63)

For each y ∈ Y, or in general, for sets and vectors similar notation will be used;

for example, for each etc.

A subset S

⊂ X × Y ⊂ A

× B

is called a linear time system if S is a linear

subspace of X

× Y and the input space D

(

S) satisfies the condition:

(10.64)

where x

is a time function ∈ A

, called the concatenation of x

and

, and is

defined by the following: For any s

∈ T,

(10.65)

Without loss of generality, we will always assume that D

(

S) = X and R

(

S) = Y.

Let

be the shift operator defined as follows: For each x ∈ X,

(10.66)

for each

ξ ∈

, where

τ ∈

can be any value if

(

x) is meaningful. Figures 10.7–

10.9 show the geometric meaning of the concept of shift operators. In Figs. 10.8

Systems of Single Relations

275

Figure 10.8. The graph of

(

) with

> 0.

and 10.9 the dotted A × T planes indicate the locations of the A × T plane before

the shift operation

functions. Therefore, when τ > 0, the application of σ

implies that we move the graph of

units to the right; when

< 0, the application

of σ

tells us to consider the portion of the graph of x on the right of the vertical

line t =

τ.

A linear time system S

⊂ X × Y is called strongly stationary if S satisfies the

condition

(10.67)

Theorem 10.3.5. Let S

⊂ X × Y and S

: Y → X be a linear time system and a

strongly stationary linear functional time system, respectively. Then the feedback

system F

(

S, S

)

is strongly stationary iff the original system S is strongly stationary.

Proof: Necessity. Suppose that the feedback system F

(

S, S

) is strongly

stationary. Then

S = {(x + S

(

y), y) : (x, y) ∈ F

(

S, S

)}

(10.68)

Figure 10.9. The graph of σ

(

) with τ < 0.

276

Chapter 10

It suffices to show that for any for every t ∈ T. we

now pick an arbitrary (

x,y) ∈ S and t ∈ T. Then

(10.69)

From the hypothesis that the systems F(S,S

) and S

are strongly stationary, it

follows that

(10.70)

and

(10.71)

Let

and Then, Eq. (10.71) shows that

Therefore,

(10.72)



where so Eq. (10.72) implies that

Sufficiency. This follows from Theorem 10.2.3 and the necessity part of this

proof.

A linear time system S ⊂ X × Y is called precausal if it satisfies the condition:

(10.73)

where

A linear functional time system S : X → Y is

called causal if it is precausal.

Theorem 10.3.6. Let S

⊂ X × Y be a linear time system. Then S is precausal iff

Proof:

Necessity. Suppose

is precausal. Then for any

implies that

This shows that when

because

is the same as Hence,

and

implies that

Sufficiency. Suppose

This

implies that



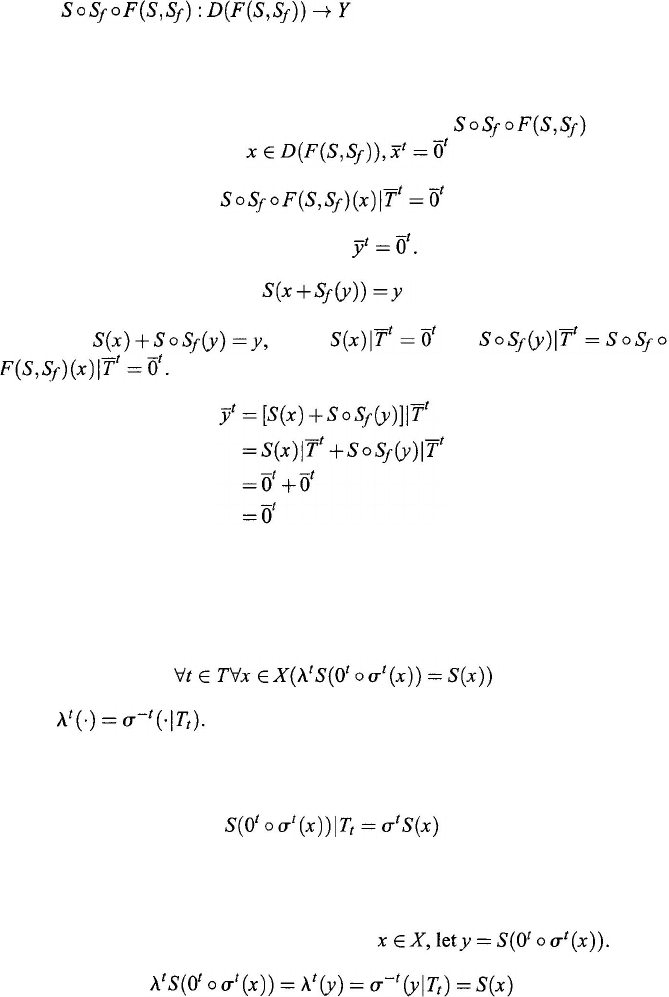
Systems of Single Relations

277

Theorem 10.3.7. Let S : X → Y and S

: Y → X be linear functional causal time

systems.

Then the feedback system F (S,S

) is causal iff the composition time

system is causal.

Proof: The argument of the necessity part follows from the fact that each

composition of causal functional time systems is causal.

Sufficiency.

Suppose that the composition system is causal.

That is, for any t

∈ T and any

implies that

(10.74)

Let y = F (S,S

) (

). We need to show that By Eq. (10.24),

(10.75)

Therefore, where

and

Thus,

This implies that the feedback system F(S,S

) is causal.



A linear functional time system S : X → Y is called time invariably realizable

if it satisfies the condition

(10.76)

where

Theorem 10.3.8.

A linear functional time system S : X → Y is time invariably

realizable iff for any t

∈ T and any x ∈ X

(10.77)

Proof: Necessity. Suppose S is time invariably realizable. Then S satisfies the

relation in Eq. (10.76). It suffices to show that Eq. (10.77) is derivable from the

relation in Eq. (10.76). For any t

∈ T and any

Then

(10.78)

278

Chapter 10

The last equality of Eq. (10.78) shows that

(10.79)

Sufficiency. Suppose

satisfies Eq. (10.77) for any

∈

and any

∈

Then

(10.80)

Comparing Eq. (10.80) and the relation in Eq. (10.76), we see that S is time

invariably realizable.



Theorem 10.3.9. Suppose that S : X → Y and S

: Y → X are two linear func-

tional time systems such that S and S

are time invariably realizable. Then the

feedback system F

(

S,S

) is time invariably realizable iff for any t ∈ T and any

i.e., the system

is time invariably realizable.

(10.81)

Proof: Sufficiency. Suppose the system is time invariably

realizable. Then for any t

∈ T and any Eq. (10.81) holds. From

the hypothesis that S is time invariably realizable, it follows from Theorem 10.3.8

that Eq. (10.77) holds. Therefore,

This implies that

This equation is the same as

(10.82)

(10.83)

(10.84)

Therefore, applying Theorem 10.3.8, we have showed that the feedback system

(

S,S

) is time invariably realizable.

Necessity. Suppose F (S,S

) is time invariably realizable. Then Theorem

10.3.8 says that

(10.85)

Systems of Single Relations

279

Let

Then from Eq. (10.85) we have

(10.86)

(10.87)

From the hypothesis that S is time invariably realizable and Theorem 10.3.8, it

follows that Eq. (10.87) can be written as

(10.88)

Equation (10.88) is the same as Eq. (10.81). This ends the proof.



We conclude this section with some discussion of chaos, attractor, and feedback

transformation.

Theorem 10.3.10.

For each linear system S ⊂ X × X there exists a feedback

component system S

: X → X, such that D ⊂ X is a chaos, attractor, or strange

attractor, respectively, of S, iff D is a chaos, attractor, or strange attractor of

(S,S

)

, respectively.

The proof follows from Theorem 10.2.4.

Theorem 10.3.11. Suppose that S ⊂ X × X and S

: X → X are a linear system

and a functional linear system, respectively. Then a subset D

⊂ X is a chaos of S

[iff D is a chaos of the feedback system F

(

S,S

)

], if

(10.89)

Proof: Necessity. Suppose D ⊂ X is a chaos of S but not a chaos of F(

S,S

Pick an element

Then

This contradicts relation (10.89). Therefore, D must be a

chaos of F

(

S,S

Sufficiency. Suppose D ⊂ X is a chaos of F

(

S,S

) but not a chaos of S. Pick

an element Then Therefore, it must be

that

Now

which contradicts relation (10.89).

So D must be a chaos of S.



Theorem 10.3.12. Suppose that S ⊂ X × X and S

: X → X are a linear system

and a functional linear system, respectively. Then D

⊂ X is an attractor of S (iff

D is an attractor of the feedback system F(S,S

)

), if

(10.90)

and

(10.91)

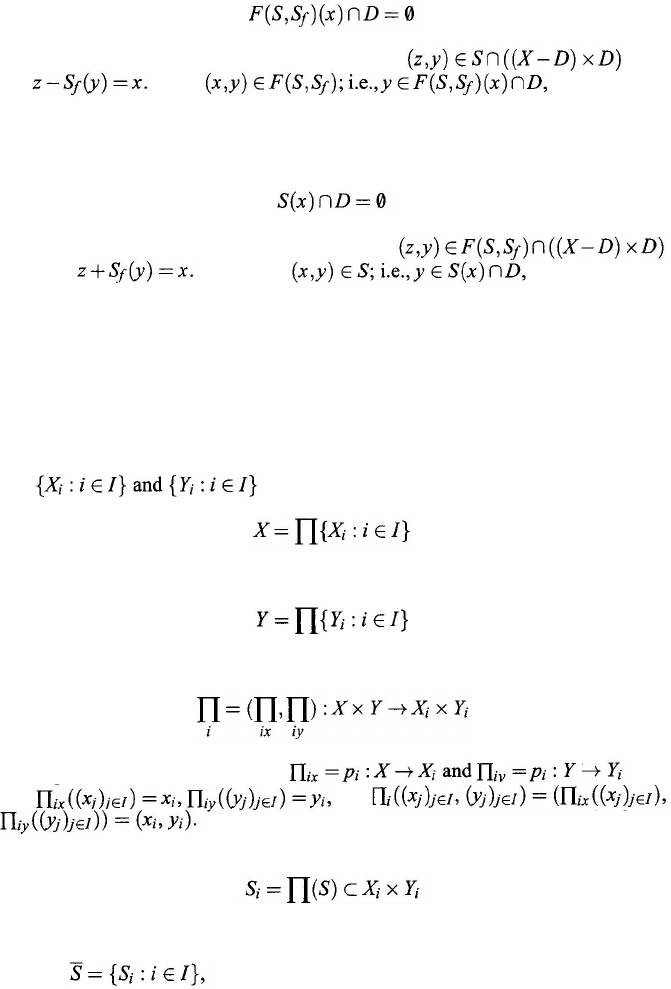
280

Chapter 10

Proof:

Necessity. Suppose

⊂

, satisfying condition (10.90), is an attractor

of S but not of F

(

S,S

). Then there exists an element x ∈ X – D such that

(10.92)

From condition (10.90) it follows that there exists

such

that

Thus,

contradiction.

Sufficiency. Suppose D ⊂ X, satisfying condition (10.91), is an attractor of

(

S,S

), but not of the original system

. There then exists an element x ∈ X – D

such that

(10.93)

From condition (10.91) it follows that there exists

such that

Therefore,

contradiction.



10.4. Decoupling of Single-Relation Systems

Let S ⊂ X × Y be an input–output system. We assume that the input space X

and the output space Y can be represented in terms of the factor sets; i.e., there are

sets

, where I is a fixed index set, such that

(10.94)

and

(10.95)

For each index i ∈ I, let

(10.96)

be the projection defined by letting such

that

and

We now can represent factor systems of S on the factor

sets X

and Y

; i.e., for each i ∈ I

, the factor system

of S can be defined by

(10.97)

The system S can now be viewed as decomposed into a family of factor

systems

and S can be represented as a relation among its factor

Systems of Single Relations 281

systems (i.e.,

by identifying

with

The systems S and S

are called the overall system

and the factor (or component) system, respectively, and

is called

a complex system representation over {

: i ∈ I

Generally, there are many interactions among subsystems, so S is a proper

subset of the product of its factor systems

∏

{

: i ∈ I}. If S = ∏

{

: i ∈ I }, S is

called noninteracted

We now consider what properties are transferable from the overall system S to

its component systems

or from the component systems S

to an overall system

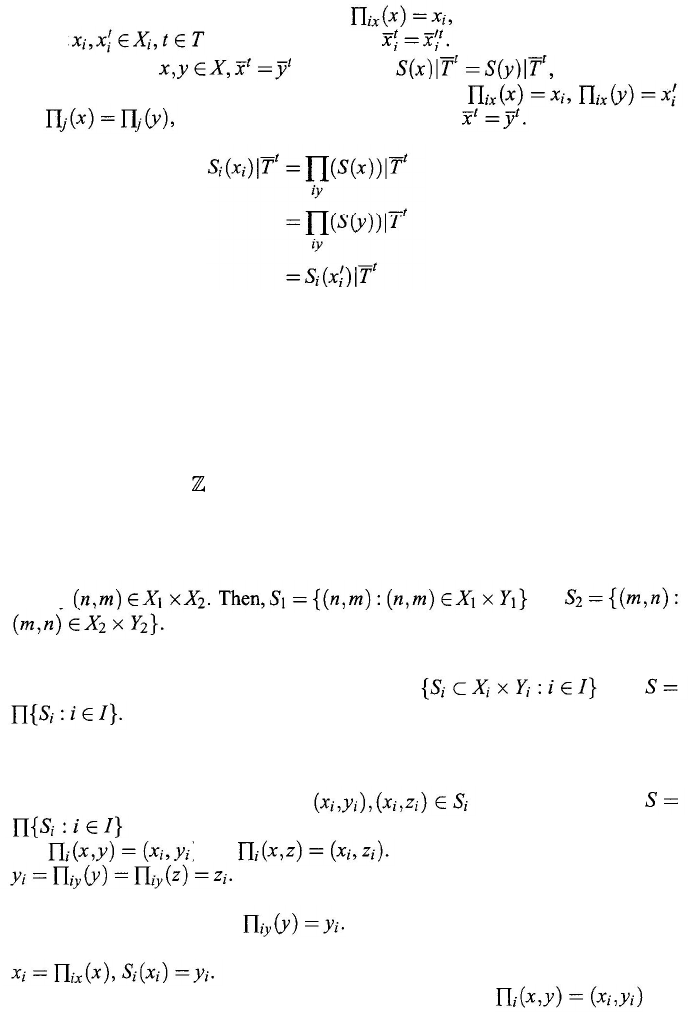
Proposition 10.4.1. Let S

⊂ ∏

{

: i ∈ I} be an input–output system which has

been decomposed into a family of factor systems

{

: i ∈ I}. If S is a linear

system over a field A, each component system S

is then also a linear system over

the field A.

Proof: From the hypothesis that S is a linear system over the field A, it

follows that, for each i

∈ I, S

is a nonempty system on X

. We need to show

two things: (1) For any for any

(

x,y

)

∈

and

α ∈

(

x,y) ∈ S

The argument of (1). For any (

) and (

) ∈ S

, there are (x

) and

) ∈ S such that

and

Therefore,

because

The argument of (2). For any (

)

∈

and any α ∈ A, there exists a pair

(

x,y) ∈ S such that

Therefore,

(10.98)

(10.99)

because

(

x,y) ∈ S.

Proposition 10.4.2. Suppose that S

⊂ X × Y is a linear time system that has

been decomposed into a family of component systems

{

⊂ X

× Y

: i ∈ I

}

; i.e.,

⊂ ∏

{

: i ∈ I

Then each component system S

is also a linear time system.



282

Chapter 10

Proof: It suffices from Proposition 10.4.1 to show that for any x,y ∈ D(S

)

and any t

∈ T, the concatenation of for each i ∈ I. In fact, for

any x,y

∈ D

(

) and any t ∈ T, there exist u, v ∈ D(

) such that

(10.100)

Therefore,

because



Proposition 10.4.3. Under the same assumption as Proposition 10.4.2 and if the

system S is strongly stationary, then each component system S

is also strongly

stationary.

Proof: Let

be arbitrary.

There then exists some element

such that

Furthermore, there exists some (

x,y) ∈ S

such that

Since

where

which implies that Hence,

Consequently,

Conversely, let

be arbitrary. There then exists some (

)

∈

such that

Furthermore, there exists some (

x,y) ∈ S such that

we have

That is, there exists some (x',y') ∈ S such that

Hence,

That is,

Combining these arguments, we obtain the following: For each index

∈

and

any

From Eq. (10.66), we can show

that the component system S

is strongly stationary.



Proposition 10.4.4. Under the same assumption as in Proposition 10.4.2 and

if the system S is precausal with D

(

) = X,

each component system S

is also

precausal.

Systems of Single Relations

283

Proof: Let i ∈ I be arbitrarily fixed. It can then be seen that D(S

) = X

. In

fact, if D

(

) ≠ X

, we can pick an element x

∈ X

– D(

). This implies that

(

S) ≠ X, because no x ∈ D

(

S) satisfies

contradiction.

Let arbitrary, and assume

Since it is assumed that

precausal, for any implies that from Theorem

10.3.6. Therefore, when the elements x,y ∈ X satisfy

and

for every j ∈ I with j ≠ i, we have

Thus

(10.101)

Applying Theorem 10.3.6 we have shown that each component system S

precausal.



Example 10.4.1.

We will construct an example to show that even though an overall

system S is functional, none of the component systems S

is functional.

Suppose the index set I = {1,2} and the sets X

, X

, Y

, and Y

are all the

same, namely the set of all integers. Let S be a functional linear system from

into

defined by

(

n,m

) = (

m,n

)

(10.102)

for any and

Then it can be easily seen that neither

nor S

is functional.

Proposition 10.4.5. Suppose that S ⊂ X × Y is a functional system that has been

decomposed into a family of component systems with

Then each component system S

is also functional. If, moreover, the

overall system S is bijective, each component system S

is also bijective.

Proof: For any fixed i

∈ I, let be arbitrary. Since

due to the assumption, there exist elements (x,y), (x,z)

∈ S such

that

and

Because S is functional, y = z, so,

That is, the component system

is functional.

Suppose S is a bijective function from X onto Y. For any y

∈ Y

there exists

an element y ∈ Y such that From the hypothesis that S is bijective,

it follows that there exists an element x

∈ X such that S(x) = y. Therefore, for

That is, S

is surjective. Let (

) and (

) ∈ S

arbitrary. Then there exist (x,y) and (w,y)

∈ S such that and