Yamaguchi H. Engineering Fluid Mechanics (Fluid Mechanics and Its Applications)
Подождите немного. Документ загружается.

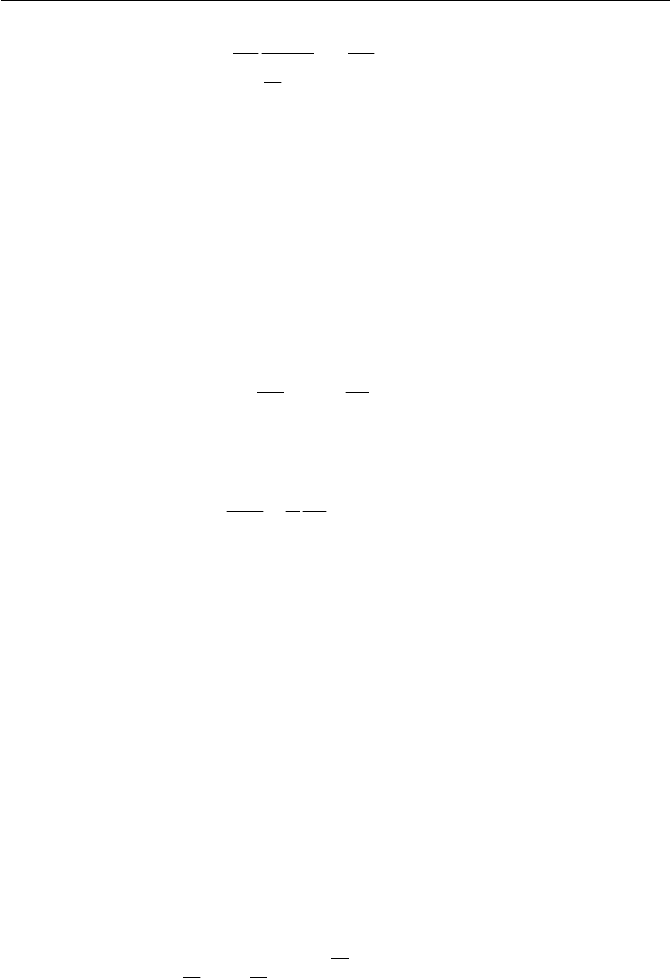
7.2 Standard Flow and Material Functions
2
0
0
4
D
F
u
u
L
E
S
W
(or
F
Q
u
L
)
(7.2.62)
If the cross section area of the filament varies with the axial distance
x
,
for example, according to
x
eAxA
0
(7.2.63)
0
A and
xA are the cross section area at 0 x and
x
respectively. It
proves useful to justify the derivation of Eq. (7.2.63). That is originated
from the thought that a measure for the amount of elongation
E
H
is often
described by the so-called Hencky strain, defined as
¸
¸
¹
·
¨
¨
©
§
¸
¸
¹
·
¨
¨
©
§
0
0
lnln
x
x
A
A
x
E
H
(7.2.64)
The strain rate
E
H
is thus expressed as
dt
dx
xdt
d
E
E
1
H
H
(7.2.65)
So that, for a constant
E
H
, the length
x
increases with time t exponen-
tially with the expression
t
E
exx
H
0
(7.2.66)
and for the cross section area where
t
E
eAA
H
0
(7.2.67)
Equation (7.2.63) is thus recovered by assuming that
E
H
is kept constant
along its length to give
x
with, likewise, only a function of time where
tx
E
H
(7.2.68)
For
Lx
, knowing
L
u , Eq. (7.2.63) may be approximated through the
following form
L
E
u
L
L
eDD
H
SS
|
2
0
2
44
(7.2.69)
Equation (7.2.66) can then be written for
E
H
as
435
7 Non-Newtonian Fluid and Flow
¸
¸
¹
·
¨
¨
©
§
»
»
¼
º
«
«
¬
ª
¸
¸
¹
·
¨
¨
©
§
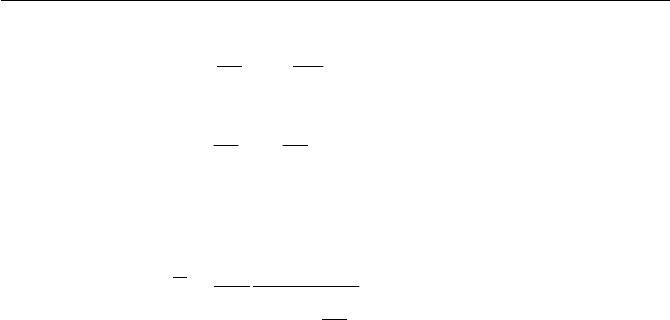
0
2
0
ln
ln
u
u
c
L
u
D
D
c
L
u
LL
L
L
E
H
(7.2.70)
c
is an experimental constant. Thus, from Eq. (7.2.62) and Eq. (7.2.70),
the apparent viscosity defined in Eq. (7.2.60) is readily calculated where
¸
¸
¹
·
¨
¨
©
§
0
2
0
0
ln
4
u
u
cD
F
u
L
L
e
S
K
(7.2.71)
In order to minimize the error and to achieve a more idealistic state to
validate Eq. (7.2.71), the filament can be extruded in isothermal cambers,
for example, Sampers and Leblans (1988).
Test fluids with higher fluidity and at higher elongational rate may be
tested for measuring the apparent elongational viscosity by using a pres-
sure-driven converging channel as illustrated in Fig. 7.13(b). The average
velocity increases monotonically as fluid particles move toward the apex.
There appears an elongational flow field, though we must aware of the fact
that there exists a boundary (shear) layer along the channel wall. To have a
well-defined elongational flow field in the channel, the upstream section of
the channel needs to be a tube with a large diameter
0
D relative to the
throat diameter
L
D . The entering flow also has to be moderate. The rela-
tive amount of a shear layer and an elongational core will depend on the
geometry of the channel, the volumetric flow rate and the fluid’s properties.
To minimize the presence of the shear layer, it has been proposed that the
channel walls may have to be lubricated with a relatively low viscosity
fluid (Hsu et al. 1980), although it is difficult to form a uniform layer of
lubricant along the channel wall.
Considering the elongational rheometer, an injection molding machine
is often used as a rheometer head. Cogswell (1978) derived an expression
for calculating an apparent elongational viscosity by using a die entry flow
field. See reference to Fig. 7.13(b) for a nomenclature in cylindrical coor-
dinates. The entry pressure drop
ent
p' is assumed to have two contributing
parts where
esent
ppp '' '
(7.2.72)
436
Exercise
s
p is the shear flow contribution and
e
p is the elongational flow contri-
bution. The velocity through the channel is calculated by the power law
model with a power law index
n , and for the power law fluid, the average
shear viscosity (shear thinning viscosity
1n )
a
K
is obtained via the ap-
parent shear rate
a
J
in the capillary viscometer whose radius is
L
R , such
that
3
4
L
a
R
Q
S
J
(7.2.73)
This is also the so-called Newtonian wall shear rate (see Exercise 7.2.4).
The derived expression for the apparent elongational viscosity
e
K
is given
in Gogswell (1978) as
entE
pn ' 1
8
3
W
(7.2.74)
ent
aa
E
pn '
13
4
K
J
H
(7.2.75)
so that
2
2
2
32
19
aa
ent
e
pn
JK
K
'
(7.2.76)
The measuring technique with a pressure-driven converging channel
has an advantage in the case of a rheometric measurement. However, an
unknown flow field in the channel would lead to future questions of
equivalency to simple uniaxial stretching that is based on a shearfree flow,
no matter how drastic assumptions have to be made. This is, in fact, the
difficulty of measuring the elongational viscosity in general.
Exercise
Exercise 7.2.1
1
N Measurement by a Cylindrical Couette Flow
By utilizing a simple shear flow in concentric rotating cylinders (refer-
ring to in Fig. 6.3(b)) the normal stress difference, especially the 1st
normal stress difference
TT
WW
rr
N
1
in cylindrical coordinates system, can
be measured. Discuss the principle of the measurement, assuming that in
the gap there are non-zero stress components of
T
W
r
,
rr
W
,
TT
W
and
zz
W
.
''
437

7 Non-Newtonian Fluid and Flow
Consider a case when the inner cylinder is rotated with an angular velocity
Z
for a small gap ratio
1
21
| rrk
r
.
Ans.
With a simple shear flow,
0,,0
T
u u
, and Cauchy’s equation of mo-
tion in
r
direction, ignoring the body force term, we have
r
r
rrr
p
r
u
rr
TTT
W
WU
w
w
w
w
1
2
(1)
Equation (1) can be rearranged, using
r
r
-component of the total stress ten-
sor
rr
T
as follows
rr
u
r
p
r
T
rr
rr
rr
TT
T
WW
U
W
w
w
w
w
2
(2)
Since
rr
T is an only function of
r
, i.e. infinite length cylinder approxima-
tion, Eq. (2) can be integrated with respect to
r
to obtain
rr
T
dr
rr
u
rTrT
r
r
rr
rrrr
³
¸
¹
·
¨
©
§
2
1
2
21
TT
T
WW
U
(3)
resulting in
³
¸
¹
·
¨
©
§
2
1
1
2
22
r
r
rrrrr
dr
r
N
r
u
rTrkT
T
U
(4)
In the case of a small gap, the cylindrical Couette flow, the shear rate
T
JJ
r
can be reduced to (Eq. 6.3.37)
r
r
k
|
1
Z
JJ
T
(5)
which is assumed to be kept constant in the gap, so that
T
u becomes the
linear velocity profile as follows
¸
¸
¹
·
¨
¨
©
§
|
2
2
1
1
r
r
rk
k
u
r
r
Z
T
(6)
Substituting Eq. (6) for Eq. (4), and noting
rNN
11
, Eq. (4) can now be
integrated to obtain
438
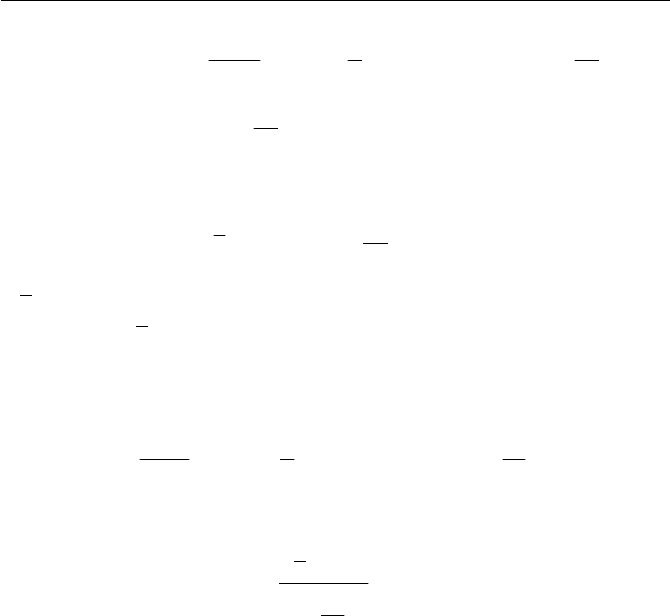
Exercise
¸
¹
·
¨
©
§
¿
¾
½
¯
®
¸
¹
·
¨
©
§
¸
¹
·
¨
©
§
r
r
rrr
r
rr
r
rr
k
N
k
kkrk
k
rTrkT
1
ln
1
ln121
2
1
1
1
2
2
2
2
2
2
Z
U
(7)
and to set
¸
¹
·
¨
©
§
r
k
Np
1
ln
1
ZI
(8)
p is the measured pressure difference between the outer and inner cylin-
drical walls.
p is the actual value of the pressure difference, which in-
cludes the normal stress components and is measurable by such flash
mount pressure transducers.
ZI
is a known function, which is written as
°
¿
°
¾
½
°
¯
°
®
¸
¸
¹
·
¨
¨
©
§
¸
¸
¹
·
¨
¨
©
§
r
rrr
r
k
kkrk
k
1
ln121
2
1
1
22
2
2
Z
UZI
(9)
Therefore,
1
N can be obtained in principle from Eqs. (7) and (9) where
¸
¹
·
¨
©
§
r
k
p
N
1
ln
1
ZI
(10)
Exercise 7.2.2 Energy Dissipation
Give an expression for the energy dissipation
c
W per cycle per unit of
volume in an oscillatory shear.
Ans.
The energy dissipation
c
W
is equal to the work done per unit volume
of a fluid undergoing one cycle in an oscillatory shear
yx
W
, so that
c
W can
be written as
³
c
yx
c
dttW
JW
(1)
The share rate
t
J
and
yx
W
in Eq. (1) are given in Eqs. (7.2.24) and in
'
'
'
'
(7.2.25) as follows
439
7 Non-Newtonian Fluid and Flow
tt
Z
J
J
cos
0
(2)
G
Z
WW
t
yxyx
sin
0
(3)
0
J
is the share rate amplitude,
0yx
W
is the stress amplitude and
G
is the
mechanical loss angle for the angular velocity
I
ZZ
. It is noted that
0yx
W
given in Eq. (7.2.25) is as follows
00
JW
*
G
yx
(4)
where
*
G is the magnitude of the complex modulus and
0
J
is the shear
strain amplitude.
Substituting Eqs. (2) and (3) for (1) and integrating gives
00
0
0
2
0
00
sin
sin
sin
sincos
JJGS
GSJW
GSJW
GZZJW
S
*
G
dtttW
yxo
yxo
yxc
³
(5)
From the relationship in Eq. (7.2.31), we find that
00
J
J
S
GW
c
cc
(6)
Therefore, the energy dissipation is directly proportional to the loss mod-
ulus.
Exercise 7.2.3 Dynamic Properties of G
c
and G
cc
Examine the storage of G
c
and loss of G
cc
moduli for a Newtonian fluid
and a Hookean solid where subjected to an oscillatory shear.
Ans.
For a Newtonian fluid, we have a linear pure viscous constitutive rela-
tionship where
JKW
yx
(1)
For the oscillatory shear, we have this from Eq. (7.2.24):
440

Exercise
t
yx
Z
J
K
W
cos
0
(2)
In comparison with Eq. (7.2.28), we can derive the relationship
Z
ZKK
G
cc
c
and 0
c
G due to
0
cc
ZK
(3)
This shows that the mechanical loss angle (phase-shift) is
2
S
since
)()( tt
Z
S
Z
cos2sin .
For a Hookean solid, we have a linear pure elastic constitutive relation
where
JW
G
yx
(4)
G is a shear modulus while the oscillatory shear from Eq. (7.2.23) leads to
tG
yx
Z
J
W
sin
0
(5)
Similarly, in comparison with Eq. (7.2.27), we can obtain results that give
us
GG
c
and 0
cc
G
These show that the mechanical loss angle (phase-shift) is zero.
Exercise 7.2.4 Rabinowitsch Procedure
Knowing a constitutive relationship for a non-Newtonian fluid (typically
for power law fluids) or a Newtonian fluid, an apparent viscosity given in
Eq. (7.1.2) is measured by a capillary rheometer. Show the principle of the
measurement and apply it to a power law fluid, for example.
Ans.
Consider a flow in a capillary tube with reference to Exercise 7.1.2. The
shear stress
rz
W
is given in Eq. (5) in Exercise 7.1.2 as follows:
¸
¹
·
¨
©
§
w
w
z
p
r
rz
2
W
(1)
At the tube wall, i.e.
R
r
, the wall shear stress
w
W
is written as
441
7 Non-Newtonian Fluid and Flow
¸
¹
·
¨
©
§
w
w
z
pR
w
2
W
(2)
From Eqs. (1) and (2), we can write
rz
W
as
r
R
w
rz
W
W
(3)
Note that Eq. (3) is valid for any fluid in a fully developed pipe flow.
For a steady state of fully developed unidirectional flow, the velocity
profile
z
u at a given cross section is only a function of
r
, so that the shear
rate 0'' zu
zrz
J
may be written by a function
g
as follows
rz
z
g
r
u
W
w
w
(4)
Here,
g
is a positive function of
rz
W
and
rz
W
is a function of
r
. Integrating
Eq. (4) for given boundary conditions, i.e. 0
z
u for R
r
, we have
³
R
r
rzz
drgu
W
(5)
Equation (5) can be transformed into an integration with respect to
W
as
³
w
dg
R
u
w
z
W
W
WW
W
(6)
where we can write
rz
WW
for brevity’s sake.
It is now desired to derive an expression for the flow rate
Q , which is
calculated by
³
³
w
w
R
R
z
dr
r
u
r
drruQ
0
2
0
2
)(
S
S
(7)
³
R
drgr
0
2
WS
(8)
The integration parameter
r
in Eq. (8) can be transformed into
W
with the
aid of Eq. (3) to give
442
Exercise
³
w
dg
R
Q
w
W
WWW
W
S
0
2
3
3
(9)
Setting the shear rate
rz
J
J
in Eqs. (4), (5) is now rewritten as
³
w
d
R
Q
w
W
WWJ
S
W
0
2
3
3
(10)
Equation (10) is also written as a differential equation of
Q by differentiat-
ing
w
W
to give
22
3
3
3
1
www
w
w
Q
d
dQ
R
WJW
W
W
S
¸
¹
·
¨
©
§
(11)
and
w
w
w
w
d
Qd
R
W
W
WS
J
3
23
11
(12)
where
w
J
is the wall shear rate at R
r
. Equation (11) is often called the
Rabinowitsch equation, noting that Eq. (11) can be written in term of the
pressures difference
L
ppp
0
for a given section L , as exemplified in
Fig. 7.3, with the following formula
¸
¸
¹
·
¨
¨
©
§
'
'
pd
dQ
pQ
R
w
3
1
3
S
J
(13)
where a relationship
LpR
w
2
W
is used for Eq. (12).
Equation (12) can be further reduced to a preferable expression to fit a
monotonous, simple polynomial form as follows:
w
w
w
w
w
d
R
Q
d
R
Q
R
Q
R
Q
d
d
W
S
SS
S
W
W
W
J
ln
4
ln
4
4
14
4
3
4
4
1
3
33
3
3
2
¸
¹
·
¨
©
§
¸
¹
·
¨
©
§
¸
¹
·
¨
©
§
¸
¹
·
¨
©
§
(14)
In order to simplify Eq. (14), we may introduce parameter
'n by defining
'
'
443
7 Non-Newtonian Fluid and Flow
w
d
R
Q
d
n
W
S
ln
4
ln
1
3
¸
¹
·
¨
©
§
c
(15)
so that Eq. (14) can be newly expressed as
a
w
n
n
R
Q
n
n
J
S
J
c
c
¸
¹
·
¨
©
§
c
c
4
13
4
4
13
3
(16)
where
a
J
is the apparent Newtonian wall shear rate. Here, the term
'/)'( nn 413 is usually called the Rabinowitsch correction. The non-
Newtonian viscosity
K
is thus determined with a shear rate
w
J
where
w
w
w
J
W
JK
(17)
Let us examine a case of a power law fluid along with the abovemen-
tioned procedure
n
ww
m
JW
(18)
The logarithm form of Eq. (18) is
ww
nm
JW
lnlnln
(19)
By combining Eq. (19) with Eq. (16) and setting
a
w
J
J
, we have
¸
¹
·
¨
©
§
¸
¹
·
¨
©
§
c
c
3
4
ln
4
13
lnlnln
R
Q
n
n
n
nm
w
S
W
(20)
The differentiation of Eq. (20) with respect to
3
4ln RQ
S
gives
nn
R
Q
d
d
w
c
¸
¹
·
¨
©
§
3
4
ln
ln
S
W
(21)
Therefore, a flow curve of
w
W
vs
w
J
is plotted in log–log scale as shown
schematically in Fig. 7.14, where the slop is equal to the power law index
n
. The intercept p of
w
W
ln and )4ln(
3
RQ
S
at a given
wpw
JJ
and
wpw
WW
will give
m
as
444
