Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.

84
The Integmtion Methods
and
It
thus follows that
dl
dt
=
(2
+p
div
2)
dU.
(4.5)
Since the function in the integral is continuous and the region
U
is arbitrary,
we can conclude that
The necessary and suficient condition for
I
=
s,
p(x,
t)dU to be an integral
invariant is that
A
function
p
satisfying
2
+
p
div
r7
=
0,
dt
(4.6)
the previous equation is called
a
Jacobi multiplier.
Finally, let
us
observe that, by using the identity
Eq.
(4.6) can also be written in the more familiar form
2
+div(pz)
=0,
dt
which the reader has already met, for instance, in electrodynamics, where
p
has
to be identified with the
electric charge density
and
p.?
with the
current
density.
Finally, let us address that in the case of
divergenceless
vector field
d,
any constant is a Jacobi multiplier.
For
these types
of
dynamics we
get
the
important result that the
measure
p(U)
of
any region
U
does not change in
A
Primer
on
the
Lie Derivative
85
time. This is certainly the case for Hamiltonian systems
631
631
for which we have
It
has already been remarked that the Jacobian determinant of a completely
canonical transformation is
1,
and that this type
of
transformation are volume
preserving.
It
has
also already been remarked that the Hamiltonian evolution
is
itself
a completely canonical transformation between a submanifold
UO
of the phase
space
and
the submanifold
U
of the same space whose points are the evolutes,
at time
t,
of
points of
Vo,
having the Hamiltonian function as
a
generator.
Thus, for canonical systems, a double conservation of the measure holds
(Liouville
remark).
4.2
A
Primer
on
the
Lie
Derivative
Let us consider the differential system
dxi
dt
-
=
X"X),
vi
E
(1,2,
*.
.
,n),
(4.7)
where the
X's
do
not depend explicitly on time, and evaluate the rate of change
of any function
of
the coordinates
x
along the solutions of the system, shortly
the
"time" derivative.
We have
Therefore, one can naturally associate, with a differential system, the first
order differential operator
i=l
86
The
Integration
Methods
whose action on an arbitrary function
f
gives its "time" derivative. Since with
each point
x
of the space we can associate
a
vector having the real numbers
Xi(x)
as components, the previous operator
X
is also called
a
vector field.
Vice versa,
with any vector field we can associate a system of differential
equations
dx'
-
dt
=
Xi(x),
Vi
E
(1,2,.
.
.
defining the curves
xi
=
xi(t),
such that the tangent vector
v'
=
(dx'/dt,.
. .
,
dx"/dt),
in
a
generic point
x,
is just given by the components of
Xi
at point
x.
Such curves are called the
integral curves
of
the vector field X.
By denoting,
as
before, with
Q
and
QO
the points whose coordinates are
(x1,x2,.
.
.
,
z")
and
(xh,
xi,
. . .
,
x;),
respectively, the equations
represent the solutions of the differential system which, at time
to,
takes the
value
Qo.
They locally define a one-to-one global map, which depends on
a
parameter
t,
called
the
flow
generated
by
the vector
field
(Xl1 X2,.
.
.
,
X"),
satisfying the
group properties
cpo
=
identity map,
(V4-l
=
9-t
,
'Ptl
O
9tz
=
(Ptl+t2
*
If
the differential system is canonical with characteristic function
R,
the map
pt
is also called the
Hamiltonian flow generated
by
R.
The derivative of
a
function
f
along the solutions of the differential system
(4.7)
is
denoted by
and is known
as
the
Lie derivative
off
with respect
to
X.
A
Primer
on
the
Lie Derivative
87
From
equations
xi
=
xi(t, xo),
it follows
axi
dxa
=
C
-dd.
j=1
8x3,
It is then easy to evaluate the “time” derivative of the differentials
dx’.
We
obtain
d(dxi)
d
a
dxi
.
j=1
j=1
dt
It
axk
a
n
‘a
n
=
xdx3,-Xi
=
--Xi) ax; dxk
j=l
823,
j=1
(k=l
axi
axi
dXk
dXk
-
=
C
-dxk.
k=l
(4.9)
The above “time” derivative is known
as
the
Lie derivative
of
dxi with respect
to
X
and
is
denoted with
Lxdxi,
so
that the above equation can be written
in the following form:
Lxdd
=
dXi
,
without any reference to the parameter
t.
By
now using
which
also
follows from
xi
=
xi(t,xO),
it is possible to evaluate the “time”
derivative of partial derivatives
d/dxi.
We
thus get
d
da
dnaxia
axk
a
axi
axk
Lx-
=
--
=
-
c
2-
=
k=l
axi dt axz dt j=l axi 6x3,
j=l
(4.10)
The last step in the above formula is explicitly performed in the Appendix
B.
88
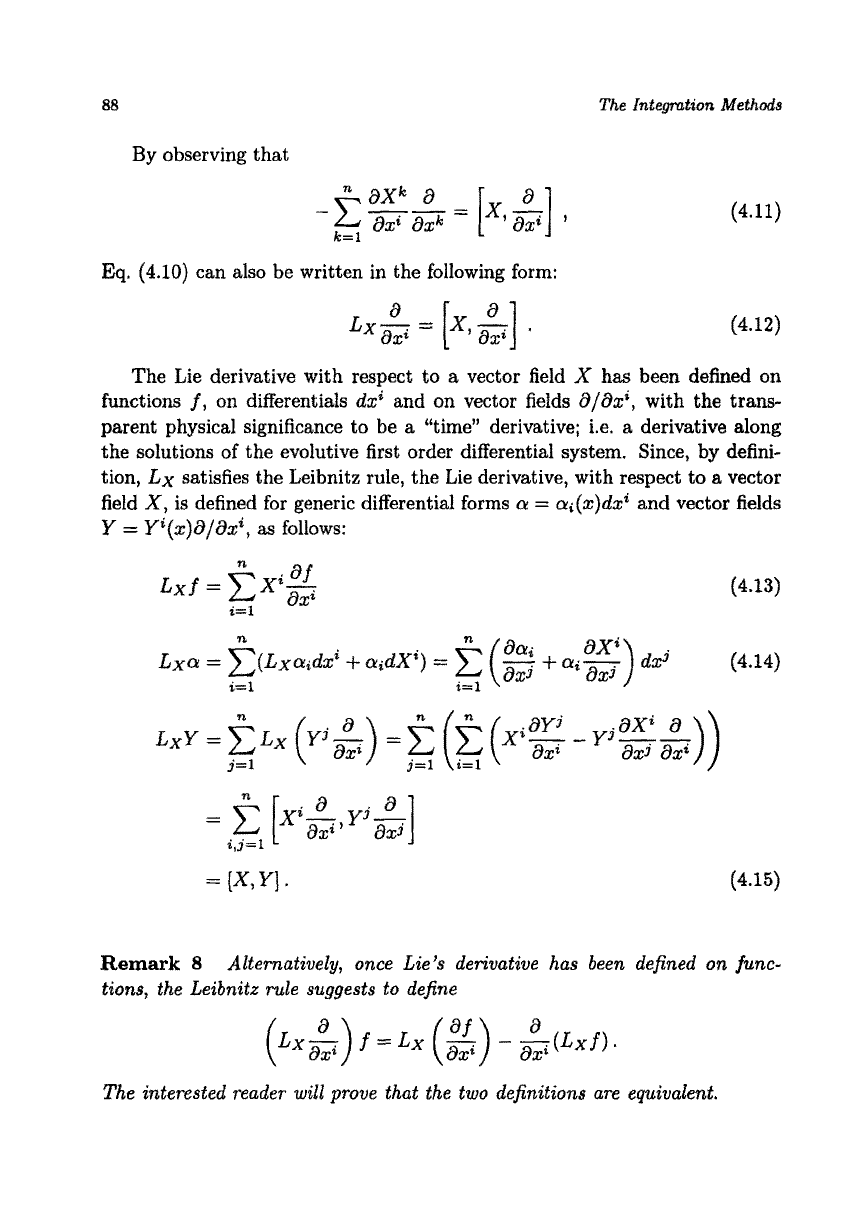
By observing that
axk
a
k=
1
Eq.
(4.10)
can also be written in the following form:
(4.11)
(4.12)
The Lie derivative with respect
to
a
vector field
X
has been defined on
functions
f,
on differentials
dxi
and on vector fields a/axi, with the trans-
parent physical significance to be
a
"time" derivative; i.e.
a
derivative along
the solutions of the evolutive first order differential system. Since,
by
defini-
tion,
Lx
satisfies the Leibnitz rule, the Lie derivative, with respect to a vector
field
X,
is
defined for generic differentia1 forms
Q
=
ai(x)dxi and vector fields
Y
=
Y~(~)a/a~~,
as
follows:
(4.13)
=
(X,Y]
(4.15)
Remark
8
tions, the Leibnitz rule suggests
to
define
Alternatively, once Lie's derivative has been defined
on
func-
(Lx&)f =Lx(g)-s(Lxf).
d
The interested reader
will
prove that the
two
definitions are equivalent.
The
Kepler
Dynamics
89
4.3
The
Kepler
Dynamics
The gravitational potential energy
of
two bodies with mass
ml
and
m2
located,
with respect to a chosen frame,
at
31
and
i5
is
given by:
where
G
=
6.6
T,
is
Nm2/kg2 is the
gravitational universal constant.
The Lagrangian function
C,
obtained subtracting
U
from
the kinetic energy
The coordinates
F1,
772
can be expressed in terms of
center
of
mass coordinate
2
and
relative
coordinate
F
defined
by
We have
+
8.
mzr'
mlr'
ml+
m2
ml
+mz
The velocities
v'l
and
$2
can also be expressed in terms
of
the center mass
velocity
+R,
$2=-
-+
rl
=
and
relative velocity
v'
as
follows:
*
mzv'
-
mlv'
v'1=v+
,
&=V-
ml
+m2
ml
fm2
The Lagrangian
L
expressed in terms
F,
8,
v'
and
becomes
1 1
k
2
2
r
~=-(ml+m2)V~+-pw~+-,
where
mlmz
ml
+mz
is the
reduced
mass.
Thus, the Lagrangian
L
is the sum
of
a
free
Lagrangian
CR
=
[(ml
+
m2)V2)/2
and
a
Lagrangian
L,
=
(1/2)pv2
-
k/r
of
a
system with
1
degree
of
freedom. The first Lagrangian describes the motion
of
the center mass which
turns out,
of
course, to be uniform. The second describes the motion
of
a
P=

95
The
Integration
Methods
particle with the reduced
mass
p
in the gravitational field force located at
center of mass coordinate. We may notice that if
m2
<<
ml,
then
p
N
m2
and
TI
-
2.
The corresponding ~ami~ton~an functionl with
m2
=
m
and
ml
=
M,
is
given by
(4.16)
It
is worth observing, by using the results of problems in the section devoted
to the Poisson bracket, that the angular momentum
L'
is a constant
of
the
motion,
so
that
{i13t)
=
0.
(4.17)
Of course the
last
property
is
shared by all central potentials; that
is,
by
all potentials
U(r>
depending only on the modulus
r
of
the vector position
F.
Therefore, the trajectory lies in the plane determined by the initial values
of position
rf,
and velocity
80,
which is the plane orthogonal to the angular
momentum
E.
4.3.1
The
Laplace-Runge-Lenr
vector
For
the Kepler potential, beyond the angular momentum
i,
there exist specific
constants of the motion expressed by the so-called
Laplace-Runge-Lenz
vector
given by
whose components are not independent
,
since
(4.18)
which simply says that the LaplaceRungct-Lenz vector lies on the plane of
the motion.
It
is
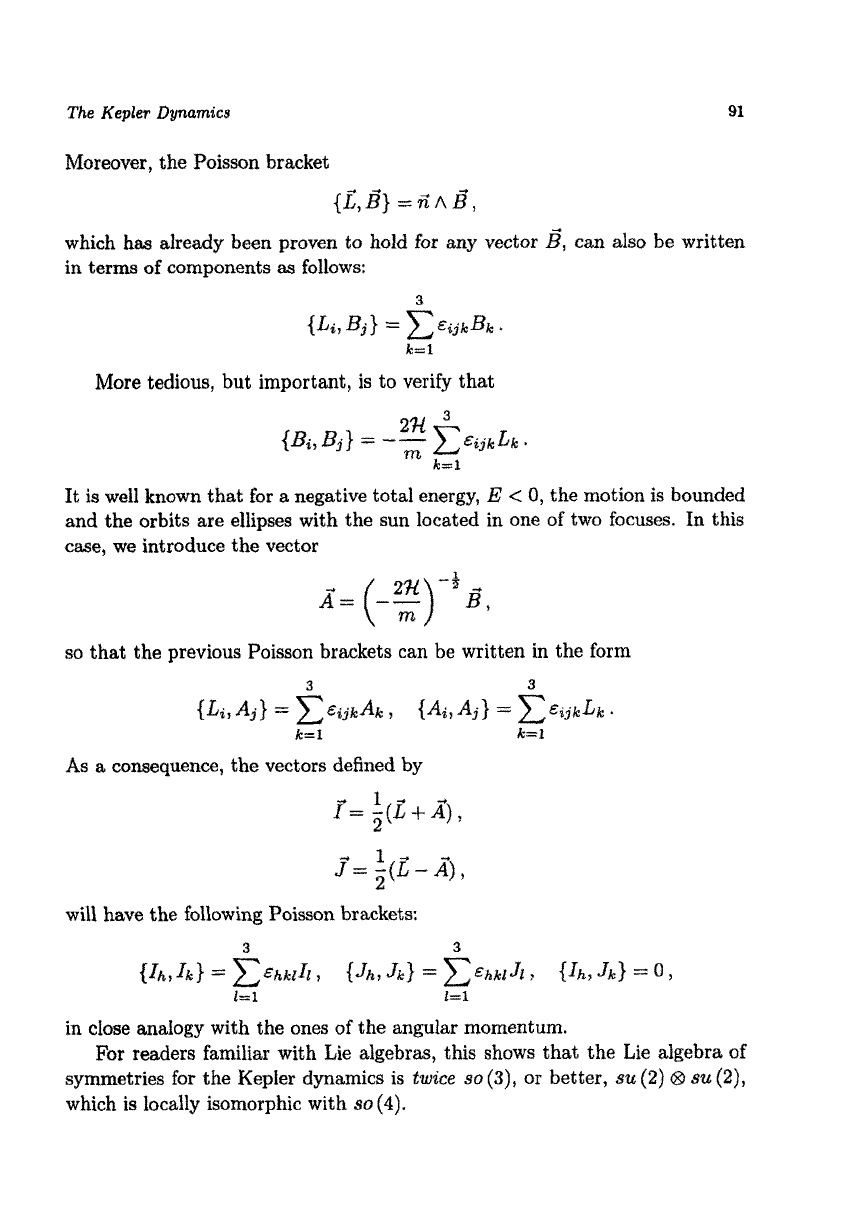
interesting to evaluate the Poisson brackets involving
B.
The reader
is invited to verify that
The
Kepler
Dynamics
91
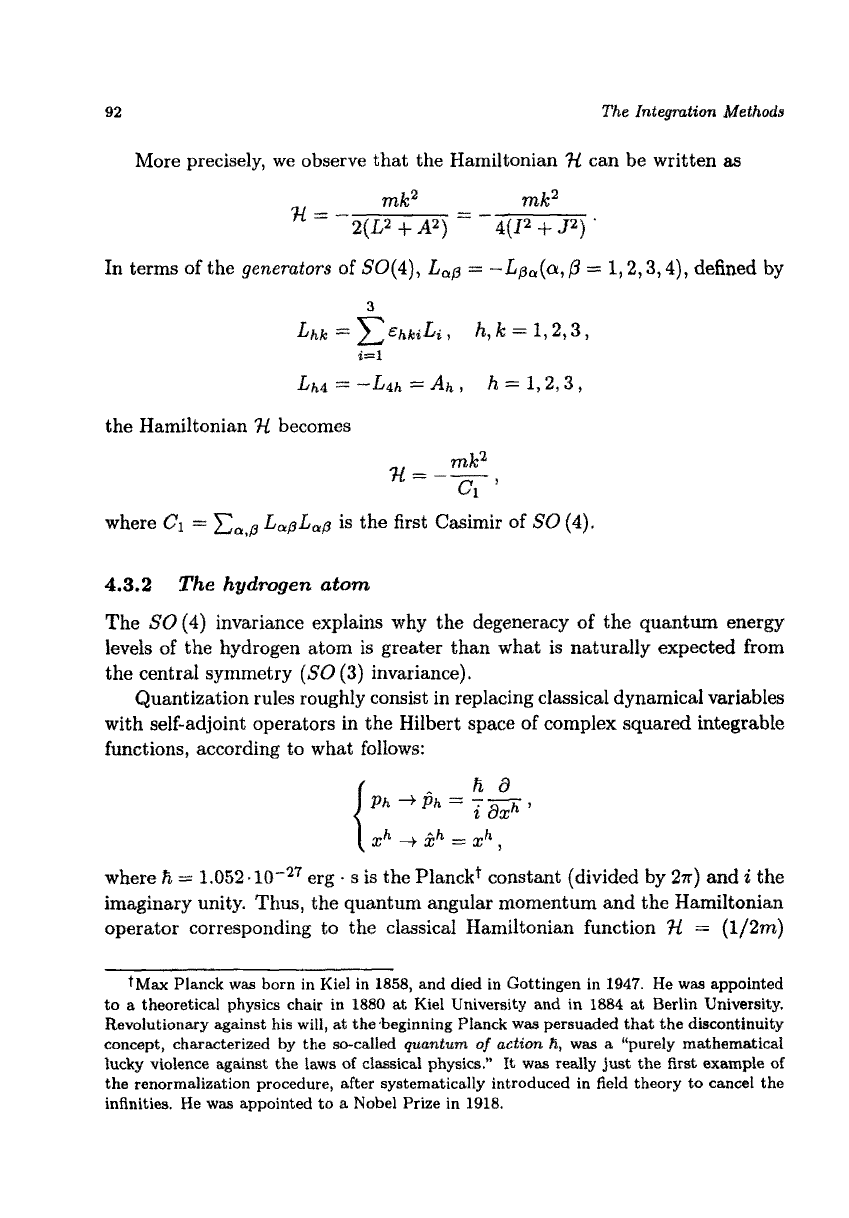
Moreover, the
Poisson
bracket
{g,g}
=n'Ag,
which has already been proven to hold for any vector
g,
can also be written
in terms
of
components
as
follows:
3
{Li,
Bj}
=
EijkBk
*
k=l
More tedious, but important,
is
to verify that
It
is
well known that for a negative total energy,
E
<
0,
the motion
is
bounded
and the orbits are ellipses with the sun located in one
of
two focuses.
In
this
case, we introduce the vector
so
that the previous Poisson brackets can be written in the form
As
a
consequence, the vectors defined by
-.
1-
2
J=
-(L-A),
will
have the following Poisson brackets:
3
3
(lh,Ik)=x&hkEIEj (Jh,Jk)=ZEhklJl,
(lh,Jk)
==o,
1=1
t=l
in
close analogy with the ones
of
the angular momentum.
For
readers familiar with Lie algebras, this shows that the Lie algebra
of
symmetries for the Kepler dynamics
is
twice
so
(31,
or
better,
su
(2)
@
su
(2),
which
is
locally isomorphic with
so
(4).
92
The
Integration
Methods
More precisely,
we
observe that the Hamiltonian
31
can be written
as
mk2
-
mk2
%=-
2(L2
+
A2)
-
-4(12
+
P)
*
In terms
of
the
generators
of
S’O(4),
La@
=
-Lp,(a,p
=
1,2,3,4),
defined
by
3
Lhk
=
EEh&,
d=l
h,k
=
1,2,3,
Lh4
z=
--Ldh
=
Ah,
h
=
1,2,3,
the Hamiltonian
3t
becomes
mk2
%=--
G1
where
C1
=
L,ijLap
is
the first Casimir
of
SO
(4).
4.3.2
The hydrogen
atom
The
SO(4)
invari~ce explains why the degeneracy of
the
qu~~~ energy
levefs
of
the hydrogen atom
is
greater than what is naturally expected from
the central symmetry
(SO
(3)
invariance).
Quantization rules roughly consist in replacing classical dynamical variables
with self-adjoint operators in the Hilbert space
of
complex squared integrable
functions, according
to
what
follows:
where
ti
=
1.052’10-27
erg
-
s
is
the Planckt constant (divided by
2n)
and
i
the
i~aginary unity. Thus, the quantum angular
moment^
and the H~iltoni~
operator corresponding to the classical Hamiltonian function
3c
=
(1/2m)
tMax
Planck was born in Kiel in
1858,
and died in Gottingen in 1947. He was appointed
to
a
theoretical physics chair in 1880
at
Kiel University and in 1884
at
Berlin University.
Revolutionary against his will, at the -beginning Planck was persuaded that the discont~nuity
concept, characterized by the so-called
qu~n~~~
of
action
h,
was
a
“purely mathematical
lucky violence against the laws
of
classical physics.” It
was
really just the
Erst
example
of
the renormalization procedure, after systematically introduced in field theory
to
cancel the
infinities. He was appointed to
a
Nobel Prize in 1918.
The
Kepler
Dynamics
93
[p’.
p’-
e2/r]
will be given by
where
V2
is
the Laplace operator and e the electric charge of the proton.
The Laplace-Runge-Lenz vector
has
to be written in the form
-1
r“
B
=
-($
A
1:
-
L’
A
8
-
e2-
2m
r’
in
order for the corr~pondi~ vector-operator, which
is
called the
Pauli
vector,
to be
a
self-adjoint operator,
Clwsical
formulae will be replaced by the corr~ponding quantum ones
3
x&hih
=
0,
(&h,
81
=
0
h
=
1,2,3
,
h=l
3
3
[ki,
Lj]
=
ifix&$jk&k,
[kd)
Bj]
=
ihxEijk&&
t
k=l k=l
where the bracket
[-,
denotes the co~utator-operator. Moreover, we have
We
can
now restrict ourselves to the Hilbert subspace which corresponds
to bound states; that
is,
to states with negative definite eigenvalues,
E
<
0,
of
fi.
In
this
subspace
we
may define another self-adjoint vector-operator
(A,,
A2,
A):
