Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.

104
Problems
1.
Find a complete integral of the Hamilton-Jacobi equation for the har-
monic oscillator with
3
degrees of freedom,
by separating the variables in spherical-polar coordinates.
pler dynamics,
2.
Find
a
complete integral
of
the ~am~lton-Ja~obi equation
for
the
Ke-
by using parabolic coordinates
(t,
v,
p),
defined by
with
<
E
[0,
+m[,
7
E
[0,
-t-oo[.
The surfaces, defined by
[
=
constant,
7
=
constant,
define two families of revolution paraboloids having the
z
axis
as
a symmetry axis.
3.
Find a complete integral
of
the Hamilton-Jacobi equation for the
Hamiltonian with
3
degrees of freedom,
describ~ng the dynamics of
a
particle in the field (~ewtoni~
or
Coulombianll~ generated by two particles, located
at
distance
a
at
IICharles Augustin Coulomb, was born in Angouldme in
1736,
and died in Paris in
1806.
He worked
as
an engineer and
was
a
member
of
the
Institute
de
Fraplce.
During
the
last
years
of
his life he has been general overseer of Paris University.
His
contributions
to
friction
laws
and
to
electromagnetism can be considered
of
basic importance.

The Liouville Theorem
on
the Complete
Integmbility
105
positions
?I,
?2.
Use elliptic coordinates
(t,
q,
cp),
defined by
5
=
uJ(p
-
1)(+
-
1)
coscp,
y
=
uJ(t2
-
1)(q2
-
1)
sincp,
{
=at77
where
u
is
an arbitrary parameter and
t
E
[l,
+m[,
q
E
[-1,1[.
(Hint:
choose
u
=
a,)
4.6
The
Liouville Theorem
on
the Complete Integrability
4.6.1
Reduction
The knowledge of
a
first integral
f
for
a
given dynamical system, described by
the equations
id
=
Xi(z/t),
vi
E
{1,2,.
. .
,m},
simplifies the integration problem, since the relation
f
(z(t)/t)
=
constant
3
fo
must be satisfied by any solution
z(t)
of the equations of motion;
of
course,
for
a
suitable choice of the constant, which depends on the initial conditions.
All
m
-
1
hypersurfaces obtained by varying the constant
fo
will
foliate
the
whole space, and each trajectory will belong to one and only one of them. The
foliation
is
called
regular,
if the hypersurfaces have the same dimension; in this
way
each
hypersurface is called
a
leaf.
Furthermore, if
an
additional functionally independent first integral
g
is
known,
a
given trajectory
also
belongs to the hypersurface
g(4t)lt)
=
go
9
defined by
g.
Each trajectory thus lies on the generically
m
-
2
dimensional in-
tersection of leaves of the two foliations.
It
follows that the knowledge of
m-
1,
functionally independent first integrals, defining regular foliations, completely
solves the integration problem, since the l-dimensional intersections of leaves
just correspond to curves representing trajectories
.
Remark
11
The previous picture
is
just the description
of
a virtual case
and
is
given
as
a motivation
for
introducing the Lie theorem below. It almost

106
The Integration Methods
never realizes, even for a simple system, as the one of a harmonic oscillator
with
2
degrees of freedom, described by the Hamiltonian
with
W~/WZ,
an irrational number. A fine global coordinate analysis can be
found
in
the Wintner
in
which the notion of isolating integral (also less
correctly called uniform) is introduced. An integral
f
(x)
is said to be isolating
if
it
“can enable one to make predictions concerning the possible future (or past)
positions of the points x
=
x(t)
of the solution path which goes at
t
=
0
through
xo
(the case x(t)
=
xo
of an equilibrium solution being not excluded).”
Thus, it is naturally expected that, for a canonical system, the knowledge
of
2n
-
1,
functionally independent first integrals, defining regular foliations,
completely solves the integration problem.
Actually, for a canonical system with
n
degrees of freedom (and with
a
2n-dimensional phase space
a),
it turns out that the integration problem is
completely solved knowing only
n
functionally independent first integrals
fi,
which are in
involution;
that
is,
such that
More precisely, the following remarkable theorem
was
proven by Sophus
J,ie.134,25
Theorem
15
(Lie)
If for a canonical system of rank
2n,
m
functionally
in-
dependent and involutive first integrals are known, which can be
solved
with
respect
to
m
of
the p’s, the integration problem reduces to the integration of a
new canonical system
of
rank
n
-
m.
In other words, while for
a
generic first order differential system the knowl-
edge of
m
first integrals reduces the rank by
m
units, for
a
Hamiltonian system
the rank is reduced by 2m units.
It
is
interesting, for concrete applications, the case in which
m
=
n;
that is,
the case in which the number of such first integrals is just equal to the number
n
of degrees of freedom.

The
Liouville
Theorem
on
the Complete Integrability
107
4.6.2
The Liovville theorem
Theorem
16
(Liouville)
If
for
a Hamiltonian svstem,
n
functionally inde-
pendent and involutive first integrals are known, which can be solved with
re-
spect
to
the p’s, the integration problem reduces
to
pure quadratures; that
is,
the equations
of
motion can be solved simply by evaluating integrals.
The proof will be carried out by means of the Hamilton-Jacobi integration
method. According to this method, in order to have the general integral of
a
canonical system
it is sufficient to find a complete integral
V
of
the partial differential equation
(4.26)
Then the Liouville statement will be proven
if
we show that the knowledge
of
n
first integrals
fr(p/q/t),
with
r
E
(1,.
.
.
,
n}
of the canonical system,
satisfyiig the following properties:
(i) are functionally independent; i.e.
(ii)
are in involution; i.e.
{fp,
fa}
=
0,
r,
s
E
(1,.
. .
,
n},
(iii)
and define an algebraic system
ft(p/q/t)
=
Ahdfh
=
0
*
Ah
=
0,
solvable with respect
to the
n
variables
p,;
i.e.
allows
us
to determine,
by
pure quadratures, a complete integral
of
Eq.
(4.26).
Let
us
first consider the case in which the Hamiltonian
31
and the functions
fr
do not explicitly depend on the time
t.
The equations
fr(P/q)=nr
r~
{1l***ln}~
(4.27)
for fixed
xls,
define
a
submanifold
Mf(=),
known
as
the level manifold,
of the
phase space
a.
By using hypothesis (iii),
Eq.
(4.27)
can be solved with respect
to
p’s
in the form
pa
=
cpa(a/n),
a
E
(1,.
.
.
,n}
I
108
The Integration Methods
Of
course, on the level manifold
M,
the differences
pa
-
cp,(q/n),
and conse-
quently, the Poisson brackets
{pa
-
Va(q/r),pp
-
cpp(q/n)}
vanish identically.
Furthermore, at the end
of
the section, we will show that the involutivity
of
the
f's
on
the whole phase space
@
implies the vanishing of the Poisson
brackets
{pm
-
pa(q/n),pp
-
(pp(q/n)}
on the whole phase space
a,
namely
On the other hand,
so
that
which implies that the differential form
n
h=l
is closed, or which is the same, locally exact. In other words, in any simply
connected part of
AdT,
a function
W(q/n)
exists, such that
(4.28)
In
this way, it
has
been shown that the involutivity of the
f's
implies that the
solutions
of
the
Eq.
(4.27),
with respect to the
p's,
can be locally expressed
as
follows:
Let
us
now define
n
new coordinates, namely
XI,
XZ,
.
. .
,
xn,
by
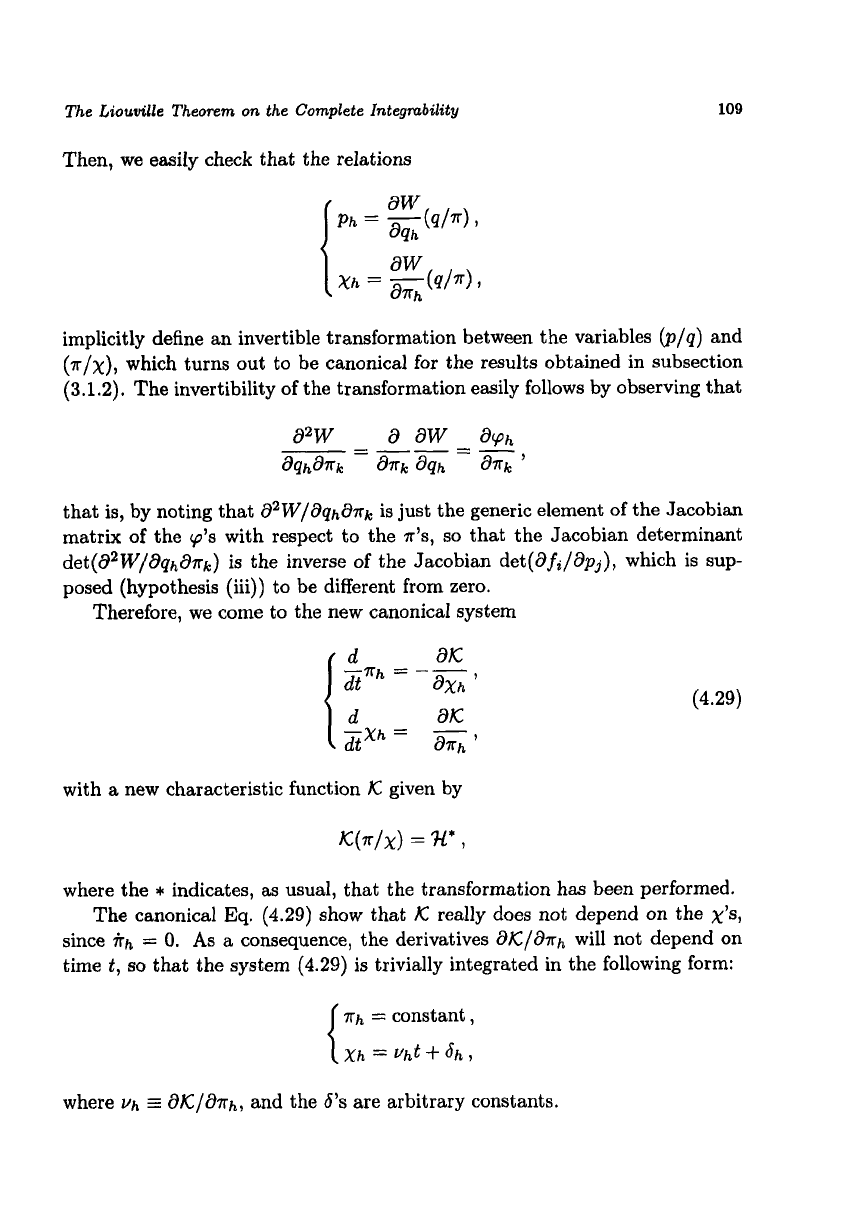
1
The
Liouville
Theorem on the Complete Integrability
’
Then, we easily check that the relations
109
implicitly define
an
invertible transformation between the variables
b/q)
and
(T/x),
which turns out to be canonical for the results obtained in subsection
(3.1.2).
The invertibility
of
the transformation easily follows by observing that
that is, by noting that
d2W/&h8Tk
is just the generic element of the Jacobian
matrix of the
p’s
with respect to the
T’S,
so
that the Jacobian determinant
det(a2W/8qh&rk) is the inverse
of
the Jacobian det(dfa/apj), which is sup-
posed (hypothesis (iii)) to be different from zero.
Therefore, we come to the new canonical system
(4.29)
with
a
new characteristic function
K:
given by
where the
*
indicates,
as
usual, that the transformation has been performed.
The canonical Eq.
(4.29)
show that
K
really does not depend on the
x’s,
since ??h
=
0.
As
a
consequence, the derivatives
aX/d?rh
will not depend on
time
t,
so
that the system
(4.29)
is trivially integrated in the following form:
where
Vh
3
bx/d?rh,
and the
6’s
are arbitrary constants.
110
The
Integration
Methods
The general case can be treated by introducing two additional auxiliary
parameters
PO,
qo,
and the
double
bracket
du
dv
du
dv
,
.
Of
course, if
u
and
v
do not depend on
PO,
and then
Furthermore, by choosing
qo
=
t,
we have
In terms of the double bracket, Liouville’s hypotheses on the knowledge of
n
involutive first integrals
fr(p/q/t),
r
E
(1,.
.
.
,
n}
can be expressed
as
follows:
{{fp,
fv))
=
0
,
P,W
E
{0,1,.
.
.
,n)
,
with
fo
=
PO
+
Ift
.
system
On the other hand, from the same hypotheses it follows that the algebraic
fr(P/q/t)
=
rr
7
p0+31=0,
can be solved in the form
pa
-
pa(q/r/t)
=
0,
a
E
{0,1,.
I
.
,
n}
,
(4.30)
with
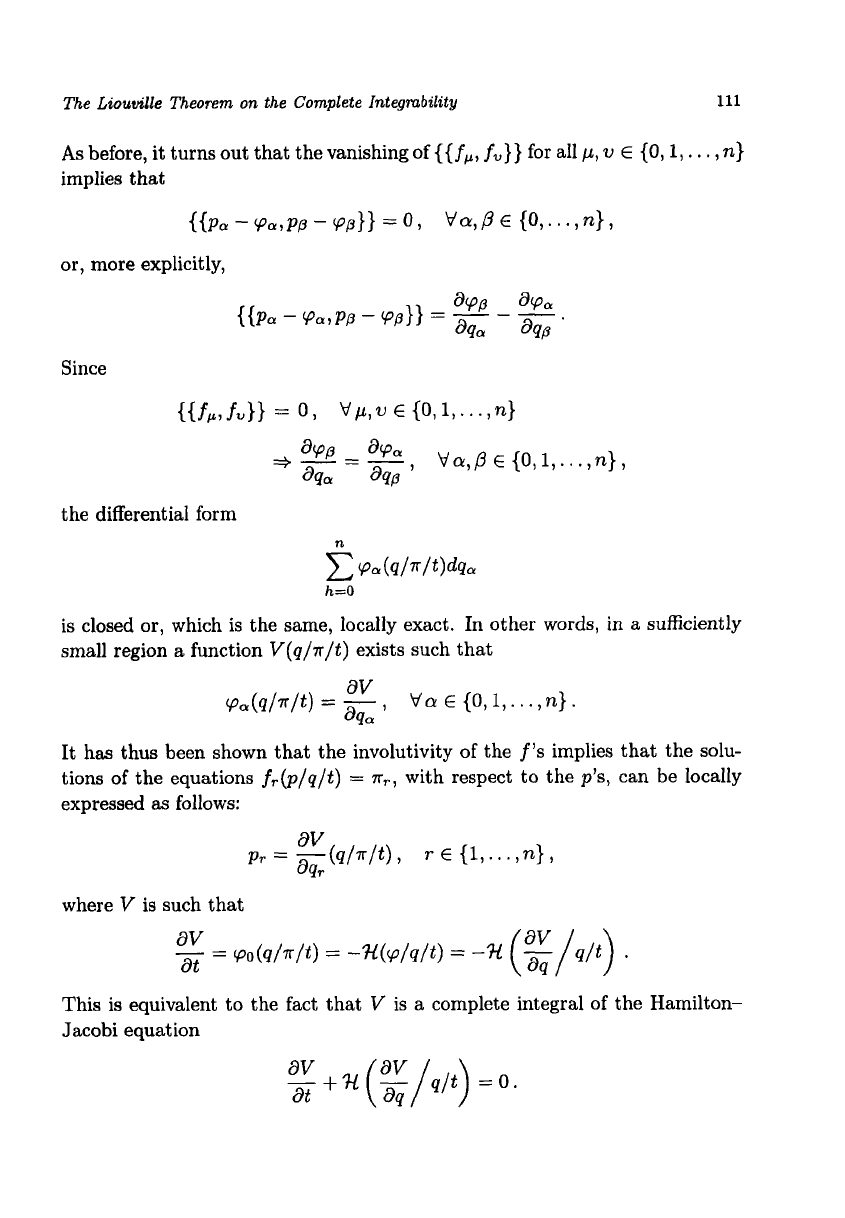
The
LiouvdlEe
Theorem on the Complete Integrability
111
As
before,
it
turns out that the vanishing of
{{fp,
fv}}
for all
p,
w
E
(0,
1,
. .
.
,
n}
implies
that
{{Pa-cpa,Pp-cpp)}
=o,
Va,PE {O,...,nL
or, more explicitly,
the differential form
n
h=O
is closed or, which is the same, locally exact. In other words, in a sufficiently
small region
a
function
V(q/n/t)
exists such that
cpa(q/n/t)
=
z,
va
E
{0,1,.
. .
,n}
.
a9a
It
has thus been shown that the involutivity of the
f’s
implies that the solu-
tions of the equations
f,(p/q/t)
=
nr,
with respect to the
p’s,
can be locally
expressed
as
follows:
f3V
8%
Pr
=
-(9/n/t)
,
7-
E
(1,.
.
. ,
n}
,
where
V
is such that
This is equivalent to the fact that
V
is
a
complete integral
of
the Hamilton-
Jacobi equation
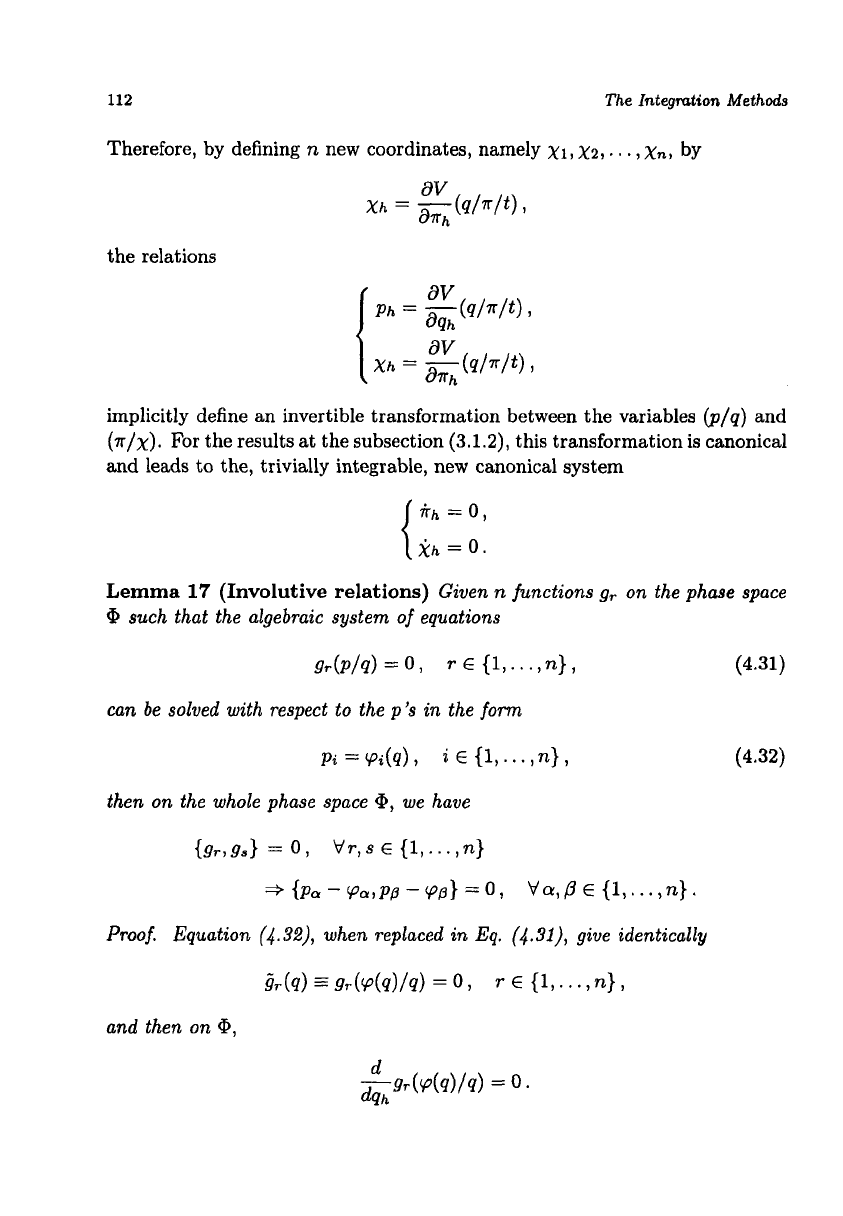
112
The Integmtaon Methods
Therefore, by defining
n
new coordinates, namely
XI,
~2,~
. .
xn1
by
the relations
implicitly define an invertible transformation between the variables
(p/q)
and
(r/x).
For
the results at the subsection
(3.1.2)1
this transformation is canonical
and
leads to the, trivially integrable, new canonical system
kh
=o,
xh
=
0.
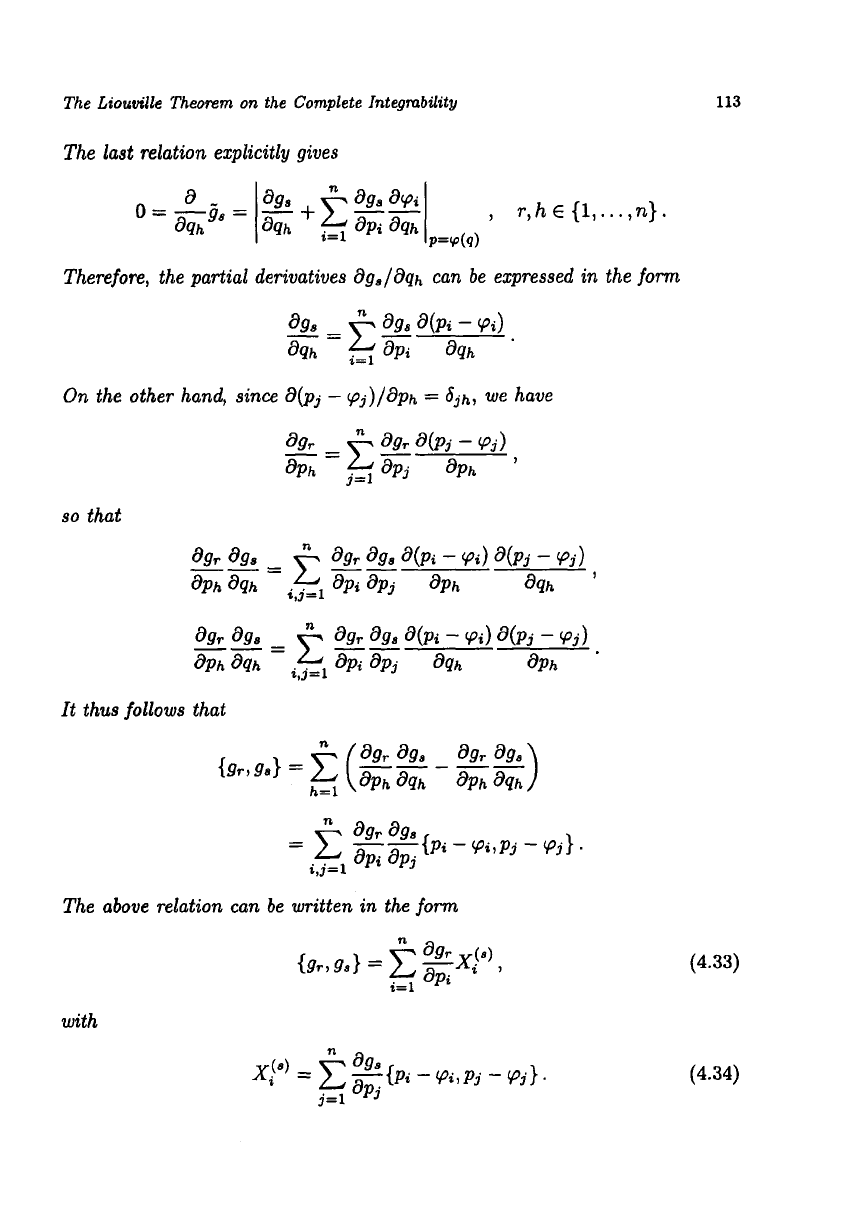
Lemma
17
(Involutive relations)
Given
n
functions gr on the phase space
such that the algebraic system
of
equations
can be solved with respect to the p’s
in
the
form
Pa=(Pi(q),
iE
{ll...,n}l
then on the whole phase space
a1
we have
and then on
Qi,
(4.32)
The
Liouville
Theorem
on
the
Complete
Integmbility
The last relation explacitly gives
Therefore, the partial derivatives
aga/aqh
can be expressed in the form
n
ag.9
--
aqh
i=l
On the other hand, since
a(pj
-
Cpj)/aph
=
bjh,
we have
n
,
so
that
It thus follows that
The above relation can
be
written in the
form
with
113
(4.33)
(4.34)
