Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.


94
The Integration Methods
so
that the previous commutation relations can be written in the form
3 3
k=l
k=l
As
a
consequence, the operators defined by
jk
=
’(Lk
-
Ah)
,
2
will satisfy the following commutation relations:
The Hamiltonian operator will thus be
and by observing that
it can be finally written
as
me4
2(4f2
+
li2)
H=-
The previous formula allows
us
to find the {quantum) energy spectrum
En,
and the corresponding degeneracy, of the hydrogen atom, by using
only
the
knowledge of the irreducible representations of
SU
(2)
@
SU
(2),
without any
mention to the Schrodingert equation.
%‘Erwin Schriidinger was born in Vienna in
1887,
and died there in
1961.
After his degree,
obtained at Vienna University in
1906,
he moved to Stoccard, Zurich and Berlin. After
Hitler’s advent, Schrodinger moved to Oxford and Dublin. Finally, he returned to Vienna
in
1956.
According to Niels Bohr, he was
a
‘‘universal man”; indeed he was
a
scientist with
large cultural interests covering physics, philosophy, politics,
biology
and classical Greek
culture. He established the basic equation
of
nonrelativistic quantum mechanics, and was
appointed, together with Dirac,
to
the Nobel
Prize
in
1933.
The Hamilton-Jacobi Integration Method
95
fn
fact,
since the operators
fh
satisfy the co~utation relations
of
an an-
gular momentum,
f2
can be quantized accordingly:
1’
=
~’~(~
+
l),
with
2
integer
or
half integer,
so
that the energy levels
of
the hydrogen atom will be
given by
me4
€3,
=
-
which
is,
of
course, Bohr’sl formula, with
n
=
22
+
1.
-
me4
2tia(21+
112
-
-m
’
The degeneracy
of
the energy levels will be
n-1
n-1 n-1
degE,
=
c(21
f
1)
=
n2
=
D
1=1
where
D(i,j>
denotes the dimension of the
(i,
j)-irreducible representation of
su
(2)
€3
su
(2)
N
so
(4):
D(i,j)
=
(2i
+
1)(2j
+
1).
4.4
The
Hamilton-Jacobi Integration Method
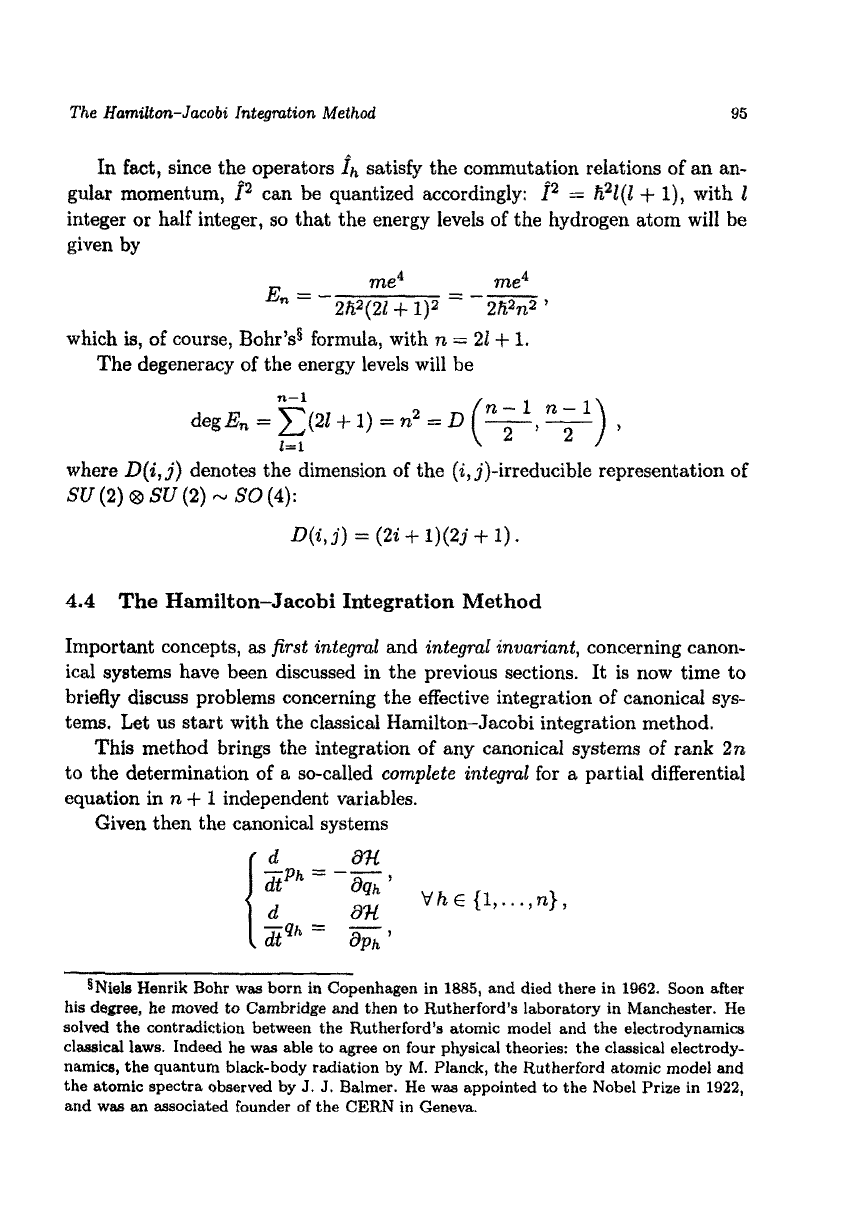
Important concepts,
as
first integral and integral invariant, concerning canon-
ical systems have been discussed in the previous sections. It is now time to
briefly discuss problems concerning the effective integration
of
canonical sys-
tems.
Let
us
start with the classical Hamilton-Jacobi integr~tion method.
This
method brings the integration
of
any canonical systems
of
rank
2n
to the determination
of
a so-called complete integral for a partial differential
equation in
n
+
1
independent variables.
Given then the canonical systems
SNiels
Henrik Bohr
was
born in Copenhagen in 1885, and died there in 1962. Soon after
his
degree, he moved
to
Cambridge and then
to
Rutherford’s laboratory in Manchester. He
solved the contradiction between the Rutherford’s atomic model and the electrodynamics
classical
laws.
Indeed he
was
able
to
agree on four physical theories: the classical electrody-
namics, the quantum black-body radiation by
M.
Planck, the Rutherford atomic model and
the atomic spectra observed by
J.
J.
Balmer.
He
was appointed to the Nobel Prize in 1922,
and
was
an
associated founder
of
the CERN in Geneva.
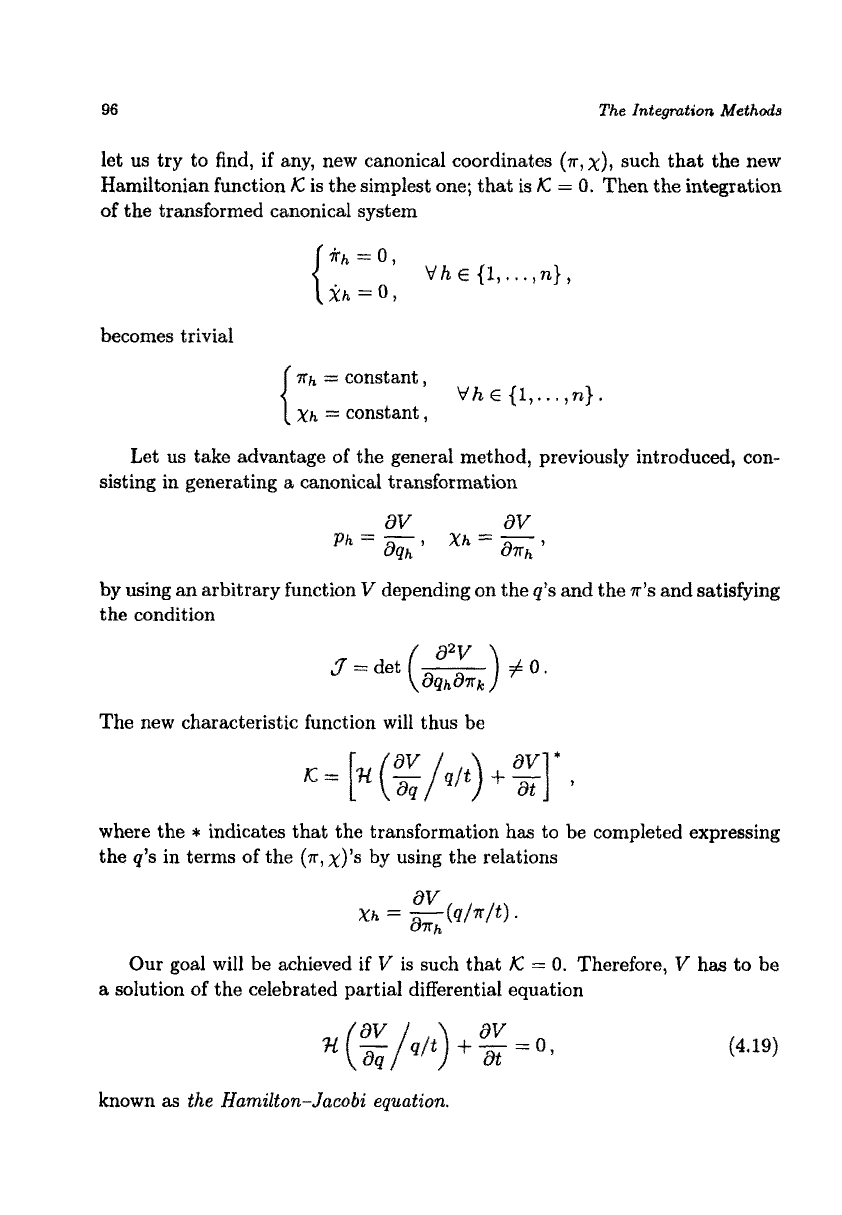
96
The
Integration
Methods
let
us
try to find,
if
any, new canonical coordinates
(n,~),
such that the new
Hamiltonian function
K:
is
the simplest one; that is
K:
=
0.
Then the integration
of
the transformed canonical system
becomes trivial
7fh
=
constant,
Xh
=
constant
Vhe
{1,
...,
n}.
Let
us
take advantage of the general method, previously introduced, con-
sisting in generating
a
canonical transformation
av
av
ph=->
Xh=-,
aqh anh
by using an arbitrary function
V
depending on the
q’s
and the
T’S
and satisfying
the condition
,.7
=
det
(-------)
a2V
#
0.
aqhark
The new characteristic function
will
thus be
where the
*
indicates that the transformation has to be completed expressing
the
q’s
in terms of the
(nl
x)’s
by using the relations
Our goal will be achieved
if
V
is
such that
K:
=
0.
Therefore,
V
has to be
a
solution of the celebrated partial differential equation
(4.19)
known
as
the Hamilton-Jacobi equation.
The
Hamilton-Jawbi
Integmtion
Method
97
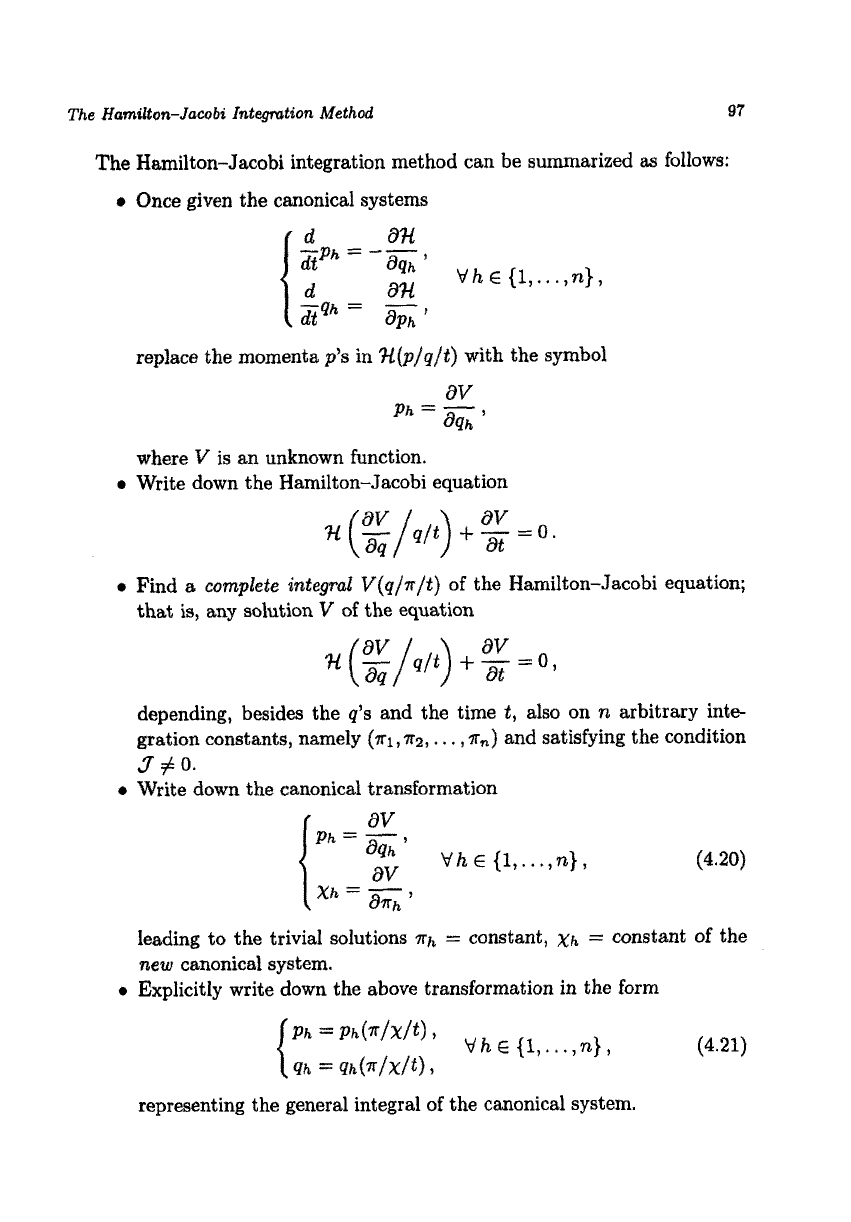
The Hamilton-Jacobi integration method can be summarized
as
follows:
Once given the canonical systems
d
a31
aqh
a31
&tph
=
--
{~
aph
'
tlh~
{I,
...,
n},
-qh=
-
replace
the momenta
p's
in
~~p/q/t~
with the symbol
av
ph=-t
&h
where
V
is
an un~own function.
Write down the Hamilton-Jacobi equation
Find
a
complete
integral
V(q/T/t)
of
the Hamilton-Jacobi equation;
that is, any solution
V
of
the equation
depending, besides the
q's
and the time
t,
also on
n
arbitrary inte-
gration constants, namely
(~Q,RZ,.
+
.
,
zn)
and sa~isfy~g the condition
Write clown the canonical trans€ormation
3#0.
dV
aqh
Vhe
{1,
...,
n),
(4.20)
Ph=-r
av
Xh=-,
i
anh
leading to the trivial solutions
Th
=
constant,
Xh
=
constant
of
the
new
canonical system.
Explicitly write
down
the above transformation in the form
representing the general integral
of
the canonical system.
98
The
Integration
Methods
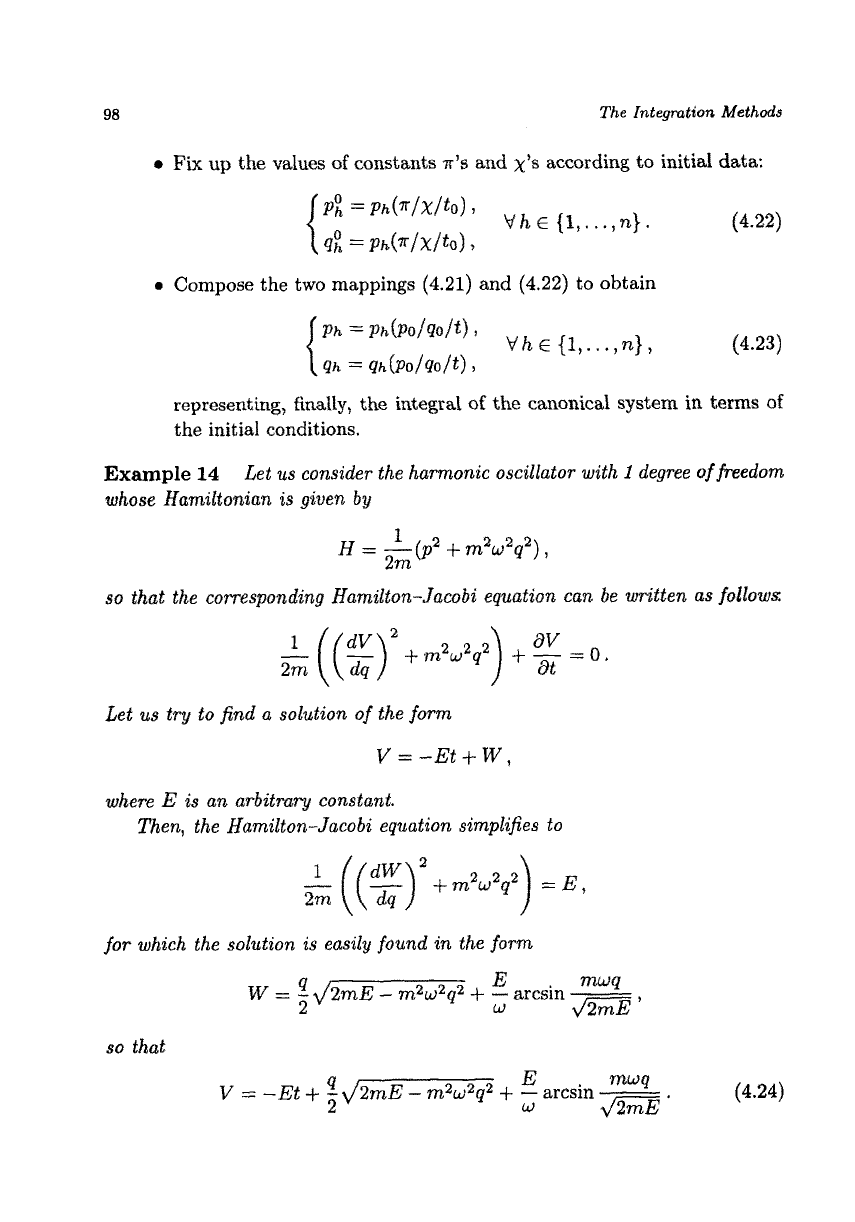
Fix
up
the values
of
constants
7~'s
and
x's
according
to
initial data:
0
Compose the two mappings
(4.21)
and
(4.22)
to
obtain
representing,
finaily,
the integral
of
the canonical system
in
terms
of
the
initial conditions.
Example
14
whose ~a~i~~on~an
is
given
by
Let
us
consider the harmonic oscillator with
1
degree
of
freedom
H=%(d+m
1
2
w
22
Q
>I
so
that the co~~sponding Numil~on-Jacobi eq~at~on can be
itt ten
as
fo~~o~~
Let
us
try to
find
a
solution
of
the form
V
=:
-Et
+-
W,
where
E
is
an arbi~~ry constant.
Then, the Namilton-Jacobi equation simplifies to
for which the solution is easily found
in
the
form
4
E
mu4
2
W
&GiZ'
W
=
-
d2mE
-
m2w2q2
+
-
arcsin
-
so
that
(4.24)
Q
E
mwq
2
W
&z'
V
=
-Et
+
-
d2mE
-
m2w2q2
+
-
arcsin
-
The Hamilton-Jucobi
Integration
Method
99
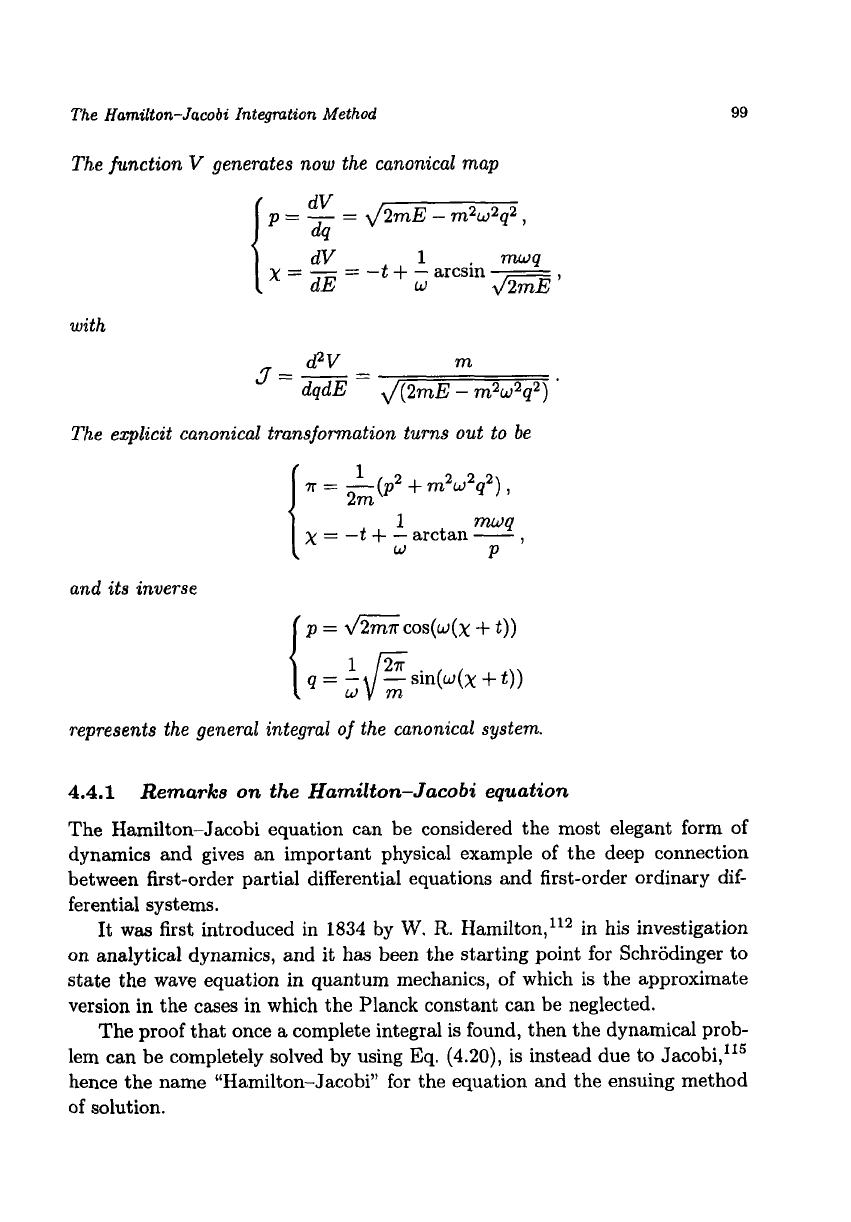
The function
V
generates now the canonical map
2mE
-
m2w2q2,
dV
1
,
wq
1
x
=
=
-t
+
-
arcsin
-
W
&GE'
with
-
d2V
m
J=--
dqdE
-
J(2mE
-
m2w2q2)
*
The explicit canonical transformation turns out
to
be
1
mwq
x
=
-t
+
-
arctan
-
,
I
W
P
and its inverse
(
p
=
&cos(w(x
+
t))
represents the general integral
of
the canonical system.
4.4.1
The Hamilton-Jacobi equation can be considered the most elegant form of
dynamics and gives an important physical example of the deep connection
between first-order partial differential equations and first-order ordinary dif-
ferential systems.
It was first introduced in
1834
by
W.
R.
Hamilton,'12 in his investigation
on analytical dynamics, and it has been the starting point for Schrodinger to
state the wave equation in quantum mechanics, of which is the approximate
version in the cases in which the Planck constant can be neglected.
The proof that once a complete integral is found, then the dynamical prob-
lem can be completely solved by using Eq.
(4.20),
is instead due to Ja~obi,"~
hence the name "Hamilton-Jacobi" for the equation and the ensuing method
of solution.
Remarks
on
the Hamilton-Jacobi equation
100 The ~nte~t~o~
Methods
Each complete integral of the H~ilton-J~obi equation gives rise
to
a
family of solutions of Hamilton’s equations, and according to Dira~v,~~
“while
the famzly does not have any importance
from
the point of view of Newtonian
mechanics,
. .
.
it..
.
corresponds
to
one state of motion
in
the quantum theory,
so
presumably the family has some deep significance
in
nature, not
yet
properly
understood.”
Once the full dynamical problem has already been solved, an explicit solu-
tion
of
the Hamilton-Jacobi equation
is
given
by
where
t
and fare two time-instants,
q’
=
dq/dr,
Ifl
and
L
the Hamiltonian and
the Lagrangian functions, and the integral has to be taken along the actual
trajectory of the dynamical system. The right-hand side
of
the above equa-
tion does indeed satisfy the Hamilton-Jacobi equation and also the additional
equation32
Remark
9
rence
t
-
f,
so
that
For conservative systems,
S
depends actually only
on
the dzf’e-
qPau1 Adrien Maurice
Dirac
was born in Bristol in
1902,
and died in
1984.
After his
degree, obtained
at
Bristof University in
1921,
he moved to Cambridge University.
In
this
university, he was Lucasian professor,
a
chair already covered by Newton, from the year
1932.
Dirac has been one
of
the most important physicist
of
our age and can be considered
the father
of
modern physics.
We
just need to mention the
Dirac
e~~~~~~n predicting the
existence
of
the positron and more generally of antiparticles, the Femi-Dirac statistics
and the constraints
method,
which
is
an essential tool
for
the Hamiltonian formulation
of
Einstein’s equation, considered then
as
a
step towards
a
quantum theory
of
gravity. The
constraints method has been also
a
fundamental step for the quantization of gauge theories.
His
books
are now considered
as
classical works. Together with Schrodinger,
Dirac
was
appointed
to
the Nobel Prize in
1933.
The
Hamilton-Jacobi
Equation
for
the
Kepler
Potential
101
4.5
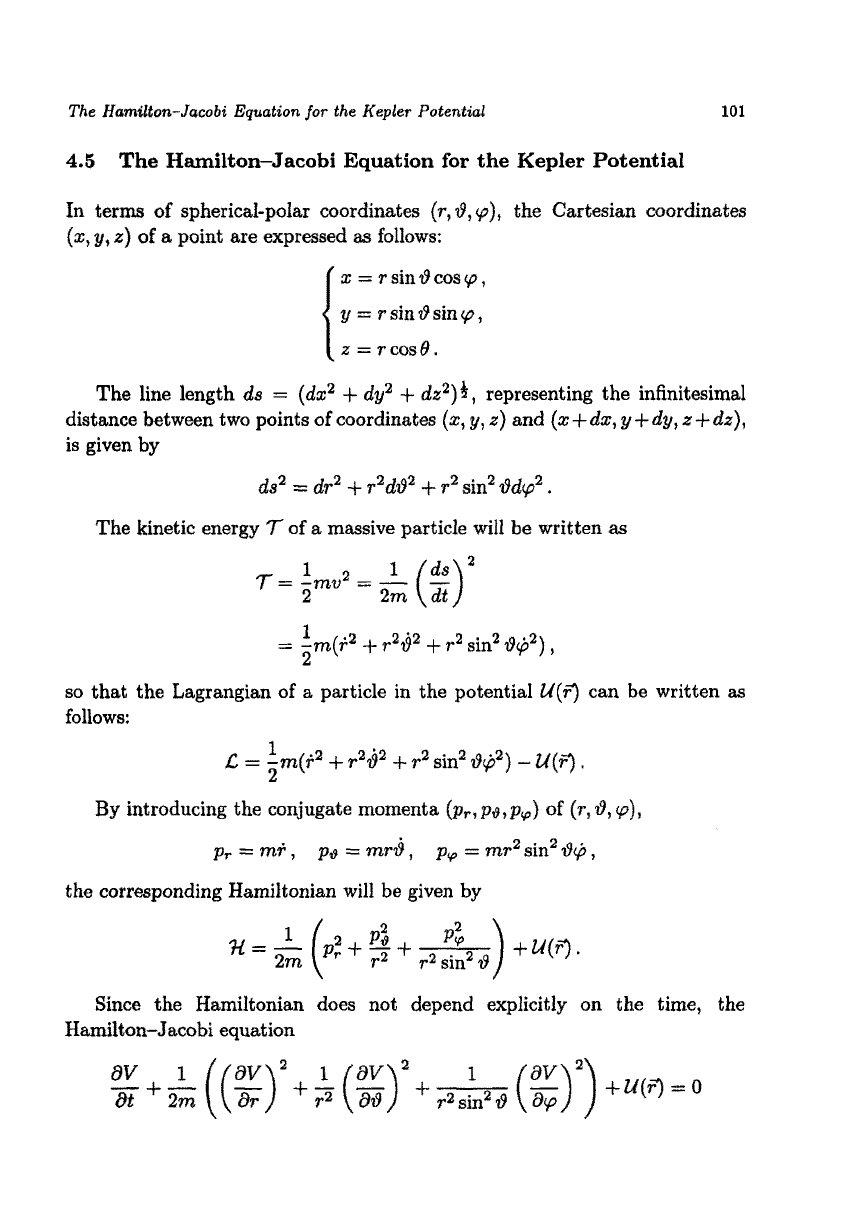
The Hamilton-Jacobi Equation for the Kepler Potential
In terms
of
spherical-polar coordinates
(T,
19,
p),
the Cartesian coordinates
(2,
y,
z)
of
a
point are expressed
as
follows:
x
=
rsinGcosp,
y
=
rsingsincp,
z
=
rcose.
The line length
ds
=
(da2
+
dg2
+
dz2)
4,
representing the infinitesimal
distance between two points
of
coordinates
(x,
y,
z)
and
(x+dx,
y+dy,
z+dz),
is
given
by
ds2
=
dr2
+
r2d@
+
r2
sin2
29dp2.
The kinetic energy
7
of
a massive particle will be writte~
as
=
5n(+2
+
r262
+
r2
sin2
t+2)
,
2
so
that the Lagrangian
of
a
particle in the potential
U(3
follows:
can be written
as
1
t
=
-rn(t2
+
~~9~
+
r2
sin2
.~(t+~)
-
~(3.
2
By
introducing the conjugate momenta
(pr,p~,p,)
of
(r,d,
p),
pe
=
mr9
,
pr
=
m+
,
p,
=
mT2
sin2
#+,
the corresponding Hamiltonian will be given by
Since the Hamiltonian does not depend explicitly on the time, the
Hamilton-Jacobi equation

102
The Integration Methods
can be reduced, with
V
=
W
-
Et
,
to the form
For
a
central potential
U(r),
it is possible to find
a
complete integral
of
previous equation by using the
method
of
separation
of
variables,
which consists
of
searching for
a
solution
W(r,
6,
cp)
of the form
W(r,
6,
cp)
=
Wr(r>
+
WG(4
+
W,(cp>
;
that
is,
for
a
solution that
is
the sum of three different functions
W,,
W,q
and
W,,
each one depends only on one of the variables
r,
6
and
cp.
In this
way,
the Hamilton-Jacobi equation for
W
becomes
and with
a
simple manipulation, it can be written in the form
1
dW8
2
(2)
=r2sin26
2m[E-U(r)]
-
($)2-
7
(w,”)
.
The left-hand side of the above equation depends only on
cp,
while the right-
hand side depends only on
r
and
19.
Since the variables
r,6
and
cp
are inde-
pendent, each side
must
be equal to
a
constant, namely
7r;.
Thus, we obtain
Once again we observe that the left-hand side depends only on
19,
while the
right-hand side depends only on
r.
Since
r
and
6
are independent variables,
both sides must be equal to
a
constant, namely
T:.
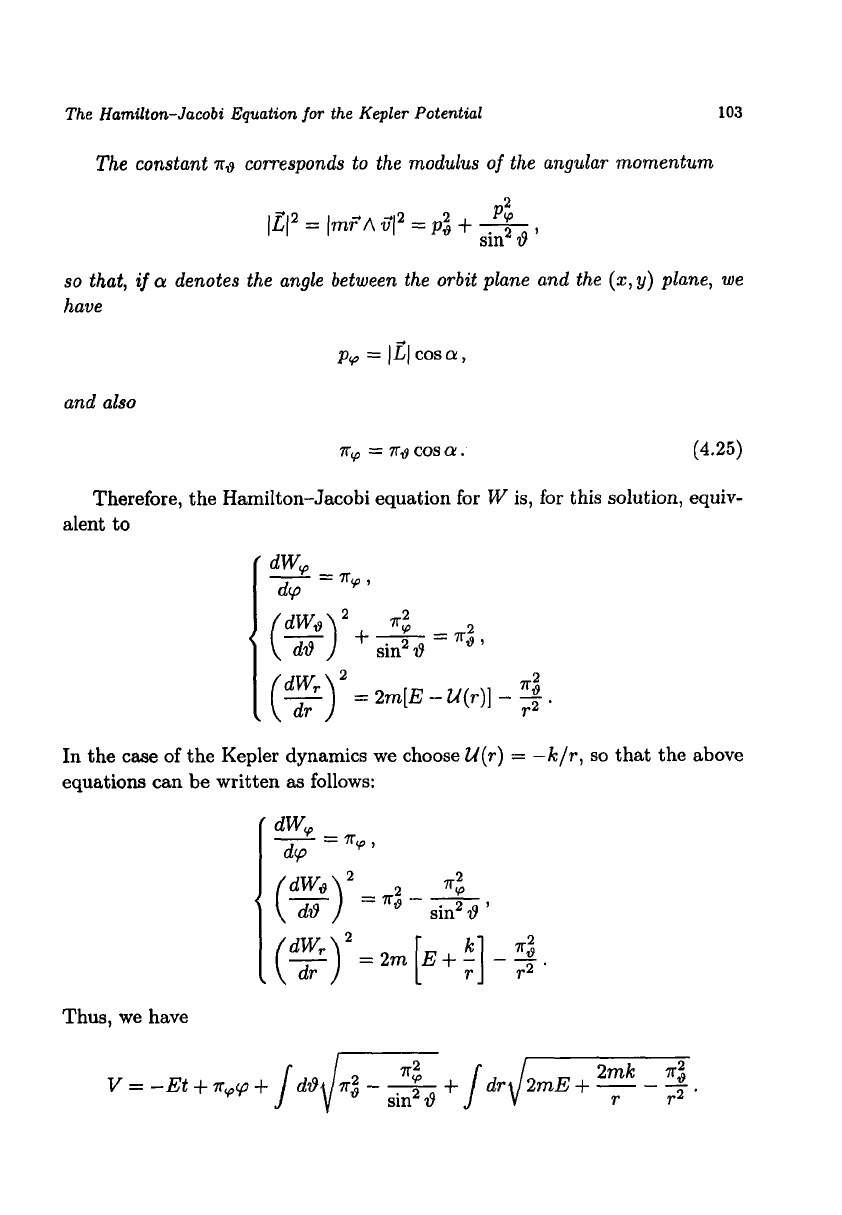
Remark
10
The
constant
T,
has a clear physical meaning:
it
simply
cor-
responds to the component p,
of
the angular momentum along the
z
axis.
Thus,
it
expresses the uniformity
of
the spanning, by the projected vector ra-
dius
8
=
F-
t.&
of
the areas
in
the
(x,
y) plane.
The
Hamilton-Jacobi Equation
for the Kepler
Potential
103
The constant
~fi
corresponds to the modulus
of
the angular momentum
so
that,
if
a
denotes the angle between the orbit plane and the
(x,
y)
plane, we
have
p,
=
$1
cosa
,
and also
T,
=
7r~COSa!.
(4.25)
Therefore, the Hamilton-Jacobi equation for
W
is,
for this solution, equiv-
alent to
In the case of the Kepler dynamics we choose
U(r)
=
-k/r,
so
that the above
equations can be written
as
follows:
Thus,
we have
