Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.

74
Tlvlnsformation
Theom
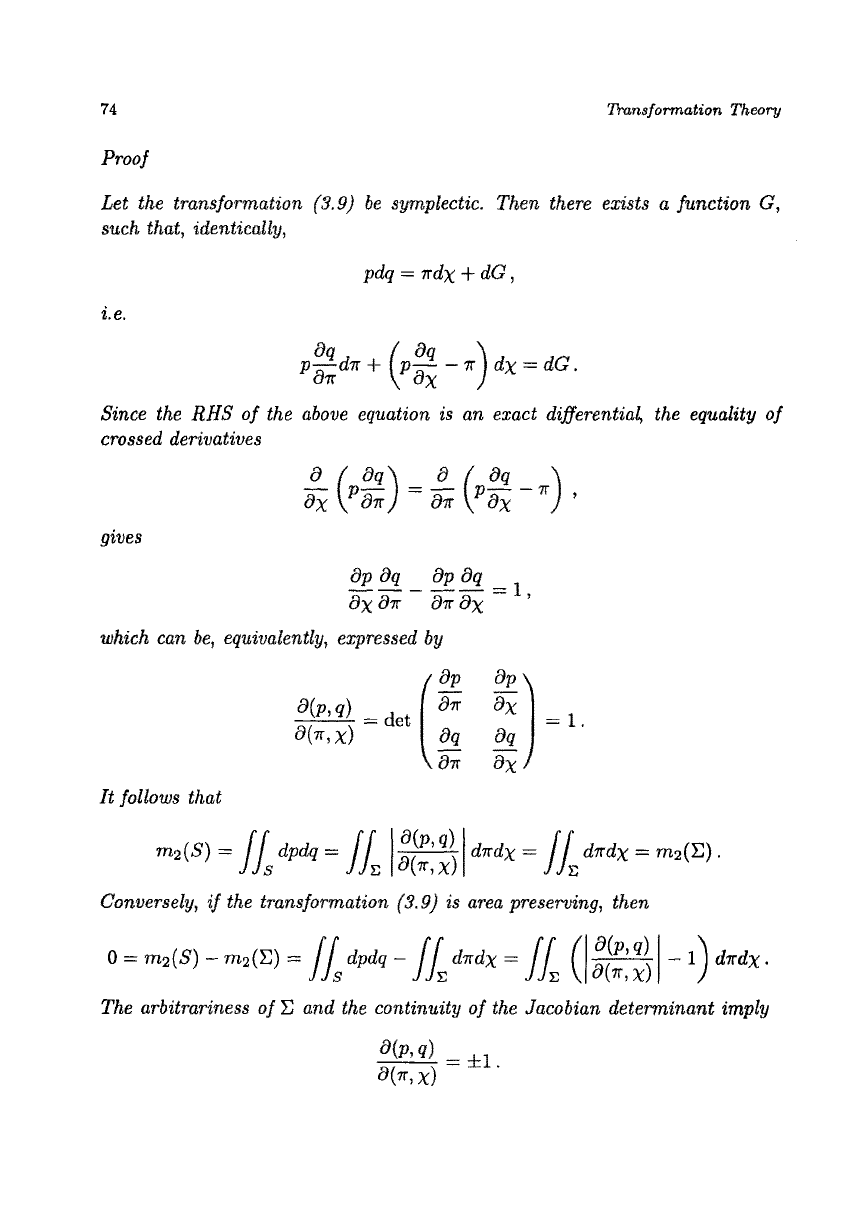
Pro0
f
Let the trunsformution
(3.9)
be ~ymplectic. Then there exists a funct~on
G,
such
that, ~~ent~cal~~,
pdq
=
ndX
+
dG
,
i.
e.
Since the
RHS
of
the above equation
is
an exact differential, the equality
of
crossed derivatives
a
-
dX
(P~)
=
(Pg
-
.>
?
gives
~hich
can be, equiva~entl~~ expressed by
It
follows
that
Con~ersely,
zf
the transfoTmataon
(3.9)
is
area prese~ng, then
The arbitrariness
of
C
and the continuity
of
the Jacobian determinant imply
-=&l.
!I)
a(n,
x)

A
New
Chamcterization of Completely Canonical lhnsformations
75
If
the Jacobian is
1,
the transformation
(3.9)
isf symplectic. The same is true
if
the Jacobian is
-1;
it
is suficient to interchange the names
of
the variables
7~'s
and
x's
to
go
back to the first case.
3.1.6
Volume
preserving
transformation
An invertible differentiable map from
52''
to itself,
(P,q)
E
!RZn
-
(n,x>
E
%",
(3.10)
will transform
a
given Lebesgue measurable region
S
C
8''
in
a
measurable
region
C
C
R",
S-Z.
The map will be said
a
volume preserving transformation,
or simply, an
equivalent transformation,
if the measures of
S
and
C
coincide:
m,(C)
=
It
will be shown, in the next section, that
a
symplectic transformation on
!R"
is
also equivalent.
It is worth mentioning that the converse
is
true only
in
the case
n
=
2.
mn
(C)
.
3.2
A
New
Characterization
of
Completely Canonical
Tkans-
format ions
The Lie condition
is
not easily handled for checking the canonical nature
of
a
differentiable invertible transformation. Thus, we are looking for conditions
to be directly required to the functions
vh,
$h,
in the invertible differentiable
transformation
Th
=
(Ph(Q/P)
1
Xh
=
$h(q/P)
>
to define a completely canonical transformations.
The condition from which we start
is
the
usual
one, namely
(3.11)
$With the
usual aseumption that the
C
and
S
be linearly simple-connected.
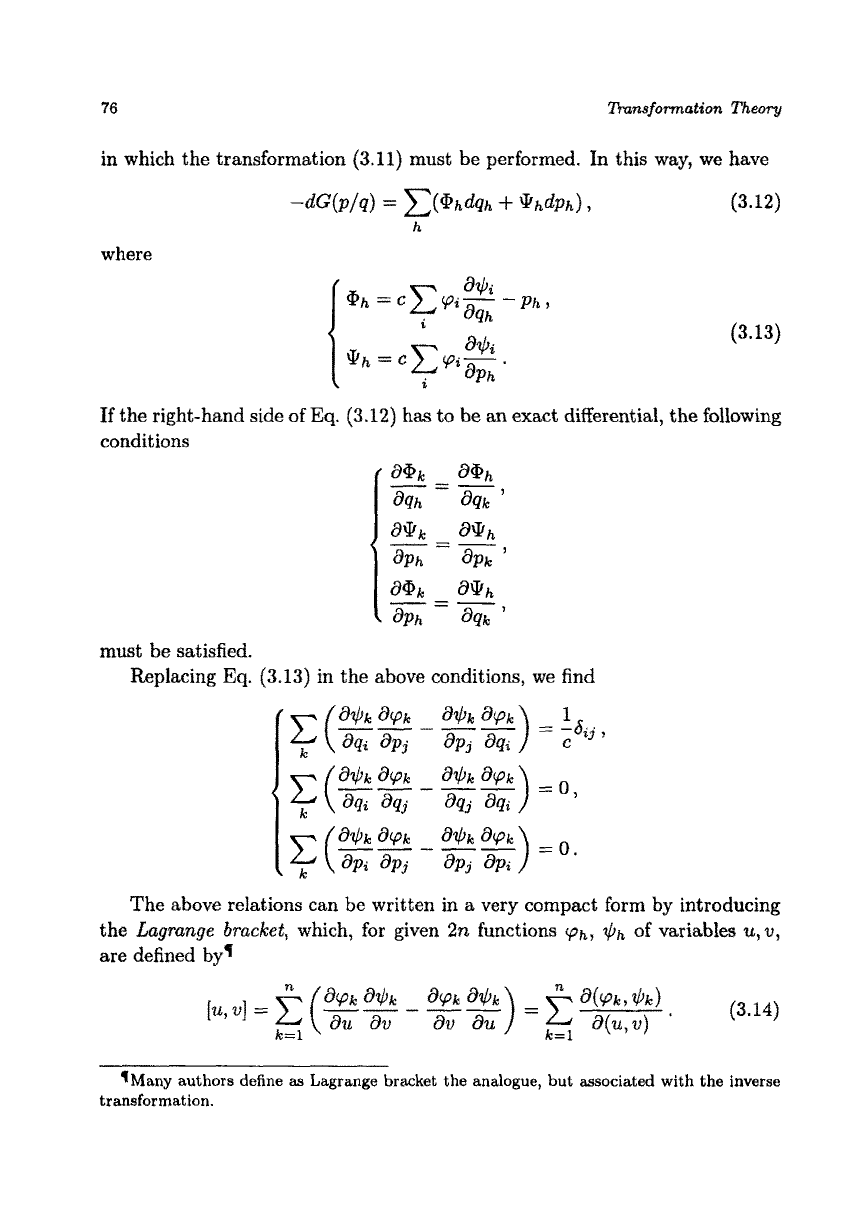
76
~nsfo~~t~on
Theory
in which the transformation
(3.11)
must be performed. In this
way,
we have
-dG(p/q)
=
x(@hd4h
+
@h&h),
(3.12)
h
where
(3.13)
If
the right-hand side
of
Eq,
(3.12)
has
to
be an exact d~ffer~ntia~,
the
fo~~ow~ng
conditions
d@k
a@h
---
-
aqh
&k
'
8$k
a@h
--
--
dph
apk
'
a@k
d@h
--
--
&h
aqk
'
1
=
-saj
,
must be satisfied.
Replacing
Eq.
(3.13)
in the above conditions, we find
'i+
(a$k
-----
aVk
h!'k
a'pk)
=
0,
aqi
&j
aqj
aqi
The above relations can be written in
a
very compact
form
by introducing
the
Lugrange
bracket,
which, for given
2n
functions
Ph, +h
of variables
w,w,
are defined
by3
qMmy authors define
as
Lagrange bracket the analogue, but associated with
the
inverse
transformation.
A
New Characteritation of Completely Canonical hnsfonnations
77
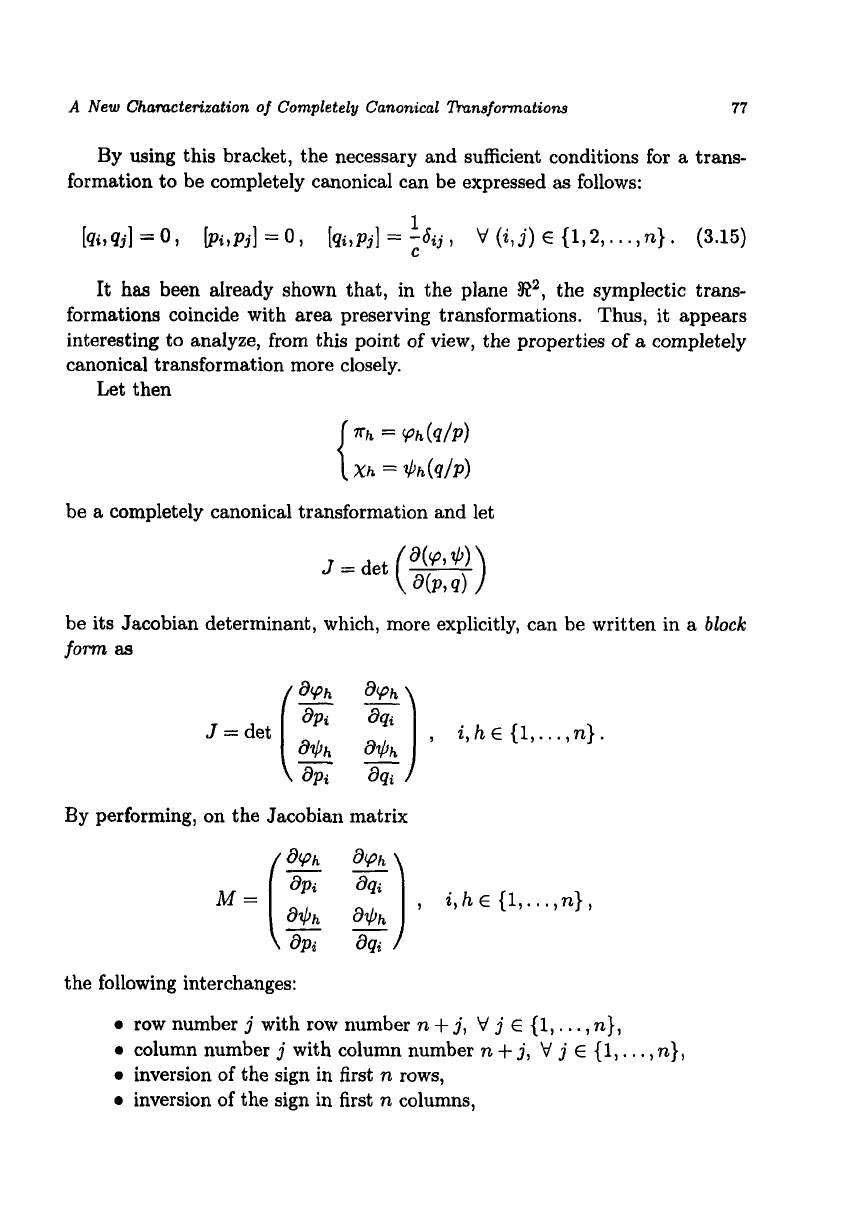
By using this bracket, the necessary and sufficient conditions for a trans-
formation
to
be completely canonical can be expressed
as
follows:
It
has
been
already shown that, in the plane
R2,
the symplectic trans-
formations coincide with area preserving transformations. Thus,
it
appears
interesting
to
analyze, from this point of view, the properties of a completely
canonical transformation more closely.
Let then
rh
=
[Ph
(q/p)
{
Xh
=
‘$‘h(q/p)
be a completely canonical transformation and let
be its Jacobian determinant, which, more explicitly, can be written in a
block
fom
as
By performing, on the Jacobian matrix
the following interchanges:
0
row number
j
with row number
n
+
j,
V
j
E
(1,.
. .
,
n},
0
column number
j
with column number
n
+
j,
V
j
E
(1,.
. .
,
n},
0
inversion of the sign
in
first
n
rows,
0
inversion of the sign
in
first
n
columns,
78
lhnsfonnation
Theory
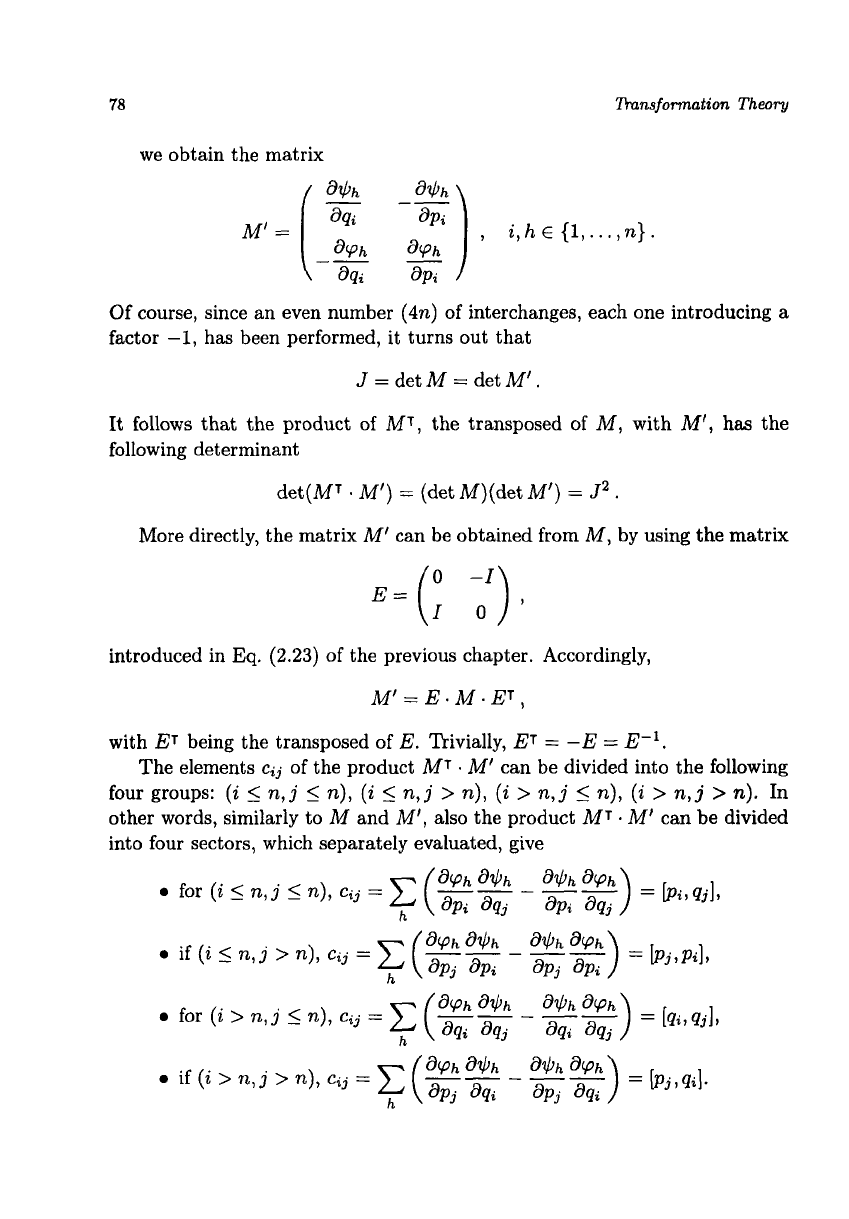
we obtain the matrix
M'
=
,
i,hE{l,
...,
n}.
a'Ph a'Ph
--
-
Of course, since an even number
(4n)
of interchanges, each one introducing
a
factor
-1,
has been performed, it turns out that
J
=
det
M
=
detM'
It
follows that the product
of
MT,
the transposed of
M,
with
M',
has the
following determinant
det(MT
.
MI)
=
(det M)(det MI)
=
J2.
More directly, the matrix
M'
can be obtained from
M,
by using the matrix
introduced in
Eq.
(2.23)
of
the previous chapter. Accordingly,
M'
=
E
M
.
ET
,
with
ET
being the transposed of
E.
Trivially,
ET
=
-E
=
E-l.
The elements cij of the product
MT
-
M'
can be divided into the following
four groups:
(i
5
n,j
5
n),
(i
5
n,j
>
n),
(i
>
n,j
5
n),
(i
>
n,j
>
n).
In
other words, similarly to
M
and
M',
also the product
MT
M'
can be divided
into four sectors, which separately evaluated, give
A
New Chamcterixation
of
Completely Canonical Transformations
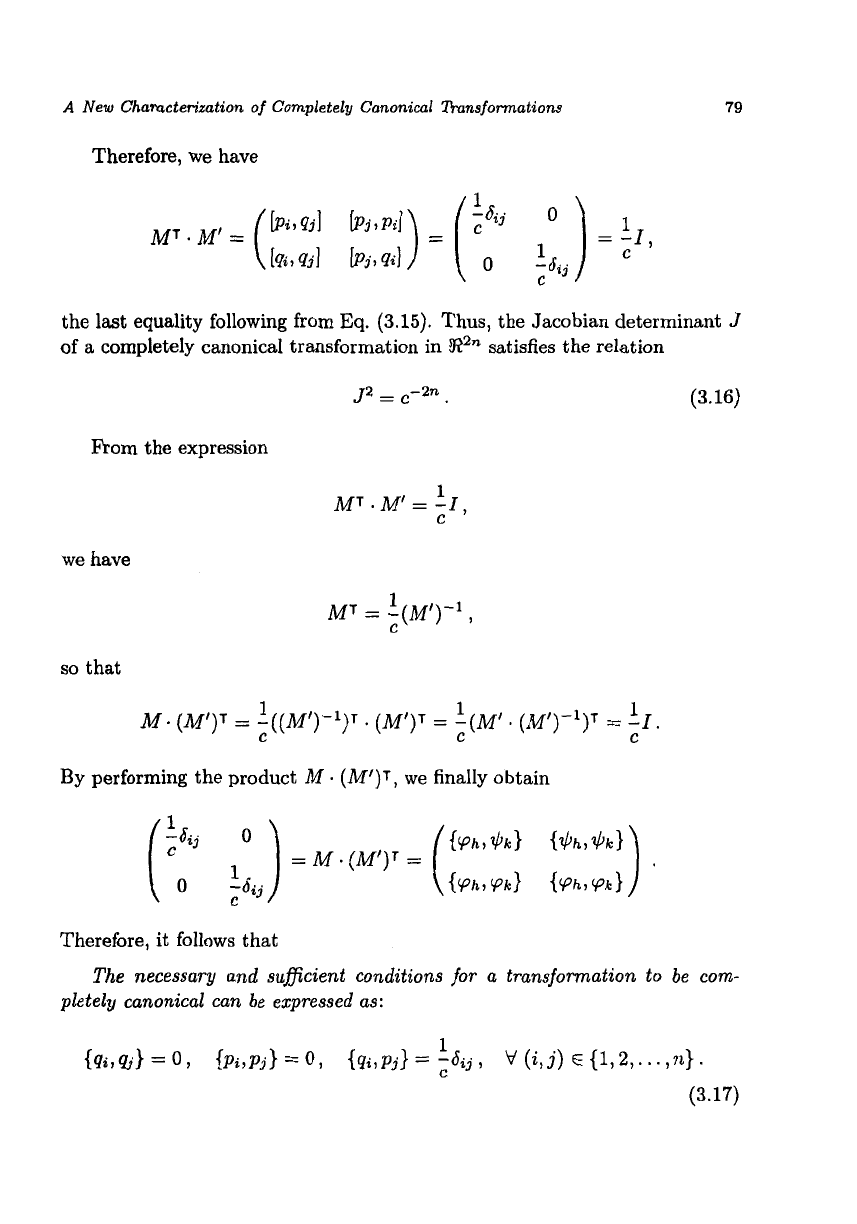
Therefore, we have
79
the last equality following from
Eq.
(3.15).
Thus, the Jacobian determinant
J
of a completely canonical transformation in satisfies the relation
J2
=
c-~~.
(3.16)
From the expression
we have
so
that
1 1
1
M.
(M’)T
=
;((M’)-l)T
.
(M’)T
=
-(MI
.
(M’)-’)T
=
-1.
C
C
By performing the product
M
(M‘)T,
we finally obtain
Therefore, it follows that
pletely canonical can be expressed as:
The necessary and suficient conditions for a transformation to
be
com-
1
{qt,qj}=Oi {pi,pj}=O, {qijpj}=;6ij>
V(iij)c{1121**.1n}.
(3.17)

80
lhnsfomation
Theory
3.3
New Characterization
of
Symplectic Transformations
From
relations
(3.15),
it turns out that
The necessary and suficient conditions for a transformation to be symplec-
tic
is
given
by
[Qi,
Qjl
=
0,
[Pi,Pjl
=
0
1
[Qi,Pjl
=
6ij
,
v
(i,j)
E
{1,2,.
.
.
,n}
,
{Pi,
~j}
=
0
7
{~i,
~j}
=
6ij
,
or
{
qi,
qj}
=
0
V
(ii
j)
E
{
1
,
2,
.
*
,
n}
*
Moreover,
from
Eq.
(3.16),
it follows that
A
symplectic transformation preserves the volume of any given region
of
the phase space.
Chapter
4
The Integration Methods
4.1
Integrals Invariants
of
a Differential System
Let
us
consider the first order differential system
dXi
dt
-
=
X"Z/t),
vi
E
(1,2,.
.
.n),
and denote with
(4.1)
xi
=
zyt,
20)
the solution, which
at
time
to,
takes the value
xo
:
X;
=
si(to,
20).
The
above relations can be equivalently expressed by
Q
=
Q(t,
Qo)
3
(4.2)
where
Q
and
Qo
denote the points whose coordinates are
(d,
.
.
.
,
zn)
and
(xi,.
.
.
,
eg),
respectively. Given any submanifold
UO
C
Rn,
whose points will
be denoted
by
Qo,
let
U
be the submanifold, depending on
t,
of
points
Q
given
by
Eq.
(4.2).
In other words,
U
represents the evolution at time
t,
according
to
Eg.
(4.1),
of
270.
Equation
(4.2)
thus define a map between
Uo
and
U.
The map
is
one-to-
one by
the
existence and uniqueness theorem, which is taken to hold for the
system
(4.1).
81

82
The Integration Methods
Let us consider an arbitrary function
p
of
x
and
t
and the integral
which, of course, will generally depend on time
t.
In the case
I
does not depend
on time, no matter how
U
is chosen, we shall say that
I
is an integral invariant
for the system (4.1).
In order for
I
to be an integral invariant,
p
is required to satisfy suitable
conditions.
Let
us
start from the natural characterization of an integral invari-
ant, which is given by
In the above expression, the transfer
of
time derivative under the integral sign
is
not permitted, since the integration region depends on time. The difficulty
is easily overcome by using the change of variables given by
Eq.
(4.2).
In this
way, we obtain
where
ij
=
PO&
is
the composed function between
p
and
Eq.
(4.2);
i.e.
~(zo,
t)
=
p(x(zo,
t), t)
and
J
is the Jacobian determinant
(4.4)
of the transformation
Eq.
(4.2).
Remark
7
The theorem on the change
of
variables
in
the integrals
requires, really, the absolute value
of
the determinant. In our case, however,
the Jacobian matrix at initial time
to
coincides with the unit matrixZ. Then,
by the continuity, it exasts a neighborhood
of
to
(an interval
of
time)
an
which
the Jacobian determinant is always positive.
It follows that
where we have used the property that the Jacobian
of
the inverse transforma-
tion is the inverse of the Jacobian.
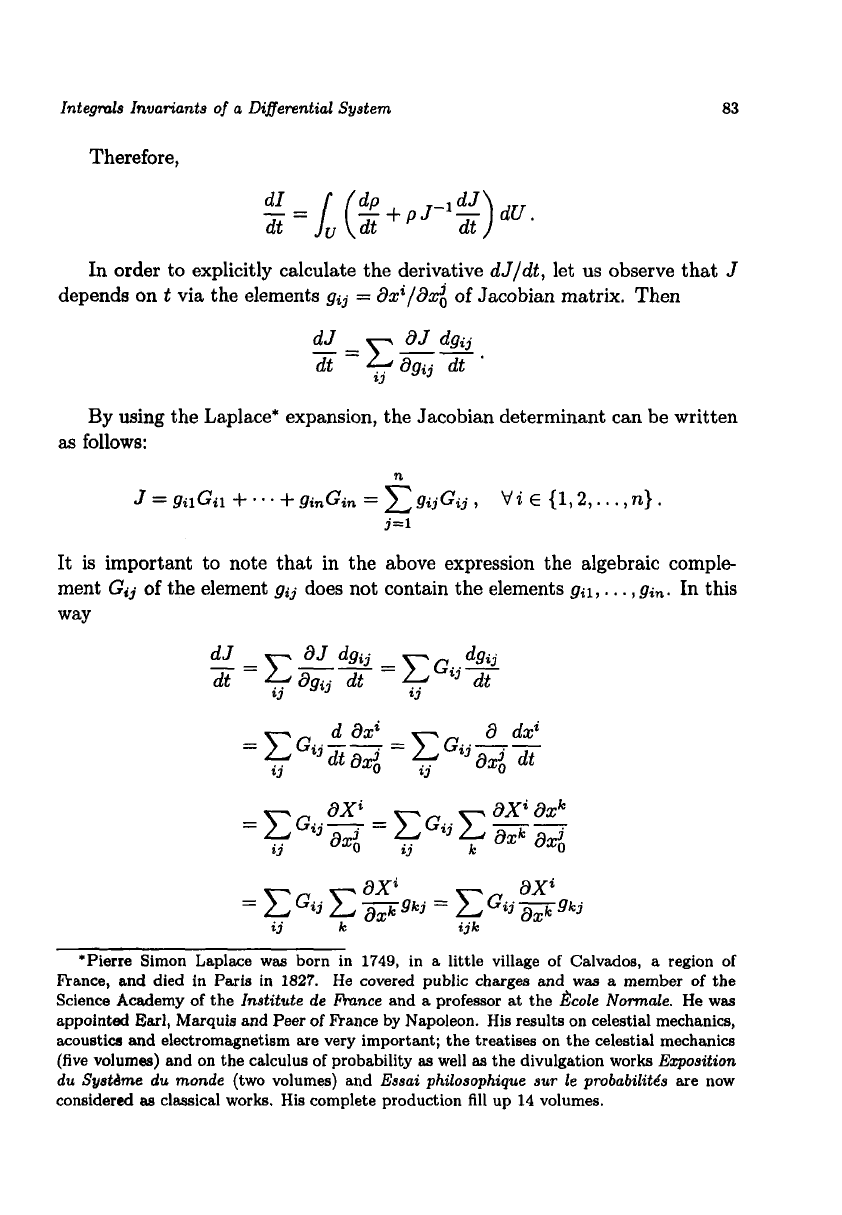
Integmls Invariants
of
a Diflerential System
Therefore,
=
(2
+pJ-l-)
dJ
dU.
dt dt
In
order to explicitly calculate the derivative
dJ/dt,
let
us
observe that
depends on
t
via the elements
gij
=
axi/&$
of
Jacobian matrix. Then
dJ
_-
83
J
By
wing
the Laplace* expansion, the Jacobian determinant can be written
as
follows:
It is important to note that in the above expression the algebraic comple-
ment
Gij
of
the element
gij
does not contain the elements
gil,.
.
.
,gin.
In this
way
dJ
w
=CGij-
dXi
=CGijC--
axi
axk
axk
ax$
ij
ax;
jj
k
axi
aXi
=
EGij aSI,gkj
=
XGijGgkj
'Pierre Simon Laplace
was
born in
1749,
in
a
little village
of
Calvados,
a
region
of
fiance, and died
in
Paris in
1827.
He covered public chargea and was
a
member
of
the
Science Academy
of
the
Institute de hnce
and
a
professor
at
the
kcole Normale.
He was
appointed
Earl,
Marquis and Peer
of
France by Napoleon.
His
results
on
celestial mechanics,
acoustics
and electromagnetism
are
very important; the treatises
on
the celestial mechanics
(five volumes) and on the calculus
of
probability
as
well
as
the divulgation works
Exposition
du
Systdme
du monde
(two volumes) and
Essai philosophique sur le probabilitb
are now
considered
aa
classical works. His complete production fill up
14
volumes.
ij
k
ijk
