Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.

54
Hami~ton~an
Systems
we can write
Therefore
,
-
+
{f,31}
=
0
*-
a{f1g}
+
{{f,g},?i}
=
0.
[:
-
+
(g,%}
=
0
at
at
Of
course, the Jacobi-Poisson theorem will not always give new first inte-
grals, since their number is bounded to be
2n
-
1,
where
n
is
the number
of
degrees
of
freedom. In some
cases,
in fact, the Poisson bracket of first integrals
simply reduces to
a
function of them
or
to a numeric constant.
Two
functions,
f
and
g,
with vanishing
Poisson
bracket
{
f,
g}
=
0,
are said to be in
involution.
Problems
1.
Show that, if one of the functions
f
and
g
coincides with
a
momentum
or
a
coordinate, the Poisson bracket simpIy reduces to
a
partial deriva-
tive
As
a particular case, notice that
where
6hk
is
the
Kronecker delta.
mentum $and the angular momentum
I"=
?A
$of
a
particle.
2.
Evaluate the Poisson bracket of the Cartesian components
of
the mo-
Solution
The
Poasson
Bracket and the Jacobi-Poisson
Theorem
so
that
55
The remaining brackets follow from a cyclic permutation
of
the indices
5,
y,
z.
Finally, denoting with
(p1,p2,p3)
and
(Z1,12,/3)
the components of
p'
and
17
respectively, we write
3
{li,Pj}
=
Ceijh~h
,
vi,j
E
{1,2,3},
h=l
where
Eijh
is
the
Levi-Civitav tensor densaty
defined by
if
i,
j,
h
is
an even permutation of
1,2,3
if
i,j,
h
is an odd permutation
of
1,2,3
in the other cases; i.e.,
if
two indices coincide.
3.
Show that
4.
Evaluate the Poisson bracket of the components of the angular momen-
tum of a particle between them by using only the algebraic properties
(2.19).
Solution
TTuIlio
Levi-Civita was born in Padova in
1873
and died in Rome in
1941.
He obtained
his degree
at
Padova University in
1894.
He was
a
professor of mathematical physics, at the
age of
24
years,
at
Padova University, where he taught until
1919.
In this same year he moved
to Flome University. In
1938,
withthe introduction
of
racial fascist laws, he was removed
from the chair and his now classical books, including the
Lezioni
di
Meccanica Razionale
(1929)
and
Lezioni
di
Calcolo Differenziale
Assoluto
(l923),
was interdicted. Fortunately,
thanks
to
Whittaker, the last had been translated in English and published
on
1926
by Blakie
&
Son.
He
died
just
in
time
to
avoid
to
be forced also
to
hide because
of
racial persecutions.
56
Hamiltmian
Systems
Since the momenta and the coordinates of different particles are independent
quantities, it is easy
to
verify that the resulting formulae of the previous prob-
lems
still hoid for the total momentum
9
and for the total angular momentum
L'
of
an arbitrary system of particles.
5.
Prove that for any scalar11 function
p
of the coordinates and momenta
of a particle, the following relation holds:
Solution
A
scalar function depends on the vectors
r'
and p'only by means
of
the combi-
nations
r2
=
r'.
r',
p2
=
3.
@
and
r'.
p.
Therefore,
we
can write
acp
p',
--
ap
-
-2g+
-
acp
ar'
ar2
a(F.p)
The relation
(I,=,
p}
=
0,
then follows by applying the definition
of
Poisson
bracket
(2.16).
The same relations hold for the remaining compon~nts
of
(
so
that, for any scalar function
cp
of the coordinates and momenta of a particle,
we can write
{L,
cp)
=
Uy,
'PI
=
{L
PI
=
0
*
6.
Prove that for
any
vector
function
fof
coordinates and momenta of a
particle, the following relation holds:
(Iz,
f)
=
n'
A
$,
where
ii
is the unit vector
along
:he
z
axis.
Analogous formuIae hold
for the remaining components
of
t.
Solution
Any vector function fof ?and @can be written in the form
f=
cplr'+
'pzp'+
cps(r'A
$,
where
cp1,
p2,
cp3
are scalar functions. Thus, by using the algebraic
properties of the Poisson bracket, we finally obtain the soiution to the problem.
IIHere, scalar and vector functions
are
understood with respect to the rotation
group
SO(3).
A
More
Compact
Form
of
the Hamiltonian Dynamics
57
2.4
A
More
Compact
Form
of
the
~~ilto~~
Dynamics
Let
us
start by considering
a
Hamiltonian system with
n
=
2
degrees of free-
dom.
By ~troducing the cofumn vectors
and the skew-symmetric matrix
.=(;
-;),
Hamilton's equations can be written in the form
or
in
components,
as
follows:
(2.21)
(2.22)
where the
sum
over the index
k
is understood. The Poisson bracket can then
be written
as
follows:
where the bracket
(a,
.)
denotes the Euclidean scalar product.
with
n
degrees
of
freedom. In this case, the matrix
E:
is
given by
Of
course, the previous nota~ion can be also used for
a
H~i~tonian system
(2.23)
where
0
and
I
denote the
n
x
n nu1 and identity matrices.
2.4.1
Geneva1 ~arn~l~o~~an
~~nurn~~s
Let
us
now consider a generic dynamics described by an equation similar to
Eq.
(2.22):
(2.24)
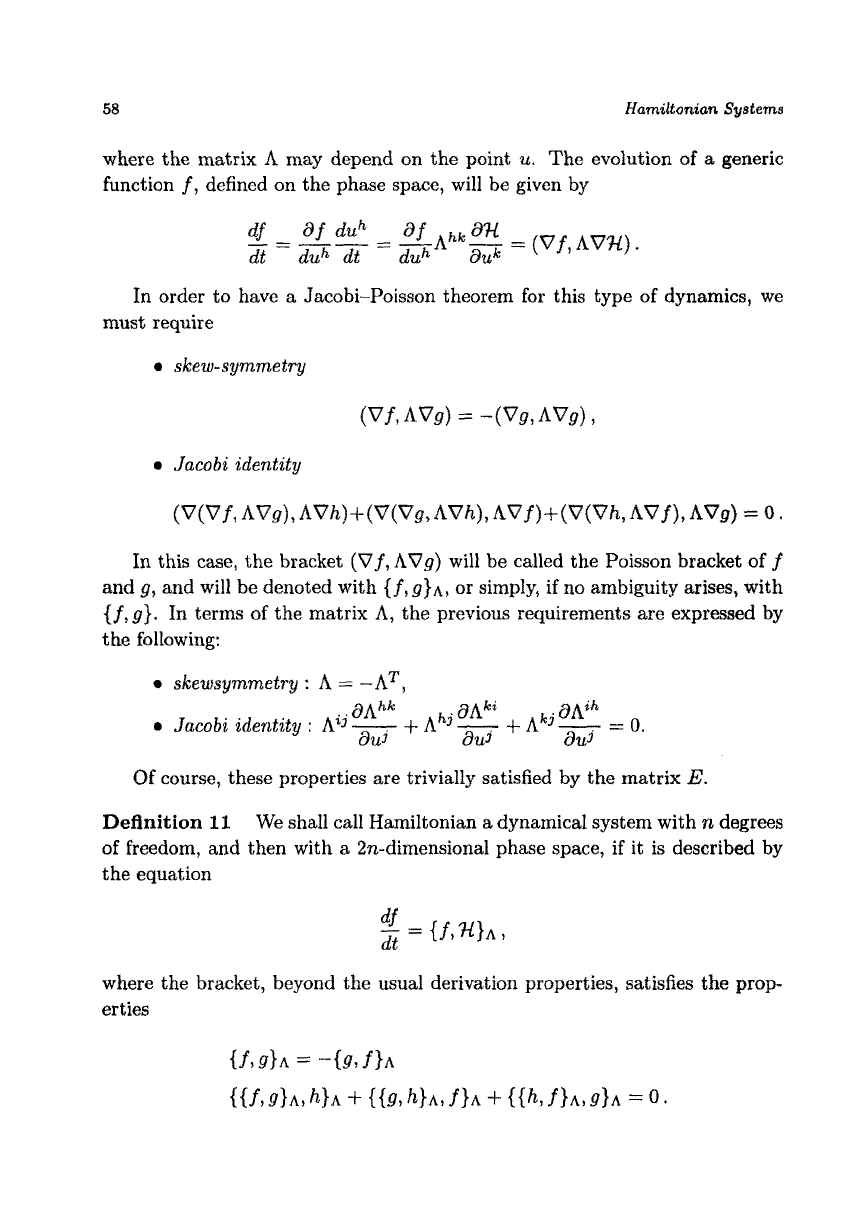
58
Hamiltonaan
Systems
where the matrix
A
may depend on the point
u.
The evolution of
a
generic
function
f,
defined on the phase space, will be given by
In order to have
a
Jacobi-Poisson theorem for this type of dynamics, we
must
require
0
slcew-symmetry
e
Jacobi
identity
(V(Gf,
AVgf,
AV~~+(V(Vg,
AVh),
AVf)+(V(Vh, AVf),
AVg)
=
0.
In this case, the bracket
(V
f,
AVg)
will be called the Poisson bracket of
f
and
9,
and will be denoted with
{
f,
g}A,
or simply, if no ambiguity arises, with
{
f,g}.
IR
terms of the matrix
A,
the previous requirements are expressed by
the following:
0
ske~sym~etry
:
A
=
-AT,
Of
course, these properties are trivially satisfied by the matrix
E.
Definition
11.
We shall call Hamiltonian
a
dynamical system with
n
degrees
of
freedom, and then with
a
2n-dimensional phase space, if it is described by
the equation
where the bracket, beyond the usual derivation properties, satisfies the prop
erties

A
More
Compact
Form
of
the Hamiltonian Dynamics
59
2.4.2
Jacobi-Poisson dynamics
Let
us
finally observe that
in
the previous definition, no role
is
played by the
even dimensionality of the phase space. Thus, it is natural to define more
general dynamics according to the following definition.
Deflnition
12
A
dynamics, described by the equations
df
-
dt
=
{f,'tC)P
,
with the bracket satisfying the properties
is
called a Jacobi-Poisson dynamics.
Of
course, a Hamiltonian dynamics is also a Jacobi-Poisson dynamics.
2.4.3
We notice that properties expressed by
Eqs.
(2.17)
and
(2.18)
endow the set
7,
of differentiable functions defined on
@,
with a
Lie algebra structure.
Remark
6
A
Lie algebra
A
is a vector space endowed with an internal com-
position
law,
denoted by
[.,
a]
and called a Lie bracket, satisfying the properties:
More
on
the Poisson bracket
[X,Y]
=
-[Y,X], VX,Y
E
A,
[X,
[Y,
211
+
[Y,
[z,
XI]
+
12,
[X,
Y]]
=
0,
VX,
Y,
E
A.
Examples
of
Lie algebras are
0
the set
of
vectors
in
!R3
endowed with the Lie bracket
[a,
.]
given by the
vector product

60
Ham~~t~n~an Systems
0
the vector space
of
rt
x
n
~~~Tices ~ndo~e~
~itfi
the Lie bracket
f.,
+]
given
by
the commutator
[M,NJ
MN
-
NM.
A
geometrical definition
of
Lie algebra
will
be given
in
Part
II.
Since it can be written in the following equivalent alternative forms:
Xi,,g}h
=
[XfiXgIh,
(2.25)
Xf{S,
h)
=
{XfS,
h)
+
(91
Xfh)
?
(2.26)
the Jacobi identity is equivalent to the following alternative state~ents sug-
gested by
Eqs,
(2.25)
and
(2.26),
respectively:
0
The map
f
-
Xf
=
u1.1
{f,9)
+-+
X{fd
(3,
(‘1
.I)
r--)
(XFl
I-,
-1)
is
a
Lie
algebra morphism
between
(3,
{ml
-})
and the
set
of
~ami~tonian vector fields
XF
endowed
with the Lie bracket given by the commutator
I-,
.I,
*
The operator
Xf
=
{
f,
.}
is
a
derivation
of
the Poisson bracket.
Last
statement,
as
it
will be shown in the next subsection, suggests the in-
troduction of more general structures named
n-Poisson brackets.
For instance,
a
3-Poisson bracket on
3
is
bracket
{a,
s,
.}
satisfying the following properties:
{fl
Sl
h)
=
-{f,
h,
9)
=
-b,
f,
h)
1
{f191
{u,
v,w}l+
{v,
w,
if,
s,
{f,
9,
hihz)
=
{f,
g1
hi)hz
i-
hi{
f
,
g1
hz)
{f,g,c}
=
0
-
(B,
w,
{f,
9,
v)}
+
{%V,
{f,
9,
w))
=
0
I
vc
E
R.
An
algebraic fomulation
We observe that properties expressed
by
Eqs.
(2.17), (2.18)
and
(2.19)
are
purely algebraic in nature,
so
that the following abstract formulation can be
introduced.

A
Mom
Compact
Form
of
the
Hamiltonian
Dynamics
61
Let
M
be
a
Poisson manifold and
F
the ring of functions defined on it.
This means that on
M
a bracket
{-,
-}
is defined such that
(1)
it
yields the structure of
a
Lie algebra on
T;
i.e.
{fld
=
--{91fL
{f,
(9,
hH
f
(91
{hl
f
1)
f
{h,
~f1gIl
=
0
1
(2)
it has
a
natural compatibility with the usual associative product
of
functions, which is
Therefore, we can define
an
abstract
Poisson aZgebra
as
an associative com-
mutative algebra endowed with
a
Lie bracket satisfying
Eqs.
(2.17), (2.18)
and
(2.19).
It
is
natural to generalize the notion of a Poisson manifold by relaxing
condition
(2)
and requiring only that
{f,g}
be just a
local type
operation:
support
{f,
g)
C
(support
f)
n
(support
9).
The bracket
{f,g}
is
then called a
Jacobi bracket
and the corresponding ma-
nifold
a
Jacobi manifold.
2.4.4
The possibility
of
further generaiizations of Jacobi-Poisson dynamics rely on
the possibility to generalize the Poisson bracket.
firther generalizations
of
the Jacobd-Poisson
dgnamico
Let
us
consider a dynamical system described by the equations
d
dt
-f
=
{flxl32}
t
where the
ternary bracket
in the right-hand side is supposed to be skewsym-
metric. This dynamics will be called
a
ternary Jawbi-Poisson dynamics
if the ternary bracket allows
for
a Jacobi-Poisson theorem on first integrals.
In such
a
case the ternary bracket will be called
ternary Jacobi-Poisson
bracket.
We are thus looking for a property
of
the ternary bracket such
that

62
Ha~~lton~a~
Systems
For this purpose it
is
useful to recall the form of Jacobi identity, for
nary
bracket,
given in Eq.
(2.26):
Xfb,
h)
=
{xfg,
h)
+
{!7>
Xfh).
This form can be immediately generalized to skewsymmetric brackets with an
arbitrary number of entries.
Indeed, given the ternary bracket
{
f,g,
h},
we require that the operator
Xf,
(vector field), defined by
Xfgh
:=
{f,
Q,
h)
f
be
a
derivation of the bracket; that
is
Xfg{hl,h2,h3)
=
{Xf&l,h2,h3)
$-
(hl,Xfgh2,h3}
f
(hl,h2,Xfgh3}-
(2.28)
The above formula can be explicitly written
as
follows:
(f,
9,
{hl,
h2, h3))
=
{
{f,
hl), h2, h3)
+
{hl,
{f
,
9,
h2),
h3)
+
{hl,
h2,
if,%
h3))
I
which would be difficult to invent without
a
deep understanding of the signif-
icance of the usual Jacobi identity.
It is not diEcult to prove that Eq.
(2.27)
is
equivalent to Eq.
(2.28).
We
will
not go on further on this subject. Much more details can be found in
Ref.
152
and references therein, where examples of n-ary Jacobi-Poisson dynamics
are
explicitly given, and the following important property, here reported just for
the case
n
=
3,
is proven:
If
{
f,
g,
h}
is
a
ternary Jacobi-Poisson bracket, the binary bracket
{
f,
g}h
=
{
f,
g,
h},
obtained by fixing one
of
the functions, is
a
binary Jacobi-Poisson
bracket. hrthermore,
a
linear combination
of
two
of
them cl{f,g}hl
+
cz{
f,
g)ha
is
agaan a bznar~ Jacob~-~~isson bracke~.
2.5
The
Variational
Principle
for
the Hamilton Equations
It
has
been shown that Lagrange’s equations
(2.1)
are differential equations
for the unknown functions
qh(t),
which are required to be an extremwn of the
action

The
Variational
Principle
for
the
Hamilton
Equations
63
S[qI
=
/””
G?/Q/t)dt.
ta
On the other hand, from Eq.
(2.6),
we have
(L)*dt
=
xphdqh
-
‘fldt
,
where
C,
is the Lagrangian
L
in which the velocities
qh
have been expressed
in terms
of
momenta and coordinates
by
means
of
Eq.
(2.3).
It
is
then natural to argue that Hamilton’s equations can be obtained
as
the equations
for
the extrema
of
h
S*[q/p/tI
=
fb
L*(q/p/t)dt,
ta
the transformed functional
of
S(qJ:
This
is
easily verified, since
As
before, by imposing
6qh(tA)
=
dqh(tB)
=
0,
we obtain
In this
way,
Hamilton’s equations
of
the motion follow from the vanishing
of
6s’
for
any choice
of
6Ph’S
and
6Qh’S.
