Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.

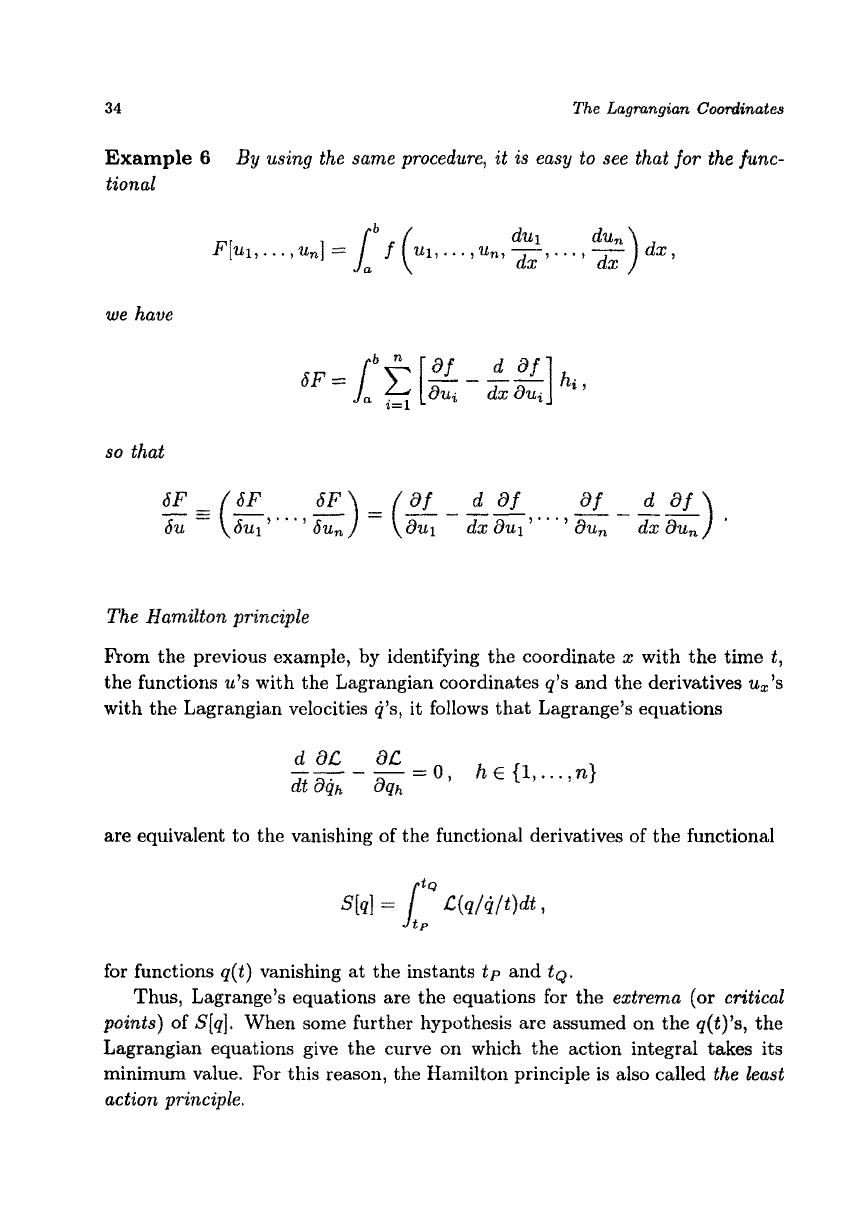
34
The
Lagrangian
Coordinates
Example
6
tional
By using the same procedure,
it
is easy
to
see that
for
the
func-
we
have
so
that
The Hami~ton pri~ciple
From the previous example,
by
identifying the coordinate
x
with the time
t,
the functions
u’s
with the Lagrangian coordinates
q’s
and the derivatives
us’s
with the Lagrangian velocities
q’s,
it
follows that Lagrange’s equations
are equivalent to the vanishing of the functional derivatives of the functional
for functions
q(t)
vanishing at the instants
tp
and
tQ.
Thus, Lagrange’s equations are the equations for the
extrema
(or
critical
points)
of
S[q].
When some further hypothesis are assumed
on
the
qft)’s,
the
Lagrangian equations give the curve on which the action integral takes its
minimum value.
For
this reason, the Hamilton principle is also called
the least
action principle.
Remorki
on
Lagrange's
Equations
35
1.5
Remarks
on
Lagrange's
Equations
1.5.1
Eq~i~alent
~a~ang~an~
Looking
at
the action integral, it turns evident that the Lagrangian equations
corresponding to Lagrangian functions
C(q/&'t)
and
C'(q/g/~),
which differ by
a
term given by the derivative of a function depending only
by
the coordinates
q
and the time
t,
d
C'(4/4/t)
=
C(q/d/t)
+
ZfW)
coincide. Indeed, the two action integrals,
differ by
a
constant term
S'M
-
SbI
=
f(Q/tg)
-
f(P/tp)
t
6S"q]
=
6S[q].
which does not contribute to the variation
Two such Lagrangians are called
equavatent.
This
is
not, of course, the most general case and there exist examples of
Lagrangian dynamics admitting more than one, not equivalent, Lagrangian.
An exhaustive treatment can be found in Ref.
157.
1.5.2
Dgnamical
similitzl.de
Let
us
start by observing that two Lagrangian functions differing by a constant
factor give the same Lagrangian equations. Thanks
to
this circumstance,
it
is
possible to infer some properties of the motion without integrating the cor-
responding equations. This happens, for instance, for simple systems with
kinetic energy
7
=
$mu2
=
$m
Ch
qi
and a potential energy
U(q),
which
is
a
homogeneous function
of
the coordinates; i.e.
a
function satisfying the
condition
WXQ)
=
XaU(q)
9
where
X
is an arbitrary constant and
a
is
the homogeneity degree of
U.
36
By performing the transformation
qh
(ri
=
Xqh,
t
3
if
=
the velocities
4h
will be multiplied by the factor
X/p
0
the kinetic energy
7
will be multiplied by the factor
(X/p)'
the potential energy
U
will be multiplied by the factor
A"
u
3
X"U;
the Lagrangian function
C
will change accoTding
to
As
a consequence, the Lagrangian function
will
be multiplied by
a
constant
factor
A*
only
if
p
=
A"?,
To
change the coordinates by
a
constant factor
X
means to pass from some
trajectories to other ones geometrically similar to the first, the only difference
lying in the linear dimensions (homothety).
We
finally arrive to the following conclusion:
If
the potential energy
of
a sample system is a homogeneous function
of
coordi~ates, the equataons
of
the motion a~m~~ geome~r~call~ ~~mi~ar trajec-
tories
for
which the time interval
t'
and
t,
between corresponding points on
trajectories, have the ratio
1-4
"=(%>
t
,
(1.35)
Example
7
For
small
oscillations, the potential eriergy
is
a quadratic fmc-
tion
of
coordinates
(a
=
2).
Equation
(1.35)
shows that the period
P
does
not
~epen~ on their amplitu~es ~~alilei~s ~bse~a~io~ on the can~eZab~m at
Duomo
in
Fisa).

Remarks
OR
Lagmnge’s
Equations
37
Example
8
In a homogeneous field, the potential energy
is
a linear function
of coordinates
(a
=
1).
Equation
(1.35)
shows that for a free falling body
in
the gravity field, the squares of time
of
falling are
in
the ratio of their initial
heights.
Example
9
In the case
of
the Newtonian attraction between two masses,
or
the Coulomb attraction of two charges, the potential energy
is
in
the inverse
proportion with their distance
(a
=
-1).
Equation
(1.35)
shows that the squares
of the revolution periods of planets,
in
their orbits, are proportional to the third
power
of
their linear dimensions (Kepler’s third law).
The previous analysis can also be carried out, of course, by means of New-
ton’s equations. The reader is invited to do it by himself.
1.5.3
Electrical circuit analysis
The circuital relations, for
a
network of coupled reactive impedances in which
a
system of electrical currents
ih
is flowing, generated by electromotive forces
vh,
are
where
Lhk
=
Lkh
are the mutual inductances
(h
#
k)
and self-inductances
(h
=
k),
ch
the capacitances,
Rh
the resistive impedances and
Fh
=
dVh/dt.
They are the Lagrange equations associated with the Lagrangian fun~tion,~~~~

Chapter
2
Harniltonian Systems
Lagrange’s equations constitute
a
system of
n
second order differential
equations in the unknown curves
qh
=
Qh(t).
By writing
d2L
dk
-t-
-
qk+xp
d
8C
d2C
a2L:
dt
a&
-
k=l
%h%k
%hat
’
k=
1
they can be explicitly written in the form
where the force
Fh
is
defined by
Thus, if the Lagrangian function is regular; that
is,
the
Hessian determinant
3
of the matrix
is not vanishing, Lagrange’s equations can be written in the following
normal
form:
39
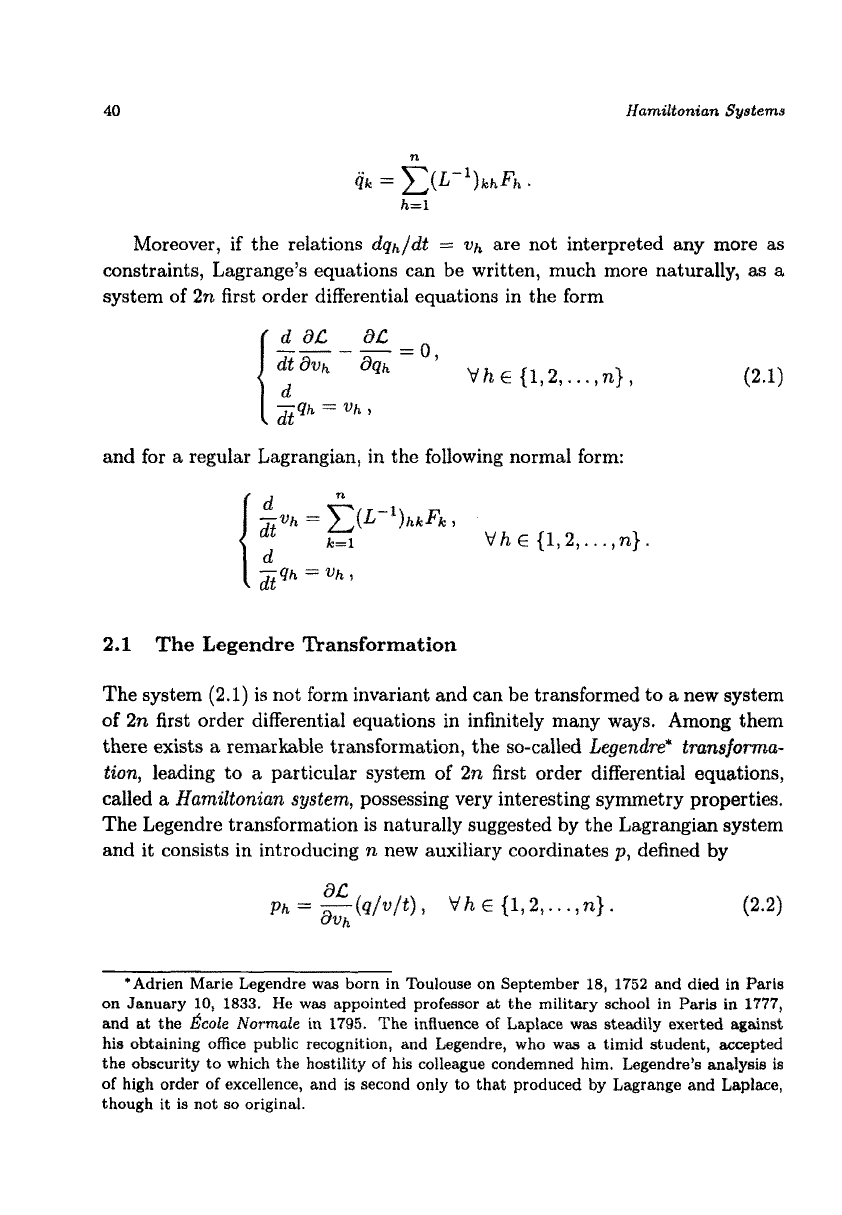
40
n
h=l
Hamiltonian Systems
Moreover,
if
the relations
dqh/dt
=
vh
are not interpreted any more
as
constraints, Lagrange's equations can be written, much more naturally, as
a
system of
2n
first order differential equations in the form
d
bC
aL
--...---
VhE
{1,2
,...,
n},
(2.1)
dtbvh
aqh
-O'
i.
dtqh
=
vh
>
and for
a
regular Lagrangian, in the following normal form:
2.1
The
Legendre Transformation
The system
(2.1)
is not
form
invariant and can be transformed to
a
new system
of
2n
first order differential equations
in
infinitely many ways. Among them
there exists
a
remarkable
tr~sformation, the so-called
Legendre* truns~o~u-
tion,
leading to
a
particular system
of
2n
first order differential equations,
called
a
Hamiltonian system,
possessing very interesting symmetry properties.
The Legendre transformation is naturally suggested by the Lagrangian system
and it consists in introducing
n
new auxiliary coordinates
p,
defined by
ar:
ph
=
-(q/~/t)l
Vhe
{1,2
l...,n},
avh
'Adrien Marie Legendre
waa
born in Toulouse on September
18,
752
and
died
in
Paris
on January
10,
1833.
He
was
appointed professor at the military school in Paris
in
1777,
and
at
the
&Cole Normale
in
1795.
The inhence
of
Laplace
was
steadily exerted against
his obtaining office public recognition, and Legendre, who
was
a
timid student, accepted
the obscurity
to
which the hostility
of
his colleague condemned him. Legendre's
analysis
is
of
high order
of
excellence, and
is
second only
to
that
produced by Lagrange and Laplace,
though it
is
not
so
original.

The
Hamilton
Equations
41
The
p’s
are called
conjugate variables
of
q’s,
or for their dynamical interpre-
tation in some typical cases,
conjugate
momenta
of
4’s.
The above system, considered
as
an algebraic system of equations, can
be solved, for
a
regular Lagrangian, with respect to the unknown
vh
in the
following form:
Vh
=
%(q/p/t)
-
(2.3)
By
using Eqs.
(2.2)
and
(2.3),
the Lagrangian equations
(2.1)
become
where the symbol
*
indicates that the velocities
Vh
have been expressed, by
means of Eq.
(2.3),
in terms of Lagrangian coordinates
q,
their conjugate mo-
menta
p
and
time
t.
Thus,
we obtain the
nonnal
system of
2n
first order differential equations
with the
2n
unknown functions
q’s
and
p’s,
Of course, the above system is equivalent to the original Lagrangian system
(2.1),
since from one side, it follows from Eq.
(2.1)
by means of the described
procedure
(J
#
0),
and vice versa, it is possible
to
go back to Eq.
(2.1),
replacing the momenta
p
in
Eq.
(2.5)
with their expression given by Eq.
(2.2).
2.2
The
Hamilton Equations
2.2.1
It is remarkable that the
RHS
of Eqs.
(2.5)
can be expressed in terms of
a
unique function of
q’s,
p’s
and
t,
called Hamilton function or characteristic
function
or
simply Hamiltonian.
In
this way, the first order system will appear
as
simple as the original
Lagrangian system
(2.1),
depending on the unique function
C.
The mentioned
From Lagrange to Hamilton equations
42
Hamiltonican
Systems
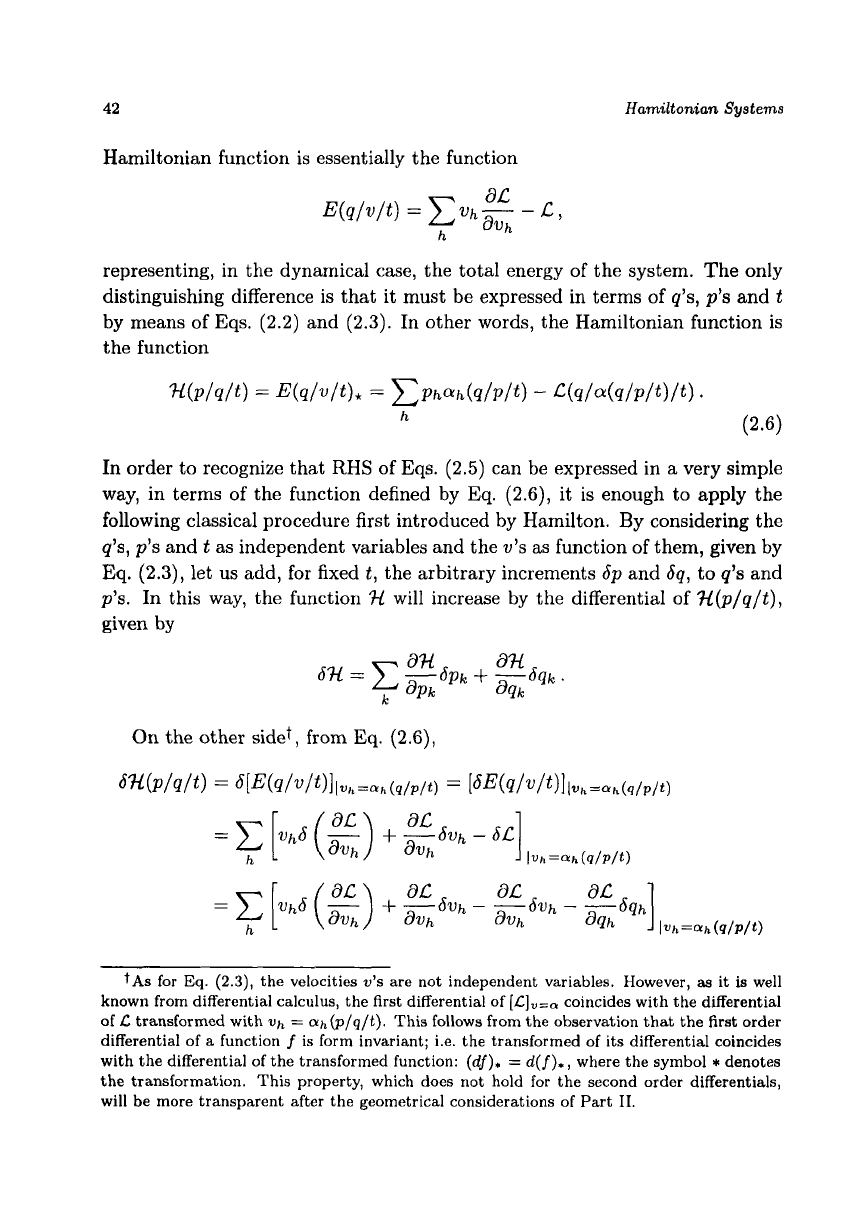
Hamiltonian function is essentially the function
representing, in the dynamical case, the total energy of the system. The only
distinguishing difference is that it must be expressed in terms of
q’s,
p’s
and
t
by means of
Eqs.
(2.2)
and
(2.3).
In other words, the Hamiltonian function is
the function
Wp/q/t)
=
E(q/v/t)*
=
CPhW&/P/t)
-
C(q/4q/p/t)/t)
*
(2.6)
h
In order to recognize that
RHS
of
Eqs.
(2.5)
can be expressed in
a
very simple
way, in terms of the function defined by
Eq.
(2.6),
it is enough to apply the
following classical procedure first introduced by Hamilton. By considering the
q’s,
p’s
and
t
as independent variables and the
ZI’S
as function of them, given by
Eq.
(2.3),
let
us
add, for fixed
t,
the arbitrary increments
dp
and
6q,
to
q’s
and
p’s.
In this
way,
the function
3t
will increase by the differential of
Ifl(p/q/t),
given
by
On the other side+, from
Eq.
(2.6),
tAs
for Eq.
(2.3),
the velocities v’s are not independent variables. However,
as
it is well
known from differential calculus, the first differential of
[L],=,
coincides with the differential
of
L
transformed with
Vh
=
ah(p/q/t).
This
follows
from
the observation that the
first
order
differential of
a
function
f
is form invariant; i.e. the transformed
of
its differential coincides
with the differential of the transformed function:
(df),
=
d(f),
,
where the symbol
*
denotes
the transformation. This property, which does not hold for the second order differentials,
will be more transparent after the geometrical considerations of Part
11.
The
Hamilton
Equations
43
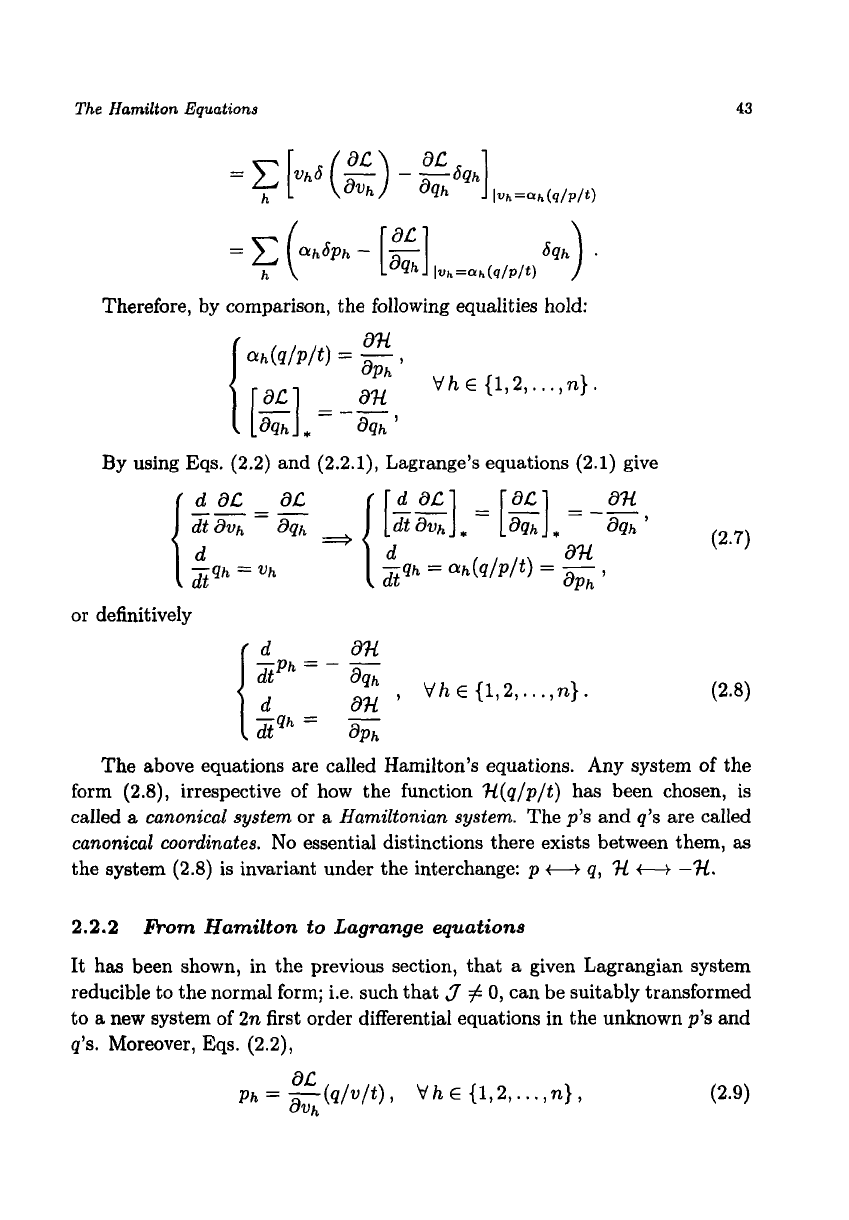
Therefore, by comparison, the following equalities hold:
By using
Eqs.
(2.2)
and
(2.2.1),
Lagrange's equations
(2.1)
give
d
ac ac
d
dC
(2.7)
or definitively
8%
,
Vhe
{1,2
,...,
n}. (2.8)
The above equations are called Hamilton's equations. Any system of the
form
(2.8),
irrespective of how the function
3t(q/p/t)
has been chosen, is
called
a
canonical system
or
a
Hamiltonian system.
The
p's
and
q's
are called
canonical coordinates.
No
essential distinctions there exists between them,
as
the system
(2.8)
is invariant under the interchange:
p
t)
q,
3t
C)
-3t.
2.2.2
It
has
been shown, in the previous section, that a given Lagrangian system
reducible to the normal form; i.e. such that
J'
#
0,
can be suitably transformed
to
a
new system of
2n
first order differential equations in the unknown
p's
and
q's.
Moreover, Eqs.
(2.2),
fiorn
Hamilton to Lagrange equations
(2.9)
ac
ph=--(Q/v/t),
VhG
{1,2,...,n},
avh
