Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.

44
Hamiltonian
Systems
once solved with respect to
v's,
have the form
(2.10)
where
7-l
denotes the Hamiltonian function defined by Eq.
(2.6).
It is,
of
course,
possible
to
go back. Suppose that we start from Eq.
(2.8)
and that the Hessian
determinant,
of
3t
is nonvanishing,
so
that the equations
can be solved with respect to
p's
to give
Ph
=
@h(q/V/t)
1
vh
E
{1,2,
-
* *
3
n>
Let
us
then define the Lagrangian function corresponding
to
7-l
by
L
=
xvhph(q/V/t)
-
x(q/P(q/v/t)/t)
7
h
or
shortly
and consider its differential,
By using again the observation that
(Sf)*
=
S(f),,
we obtain

The
Hamilton
Equations
45
Therefore, we have
(2.11)
By
using the above relations, Hamilton’s equations
(2.8)
give
d
813
d
dC
--
-
=-
[g]
*
=
dQh
1
-Ph
=
--
which are just the Lagrange equations
(2.1).
We finally observe that the Hessian matrices
of
the Lagrangian and of the Hamiltonian, respectively, are inverse to each
other, since
a2c
a2~
SO
that
I?
=
3-l.
2.2.3
Remarks on Hamilton’s equations
The
virial
theorem
The time average
f
of
a
function
f(t)
is
defined by the following limit:

46
Hamzltonian
Systems
From the definition, it turns out that the time average of
a
function, which
is the time derivative
f
=
dF/dt
of
a
bounded function
F(t),
is vanishing.
Indeed,
If
the motion
of
a simple system, whose potential energy
U(q)
is
a
homoge-
neous function of coordinates, develops in a bounded region of the space, there
exists a very simple relation among the time averages of the potential and
kinetic energies. This relation
is
known under
the
name
of
the
virial
theorem.
Let us start from Eq.
(2.6)
written in the following form:
The above relation can also be written as follows:
In the case the motion takes place with bounded “velocitied’ in
a
bounded
region
of
the configuration space, by taking the time averages of both sides,
we have
~
d3c
m=xqh-.
%h
For a simple system, the quantity
3c
+
C’
is
twice the kinetic energy
7*,
so
that we can write
We
can finally argue that, for
a
simple system whose potential energy
U(q)
is a homogeneous function of coordinates of degree
a,
and for motion with
bounded velocities in
a
bounded region
of
space, the interesting relation
holds.

The
Poisson
Bracket
and
the
Jacobi-Poisson
Theorem
47
Given that, for
a
simple system,
3t
=
7"
+
U,
the above relation can be
also written in the following equivalent form:
where
E
is the total energy.
In particular,
0
for
small oscillations:
7;
=
ii
=
(1/2)E,
for
the Newtonian interaction:
27;
=
-a,
or
E
=
-7,
which given
that
F
>
0,
says that the Newtonian motion will be bounded in space
only if the total energy
E
is negative.
The above analysis can be also carried out,
of
course,
by means
of
Lagrange's equations. The reader is invited to do it himself.
2.3
The
Poisson
Bracket
and
the
Jacobi-Poisson
Theorem
2.3.1
The
state space
The solution of the Lagrangian equations, describing the motion of
a
given
arbitrary holonomic system
S
with
n
degrees of freedom and Lagrangian
coordinates
q1,
q2,
.
.
.
,
qn,
is given by
n
functions of time
qh=qh(t),
VhE
{1,2,..*,n}, (2.12)
representing,
at
each time
t,
the position of
S.
Their derivatives
~h
=
&(t)
,
Vh
E
{1,2,.
..,n},
(2.13)
will give at each time
t
the corresponding velocities. From Cauchy's theorem
view point, the state
of
S
is
characterized by the
2n
parameters
(q/q).
In this
way, it appears convenient in the analysis
of
the motion, to use a hyperspace
representation, considering the
2n
parameters
q
and
q
as
Cartesian coordinates
of
a
2n
dimensional space
E.
Since each point of this space represents
a
state
{P,vp}
of
S,
E
is called the
state space.
The motion in
E,
or
to be more
precise, the sequence of states in
E
will be represented by the parametric
equations
(2.12)
and
(2.13).
48
Harniltonian Systems
2.3.2
The
phase space
An analogue geometrical representation is introduced for the canonical coor-
dinates
p,
q,
by considering them
as
Cartesian coordinates of
a
2n dimensional
Euclidean space
@
called, after Gibbs, the
phase space.
In this space any
solution,
of the canonical system
(2.8)
is represented
by
a
(integral) curve often called,
regarding
t
as
a
measure of time,
a
trajectory. In this way, we will have
ooZn
trajectories corresponding to possible choices of the
2n
arbitrary constants,
from which the general integral
of
the canonical system depends.
2.3.3 First
integrals
Let us consider the following canonical system again:
As
for
any first order differential system of equations, any function
f,
such
that the relation
f(p/q/t)
=
constant
(2.14)
is identically satisfied for
all
solutions of the system is called
a
first
integral,
or
shortly, an
integral
of the canonical system. In other words, if
denotes an arbitrary solution of the given canonical system, representing the
parametric equations of an integral curve in the phase space, the function
f
in
the left hand side
is
such that
f(p(t)/q(t)/t)
=
constant.
For
this reason, the function
f
is also called an
invariant.
The Poisson Bmcket and the Jacobi-Poisson Theorem
49
Of
course, the function
f
may take
on
different constant values for different
trajectories in the phase space. More precisely, denoting with
po,qo,to,
the
corresponding initial values
of
p,
q,
t,
the constant must be chosen to coincide
Let
us
recall that, if the Lagrangian does not depend explicitly on time
t,
with
fb0,
qo,
to).
the total energy is
a
first integral:
a.c
E(q/v/t)
=
XVhG
-
L
=
constant.
h
As
for the equivalence between any Lagrangian system and the correspond-
ing canonical system, it is expected that the Hamiltonian function,
if
not de-
pending explicitly on time
t,
is
a
first integral of the canonical system.
It
is interesting to prove the above statement directly, since the result
follows from a general identity which will turn out useful later.
The rate of change
of
any function
f,
depending on the
2n
coordinates
(q/p)
and the time
t,
under the evolution described by
Eq.
(2.8),
is given by
(2.15)
Thus, for
f
=
31,
we have
a31
-
-_-
dt at
*
Therefore,
if
the Hamiltonian does not depend on time
t,
the Hamiltonian
function
31
defines, for the canonical system, a first integral which can
be
also
called the generalized integral of the energy.
Simple first integrals exist when the characteristic function
3t
does not
depend on some
q's.
For instance,
if
a3c/aqr
=
0,
from
Eq.
(2.8)
it follows:
p,
=
constant
These types of integrals also are called
generalized momenta,
as
they coin-
cide with the ones given by Lagrangian systems for cyclic coordinates. In this
case, in fact, if the Lagrangian function does not depend, for instance, on
qrl

50
Hamiltonian Systems
the same is true for
and for their inverse
oh
=
ah(q/p/t)
,
v
h
E
{
192,
.
.
.,
n)
.
It follows also that the characteristic function
31
will not depend on
qr.
a
canonical systems, it does not appear in
Vice versa, if
q,.
does not appear in the characteristic function
31(p/q/t)
of
az
qh
=
-
,
'dh
E
{1,2,
.
.
.
,
n}
,
aph
2.3.4
The
Poisson
Bracket
Equation
(2.15)
can be written in the following form:
where the bracket of any two functions,
f
and
g,
defined by
(2.16)
is called the
Poisson$ Bracket
of
f
and
g.
The Poisson Bracket satisfies the following identities:
antisymmetry
{f,g)
=
-{g,fI,
(2.17)
Jacobi identity
{f,
(9,
h))
+
(9,
{h,
f))
+
{h,
{f,g))
=
0
>
(2.18)
$Sirneon Denis Poisson, author
of
the
%it2
de
mdcanique
(Paris,
1831),
was
born in
Pithiviers (Loiret) in
1781
and died in Paris in
1840.
He
was
a professor
of
mechanics at the
Sorbonne University.

The
Poisson
Bracket and the Jacobi-Poisson Theorem
51
a
derivation
{f,
9
+
h)
=
if,
9)
+
if,
h),
{h,c}
=
0
If,
Sh)
=
{f,
dh
+
df,
h)
9
(2.19)
vc
E
R.
Properties
(2.17)
and
(2.19)
follow easily from the definition, and their
proof is left to the reader. Property
(2.17)
simply expresses the antisymmetry
of the bracket, while properties
(2.19)
simply say that the Poisson bracket
has
a
natural compatibility with the usual associative product of functions, on
which it acts
as
a derivative.
The Jacobis identity also follows directly from the definition and the reader
can check it by “brute force.”
An elegant proof can be given
as
follows:
Let
us
observe that the left hand side of
Eq.
(2.18)
is
a
sum of terms, each
one being a product .of first partial derivatives of two of the three functions
f,
g,
h
with a second partial derivative
of
the remaining function like
Therefore, the Jacobi identity will be proven
if
we are able to show that the
left-hand side of
Eq.
(2.18)
does not contain any second partial derivative. For
this purpose, let
us
introduce, for any function
f,
the first order differential
operator
Xp
defined by
xfg
=
{f,&J)
1
which will be called the Hamiltonian vector field associated with
f.
The explicit
expression
of
Xf
is
given
by
h
$Karl
Gustav Jacobi was born in Postdam in
1804
and died in Berlin in
1851.
He
is
universally known for the investigations on elliptic function, for his papers on determinants
and particularly the
Jacobian determinant,
for the
Jacobi identity,
which is basic almost
everywhere in physics and mathematics, and for the
Hamilton-Jacobi theory,
which was a
starting point for quantum theory. Most of the results of the researches are included in his
Vorlesungen
uber Dynamik.
52
Hamiltonion
Systems
In
terms of these operators, the left hand side of
Eq.
(2.18)
can be handled
as
follows:
Therefore, the following remarkable relation holds:
{f!
(9,
h))
4-
(9,
{h,
f))
+
{h9
if,
9))
=
[Xf,
Xglh
-
X{f,g}h
1
(2.20)
where the bracket
[X,
Y]
denotes the
commutator
of
the differential operators
X
and
Y.
The final observation is that
(a) the last term Xif,,)h does not contain second derivatives of
It.
(b)
the commutator
[X,
Y]
of two first order differential operators
is
again
a first order differential operator.
Indeed,
if
a
a
X
=
xXi(z)-
and
Y
=
xYi((z)-
i
axi
i
denote two first
order
differential operators, we have
i
a
ij
ij
axj
axi
ij
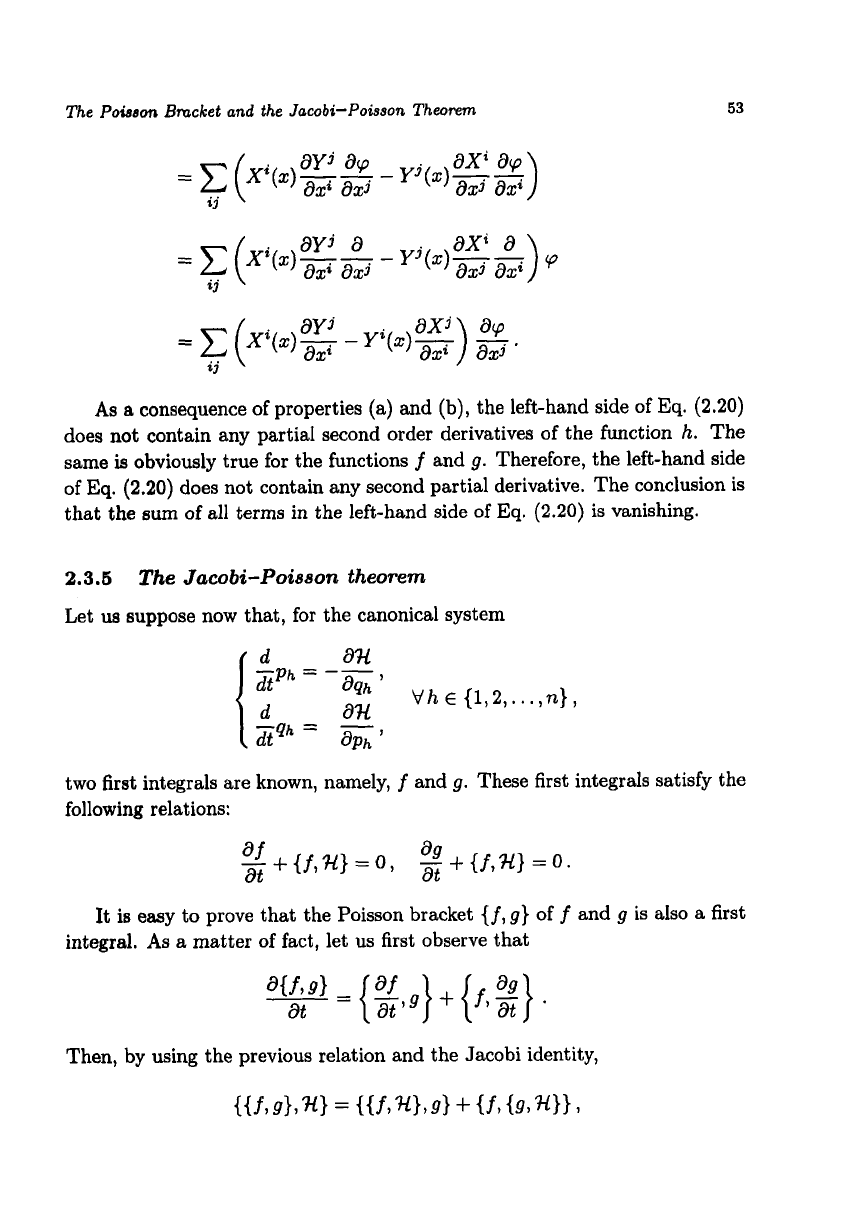
The
Pobson
Bmcket
and
the Jacobi-Poisson Theorem
53
As
a
consequence of properties (a) and (b), the left-hand side of Eq.
(2.20)
does not contain any partial second order derivatives of the function
h.
The
same
is
obviously true for the functions
f
and
g.
Therefore, the left-hand side
of
Eq.
(2.20)
does not contain any second partial derivative. The conclusion is
that the
sum
of all terms in the left-hand side
of
Eq.
(2.20)
is
vanishing.
2.3.6
The
Jacobi-Poisson
theorem
Let
us
suppose now that, for the canonical system
8%
a3c
ZPh
=
--
VhE
{1,2
,...,
n},
aqh
’
{I
aph
’
Zqh=
-
two first integrals are known, namely,
f
and
g.
These first integrals satisfy the
following relations:
af
-
+
{f,3t}
=
0,
g
+
{f,3t}
=
0.
at
It
is
easy to prove that the Poisson bracket
{
f,
g}
of
f
and
g
is also
a
first
integral.
As
a
matter
of
fact, let us first observe that
Then, by using the previous relation and the Jacobi identity,
