Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.


114
The
Integration
Methods
Since the Jacobian determinant
d(g1,
. . .
,
g,)/d(pl,
.
.
.
,
p,)
is nonvanishing,
from
Eq.
(4.33)
it
follows
that
{g,.,
gs}
=
0
==+
xp
=
0
*
x,!~)
=
o
=+
{pi
-
qi,pj
-
pj}
=
0.
Similarly,
by
using
for
Eq.
(4.34)
the same argument,
we
finally have
4.6.3
Let us consider
a
canonical system with a Hamiltonian function
'?I,
which does
not explicitly depend on the time. It has been shown that the knowledge of
n
first integrals
f,.(p/q),
r
E
{I,.
.
.
,n},
which are functionally independent;
i.e.~~=lXhdfh=O~~~=O,ininvolution;i.e.{f,,f,}=O,r,s~{1,
...,
n},
and such that the algebraic system defined by
f,.(p/q/t)
=
?rh
can
be
solved
with respect to the
n
variables
p,.,
allows us to trivially integrate the equations
of
the motion.
Arnold has given
a
global formulation of the theorem by requiring that the
level manifold
M,
be compact and connected. This will be treated in details
in Part
111.
Here we shall limit ourselves
to
the following considerations.
Let us first observe that
W,
in Eq.
(4.28),
is defined only locally.
AS
a
consequence, the coordinates
x
are not uniquely defined on
Mf(,).
They will
be continuous multivalued functions of the point
p
E
Mf(,):
Remarks
on
the Liozlville theorem
x
:
P
E
Mf(,)
+
P'
=
X(P)
E
8,
*
Therefore, to each point
p
E~
Mf(,)
we can associate
a
point
p'
E
Rn
whose coordinates are just given by
x',
x2,.
.
.
,
xn.
Really,
as
the
x's
are not
uniquely defined,
we
can associate to
p
infinitely many points, one for any
chosen determination of the
x's:
P
E
Mf(?r)
-b
P'l,P;,PL..
.
'
It is clear that,
as
the
x's
change continuously,
all
points
p',
associated with
Let us investigate more closely the multivalued structure of the
x's.
A vector
a'
will be called
a
period
if
V
2,
2
and,
2
+
a"
represent the same
point in
Mf(..).
Of course, it will be independent
on
2,
as
2
and
g+
a'
are both
solutions
of
Eq.
(4.29).
Moreover, the modulus
la'/
of a'
cannot be arbitrarily
small since, in
a
sufficiently small region,
2
is single-valued.
all the points
p
of
Mj(,),
will
cover the whole space
Rn.

The
Liouville
Theorem on the Complete Integrability
115
If
a'l
is
a
period with
a
minimal modulus, then
mla'l,
with
ml
E
N,
is still
a
period. Furthermore, any period which is parallel to
a'l
must be an integer
multiple of
a'1.
In fact,
if
a''
=
XZl,
with
X
E
(R
-
N),
then by denoting with
[A]
the maximal integer lower than
A,
Z'-
[XI21
=
(A-
[A])Z1
would be
a
period
with modulus lower than
Ia'11.
As
a consequence, any new period
a'
will have
a component which is orthogonal to
Zl.
By choosing among them, the period
Z2
whose component orthogonal to
a'l
has the lowest modulus, it turns out
that the vectors
mla'l+
m&
are periods. Moreover, in the plane spanned by
21,
a'2
there are no periods of different form. It follows, inductively, that all
periods are of the form
mla'l+
771222
+
+
m,Z,
,
r
E
(0,.
.
.
,n},mi
E
N,
where
r
=
0
corresponds to the absence of periods and
r
5
n,
since all vectors
cii
are, by construction, linearly independent. Thus, each point
p
E
Mf(,)
will
have just one image in each parallelepiped with sides
Zi
(if
r
<
i
5
n, then
cia
=
00).
The motion region is bounded if
r
=
n and unbounded
if
r
#
n.
If
n
=
2,
three cases can occur:
0
T
=
0
+
Mf(,)
is topologically equivalent to a plane;
0
T
=
1
+
Mf(,)
is topologically equivalent to a cylinder;
0
T
=
2
+
Mf(,)
is topologically equivalent to
a
torus.
Only
in the last case the motion region is compact.
More generally,
if
dim
Mf(,)
=
n,
the compact hypersurface corresponding
to
r
=
n
is called an n-torus
T".
In any case, the motion develops on
Mf(,)
c
a,
which
is
invariant.
4.6.4
Action- angle coordinate8
Let
us
consider more closely the case of the torus. By denoting with
71
the
closed curves, which on
Mf(,)
are images
of
segments
Xcil
with
0
5
X
6
1,
let
us define
The
J's
will be first integrals,
as
they are functions
of
the
f's
and
1
Jl=
-AlW,
2n

116
The Integration Methods
where
AlW
represents the variation of
W
along the curve
71.
Moreover, they
will be independent and involutive since
Therefore, starting from the very beginning with the
J’s
instead of the
f’s,
we can introduce their conjugate variables
Ph
in the same way
as
the
x’s
were
introduced
as
conjugate variables to the
f
’s.
Along
a
cycle
Th,
we will have
(4.35)
According to the above equation, the
‘p’s
are angle variables, since their
variation is
27r
along any closed walk, turning the torus just one time. Their
conjugate momenta
J
give, apart from a constant factor the variation of the
action
W
along
a
cycle in which all the
p’s,
but one, are constant.
For
this
reason they are called action variables. The Hamiltonian function
K
=
31*
will
be function of the action variables
J
alone, and the angle variables satisfy the
equations
whose integration give
The motion described by them is called a multiperiodic
motion
with
fre-
quencies
Vh.
Let
us
finally observe that the action-angle variables are not uniquely de-
fined, since any linear transformation
of
the
(p’s,
with integer coefficients and
determinant of the associated matrix equal
to
1,
will again give angle variables,
whose conjugate variables will still be action variables.
4.6.5
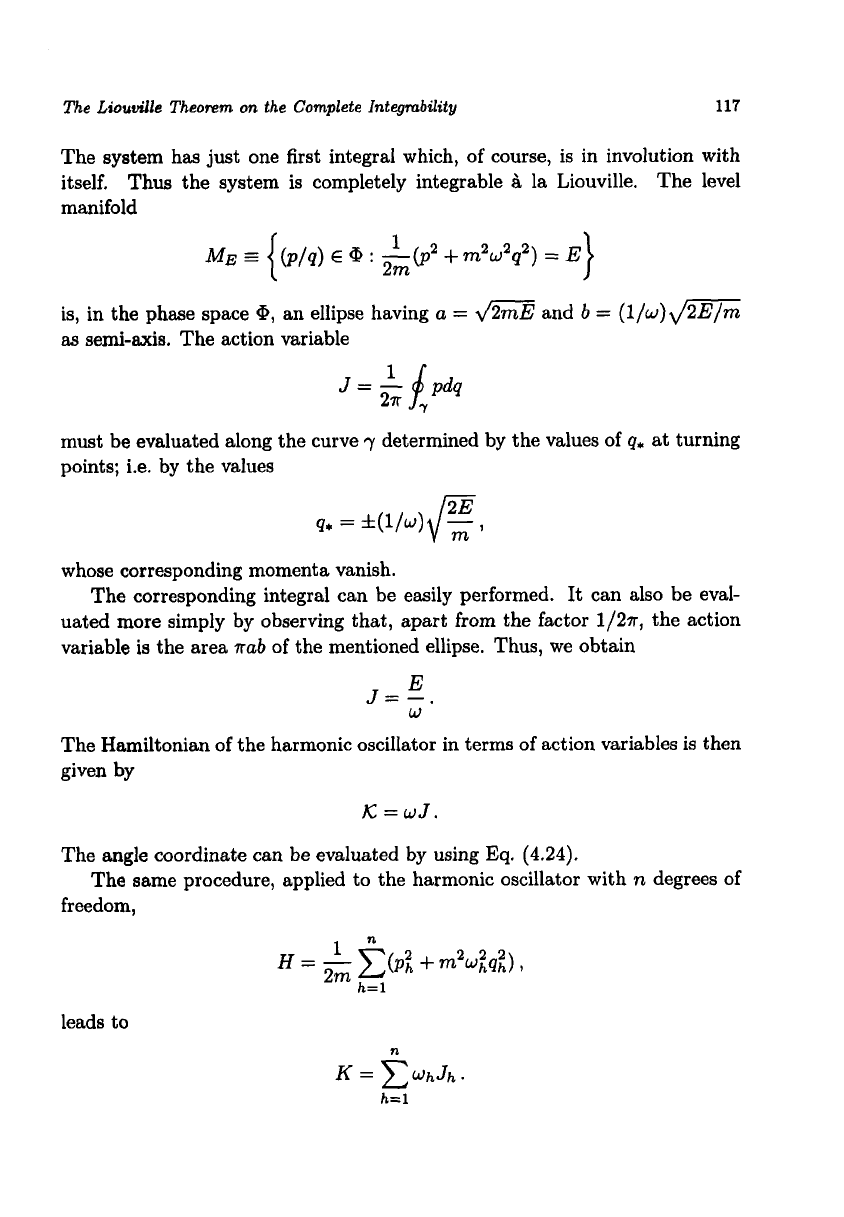
The Hamiltonian
of
the harmonic oscillator, with
n
=
1
degree
of
freedom,
is
given by
The action-angle coordinates for the
harmonic
oscillator
The
Liouville
Theorem on the Complete Integmbility
117
The system has just one first integral which,
of
course, is in involution with
itself.
Thus
the system is completely integrable
B
la Liouville. The level
manifold
is, in the phase space
a,
an ellipse having
a
=
as
semi-axis. The action variable
and
b
=
(l/w)dm
must be evaluated along the curve
7
determined by the values of
q*
at
turning
points; i.e. by the values
whose corresponding momenta vanish.
The corresponding integral can be easily performed.
It
can also be eval-
uated more simply by observing that, apart from the factor
1/2n,
the action
variable
is
the area
nab
of
the mentioned ellipse. Thus, we obtain
E
J=
-.
W
The Hamiltonian of the harmonic oscillator in terms
of
action variables
is
then
given
by
IC=wJ.
The angle coordinate can be evaluated by
using
Eq.
(4.24).
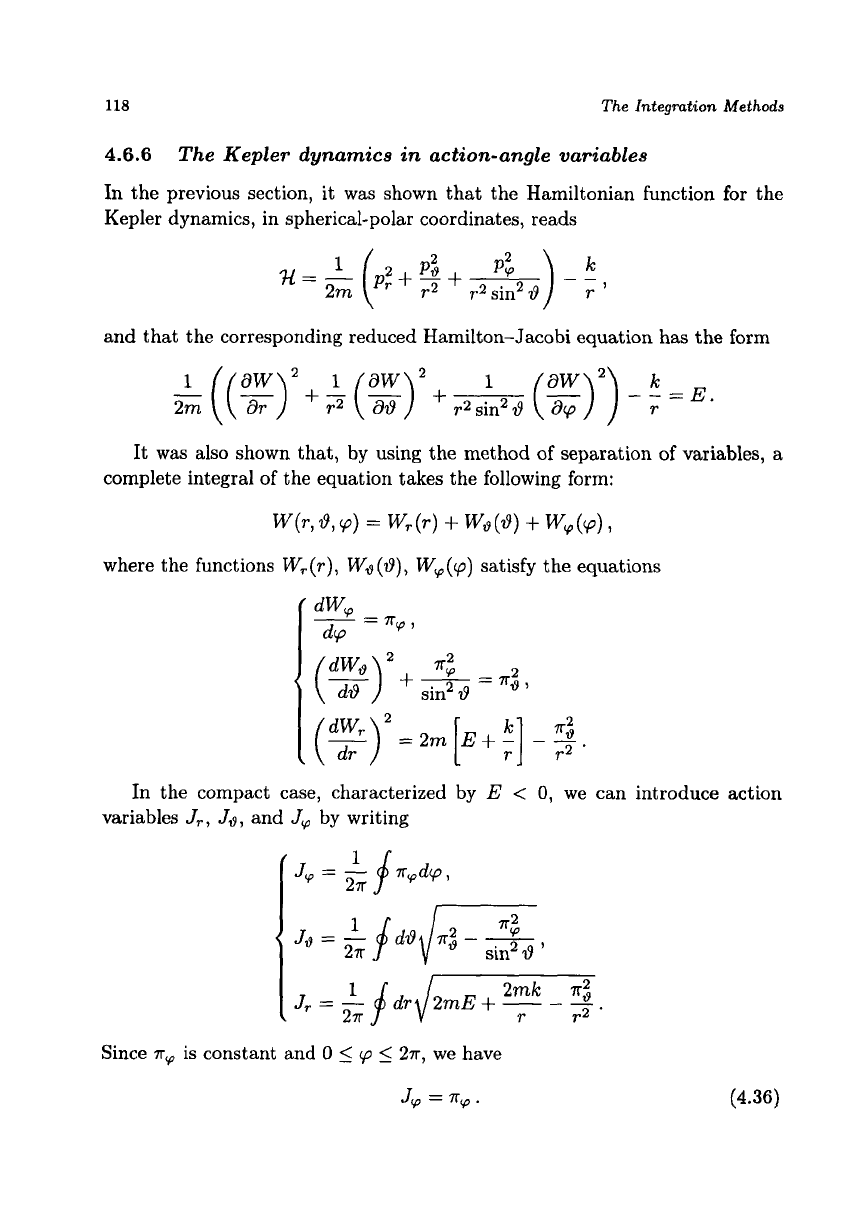
freedom,
The same procedure, applied to the harmonic oscillator with
n
degrees of
leads to
118
The Integration Methods
4.6.6
In the previous section, it was shown that the Hamiltonian function for the
Kepler dynamics, in spherical-polar coordinates, reads
The Kepler dynamics
in
action-angle variables
and that the corresponding reduced Hamilton-Jacobi equation has the form
It
was
also shown that, by using the method of separation of variables,
a
complete integral of the equation takes the following form:
where the functions W,.(r),
We(d),
W,(cp)
satisfy the equations
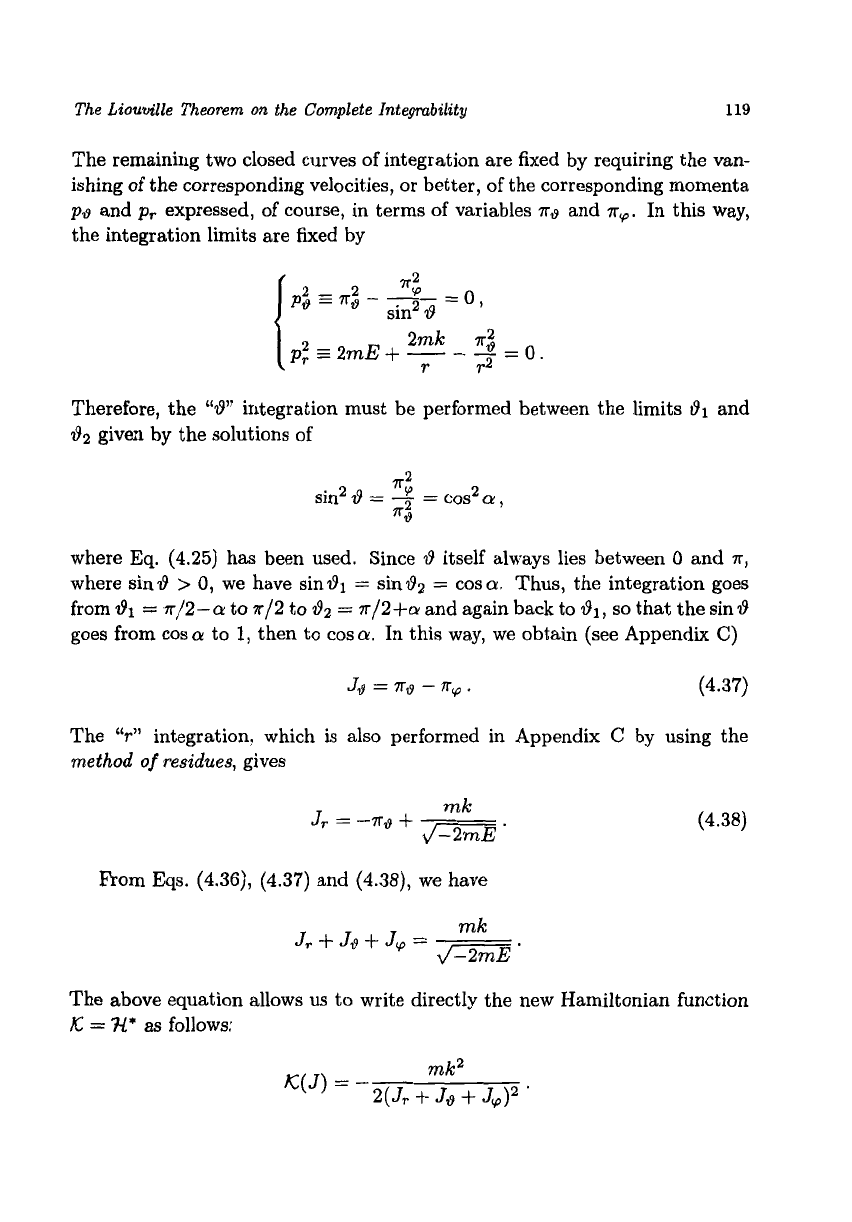
In the compact case, characterized by
E
<
0,
we can introduce action
variables
J,,
JG,
and
Jp
by writing
I
n2
2n
I
Since
n,
is constant and
0
5
cp
5
2n,
we have
J,
=
IT,+,
.
(4.36)
~
The
Liouville
Theorem
on
the
Complete Integrability
119
The remaining two closed curves of integration are fixed by requiring the van-
ishing of the corresponding velocities,
or
better, of the corresponding momenta
p.9
and
p,
expressed, of course, in terms of variables
n.9
and
xv.
In this way,
the integration limits are fixed by
Therefore, the
“6”
integration must be performed between the limits
61
and
62
given by the solutions of
where Eq.
(4.25)
has been used. Since
6
itself always lies between
0
and
T,
where sin6
>
0,
we have sin61
=
sin192
=
COSQ.
Thus, the integration goes
from
291
=
1r/2-~
to
7r/2 to
62
=
7r/2+a
and again back to
61,
so
that the sin6
goes from
COSQ
to
1,
then to
COSQ.
In this way, we obtain (see Appendix
C)
The
“P
integration, which is also performed in Appendix
C
by using the
method
of
residues,
gives
(4.38)
From Eqs.
(4.36), (4.37)
and
(4.38),
we have
The above equation allows us to write directly the new Hamiltonian function
K:
=
‘H*
as
follows:
mk2
2(JT
+
J.9
+
Jv)2
K(J)
=
-

120
The Integmtion
Methods
4.6.7
The perturbations
of
integrable systems and the
KAM
theorem
There exist very few dynamical systems which satisfy Liouville’s theorem hy-
potheses. Generally, for Hamiltonian function not depending explicitly on the
time
t,
there exists just the first integral given by the Hamiltonian. Some classi-
cal integrable systems are given by
systems with a central symmetry, a particle
in a Newtonian gravitational field generated
by
two fixed points, the spherical
top in a Newtonian gravitational field.
There exist some more dynamical sys-
tems with finitely many degrees
of
freedom, recently found in connection with
integrability problem in field theory, where much more examples
OCCU~.~~~**~
For
the applications, it is interesting to elaborate methods which will al-
low
us
to study
a
given Hamiltonian dynamics by separating its Hamiltonian
function
‘fl
as
the sum of two parts. The first part, namely
%o,
is required to
be a completely integrable one,
so
that
‘fl
=
‘flo
+
XZ1
,
where
X
is
a
“small” parameter and is an analytic function of
2n
variables.
By using action-angle variables
(J,
cp),
the above equation can be written
as
follows:
%(J,(P)
=%o(J)
+X’Hi(J,cp).
For
X
=
0,
the phase space is foliated, according to Liouville’s theorem, in
n-dimensional invariant tori
MJ(~),
defined by
Jh
=
?Th,
on which the curves,
‘Ph
=
vh(J)t
+
(Ph(0)
1
completely wound.
It was common opinion, before
1954,
that
X
#
0
completely destroys the
foliation in invariant tori and the beautiful geometrical structure underlying
integrable systems, giving rise to the ergodic behavior; that
is,
to orbits densely
filling the submanifold
3t
=
constant. Therefore, the question
is
to know what
remains of this geometrical structure when
X
#
0.
This opinion was supported
by the fact that in the perturbative series there appear denominator terms
like
v’
k,
where
k
=
(k1,
ka,
. .
.
,
kn)
are integer numbers. Therefore,
when
the
ratios
vi/vj
are rational numbers, the series diverges. To say that the ratios
v,/vj
are rational numbers is equivalent to say that there exists a period
TI
which
is
a multiple of all period
rj
=
l/vj,
so
that the orbit on the torus
T”
is
closed.
In
such cases the torus is said to be
resonant.
Beyond this case, close
+
-I

The Liouville Theorem
on
the Complete Integrability
121
to the resonance there will appear, however, terms too large (little divisors),
since the rational numbers
Q
are dense in
R.
This happens, for instance, in
the case
of
Jupiter and Saturnus, which move along their orbits each day by
299"l'
and
120"5'
degrees, respectively,
so
that
2vl-
5vz
N
0.
The existence of
a strong perturbation, with a large period, of the motion
of
planets, connected
with the little denominator
2vl
-
5v2,
was
already known
to
Laplace.
The presence of
v'
-
i
in the denominators can be easily understood, by
considering that the terms in the Fourier's expansion of
3c1:
in
a
perturbative scheme, will be derived or integrated in
t.
Finally, in
1954,
a positive answer about the applicability of perturbative
methods and the role
of
the parameter
X
in the convergence of corresponding
series
was
offered by Kolmogorov. His theorem, extended and formalized by
Arnold
(1963)
and Moser
(1967),
is today known
as
KAM
theorem.
The theorem2 proves that, for
sma2l
values of
1A1
and nonvanishing Hessian
of the Hamiltonian, only few invariant tori are destroyed.
A
large number
of them are only deformed by the perturbation. On such deformed tori the
orbits are still dense and almost periodic with
n
frequencies everywhere.
Such
invariant deformed tori correspond to unperturbed initial conditions for which
with
a
and
b
positive constants. It is shown that, for sufficiently large
b,
the
constant
a
is
of order
O(X)
and gives the measure of the lost tori.
We
will not
go
into more details and refer the interested reader to the
literature (besides Arnold's book,2 see for instance Refs.
45
and
3).
4.6.8
The Poincard representation
It is possible to concretely
see
what happens by using the
so
called PoincarP
map.
Let
us
consider a Hamiltonian system with
n
=
2
degrees
of
freedom whose
Hamiltonian function
H
does not depend explicitly on the time
t.
Let
us
also
**Henry Poincar6
was
born in Nancy in 1854, and died in Paris in
1912.
He
was
a
pro-
fessor
at
Paris University and hole Polytechnique. An analysis, by Hadamard, Langevin,
Boutroux, and Volterra, of his basic contribution
to
mathematics and theoretical physics
can be found in
La
Nouvelle Collection Scientifique (Paris: Alcan, 1914).

122
The
Integration
Methods
suppose that the 3-dimensional manifold
ME
=
{p
E
Q
:
%(p/q)
=
E},
defined
by the first integral of the energy, is compact. We know that the existence of
a
second first integral, namely
f,
will ensure the complete integrability, and
that the manifold
ME
will be foliated in 2-dimensional invariant tori
T2.
For
a
given initial condition, the motion
will
be represented by
a
helix belonging just
to one torus and densely winding on it, never returning, provided the torus is
not resonant, exactly at the same point.
Let us now consider the 2-dimensional manifold
C
defined by the equation
representing the intersection
of
ME
with the hyperplane defined by
q2
=
0,
and
a
point
uo
E
C,
which can be fixed by giving
a
point in the plane
S
5
(PI,
q1).
By considering
00
as
the initial condition at the time
to
of the flow of
a,
there will exist
a
time instant, namely
tl,
in which the trajectory will again
meet
C
in
a
point
u1
E
C.
Thus, recursively, there will be a sequence
of
time
instants
tk
in which the trajectory will meet
C
at points
(Tk
E
C.
Since the
(k)
(k)
sequence of points
Uk
E
C
is the image of a sequence
sk
=
(pl
,
q1
)
of
points
in the plane
S,
it is possible
to
describe the evolution by giving the sequence
Sk
E
s.
The map
which describes the evolution, is called the
Poincare'
map.
Furthermore, as it winds on the torus
T2,
the trajectory meets
C
on the
1-
dimensional submanifold determined by the ulterior equation
f(p1
,pz,
q1,
qz)
=
T;
that is, on
a
smooth closed curve, which is also the image of
a
similar curve
of
S.
Therefore, for
a
not resonant torus, the points
sk
will dispose along
a
regular curve, while for
a
resonant torus the sequence will stop; that is, there
will be an integer number
r
such that
sk+r
=
sk,
and
so
on.
The above description can be also applied to
noncompletely integrable
dy-
namics. In this cases the trajectory will not meet
C
along
a
regular curve but in
points
(Tk
covering a 2-dimensional region, which is an image
of
a
2-dimensional
region of
S
(chaotic behavior).
The
KAM
theorem predicts that, by increasing the value of the parameter
A,
it
is
possible to observe, in
S,
a transition from
a
picture composed by regular
curves to
a
picture composed by a large part of previous curves together with
extra points, and then
to
a
picture composed by few curves and
too
many
isolated points covering the whole interested region.

The Liouville Theorem
on
the Complete Integrability
123
Computer analysis gave,
of
course, exactly what was expected and
was
allowed to discover new remarkable completely integrable systems.
Further
Readings
0
G.
dell’
Antonio,
Elementi
di
Meccanica
(Liguori, Naples,
1996).
0
A.
Lichnerowicz,
“Les variktds de Poisson
et
leurs dghbres de Lie associkes,”
J.
Diff.
Georn.
12,
253
(1977).
0
A.
Romano,
Leioni di Meccanica Radonale
(Liguori, Naples,
1990).
0
E.
C.
G.
Sudarshan and
N.
Mukunda,
Classical Dynamic:
A
Modern Perspective
0
W.
Yourgrau and
S.
Mandelstam,
Variational Principles in Dynamics and Quan-
(John
Wiley,
1974).
tum theory
(Dover,
1968).
