Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.


134
Manifolds
and
Tangent
Spaces
5.3.2
The equivalence class
of
the curves
Y(T)
on
M
through
p
is called
tangent
vector
to
the manifold
M
at the point p.
The set of
all
the tangent vectors
to the manifold
M
at
the point
p,
with the
sum
and the product by scalars
defined by
Tangent vectors
to
a manifold
(ax,
+
bYp)(f)
=
aXp(f)
+
bYp(f)
>
is
a
vector space called
tangent space to
M
at p
and it will be denoted with
7,M.
This space has the same dimension of the manifold, and the components
of every tangent vector
X,
E
7,M,
in the local chart
(U,cp)
on which
p
is
represented, are given by the relation
Remark
12
A
tangent vector to
a
manifold
M
at a point
p,
could
equivalently, be defined as a linear function from the space
3(U)
of
differ-
entiable functions, defined
on
a
neighborhood
U
of
p, to
R:
w.4
+
9,
satisfying the Leibnitz rule; i.e.
X,(af
+
bg)
=
aX,(f
+
bXp(9)
,
Xp(fg)
=
f(P)XP(d
+
S(P)X,(f)
1
with
a,
b
E
8,
and
f
and g differentiable functions defined on a neighborhood
of
P.
The value of
X,
in
f
is
X,(f)=
-
x;,
(z)
P
(5.3)
where
2'
=
cpi(p)
and
Xj
are the components of
X,
in the given chart
(U,
cp).
The previous formula
is
often written in the form
A
Lligresaion
on
Vectors
and
Covectors
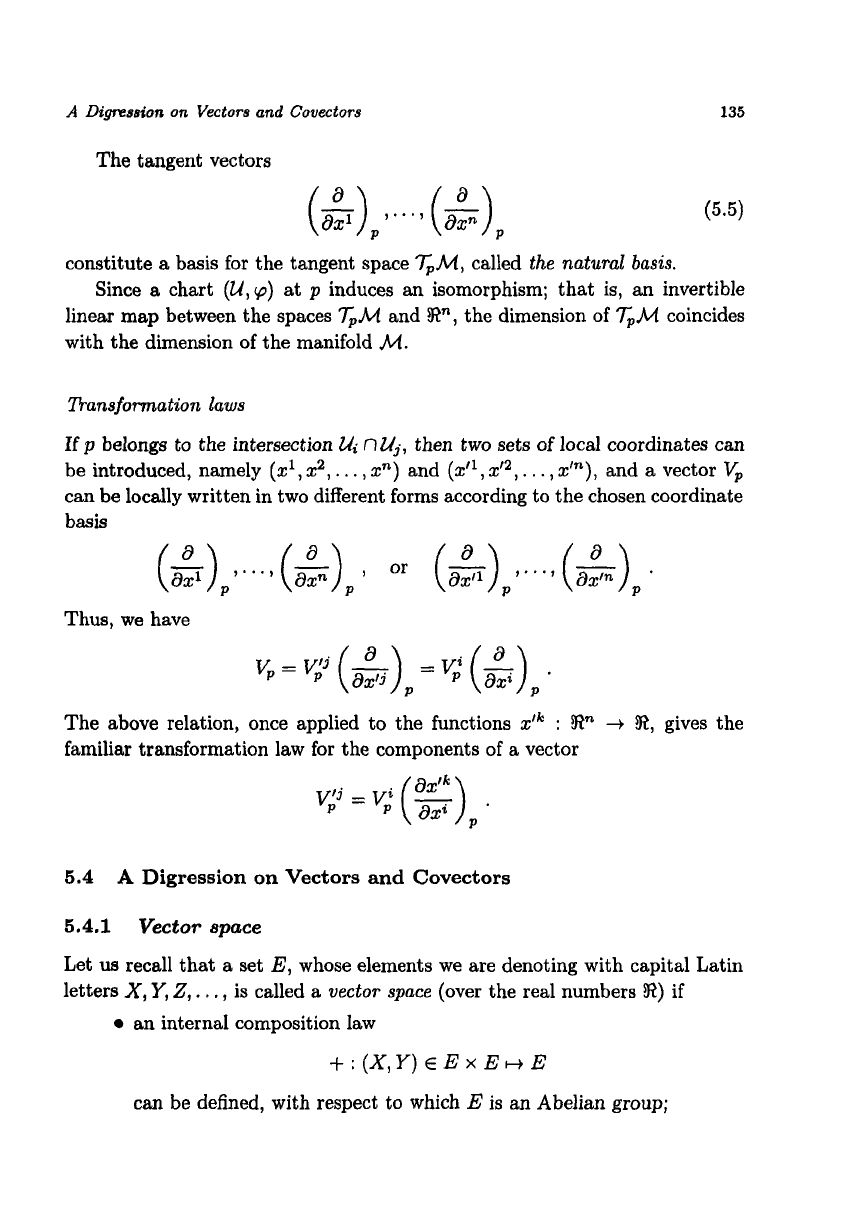
The tangent vectors
135
constitute
a
basis for the tangent space
7,M,
called
the natural
basis.
Since
a
chart
(2.4,~)
at
p
induces
an
isomorphism; that
is,
an
invertible
linear
map
between the spaces
7,M
and
Rn,
the dimension of
7,M
coincides
with the dimension of the manifold
M.
Transformation
laws
If
p
belongs
to
the
intersection
Ui nUj,
then two sets
of
local coordinates
can
be introduced, namely
(d,
z2,.
.
.
,
P)
and
(dl,
xt2,.
.
.
,
xtn),
and
a
vector
Vp
can be locally written in two different forms according to the chosen coordinate
basis
(&)
p,..., (&)p
Or
(gi)pl**..
(&)
*
P
Thus, we have
The above relation, once applied to the functions
dk
:
R"
+
R,
gives the
familiar transformation law for the components of a vector
5.4
A
Digression on Vectors and Covectors
5.4.1
Vector
space
Let us recall that a set
E,
whose elements we are denoting with capital Latin
letters
X,
Y,
2,.
.
,
,
is
called a
vector space
(over the real numbers
8)
if
an internal composition law
+:
(X,Y)
EEXEHE
can
be defined, with respect to which
E
is
an
Abelian group;
138
~~~i~o~ds
and
Tangent
Spaces
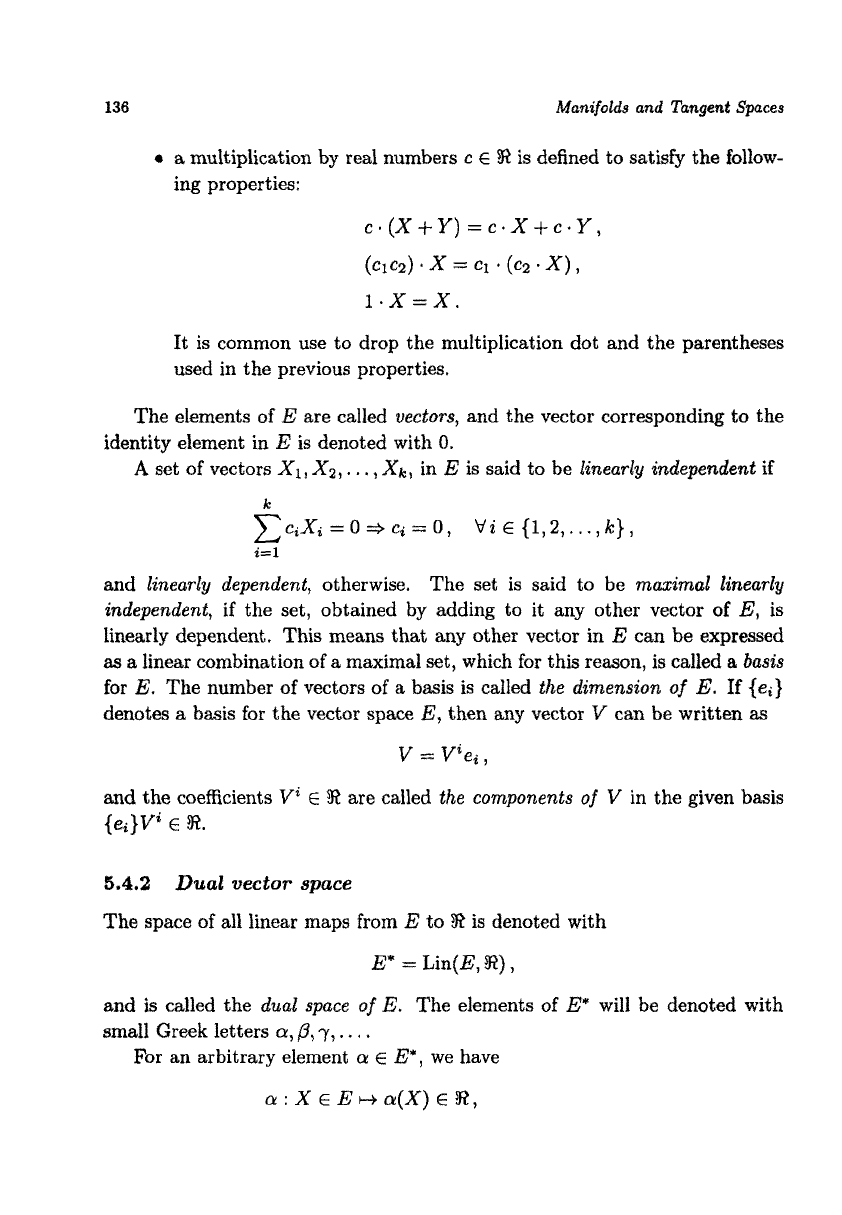
0
a
mu~tip~~~ation
by
real numbers
c
E
92
is defined to satisfy the folfow-
ing properties:
c- (X+Y) =c.X+c.Y,
(c1c2)
x
=
c1
'
(c2
*
X),
l*X=X.
It
is
common use to drop the multiplication dot and the parentheses
used in the previous properties.
The elements
of
E
are called
vectors,
and the vector corresponding to the
A
set
of
vectors
XI,
X2,
. .
.
,
xk,
in
E
is said
to
be
linearly independent
if
identity element in
E
is
denoted with
0.
k
ccixi
=
0
=+
ci
=
0,
vi
E
{1,2,.
,
,
,
k},
i=l
and
linearly dependent,
otherwise. The set
is
said to be
mmimal linearly
independent,
if
the set, obtained by adding to it any other vector
of
E,
is
linearly dependent. This means that any other vector in
E
can
be
expressed
as
a
linear combination
of
a
maximal set, which for this reason,
is
called a
basis
for
E.
The number
of
vectors
of
a basis
is
called
the dzmens~o~
of
E.
If
{ej)
denotes
a
basis
for
the vector space
E,
then any vector
V
can be written
as
V
=
Viei,
and the coefficients
Vi
E
9
are called
the components
of
V
in the given basis
{ei}Vi
E
R.
5.4.2
Rual
vector
space
The space
of
all linear maps
from
E
to
!R
is denoted with
E*
=
Lin(E,
R)
,
and
is
called the
dual
space
of
E.
The elements
of
E*
will be denoted with
small Greek letters
a,
P,y,.
.
.
.
For
an arbitrary element
a
E
E*,
we have
a
:
x
E
El-,
.(X)
E
R,

A
Digression
on
Vectors
and
Covectors
137
.(X
+
Y)
=
.(X)
+
.(Y)
,
vx,
Y
E
8,
.(cX)
=
ca(X),
vc
E
R.
It
is
easy to
see
that
0
E*
is also a vector space, the internal composition law and the multi-
plication by real numbers being trivially defined
as
(.
+
P)(X)
=
.(XI
+
P(X)
9
(ca)(X)
=
c.(X)
.
The vectors of
E*
are called
covectors
in order to distinguish them
from the vectors of
E;
If
el,
e2,
.
.
.
,
en
is
a
basis
of
E,
a
given vector
X
E
E
can be expressed
0
E*
has the same dimension
of
E.
&8
x
=
X'ei,
i
E
{1,2,.
.
.
,n}.
Associated with the given basis
{ei}
of
E,
let
us
introduce the elements
of
E',
namely
d1,d2,.
.
. ,8",
defined by
d'(X)
=
X',
i
E
{1,2,.
.
.
,n},
or equivalently
d'(ej)
=
6;
,
i,j
E
{1,2,,
.
.
,n}
.
Since for any elements
a
E
E*
and
X
E
E,
we have
a(X)
=
cY(Xzei)
=
Xia(ei)
=
.id'(X),
with
ad
=
a(ei)
E
R,
the element
a
can be expressed
as
a
=
.id2.
The set
d1,d2,.
.
.
,dn
is then a basis for
E*.
It
is
also
possible
to
consider the dual
E**
=
(E*)*
of the dual of a given
vector
space
E.
The reader can easily prove that
if
the dimension of
E
is finite,
then
E**
is
isomorphic to
E
and they can be identified.

138
Manifolds and Tangent Spaces
Thus,
E
and
E*
are duals
of
each other; in order to underline the reciprocal
duality, the value that
a
covector
a
takes
on
a
vector
X
is also denoted by the
bracket
(.,
.),
so
that
a(X)
=
(a,X)
.
If
{e:}
and
{ei}
are two different bases and
(19’~)
and
{Si}
are their dual
bases, respectively,
a
given vector
V
can be represented in two different forms.
Then, we have
v
=
V’iei
=
Viei.
Thus, the value of
a
generic element
8”
of
{@}
on
V
will
be
#‘(v)
=
V’i19’k(e:)
=
Vi19’’(ei),
and then
Vtk
=
Mk
iVi,
M
denoting the matrix whose elements are
29”((ei).
5.5
Cotangent
Space
at a Point
As
for
any vector space
E,
we can introduce the dual
TM
of
the vector space
7,M.
The vector space
7,*M
is called the
cotangent space
to the manifold
M
at the point
p.
Special covectors of
TM
can
be
associated with any
differentiable function
f
defined on
M,
as
follows:
Iff
:
M
+
?R
is
a
differentiable function at the point
p
E
M,
its
differential
(or
gradient, or exterior derivative)
dfp at the point p
is the linear map
df,
:
7,M
-i
R
of
the tangent space
7,M
in
R
defined by
(dfP,XP)
=
Xpf
,
vx,
E
7,M.
More explicitly, let
X,
be a tangent vector to
M
in
p,
and
$7)
a
curve,
belonging to the equivalence class of curves through
p,
($0)
=
p),
which
represents
X,,
then
Maps
Between
Manifolds
139
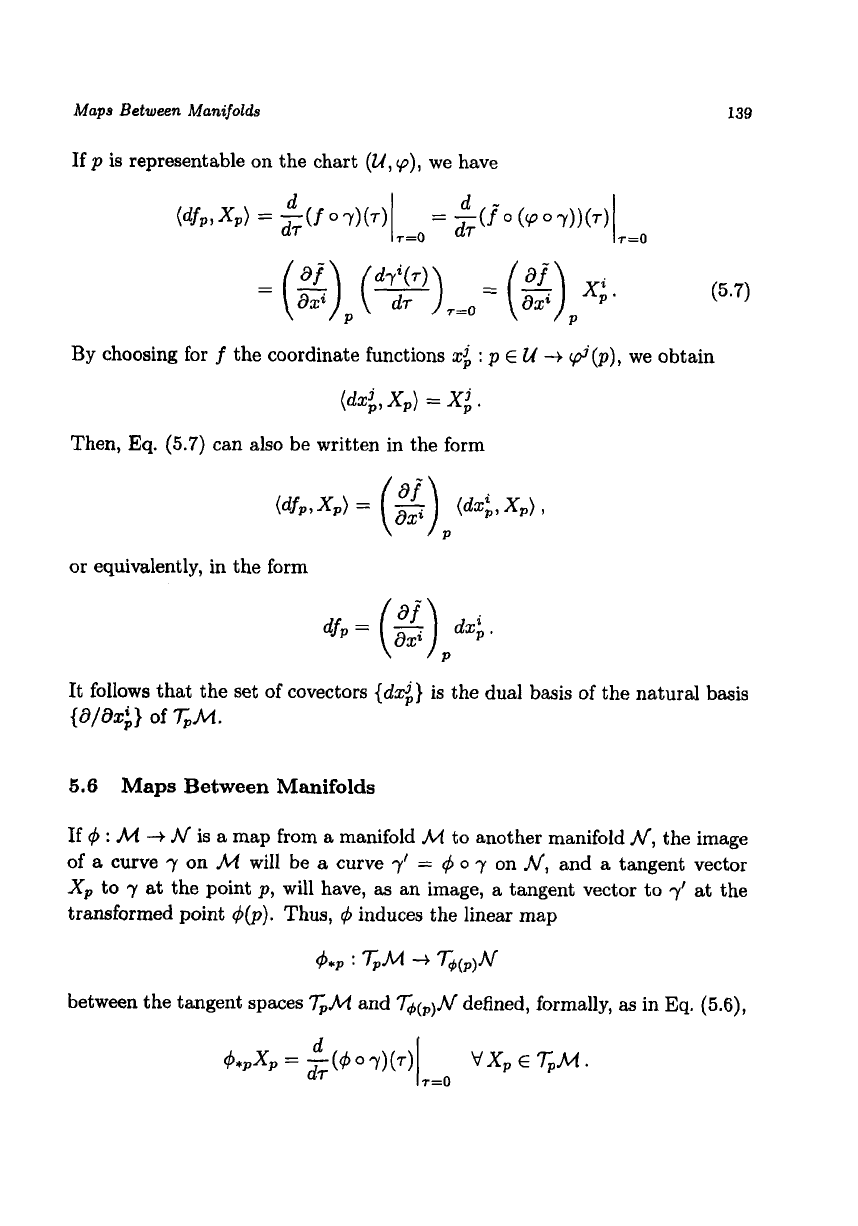
If
p
is representable on the chart
(U,
cp),
we have
By choosing for
f
the coordinate functions
z:
:
p
E
U
+
(pi@),
we obtain
(dxi,
X,)
=
Xi.
Then,
Eq.
(5.7)
can also be written in the form
or equivalently, in the form
df,
=
(3)
dxi.
P
It follows that the set of covectors
{d<}
is the dual basis
of
the natural basis
{a/ax:}
of
7,M.
5.6
Maps
Between
Manifolds
If
4
:
M
-+
N
is a map from a manifold
M
to another manifold
N,
the image
of
a
curve
7
on
M
will be
a
curve
y'
=
q5
o
y
on
N,
and
a
tangent vector
Xp
to
7
at
the point
p,
will have,
as
an image, a tangent vector to
y'
at
the
transformed point
&I).
Thus,
#
induces the linear map
4*p
:
7,M
+
7,(p)N
between the tangent spaces
7,M
and
%(,IN
defined, formally,
as
in
Eq.
(5.6),

140
Manifolds and Tangent
Spaces
However, here
4*,Xp
is a vector belonging to
T&)N.
Indeed, if
p
is
representable in the chart
(24,
cp),
and
q
=
4(p)
in the chart
(V,
$J),
then we
r=o
Vice versa we can associate with
a
given covector
aq
E
TN,
the covector
pp
E
7p'M
defined by
(Pp,
XP)
=
(ap,
4*,X,)
I
with
q
=
+(p).
the pull-back
of
a+(p).
Thus,
4.,Xp
is called the
p~sh-fo~~~rd
of
X,,
while
pP
=
#$a+(,)
is called
5.7
Vector
Fields
A
vector field
X
on
a
manifold
M
is
a
rule which to every point
p
E
M
associates
a
tangent vector
Xp
E
7,M;
i.e.
a
map
X
:p
E
M
-+
X(p)
=
Xp
E
7,M
is defined
on
M.
In
a
local coordinate system,
a
vector field
X
can be written
in the
form
The vector field
X(p)
is
said to be
Ch
differentiable
on
a
Ck
manifold
M,
with
h
5
k
-
1,
if the functions
X'(p)
are
Ch
differentiable
on
the manifold
M.
5.7.1
The natural
basis
{a/&?}
is not,
of
course, the sole possible basis for vector
fields. By taking
n,
point-wise linearly independent, combinations
ei
of
its
elements; i.e.
Holonomic and anholonomic basis
of
vector
fields
d
6x3
ei
=
a:
(z)-
,
i
=
1,.
.
.
,
n
,
Vector
Fields
141
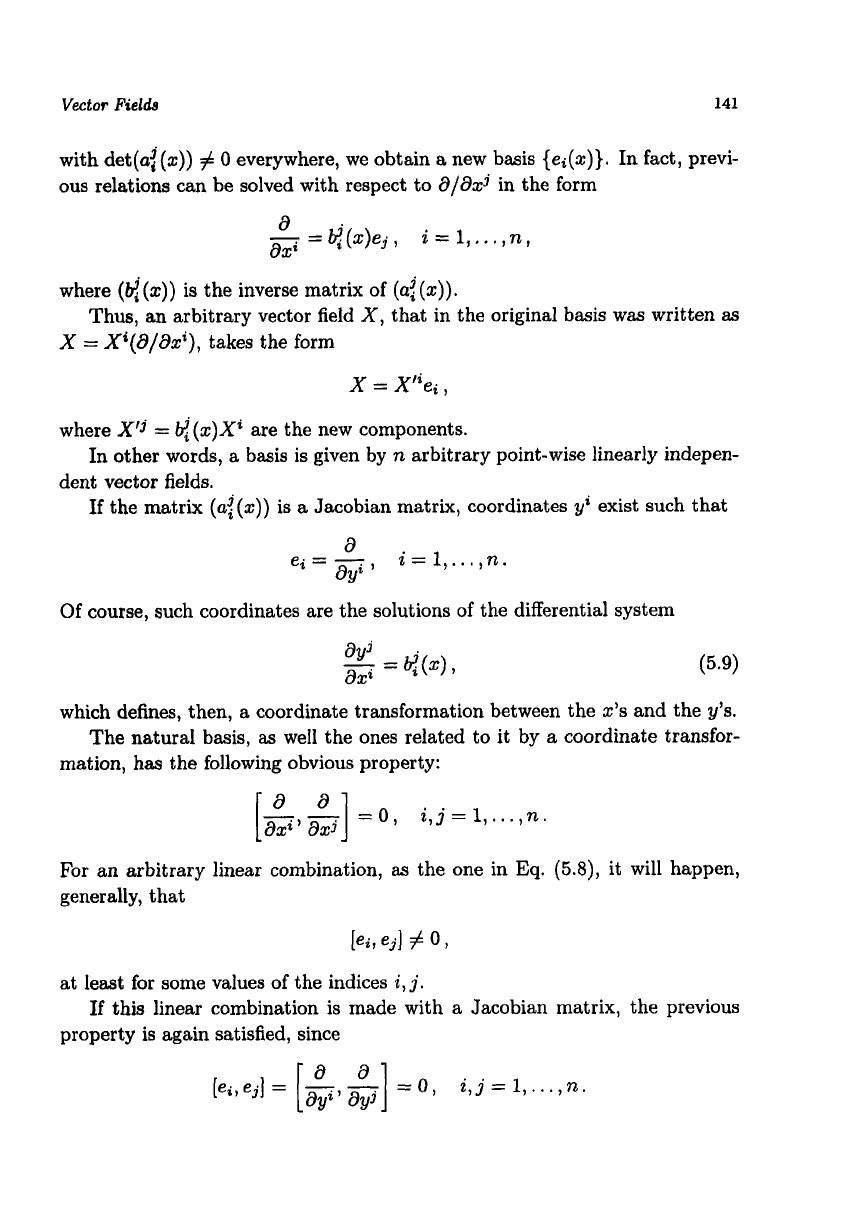
with det(ai(x))
#
0
everywhere, we obtain a new basis
{ei(x)}.
In fact, previ-
ous relations can be solved with respect to
a/dxj
in the form
a.
q(x)ej,
i
=
1
,...,
n,
where (e(x)) is the inverse matrix of (a{(x)).
X
=
Xi(a/axi),
takes the form
Thus,
an
arbitrary vector field
X,
that in the original basis was written
as
x
=
Xtiei,
where
X'j
=
g(x)Xa
are the new components.
dent vector fields.
In other words, a basis is given by
n
arbitrary point-wise linearly indepen-
If
the matrix
(a:(.))
is
a
Jacobian matrix, coordinates
yi
exist such that
i=
1,
...,
n.
a
ei
=
-
ayi
'
Of course, such coordinates are the solutions of the differential system
which defines, then, a coordinate transformation between the
x's
and the
y's.
The natural basis,
as
well the ones related to it by a coordinate transfor-
mation, has the following obvious property:
[&,&I
=o,
i,j
=
1,
...,
n
For an arbitrary linear combination,
aa
the one in
Eq.
(5.8),
it will happen,
generally, that
at least for some values of the indices
i,j.
property is again satisfied, since
If
this linear combination is made with a Jacobian matrix, the previous
(ei,ej]=
-,-
=O,
i,j=l,
...,
n.
[[a
lJ]
142
Manifolds
and
Tangent
Spaces
It is true also the converse, namely:
If
the elements
of
a
basis
{ei}
of
vector
fields
fulfill
the property
[ei,ej]
=0,
i,j
=
1
,...,
n,
then coordinates
yi
exist such that
d
e.--,
i=l,
...,
n.
a
-
dya
This can be understood by observing that the elements
ei
of
a
basis, however,
this one is chosen, are linear combination (with functions
as
coefficients) of the
partial derivatives
d/dxi:
‘a
ei
=
ai(x)-,
i
=
1,.
.
,
,n.
dX.1
Thus,
Then,
and, since det(af)
#
0,
(5.10)

The Tangent Bundle
143
which is just the compatibility conditions to be satisfied, in order
a
differential
system, like
Eq.
(5.9),
admit solutions. In other words, the conditions
say that the differential forms
dp
=
3dx"
p
=
1,.
. .
,n
are closed; that is, functions
yp,
at least locally, exist such that
19,
=
dyp.
an arbitrary basis are of the form
Equation
(5.10)
shows that the commutation relations between elements
of
[ei, ej]
=
4'eP
A
basis for which
=
0,
Vi,
j,
p,
will be called
holonomic
(integrable), or
anholonomic
(nonintegrable), otherwise.
5.8
The
Tangent
Bundle
The union
UpE~7pM
of
all
tangent spaces
7,M
to a manifold
M
can be
endowed with
a
structure of
a
differential manifold in a very natural way. An
element
of
it is
a
pair
(p,
X,)
with
p
E
M
and
X,
E
7,M.
Thus,
a
sys-
tem of local coordinates
(d,
x2,.
.
.
,
x",
X',
X2,.
. .
,X")
for
UpE~7,M
can
be introduced by using the local coordinates
(d,
x2,.
.
.
,
x")
of
p
and the
ones
(X1,X2,.
,
.
,Xn)
of X,.
Regarded
as a
differential manifold, the set
Upc~7&f
is denoted with
TM
and it is called the
tangent bundle of
M,
while
a
single tangent space
7,M
is called
a
fiber
of
TM.
Of course, the
dimension of
TM
is twice that
of
M.
A
curve on
TM
identifies
a
vector at each point of
M,
and
so
it defines
a
vector field on
M.
Such
a
curve; that is,
a
curve
transversal
to the fibers, is
called
a
cross-section of
TM.
The names
bundle, fiber, and cross-section
refer, however, to general struc-
tures that our "special" differential manifold
TM
shares with
a
general class
of
differential manifolds called
fiber bundles.
A
general fiber bundle consists
of
a
base
manifold,
which in our case is
M,
and of one
fiber
attached to each
point of the base space.
If
the dimension of the base space is
n
and the one
of
each fiber is
m,
the bundle has
m
f
n
dimensions. The points
of
each fiber are
related to one another, while points on different fibers are not.
