Teodorescu P.P. Mechanical Systems, Volume III: Analytical Mechanics

Подождите немного. Документ загружается.

MECHANICAL SYSTEMS, CLASSICAL MODELS

382

then, we can write

()

() ()

()

()()

()

111

δ=⋅δ−⋅−⋅δ−⋅δ−⋅δ−δ

′

=⋅δ− ⋅ ⋅δ−δ−⋅δ− ⋅δ

′

−⋅ ⋅δ−δ−⋅δ− ⋅δ−δ

′

δ = ⋅δ − ⋅ ⋅ δ −δ − ⋅ δ − ⋅δ

−⋅ ⋅δ−δ−⋅

vpvv vvvr v r r

vp vv r r vv vv

vr r r vr v r r

v p vv r r vv v v

vr r r v

11112 121 121

11 12 2 1 12 12

1211121

22212 2112 21

22 21 2

mc l l

lll

μμ

μμμ

()

′

δ− δ−δ

rvrr

22 1

,ll

as well as

()

′

δ= ⋅δ−δ+δ

′

δ= ⋅δ−δ+δ

22 2

cc c

μμ μ

νν ν

where the label

r indicates the differentiation with respect to this argument, while

′

indicates the variation with to the other arguments. Hence, for the energy (21.3.46) we

obtain

[

{()

()

}( )

()

()( )

δ= ⋅δ+ ⋅δ

−⋅++ ⋅− +

⎤

⎦

′

++ ⋅δ−δ+ −⋅δ

′′ ′

−δ ⋅ = δ + δ ⋅ + δ

vpvp

vv v v r

vv rr vv

vv v v r

1122

12 1122

11 22 2 1 1 2

12 11 22

rrr

ll c

μμν

μν

(21.3.47)

Applying the Principle 21.3.1 in the form (21.2.41') and taking into account

(21.3.47), we can write

()

() ( )

⋅

⎛⎞⎛⎞

′

−⋅δ+ −⋅δ+ − δ

⎜⎟⎜⎟

⎝⎠⎝⎠

′′′

−δ ⋅ + δ − δ + δ ⋅ − δ=

pp vv

Lr Lr

vv v v r

12 12

12 11 22

20,

cll t

μν

(21.3.48)

with

()

()()

11 22 1 1 2 2

21 2 .

ccllll

μν

⋅

⎡⎤

=− +−+⋅−+

⎢⎥

⎣⎦

Lvvrvv

(21.3.48')

The equations of motion are thus obtained in the form

==−==

,, const

(21.3.49)

Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

383

In a Newtonian language,

L and −L correspond to the internal forces which act upon

the two particles

P and

P , respectively. Taking into account (21.3.46), the condition

of conservation of the energy

leads to a relation of conservation of mass

+++=++ +

12 1 2

22 2 2mm m mμν μ ν,

(21.3.50)

formed by the individual masses

m and

m , the mass of gravitational interaction μ

and the mass of dilatational interaction

ν .

Separating the terms which contain the mass

μ in the relation (21.3.48), we can

write

()

⋅

′′

−δ=δ⋅

21c

μμ

(21.3.51)

where we took into account that the scalar product

⋅vv

does not depend on r ;

writing this relation in the form

(

)

⋅

′

⋅

−

we get

⋅

−

()

(21.3.52)

where

()rϕ is a function which remains to be determined. Taking into account

(21.3.45), this function will be of the form

⎡

⎤

=− +

⎢

⎥

⎣

⎦

()

() 1

cr c

(21.3.52')

the function

()rλ being obtained by considering a particular motion. In case of usual

distances and velocities one can take

=−

(21.3.53)

the respective energy being the potential energy

−

/fm m r of the Newtonian

modelling. The terrestrial experience and that concerning the solar system of our

Galaxy show that the mass

ν can be neglected; we can write, with a good

approximation,

MECHANICAL SYSTEMS, CLASSICAL MODELS

384

()

=++

pvv

111 2

222 1

Hcm m

(21.3.54)

the equations of motion being (21.3.49), with

()

⋅

=−

(21.3.54')

Choosing

==() /, constrkrkλ , we can also write

()

=− +

⋅

−

(21.3.54'')

Once

μ is determined, the condition (21.3.48) leads to

()

′′′

δ= δ+δ ⋅vv r

11 22

2cllν ,

(21.3.55)

where

′′′′′′

δ=δ+δ δ=δ+δ

11 122 2

,lh hlh hνν νν ;

(21.3.55')

we can also write

()()

′′

′

+⋅ δ+δ⋅

⎡⎤

−=

⎢⎥

⎣⎦

vvr v v r

11 22 1 1 2 2

hh h h

This equation can be integrated in the form

()

+⋅

−

vvr

11 22

()

(21.3.56)

if we choose

=⋅=⋅vr vr

111 222

() , ()hrhrψψ,

(21.3.56')

Putting

00 00

12 12

() , ()

mm mm

ψψ

(21.3.56'')

we can write

Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

385

()

vr vr

02 2 02 2

11 1 22 2

002

cos( ,) cos( , )

mv mv

mmc

(21.3.56''')

too, so that we have

≤ 1u , being led to a real value for ν . We notice that ν is

negligible for infragalactic distances; we impose thus

()

=−

()

rgl

(21.3.56

)

where

g is a universal constant,

l being a characteristic parameter of the two particles

in interaction.

In conclusion, in the frame of the invariantive model of mechanics we can write

()

=− +

⋅

−

+−

=− +

⋅

−

+−

122

111

11 1

004

121

222

22 2

004

cos( , )

rm v

mmc u

rm v

mmc u

(21.3.57)

()

=+− +−

⋅

−

12 12

Hcm m f gl

cr c u

(21.3.57')

These results allow to pass to the study of a discrete mechanical system (system of

particles), as well as to the study of a continuous mechanical system.

21.3.3 Applications

We apply the results thus obtained to the study of some problems of celestial

mechanics, making – with this occasion – also some specifications concerning the

functions introduced and their mechanical interpretation; we consider thus the problem

of dilatation of the Universe (Hubble’s law) and the problem of secular motion of the

perihelion of Mercury.

21.3.3.1 Dilatation of the Universe. Hubble’s Law

Let be the system formed by two particles

P and

P , considered in Sect. 21.3.2.3;

starting from (21.3.44), the conservation of the momentum of this system leads to

+++++ ==vvvv rC

11 22 1 2 1 2

()() constmm hhμν

JJJJG

(21.3.58)

MECHANICAL SYSTEMS, CLASSICAL MODELS

386

A scalar product by

r , where we take into account (21.3.56'), (21.3.56''), we denote

cos( , ), 1,2

vjξ ==vr , and divide by r , leads to

++++ = =

122

11 22 1 2

() cos(,)

mm C

ξξ

ξξμξξ ν Γ

Assuming that

+≠

11 22

0mmξξ, dividing by this sum and using the relations

(21.3.54''), (21.3.56), (21.3.56

), we obtain the equation

′′′′

++ + =

2424

11 22

111

1 MMNr

cr cr c

ξξ

(21.3.59)

where

′′′′

=− =

⋅

−

′

=−

−

12 1 2

11 22

12 11 22

11 22

Mf MkM

lmm

ξξ

(21.3.59')

()

02 00

002

mmc

ξξ

;

(21.3.59'')

denoting, further,

′′′

−−

′

−

11 22 11 22

11 22

mm mm

ΓΓ

ξξ ξξ

ξξ

(21.3.60)

it results the equation

++=

2424

MMN

cr cr c

(21.3.60')

Differentiating with respect to

t and dividing by

(2 / )rc N, we get

⋅⋅⋅

−+−++=

rr rr rr

11 22

23 34

22 2

MM MM

cc rN

NNr NNrNr

rr rr







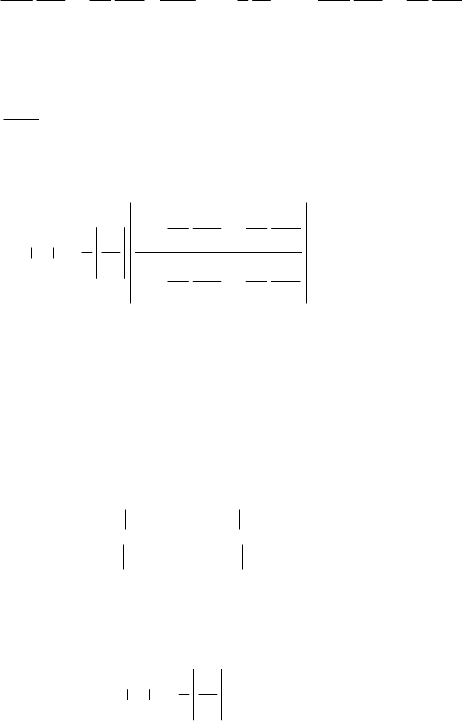
Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

387

⋅

⎛⎞

−− =−+−

⎜⎟

⎝⎠

12 12

34 34

MM MM

crNc

NNr N

rr rr







Observing that

⋅

== =− =−

vr vr

22 11 21

cos( , ) cos( , )wv v

ξξ



(21.3.61)

represents the relative velocity of the particles, the preceding relation leads to

−−







;

(21.3.61')

this constitutes an exact law of mechanics of an isolated system formed by two particles

in inertial motion.

Let be two galaxies, the relative motion of which will be considered in a particle

modelling, by means of the results obtained above. In the hypothesis

+≠

11 22

0mmξξ

and

+≠

0mmξξ, we will consider galaxies for which there exists

<<,0 1KK

so that

+< + < +

12 1 2 12

12 1122 12

0000 00

() (),

() ().

Kc m m m m c m m

Kcmmmm cmm

ξξ

(21.3.62)

Assuming that

≤ (9/10)

vc and supposing that the accelerations =,, 1,2

vjξ



, are

sufficiently small, O. Onicescu finds, with a good approximation, the formula

≅



(21.3.61'')

where the ratio

/NN



does not depend on r , being approximately constant. It results

thus a law which corresponds to Hubble’s law, obtained on an empirical way for

ultragalactic distances.

Considering the energy

H given by (21.3.57'), we observe that the last two terms can be

interpreted as potentials; for usual velocities, the first one is the Newtonian gravitational

potential and the second one is an elastic potential, in that concerns the dependence on

r .

Hence, “between two bodies (two particles) of the Universe are exerted two forces of

inertial interaction: a force of reciprocal attraction (gravitational), the principal part of which

is in inverse proportion to the square of the distance, and an elastic repulsive force, in direct

proportion with the distance, very small (even at distances of planetary order)”. Especially,

to this latter force is due Hubble’s effect mentioned above.

Thus, the invariantive model of mechanics puts in evidence the dilatation of the

Universe, as well as the fact that it is finite. The ratio

/NN



can be positive or

MECHANICAL SYSTEMS, CLASSICAL MODELS

388

negative, so that, during the dilatation can take place also oscillatory phenomena. One

can make many interesting considerations in this order of ideas too.

21.3.3.2 Secular Motion of the Perihelion of Mercury

Let be the Sun and a planet which will be modeled as two particles, by means of the

results in Sect. 21.2.3.3; neglecting the mass of dilatational interaction

ν , we will use

the equation of motion (21.3.49), (21.3.54'), the momenta being given by (21.3.54).

To obtain the equations of motion of the planet with respect to the Sun, we choose a

preferential frame of reference; we consider thus the point of position vector

+++

1122

()()

μμ

ρ .

(21.3.63)

Taking into account the law of conservation of energy

=++

(2)Hcm m μ

= const , the relation (21.3.54) and =−rr r

, it results

++ ++

12 2

12 12

mm mm

μμ



(21.3.63')

Remaining to the terms in

/vc and

/vc and assuming that < r

00 22

/||/mm c



for the majority of the planets, we find

μμ, so that

2mm μ



ρ ;

(21.3.64)

hence, the frame of reference with the origin at the point of position vector

is, in the

limits of the considered approximation, an inertial frame.

Evaluating

⋅⋅ ⋅

−≅− ≅+

⋅

−

vv vv vv

12 12 12

22 2

11, 1

cc c

and taking

μμ, we can write the equation of motion (21.3.49), (21.3.54') in the

form

()()

⋅

⎡⎛ ⎞ ⎤

++= − +

⎜⎟

⎢⎥

⎣⎝ ⎠ ⎦

⋅

⎡⎛ ⎞ ⎤

++=− − +

⎜⎟

⎢⎥

⎣⎝ ⎠ ⎦

200

11212

11 2

2222

200

21212

12 1

2222

d1 1 2

111,

d2 2

d1 1 2

111.

d2 2

rmm

crccr

rmm

crccr











Passing to the above inertial frame, the position vectors

and

verify the relations

Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

389

+++=

+=+

1122

()(),

()();

μμ

μρ μρ



ρρ

(21.3.65)

in this frame, the equations of motion become

()

⎛⎞

⎡⎛ ⎞⎤

++ = + +

⎜⎟

⎢⎥

⎣⎝ ⎠⎦

⎝⎠

⎛⎞

⎡⎛ ⎞⎤

++ =− + +

⎜⎟

⎢⎥

⎣⎝ ⎠⎦

⎝⎠

20 0 0

11 2 1

22 2 2 2

20 0 0

22 1 2

22 2 2 2

d11 1 2

111,

d22 2

d11 1 2

111.

d22 2

fm fm m

ccr r c cr

fm fm m

ccr r c cr



(21.3.66)

Retaining the terms of second order in r

||/c



, after subtracting the equations

(21.3.66) and after some calculations, one obtains

()

⎛⎞

=− − +

⎜⎟

⎝⎠

222

fm m

rccr





(21.3.67)

or, taking into account the admitted approximation,

()

⎛⎞

=− + −

⎜⎟

⎝⎠

222

fm m

rcrc





(21.3.67')

The corresponding motion is, obviously, plane, taking place under the action of the

Newtonian force in the relative motion and of the perturbing force

()

⎛⎞

−−

⎜⎟

⎝⎠

222

fm m

rcrc



(21.3.67'')

The perturbing force being small, one obtains – in a first approximation – an elliptic

orbit. The action of the perturbing force leads to a perturbation in the elliptic motion;

thus, the motion of the perihelion can be studied by the method of the variation of the

constants.

Comparing with the known results concerning the secular motion of the perihelion of

Mercury (the deviation is the greatest for this planet), I. Mihăilă, who has studied the

problem in an invariantive modelling, obtains

()

kfmm

;

(21.3.68)

hence,

()

fm m

λ .

(21.3.68')

MECHANICAL SYSTEMS, CLASSICAL MODELS

390

The mass of gravitational interaction introduced in Sect. 21.3.2.3 will thus have the

expression

()

⎡

⎤

=− +

⎢

⎥

⋅

⎣

⎦

−

fm m

μ .

(21.3.69)

21.4 Formalisms in the Dynamics of Mechanical Systems

In general, there are not searched methods of study for particular dynamical

problems, but methods of study for classes as large as possible of such problems; thus,

Lagrange’s equations and Hamilton’s equations, applicable to the study of the motion

of many discrete and continuous mechanical systems, have been introduced. In this

case, starting from a physical concept, one obtains a final formula, through the agency

of a geometric image of a discrete mechanical system, that is a representative point in a

representative space; a geometric intuition can lead to the choice of a representative

space, the most adequate to the considered problem. Starting from the space

E , one

obtains thus the space

Λ and then space

Γ , hence the configuration space ()q and

the phase space

(, )qp , respectively, where we have put in evidence the generalized

co-ordinates and the generalized momenta. It is convenient, in some cases, to use

formalisms of calculation in spaces with

+ 1s dimensions (the space (,)qt or the

space

(, )pH ) or in spaces with +21s or with +22s dimensions (the space (,, )qtp

or the space

(,, , )qtpH , respectively).

In what follows we present some results concerning these formalisms. As well, we

will give some notions concerning the inverse problem of Newtonian mechanics and the

Birkhoffian formalism (Birkhoff, G.D., 1927; Obădeanu, V., 1987; Sabatier, P.C.,

1978; Synge, J.L., 1960).

21.4.1 Formalisms in Spaces with + 1s Dimensions

A space with + 1s dimensions can be of the form (,)qt or of the form (, )pH ; in

what follows, we put in evidence the connection between these spaces too.

21.4.1.1 Description of the Motion of a Mechanical System in the Space of the

Events

(,)qt

The most simple representative space is – obviously – the space ()q ; however, the

image of all the trajectories is not very simple, because a trajectory which passes

through the representative point

is determined not only by the direction

d , d ,...,d

qq q at this point. If the given forces are conservative (e.g., gravitational

forces), then to a given direction at

P corresponds a simple infinity of trajectories; in

case of non-conservative forces there exists a double infinity of trajectories. In the

space

(,)qt , which we call the space of events, it is easier to put in evidence the totality

Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

391

of the trajectories; indeed, in this space a trajectory which passes through the point

is completely determined by the quantities

d ,d ,...,d

qq q and dt , which specify the

direction. Moreover, this affirmation can be made even if the given forces are not

conservative.

From reasons of homogeneity, it is convenient to denote

== =

, 1,2,..., ,

xqj s x t,

(21.4.1)

in the space

(,)qt . Let be, in this space, a curve C of equations = ()xxu

αα

=+1,2,..., 1sα ; we assume that these functions of class

C are smooth, so that the

derivatives

′

d/dxxt

αα

cannot be simultaneous zero for the same value of u . The

curve

, with a determined sense but without a special parameterization, is a geometric

object which corresponds to a possible motion of the mechanical system.

We introduce a function of Lagrange type

′

≡

12 1

( , ) ( , ,...., ,

xx x x xΛΛ

′′ ′ ′

≡

12 1

, ,..., ) ( , )

xx x x x

αα

Λ (in the space (,)qt we use the Greek indices, which take

values from

+1to 1s

in the corresponding condition of summation), which we

assume to be positive and homogeneous of first degree in the derivatives

′

, so that

∂

′′′

=>=

′

∂

(, ) (, ), 0,xkx k xx k x

ΛΛ Λ

(21.4.2)

the last relation corresponding to Euler’s theorem; in what concerns the smoothness, we

assume the existence and the continuity of any derivative which intervenes. We have

the relation of invariance

′′

(, ) (, )xx xxΛΛ by a change of co-ordinates

′′

→(, ) (, )xx xx

The Lagrangian action on the curve C from =

uu to =

uu is given by

′

∫

() (, )d

Cxxu

ΛA

(21.4.3)

so that ()C

is a functional of the curve C , independent of the parameterization

(due to the homogeneity). The elementary Lagrangian action is

′

=d(,)d (,d)xx u x x

; thus, the space of the events becomes a Finsler space. If

(,d)xxΛ is the square root of a homogeneous quadratic form in differentials, then the

space

(,)qt is a Riemannian space.

Choosing

=ut, we can define Lagrange’s function (;;)qtqL by

12 12 12 12

( , ,..., ; ; , ,..., ) ( , ,..., ; ; , ,..., ,1)

ss ss

qq qtqq q qq qtqq qΛ   L ,

(21.4.4)

the elementary Lagrangian action being

=d(;;)dqtq t

L .

Let us pass, by a synchronous variation

δ , from the curve C to a curve

, so that