Teodorescu P.P. Mechanical Systems, Volume III: Analytical Mechanics

Подождите немного. Документ загружается.

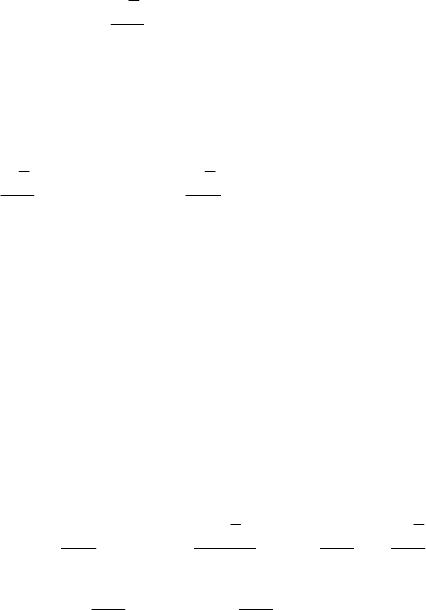
MECHANICAL SYSTEMS, CLASSICAL MODELS

362

transformations (see Sect. 20.2.1.6). The formulae (20.2.33) suggest the introduction of

the new variables

∂

, 1,2,...,

wjs

(21.2.18)

called angle variables. Thus, the canonical transformation is completed.

The new Hamilton function is (21.2.17'), so that we can write the new canonical

equations in the form

∂∂

== =−==

∂∂

( ), 0, 1,2,...,

kkk

wJJ ks



(21.2.19)

Hence, the angle variables are linear functions of time

=+ =, 1,2,...,

kk k

wtk sνγ ,

(21.2.19')

with

=,const

νγ . One can thus show that the action and the angle variables are

canonically conjugate

(

=δ(, )

kjk

Imposing a complete cycle of variation

C to the co-ordinate

q , the other

co-ordinates remaining unchanged, and denoting by

wΔ

the variation of the

co-ordinate

w on this cycle, we can write (without summation)

∂

∂∂∂

== =

∂∂∂∂∂

∂

===δ

∂∂

∫∫ ∫

∫

dd d

jj j

CC C

jj j

wq q q

qqJJq

vv v

hence, only the angle variable

w has a variation on the cycle of variation

(

= 1

wΔ

), the other variables having null variations ( =≠0,

wkjΔ ). If the

period of

w is

T , we have, obviously,

jjj

wTΔν

; hence,

ν are variation

frequencies with respect to

q .

It results that, in case of a libration, for a complete cycle of

q (it returns to the

initial value),

w increases with a unity; the function

q is periodical with respect to

w and we can use Fourier’s formalism of representations. If the motion with respect to

q is a rotation, then

w does no more return to the initial value (for a unitary variation

w ), being non-periodical; the function

−

kkk

qwQ

(

is the increment of

q ),

which allows a Fourier representation, is periodical in this case. As we have seen in the

preceding subsection, if the frequencies are commensurable, then the motion is purely

periodical, the system being degenerated.

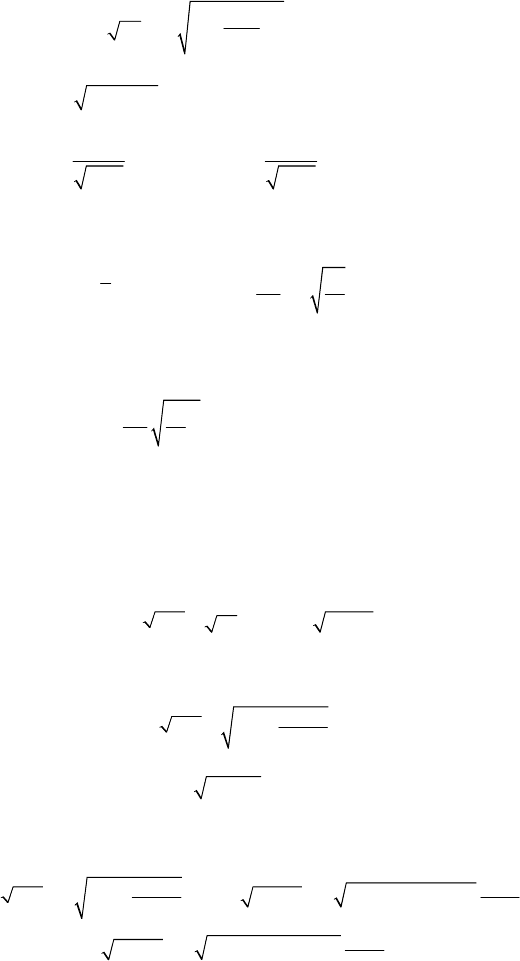
Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

363

21.2.2.2 Applications

In case of the anisotropic linear oscillator, considered in Sect. 21.2.1.1, the relation

(21.2.3') allows to write (without summation)

== − =

∫∫

d 1 d , 1,2,...,

jjjj jj

Jpxa xxj s

(21.2.20)

The substitution

= /sin

jjj

xamkϕ leads to

===

∫

cos d , 1,2,...,

Jjs

mk mk

ϕϕ

Taking into account (21.2.3), (21.2.3'). it results

∑12

( , ,..., )

HJ J J J

so that

, 1,2,...,

(21.2.20')

Let be now the case of a free particle of mass

m , subjected to the action of a

conservative force of potential (19.2.79); assuming that

==() () 0UU

ϕθ and

() /, 0

Ur krk=>, corresponding to a force of Newtonian attraction, we obtain the

generalized momenta (19.2.80'). We may calculate

== =

∫∫

d2 d22Jp ma ma

ϕϕ

ϕϕπ

(21.2.21)

To calculate the action variable

== −

∫∫

d2 d

sin

Jp ma

θθ

(21.2.22)

we consider the equation

sin /aaθ ; if

θ and >

θθ are the first two

solutions of this equation, then it is necessary that

≤≤

θθθ to have J

real. It

results

=−= −

=−

∫∫

∫

22 d 22 sin sin

sin

22 cos cos .

sin

Jma ma

θθ

θθθ

θθ

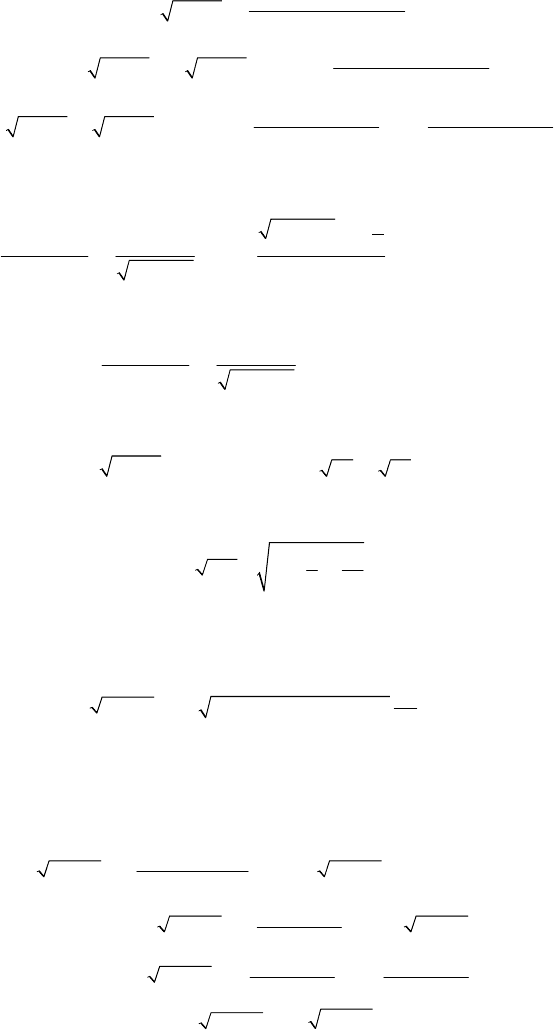
MECHANICAL SYSTEMS, CLASSICAL MODELS

364

The substitution

cos cos cosuθθ leads to

−

=−

−

⎛⎞

=− +

⎜⎟

+−

⎝⎠

∫

∫∫

cos sin

22 d

1cos scos

22 22 sin

1 cos s cos

22 2 sin .

1 cos cos 1 cos cos

Jma u

ma ma

ππ

πθ

θθ

Observing that

−

∫

tan

arctan ,

cos

ab x ab

(21.2.23)

we can write

−

∫

cos

ab x

(21.2.23')

In this case

()

=−=−

221

22 (1sin)2Jma aa

πθπ.

(21.2.22')

As well, to calculate the action variable

== +−

∫∫

d2 d

Jprmh r

(21.2.24)

we introduce the apsidal distances

min

r and

max

r (at the pericentre and the apocentre,

respectively), so that

()

=− − −

∫

max

min

max

min

Jmhrrrr

(21.2.25)

Observing that

max

min

(1 cos ), (1 cos ), (1 cos )ra e ur rae urr ae u=− −= + − = − ,

where

a is the semi-major axis of the ellipse, e is the eccentricity and u is the eccentric

anomaly (see Sect. 9.2.1.3), we can write

()

=− = − +

−

−− − = −

−

−− − +

−+

=− −−

∫∫

∫

∫∫

sin

2d2(1cos)d

(1 cos )

(1 ) 2 2 2

1cos

(1 ) 2

1cos 1cos

2211,

ae u

Jmh uamheuu

aeu

ae mh amh

ae mh

eu eu

amh e

ππ

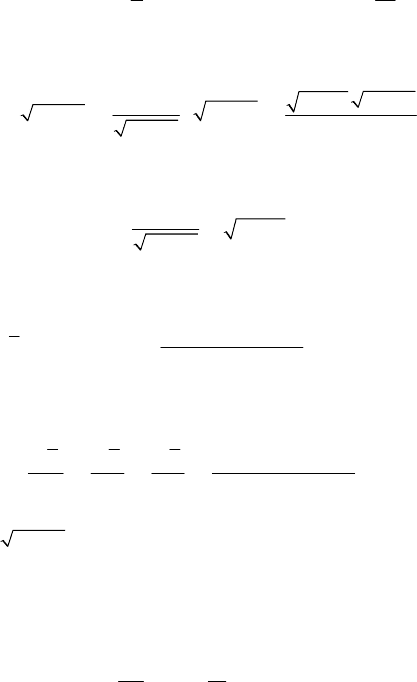
Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

365

where we took into account (21.2.24'). By means of the relations

+ = =− = − =−

max max

min min

2, (1)

rr a rrae

(21.2.26)

we obtain

−

−= −=

−

2,1

ma mh

amh e

so that

()

=−

−

Jma

(21.2.25')

There results, finally,

()

=− =

(,,)

HJ J J h

JJJ

(21.2.27)

The three frequencies are equal, in this case, and we can write

()

∂∂∂

====

∂∂∂

HHH mk

JJJ

(21.2.28)

< 0h

, then −2mh is real and the motion (on an ellipse) is periodical. Eliminating

the sum

JJJ

between (21.2.27) and (21.2.28), taking into account (21.2.26)

and observing that

= 1/Tν , where

is the period, we get Kepler’s third law (see

Sect. 9.2.1.4 too, formula (9.2.17''))

π .

(21.2.29)

21.2.3 Adiabatic Invariance

If the parameters on which depends a mechanical system have a sufficient slow

motion, then the action variables preserve a practically constant value (a property of

adiabatic invariance). In connection to this property, the quantification criteria of

Sommerfeld, as well as the Burgers and the Gibbs–Hertz theorems are presented.

21.2.3.1 Sommerfeld’s Quantification Criteria

In case of a holonomic and scleronomic mechanical system with only one degree of

freedom, the first integral of energy (

==(, ) constHqp h ) determines, in the phase

space

Γ , a closed curve C , without double points, which bounds a domain the area of

which is given by the integral

MECHANICAL SYSTEMS, CLASSICAL MODELS

366

∫

dconstpq

(21.2.30)

If the mechanical system has

s degrees of freedom, then one uses the methods of

calculation foreseen for the quasi-periodic motions (see Sect. 21.2.1.3), when the

variables can be separated. In this case, beside the first integral of energy, can intervene

other

<,mm s, ones ( const, 1,2,...,

pc k m== = ), corresponding to the

apparition of cyclic co-ordinates; we remain with

−sm degrees of freedom, while the

phase space

−2( )sm

will be of canonical co-ordinates

++12

, ,...,

qq q,

++12

, ,...,

pp p. Assuming that one can write the first integral of energy

++ ++

12 1 2 12

( , ,..., , , ,..., , , ,..., ) const

ssm

mm m m

Hq q q p p p c c c h ,

in a closed manifold

−2( )sm

, the integrals

−

++ ++

∫

2( )

12 12

d d ...d d d ...d

mm mm

qq qpp p,

(21.2.31)

are integral invariants, corresponding to the phase volume (see Sect. 21.1.1.3 too).

Let be a separable canonical system for which, beside the first integral of energy,

there are known other

− 1s first integrals. These last first integrals allow us to express

the generalized momenta

−12 1

, ,...,

pp p as functions of

p (we solve a system of

− 1s equations); replacing in H , it results a new Hamiltonian

′

. We obtain thus the

canonical system

′′

∂∂

==−

∂∂

 ,

(21.2.32)

which corresponds to only one degree of freedom, so that one can impose a condition of

the form (21.2.30). Writing such equations for all the other

− 1s generalized momenta,

we obtain – totally –

s conditions of the form (21.2.30), corresponding to the s

degrees of freedom of the mechanical system.

If the mechanical system is an atom with

s degrees of freedom, then the conditions

of the form (21.2.30) which are verified by the action variables will be A. Sommerfeld’s

quantification conditions.

21.2.3.2 Adiabatic Invariants

The integrals of the form (21.2.30), which express quantic conditions are – in fact –

adiabatic invariants. A mechanical system is not, in general, isolated. For instance, an

atom (or a set of atoms) is continuously subjected to external influences: masses,

electric fields, thermic fields, electromagnetic forces, radiation fields etc.; these

influences are exerted, usually, as a continuous, very slow perturbation and can be

represented by parameters which vary slowly in time. Thus, in Hamilton’s function

( , ; , ,..., )

HHqpaa a the adiabatic parameters ( ), 1,2,...,

at k m= , vary

slowly with the time

t , as the adiabatic processes in case of phenomena of

thermodynamic nature; if the qualitative aspect of the mechanical system is not altered,

then the respective process is adiabatic.

Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

367

Let be thus a mechanical system for which Hamilton’s function

= (, ; )HHqpλ

depends on the parameter

= ()tλλ; we assume that the function λ is known, varying

slowly in a time interval

. If we can use action-angle variables (for a constant λ ),

then, in the interval

, the variation of

is in direct proportion to /TΔλ where

Δλ is the variation of the parameter λ ; it can be thus noticed that, if T does not pass

beyond a certain limit, but is sufficiently great, then the variation of

can be made as

small as necessary.

Introducing the action variable (21.2.16), we determine the constants

(;)

aJλ ,

= 1,2,...,ks, and then the function (, ; )SqJλ , which generates the canonical

transformation

→(, ) ( , )qp wJ , by the relations

∂∂

===

∂∂

, , 1,2,...,

pw j s

;

(21.2.33)

the transformation remains canonical if

λ varies in time, Hamilton’s function being

given by

∂

=+= + =

∂

(

)

(;) ,

qqwJ

HHSHJ S S

λλ



(21.2.34)

In this case, the equations of motion will be

∂∂

==+ = +

∂∂∂ ∂

∂

=− =−

∂∂

(;) ,

jjj j

JJJ J

λλ

λν λ λ







(21.2.35)

where

ν are the frequencies of the system with = constλ .

We assume that

λ and



are constant on T (as it was evaluated by Kneser for the

mechanical systems with a single degree of freedom). The variation of the action

J is

thus given by

∂

==−

∂

∫

JJ t

Δλ



(21.2.36)

One can show that the integral which intervenes in (21.2.36) remains bounded how

great could be

; if sup | |λ



is sufficiently small, then

JΔ remains small. We say

that a variable which has this property is called adiabatic invariant; the action variables

have this property.

Thus, P. Ehrenfest notices that the quantities which define “the stable trajectories” in

an atom (the mechanical energy and the action variables of the form (21.2.30)) must be

MECHANICAL SYSTEMS, CLASSICAL MODELS

368

invariant not only by canonical transformations but also by variations imparted to this

mechanical system (supposed to be of Liouville type) by adiabatic processes.

21.2.3.3 The Gibbs–Hertz Theorem. Burger’s Theorem

P. Ehrenfest, in 1916, and then

. Levi-Civita, in 1928, have considered the

problem of adiabatic invariants for canonical systems which do not depend explicitly on

time, but in which the time appears through the agency of a parameter

()at , which

varies very slowly with

t ( = (, ;())HHqpat). In connection to these systems, one

can state

Theorem 21.2.1 (Gibbs–Hertz). If the mechanical system is quasi-ergodic, existing

only a single uniform first integral, then the volume

−21s

V bounded in the phase space

Γ by the manifold ===(, ;()) constHHqpat h is an adiabatic invariant.

We notice that this one is, in fact, the only adiabatic invariant in the mentioned

conditions. In this case, each trajectory in

−21s

V passes as much as possible near to each

point

−21s

V .

As well, we can state

Theorem 21.2.2 (Burgers). In case of a quasi-periodic system of Stäckel type, with

degrees of freedom, the

s cyclic integrals of the form (21.2.30) of Sommerfeld are

adiabatic invariants.

Between these two limit cases is situated the intermediary case in which, besides the

first integral of energy, there exist still

<,mm s, uniform and independent first

integrals; such systems have been called by Levi-Civita primitive systems of order

m .

These theorems have interesting applications both in celestial mechanics and in

atomic mechanics; as well, they can be used in problems concerning mechanical

systems of variable mass.

21.3 Methods of Exterior Differential Calculus. Elements of

Invariantive Mechanics

After applying some methods of exterior differential calculus to mechanical systems,

one passes – in this order of ideas – to the presentation of some elements of invariantive

mechanics; some of the results thus obtained are applied to the study of the motion of a

mechanical system.

The invariantive mechanics has been elaborated by O. Onicescu. beginning with

1954, by a number of papers; these have been continued by other ones, as well as in

some monographs. We will present in this paragraph the most important results in this

direction, using the expositions made by the author of this mathematical model of

mechanics (Onicescu, O., 1974).

21.3.1 Methods of Exterior Differential Calculus

By introducing the fundamental differential form, one can enounce Cartan’s

principle; this allows a study of the inertial motion of a particle.

Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

369

21.3.1.1 The Fundamental Differential Form. Cartan’s Principle

In Sect. 19.1.1.9 we have introduced the bilinear covariant (19.2.41') of a Pfaff form

ω (the differential form (19.1.40)); if this bilinear covariant is equated to zero, then the

Pfaffian is an exact differential, corresponding to the associate differential system

(19.1.44). The differential system associated to the Pfaff form of Hamilton (19.1.45) is

the system of canonical equations of motion of the representative point in the space

Γ , to which is associated the energetical relation =d/dHtH



. We mention the

integral invariant Poincaré-Cartan (21.2.31), studies in Sect. 21.1.2.4 and obtained by

E. Cartan by extending Poincaré’s integral invariant to contours of non-simultaneous

states.

We consider thus that Pfaff’s form of Hamilton is the fundamental differential form

which allows the setting up of mathematical models of mechanical systems; we write

this differential form in the form (

q is the generalized position vector and p is the

generalized momentum vector)

=⋅ − −pqd( )dTWtω ,

(21.3.1)

making an extension to the case in which the mechanical system is non-natural (the

given forces are not conservative or quasi-conservative, as it has been considered by

Cartan). We introduce the notion of exterior derivative in the sense of Elie Cartan and

calculate (see App., Subsec. 1.2.2)

()()

δδ

= −δ= ⋅δ−−δ−δ⋅−−

=⋅δ−δ⋅− − δ+δ−δ

⎡⎤

=+ −⋅δ−− ⋅δ−−δ

⎢⎥

⎣⎦

pq pq

Qq p

Dd d[ ( )][d( )d]

dd(dd)()d

grad grad (d d ) d ,

TWt TWt

TTTWtt

ωωω

taking into account that

= qp(,)TT and δ=⋅δQqW , where Q is the generalized

force vector.

If the generalized momentum is given by

p grad T ,

(21.3.2)

where



is the generalized velocity vector, then Lagrange’s equations read

⋅δ = + ⋅δ

qQq

(grad )

;

(21.3.3)

replacing in the expression of the calculated differential, we obtain

=⋅δ−⋅δ+⋅δ−−δ

=δ + ⋅δ−δ(⋅)+ ⋅δ− − δ

qqp p

qqppq

D [2grad grad (d d ) ]d

[grad (dd)]d,

TTTWtt

TT TWtt





where we took into account

δ= ⋅δ+ ⋅δ

qpgrad gradTT T. According to the

theorem of kinetic energy (

=ddTW), it results

MECHANICAL SYSTEMS, CLASSICAL MODELS

370

=δ + ⋅δ −δ( ⋅ )+ ⋅δ

qqppqD[ grad ]dTT tω



Taking into account (21.3.2), of canonical equation

=−

q grad T



(21.3.4)

Euler’s relation

⋅=

p grad 2TT (T is a quadratic form in the generalized momenta)

and the relation δ= ⋅δ+ ⋅δ

qqgrad gradTT T



, we obtain

δδδ

=−δ=Dd 0ωωω .

(21.3.5)

As well, starting from (21.3.5), we find again the equations of motion of the

representative point. We can state

Theorem 21.3.1 (E. Cartan). The canonical equations of motion of a mechanical

system and the corresponding theorem of kinetic energy are a consequence of the

relation (21.3.5).

Thus, the relation (21.3.5) can be seen as a principle: Cartan’s principle, the

fundamental differential form being given by (21.3.1).

We can state that these equations are a consequence of the principle according to

which the motion is definite by the property of invariance of the integral

[]

⋅−−δ

∫

pqd( )

TWt,

(21.3.6)

for any

δ . By δ has been denoted the elementary displacement on the curve C , while

d is the displacement on trajectories (from

C to C ), corresponding to the equation of

motion (see Fig. 21.4).

21.3.1.2 Inertial Motion of the Particle

As mathematical model for a mechanical system, a particle

P is characterized, after

O. Onicescu, by two quadrivectors of a space with four dimensions (the space

=×

ETM ):

(i) The quadrivector position-time

rr(,),t being the position vector of components

123

,,xxx in

E ; the frames of reference for space and time are considered to be

inertial (Newtonian).

(ii) The quadrivector momentum-energy

p(; )E , the generalized momentum p

having the components

123

,,ppp, while = ()EEt is a function not yet specified of

invariant

()

== ++p

2 222

123

ppp

(21.3.7)

To describe the motion of the particle

P , one introduces the geometric differential

of the quadrivector

p(; )E , using the exterior derivative of the fundamental

differential form

Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

371

=⋅δ−δprEtω ;

(21.3.8)

we obtain thus

Dd d dd dEt Etωωω

δδ

=−δ=⋅δ−δ⋅−δ+δpr pr

Dd [()dd] dEt Etωα

′

=⋅δ+ −⋅δ−δ

pr p r p ,

(21.3.8')

where we have put in evidence the derivative of the function

()E α with respect to α .

Hence, the components of the geometric differential

pD( ; )E are

d,p

()d dEtα

′

−

and

. We can enounce

Principle 21.3.1 (principle of inertial motion of the particle). The inertial motion of a

particle is definite by equating to zero the geometric differential of the quadrivector

momentum-energy

=pD( ; )E 0 ,

(21.3.9)

hence, by the equations

′′

====⋅=

pp p

pv p

dd d

,() , ()

dddd

tttt

αα00.

(21.3.9')

In an equivalent manner, we can enounce

Principle 21.3.1' The inertial motion of a particle is definite by equating to zero the

exterior derivative of the fundamental form

ω for any δ (equation (21.3.5)).

The last equation (21.3.9') is verified together with the first one, hence

== =pp p

0000

,() ( ),

EEααα

(21.3.10)

the momentum being constant during the inertial motion. Denoting by

′

= 1/ ( )mE

α ,

′

≠() 0E

α , the mass of the particle

, the second equation (21.3.9') shows that the

momentum is given by

=pvm .

(21.3.10')

The fundamental form

ω is thus

()

ω= ⋅δ− δvr

(21.3.11)

containing all the elements of the motion. The space

M being uniform and isotropic,

besides position and velocity, the motion of the particles differs only by mass, so that