Teodorescu P.P. Mechanical Systems, Volume III: Analytical Mechanics

Подождите немного. Документ загружается.

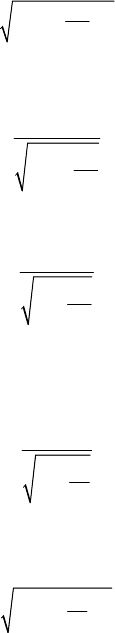
MECHANICAL SYSTEMS, CLASSICAL MODELS

372

the expression

⋅δ − δvr(/)Em t is the same for any particle. We can thus postulate

that the ratio

/Em is a universal constant; this constant being of the nature of a square

of velocity, from a dimensional point of view, we denote

==±

/,1Em εεω

, where

ω is a velocity, so that

=ω

Emε

(21.3.12)

According to the principle (21.3.9'') and taking into account (21.2.8') and (21.3.10),

we can write

′′

⋅δ = ⋅δ = δ =δ

vp pp() () ()EEEαααα; by means of the relations

(21.3.10'), (21.3.12) too, we may write ⋅δ = ⋅δ

mmεω or δ( − =p

222

)0mεω .

Denoting by

m the mass of rest (the mass which corresponds to the velocity

hence to the generalized momentum

0 ), we obtain

−ω =−ω

222 22

mmεε

;

(21.3.13)

hence, it results

(21.3.14)

Taking into account (21.3.10'), the relation (21.2.13) leads to

−

(21.3.15)

Choosing

= 1ε

, we get

−

(21.3.15')

ω being a superior limit velocity (to have a positive quantity under the radical).

Corresponding to the experimental data, we choose

= cω , where c is the velocity of

propagation of light in vacuum; thus, we find again the formula

−

(21.3.15'')

obtained by Einstein in the frame of the relativistic model of mechanics for the

dependence of mass on velocity. The relation (21.2.14) becomes

=+p

(21.3.14')

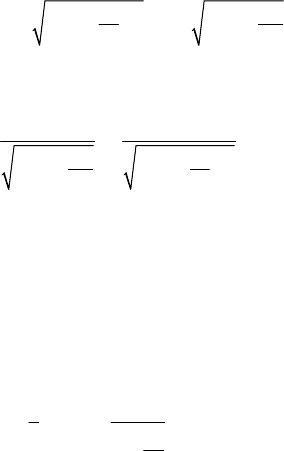
Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

373

and the relation (21.3.12) leads to

=== += +p

222 222

()EE mc cm cm

(21.3.12')

We can also calculate

== ≠

++p

222

() 0

verifying thus the condition previously imposed.

The choice

= 1ε

and = cω puts in evidence the structural characteristic of matter

in motion, hence a relation between matter, space and time; the inertia of matter is thus

closely connected to the structure of space and time.

We notice that

≠ 0m if ≠

0m , the velocity v being constant during the motion,

which is rectilinear and uniform. The energy of the particle is, as well, constant, being

given by (we use the relation (21.3.15'))

== + + <<

−

22 2

,0 | | 1

Emc mc mv

(21.3.12'')

where the prevailing rôle is played by the internal energy

mc , corresponding to the

matter represented by the mass of rest

m .

21.3.2 Elements of Invariantive Mechanics

O. Onicescu called invariantive mechanics the mathematical model of the mechanics

which he set up. In this order of ideas, we present the construction of the

Minkowski-Einstein universe, as well as the possibility to build up new

antiMinkowskian universes for a particle. We will consider also the problem of a

system of two particles.

21.3.2.1 The Minkowski–Einstein Universe

The fundamental form (21.3.1) leads to the inertial fundamental form

=⋅δ−δvr

()

mctω ,

(21.3.16)

the momentum-energy quadrivector being (

,mmc), with the mass given by

(21.3.15'). We may also write

=⋅δ−δrr

()

(d d )/d

mctttω

, putting in evidence the

bilinear form

=δ−⋅δrr

ddcttϕ ,

(21.3.17)

MECHANICAL SYSTEMS, CLASSICAL MODELS

374

which, together with the metrics (a quadratic form)

=−++

123

222 2 2 2

dd(ddd)sct x x x

(21.3.18)

is invariant to Lorentz’s continuous group of linear transformations with ten

parameters; besides, the bilinear form (21.3.17) is a scalar quasi-product for the

pseudoEuclidean metrics (21.3.18). Starting from (21.3.15''), we notice that

−

22 2

so that

δ− ⋅δ

=−

()

ctt

;

hence, the fundamental form

()i

ω is invariant to Lorentz’s group of transformations.

The pseudoEuclidean space

M , endowed with the metrics (21.3.18), is

Minkowski’s space. Starting from the point (

123

,,,xxxt), the motions

(

123

d,d,d,dxxxt) correspond to real motions if −++ >

123

22 2 2 2

d(d d d)0ct x x x ;

the motions for which

123

22 2 2 2

d= d d dct x x x are limit motions. The manifold

−−−=

123

22 2 2 2

0c ξξξξ , in which

123

,,,ξξξξare the direction parameters of the

motion for which

===

123

d/ d / d / d /tx x xξξξ ξ is a hypercone with the

vertex at the point (

123

,,,tx x x

) in the four-dimensional space

123

,,,ξξξξ; it

separates two domains, from which the interior one corresponds to the universe of

motions. We can say that the point

123

(, , , )Ptx x x carries with it the cone which

separates the motions (the luminous cone, on which the motion is effected with the

velocity of propagation of light in vacuum).

The passing from the inertial motion of the particle

to the motion in a field is

realized by characterizing the field by a potential quadrivector, which can be put in

correspondence with the momentum-energy quadrivector of the inertial motion; hence,

besides the formal structure of the law of motion is imposed a structure for the field too.

Let thus be the potential quadrivector

(,), 0,1,2,3

Atj=r , in the space

M , and the

potential differential form

ω =− δ+δ+δ−δ=− ⋅δ−δAr

()

11 22 33

()()

qAxAxAxAt q At,

(21.3.19)

where

is the vector of components

123

,,AAA in

, while

is the electric charge

of the particle

; being a scalar product, this form is an invariant to the transformations

for which the inertial form (21.3.16), hence the form

ω= δ+ δ+ δ− δ

()

11 22 33

mx x mx x mx x mc t 

(21.3.16')

is invariant. We can thus introduce

Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

375

Principle 21.3.2 The geometric derivative of the momentum-energy quadrivector of a

particle is equal to the geometric derivative of the potential quadrivector.

Observing that the geometric derivative (Cartan’s derivative) of the potential

quadrivector has as components the coefficients of the exterior derivative of the

potential form, we also can state

Principle 21.3.2' The motion of a particle in a potential field is definite by the equality

between the exterior derivative of the inertial form and the exterior derivative of the

potential form

δδ δ δ

ω=ω−δω=ω−δω =ω

() () () ( ) ( ) ( )

Dd d D

iiipp p

(21.3.20)

for an arbitrary

δ .

We notice that the exterior derivative of

()p

ω introduces quantities of vector

character, invariant to Lorentz’s group; we have

∂∂

⎡⎛ ⎞ ⎛ ⎞

ω=− + δ− δ+δ

⎜⎟⎜⎟

⎢

∂∂

⎣⎝ ⎠ ⎝ ⎠

∂∂

⎛⎞⎛⎞⎤

−+δ+δ+δ

⎜⎟⎜⎟

⎥

∂∂

⎝⎠⎝⎠⎦

()

Ddd d

dd d,

jjj

qxAtx xAtx

xAtt xAtt



so that

()

∂

⎡⎛ ⎞

ω=− − − δ−δ

⎜⎟

⎢

∂

⎣⎝ ⎠

∂

⎛⎞ ⎤

++ δ−δ

⎜⎟

⎥

∂∂

⎝⎠ ⎦

()

Ddd

dd.

jj j

qAxtxt

xx xx



(21.3.21)

The vectors

=− − =EABA

grad , curlA



(21.3.22)

are thus put in evidence and we are led to the characteristic relations of the field

+= =EB Bcurl , div 00



(21.3.23)

We denote

=+A

divaA



(21.3.24)

and introduce d’Alembert’s operator

∂

=Δ−

∂

;

(21.3.24')

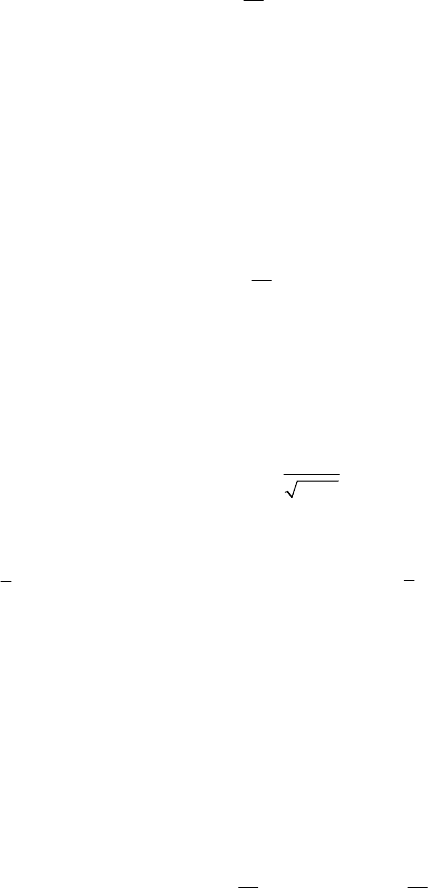
MECHANICAL SYSTEMS, CLASSICAL MODELS

376

taking into account the operator relation

=−curl curl grad div Δ , we can calculate

−= −

=−

BE A

curl grad ,

div .



,

(21.3.25)

Imposing the conditions

== =A

0, 0, 0

aA,,,

(21.3.26)

there results the second group of equations of the stationary Maxwellian field in

vacuum

−= =BE E

curl , div 0.



(21.3.23')

We assume that

is the intensity of the electric field and

is the magnetic induction,

these quantities deriving from the vector quasi-potential

and from the scalar

quasi-potential

A ; these quasi-potentials verify the equations of wave propagation

(21.3.26). We notice also that the velocity of propagation

c is given by

εμ

(21.3.27)

where

ε is the permittivity of vacuum, while

μ is the absolute permeability of

vacuum. We mention also that by replacing the vector

A by the vector

=+AAgradϕ and the scalar

A by the scalar =+

AAϕ , the vectors E and B

are given by (21.3.22) too; the two quasi-potentials which lead to the electromagnetic

field are thus determined excepting an arbitrary function

ϕ , given by its gradient and

by its partial derivative with respect to time (a gauge transformation). As well, the

conditions (21.3.26) remain invariant if = 0

ϕ, .

In case of non-stationary fields, the conditions

==− =−AkA

0, 4 , 4

a ππθ,,

(21.3.28)

where

k is a vector of the field and θ is a scalar of it, lead to the equations

−= =BEjE

curl , div



(21.3.29)

with the notations

(1/4 ) , (1/4 ) / , πμ θ πρ ε==k

being the macroscopic

density of the induction current and

ρ being the macroscopic density of the free

electric charges.

Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

377

Observing that

()

∂

⎛⎞

−δ−δ

⎜⎟

∂∂

⎝⎠

∂

⎛⎞

=− δ δ −δ δ δ −

⎜⎟

∂∂

⎝⎠

∂

∂∂

⎛⎞

=− ∈ ∈ δ − =∈ ∈ δ

⎜⎟

∂∂ ∂

⎝⎠

=∈ δ = δBrr

d(,d,),

jm jn

kn km

mn mn

jkl lmn jkl lmn

lmn

xx xx

xx x

Bx x

we can express the exterior derivative of the form

()p

in the form

=+×⋅δ−⋅δErBrEr

()

D(dd) d

qt q tω .

(21.3.21')

The exterior derivative of the form

()i

ω is given by

=⋅δ−δ−δ⋅+δvr vr

()

Dd()d()()d()d

mmctmmctω ;

observing that

[]

()

−δ ⋅ + δ = − ⋅ δ + δ

=− ⋅δ +δ = δ −δ

⎡

⎤

⎣

⎦

=δ − =−δ =

⎡⎤

⎣⎦

vvv

vv v

22 2 22

()d ( )d [ () ( )]d

() ()d ( ) ()d

()d d0,

mrmct m mct

mmmcmct mc m t

mc v t mc t

where we took into account (21.3.15''), we remain with

=⋅δ−δvr

()

Dd()d()

mmctω

(21.3.30)

The Principle 21.3.2', expressed in the form (21.3.30) leads to

=+× =⋅vErB Er

d( ) ( d d ), d( ) dmqt mcq

where we took into account (21.3.21') and (21.3.30). The first of these equations

represents – in fact – the equation of motion of the particle

P in the form

=+×=vEvBF

() ( )

(21.3.31)

where

F is Lorentz’s generalized force given by (18.1.36); this equation corresponds to

the equation (18.2.60'), obtained for

= constm . The second equation reads

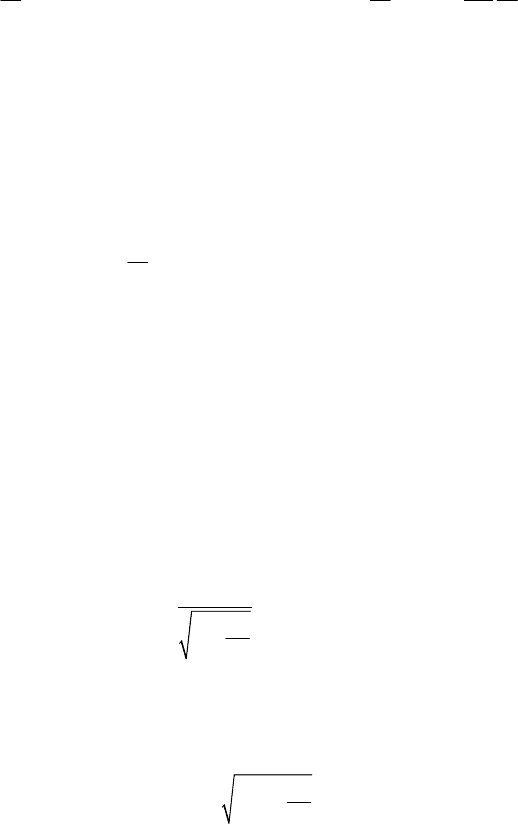
MECHANICAL SYSTEMS, CLASSICAL MODELS

378

=⋅= +×⋅=⋅ =Ev E v B v v v v

2 2

dd1d

( ) ( ) () ()

dd2d

mc q q m m

ttmt

wherefrom

d( )/d d( )/dmc t mv t

−=

d[ ( )]/ d 0mc v t

; taking into

account (21.3.15''), this last form of the equation is verified.

If, in particular,

curl , ==00



AA,

(21.3.32)

then the equation (21.3.31) becomes

==−vE

( ) grad

mq q A

(21.3.31')

corresponding to the mechanical motion of a particle

P subjected to the action of a

quasi-conservative force.

21.3.2.2 AntiMinkowskian Universes

In the preceding subsection we have chosen = 1ε in the relation (21.3.12), which

led us to the Minkowski–Einstein universe, where

= cω is a superior limit velocity.

The second possibility (

=−1ε

) cannot be a priori excluded; there could exist forms of

matter and physical fields for which the velocities could reach values arbitrary great, so

that the universal constant

ω have no more an interpretation of limit velocity. As a

matter of fact, one obtains thus a new mathematical model of universe.

We have

==−

mEm

(21.3.33)

and one must give another interpretation to the velocity ω ; e.g., one can admit that the

velocity

ω is an inferior limit velocity. In exchange, taking into account (21.2.10'), we

can write

=−

;

(21.3.33')

it results that the mass is decreasing by the velocity, the mass of rest being the greatest

one (

≤

). The mass which behaves corresponding to the formulae (21.3.33),

(21.3.33') is called antimass of

ω kind.

The four-dimensional antiMinkowskian (antiEinsteinian) universe associated to this

antimass derives from the inertial form

()

=⋅δ+δvr

()

mtωω,

(21.3.34)

corresponding to the Euclidean metrics

Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

379

=+++

222222

123

dddd dsxxx tω .

(21.3.35)

The structure of the corresponding spaces is, obviously, much more simple from a

geometric point of view; these spaces are homogeneous, differing after the value of

(if the measure of time is definite from a physical point of view).

In this case, the vectors of the two fields are definite in the form

=−=EABA

grad , curlA

ωω



(21.3.36)

the vector quasi-potential and the scalar quasi-potential verifying the equations

=− =Δ+=Δ+ =AAA

000

222

111

div 0, , 0aA AA

ωωω





(21.3.37)

The functions of the stationary field verify the antiMaxwellian equations

ωω ω

+= =

EB B

BE E

curl , div 0,



(21.3.38)

the first group of equations being identical with the Maxwellian one. We can introduce

a non-stationary field too, for which the quasi-potential verify the non-homogeneous

equations

Δ+ =− Δ + =−AA k

4, 4AAππθ

ωω



(21.3.39)

where the vector

k and the scalar θ are given.

Obviously, it remains to be seen to what physical realities can correspond such a

mathematical model of mechanics.

21.3.2.3 The Invariantive Mechanics of a System of Two Particles

To study a system of particles it is necessary to know, besides the motion of a

particle, the motion of a system of two particles too. In the case of an inertial motion

intervene the gravitational interaction (put in evidence by Newton) and the dilatational

interaction (put in evidence by Hubble, together with the considerations of dilatation of

the Universe). These two interactions correspond to the fact that the Euclidean

invariants of a system of particles are distances and angles.

Let be the particles

P and

P of position vectors r

and r

and of momenta p

and

, respectively, the distance between them being given by =−rr r

. The

invariants

===⋅

=⋅ =⋅ =

pppp

rp rp r

1212

,, ,

αα α

ββ β

(21.3.40)

MECHANICAL SYSTEMS, CLASSICAL MODELS

380

allow to consider the function of state

12 12

(,,,,,)HHαααβββ. The inertial form

=⋅δ−δpr

()i

Htω

(21.3.41)

where the summation takes place for the values 1 and 2 of the dummy indices, leads to

=⋅δ−⋅δ−δ+δ=pr rp

()

Ddddd0

jj j j

Ht Htω ,

(21.3.41')

for any

δ , according to the Principle 21.3.1. We notice that

()()

δ = ⋅δ + ⋅δ + ⋅δ + ⋅δ

+ ⋅δ + ⋅δ + ⋅δ + ⋅δ + ⋅δ

pp pp pppp

rr rp p r rp p r

11 22 2112

11 22

()

HH H H

HH H

αα α

ββ β

where

, ,...,HH H

αα

are the partial derivatives with respect to the respective

arguments, while

δ=δ −δrr r

. In this case,

⎛⎞

−−−⋅δ

⎜⎟

⎝⎠

⎛⎞

++ + + ⋅δ

⎜⎟

⎝⎠

⎛⎞

−− − − ⋅δ

⎜⎟

⎝⎠

⎛⎞

+− − − ⋅δ−δ=

⎜⎟

⎝⎠

pprr

pp rp

12 1

12 2

12 1

22 1 2

HHH

HHH t

ββ β

αα

(21.3.42)

Equating to zero the coefficients of the variations

δδδ δrrp p

12 1 2

,, , and δt , we

obtain the equations of motion

=++

=− − −

ppr

HHH

ββ β

(21.3.42')

and the relations which define the momenta

==vr vr

11 22

( d /d , d /d )tt

=++

vppr,

vppr

112

221

HHH

αα

(21.3.42'')

The equations (21.3.42') lead to the conservation of the momentum of a system of two

particles

+=pp

(const)

JJJJG

and to the conservation of the mechanical energy

= constH

. Assuming that

Other Considerations on Analytical Methods in Dynamics of Discrete Mechanical Systems

381

Δ= − ≠

0HH H

αα α

(21.3.43)

we obtain the momenta

−

=−+

ΔΔ Δ

−

=−+

ΔΔ Δ

pvv r

221

112

221

;

HH HH

αα

αββ

αα

αββ

if we denote

===

ΔΔΔ

−−

===

ΔΔ

11 12

1122

,,,

HH HH HH HH

lhlh

αα

αα αα

ββ ββ

νν

(21.3.43')

then it results

=++

pvvr

111 21

222 12

μν

(21.3.44)

where

m and

m are the masses of the particles, μ is a mass of gravitational

interaction, while

ν (to which we give a character of mass) is a mass of dilatational

interaction.

In a Newtonian model, the energy

H is given by

=+− + =

02 02

11 22

const, const

Hmv mvf f

;

(21.3.45)

in an invariantive modelling, we can write

()

=+++

22Hcm m μν,

(21.3.46)

where

H must verify the conditions imposed by the Principle 21.3.2.

If we write the relation (21.3.14') in the form (without summation)

()

=+=

,1,2

mm j

then we get

δ=⋅δvv

()

jj jj

cm m , wherefrom, by means of the relations (21.3.44), it

results

()

δ=⋅δ−−

vpvr

1112

2211

;

mc l