Teodorescu P.P. Mechanical Systems, Classical Models Volume II: Mechanics of Discrete and Continuous Systems

Подождите немного. Документ загружается.

the initial conditions are put in the form

303

(;0) ()xxϑϑ= ,

303

(;0) ()xxϑϑ=



[

]

0,xl∈ .

(12.2.46'')

Thus, the study of the boundary value problems is made as in the preceding cases.

2.2.3 Transverse Vibrations of the Straight Bar

In case of a straight bar subjected to bending and shearing in the plane

Ox x , due to

an external load

0p ≠ , it results

NT M M== = = and we have

221,33

MEIu= , corresponding to the Bernoulli-Euler equation,

311,3

uxu=− and

1,1 2,2

0uu==, corresponding to the hypothesis of plane cross sections of Jacob

Bernoulli (a plane cross section, normal to the bar axis before deformation remains

plane and normal to the deformed axis after application of the external loads; a segment

of a line normal to this axis is not subjected to linear strains); here,

EI is the bending

rigidity of the bar,

I being the moment of inertia with respect to the

Ox -axis (the

neutral axis of the bar). The equation (12.2.41'') becomes

21,3333 1 21,33 1

EI u u I u p

+− =  .

(12.2.47)

The influence of the term

21,33 21,33

(1/ )AIu iu=  , where

i is the gyration radius with

respect to the

Ox -axis, given by a formula of the form (3.1.30'), on the acceleration



is small; the researches of Rayleigh showed that this term is important only for high

frequencies of the vibrations. We will use thus the simplified equation of the transverse

vibrations of the straight bar

1,3333 1

cu u



= ,

(12.2.47')

to which we associate the initial conditions (12.2.23'), together with two other

conditions at the end cross sections of the bar (bilocal conditions). The efforts on an

arbitrary cross section of the bar are given by (the third relation (12.2.41') leads to

2 2 1,333 2 1,3

TEIu iuμ=−, so that we make the same approximation as above)

23 21,33

(;)Mxt EIu= ,

23 21,333

(;)Txt EIu= ,

(12.2.47'')

where

113

(;)uuxt= is the solution of the equation (12.2.47'). If the end cross section

0x =

is hinged, then it results

(0; ) 0ut= ,

221,33

(0; ) (0; ) 0MtEIu t==,

(12.2.48)

if this section is built-in, then we have

(0; ) 0ut= ,

1,3

(0; ) 0ut=

(12.2.48')

123

12 Dynamics of Continuous Mechanical Systems

and, finally, if the respective cross section is free, then we put the conditions

221,33

(0; ) (0; ) 0MtEIu t==

221,333

(0; ) (0; ) 0TtEIu t==

(12.2.48'')

In case of free vibrations of a bar of infinite length one puts only initial conditions of

the form

13 1 3

lim ( ; ) ( )

uxt u x

→+

= ,

13 1,333

lim ( ; ) ( )

uxt cU x

→+

= ,

(12.2.49)

where

c is a constant, while

()ux and

()Ux are given distributions, the

differentiation being effected in the sense of the theory of distributions; the equation of

motion will be

1,3333 1

0cu u+= , 0t > .

(12.2.49')

To can replace the differential equation (12.2.49') by a differential equation in

distributions, as it was shown by W. Kecs and P.P. Teodorescu, we introduce the

generalized displacement (

()tθ is Heaviside’s distribution)

13 13

(;) ()(;)uxt tuxtθ= ,

(12.2.50)

by a prolongation at left with null values; at the initial moment

0t = appears a

discontinuity of the first kind. Using the formula (1.1.51), we can write

13 13

(;) (;)uxt uxt

∂∂



13 13 1 3

(;) (;) ()()uxt uxt ux t

∂∂



13 13 1,333 1 3

( ;) ( ;) ( )() ( )()uxt uxt cU x t u x t

δδ

∂∂

=+ +

∂∂





so that the equation (12.2.49') becomes, in a modified form (we have

/uut=∂ ∂





and

1,3333 1 3

/uux=∂ ∂



for

0t >

200

1,3333 3 1 3 1,33 3 1 3

(;) (;) ()() ()()cu x t u x t cU x t u x tδδ+= +





(12.2.49'')

which contains the initial conditions too, being written in distributions. To find the

solution of the equation (12.2.49''), we apply the Laplace transform, with respect to the

time variable, and the Fourier transform, with respect to the space variable. We obtain

thus

[

]

[

]

[

]

[

]

24 2

313 13

(i )FL ( ;) FL ( ;)cuxtpuxtα−+

[

]

[

]

20 0

313 13

(i ) F ( ) F ( )cUx p uxα=− + ,

wherefrom

124

MECHANICAL SYSTEMS, CLASSICAL MODELS

[][]

[] []

13 1 3 1 3

242 242

FL ( ;) F ( ) F ( )

uxt u x U x

pc pc

αα

=−

Applying the inverse Laplace transform and taking into account formulae of the form

[]

L()cos

θω

[]

L()sinxx

θω

, Re 0p > ,

(12.2.51)

we can write

[

]

[

]

()

[

]

()

0202

13 1 3 3 1 3 3

F(;) F()cos F ()sinuxt u x ct U x ctαα=−.

Applying the inverse Fourier transform to the latter relation, we get

[

]

()

[

]

[

]

()

[

]

10 2 1 0 2

13 1 3 3 1 3 3

(;) F F ()cos F F ()sinuxt u x ct U x ctαα

−−

=−.

Observing that

()

Fcos sin 22 cos

ct ct

πα

⎡⎤

⎢⎥

⎣⎦

()

Fcos sin 22 sin

ct ct

πα

⎡⎤

−=

⎢⎥

⎣⎦

and taking into account the formula (A.3.15) concerning the Fourier transform of a

convolution product, in the case in which one of the factors is a temperate distribution,

while the other factor is a distribution with bounded support, we can write the solution

of the problem in the form

13 1 3

(;) () cos sin

uxt ux

ct ct

⎡

⎛⎞

=∗+

⎜⎟

⎢

⎣

⎝⎠

() cos sin

ct ct

⎛⎞⎤

−∗ −

⎜⎟

⎥

⎝⎠⎦

(12.2.52)

()ux and

()Ux are locally integrable functions, then we have

13 1 3

(;) ( )cos sin

uxt u x

ct ct

ξξ

∞

−∞

⎡

⎛⎞

=−+

⎜⎟

⎢

⎣

⎝⎠

∫

( ) cos sin d

ct ct

ξξ

⎛⎞⎤

−− −

⎜⎟

⎥

⎝⎠⎦

, 0t ≥ ;

(12.2.52')

introducing a new variable

λ by the relation

/4ctλξ= , we find again the solution

given by Boussinesq

125

12 Dynamics of Continuous Mechanical Systems

()

[

022

13 1 3

(;) 2 cos sin

uxt u x ct

λλλ

∞

−∞

=−+

∫

()

]

022

2cossindUx ctλλλλ−− −

, 0t ≥ .

(12.2.52'')

In case of a semi-infinite bar (

[0, )x ∈∞) with homogeneous initial conditions

(

(;0) 0ux = ,

(;0) 0ux =



) and for which the displacement

(0; ) ( )utut= ,

0t >

, has been imposed, the end cross section being free of stresses (

1,33

(0; ) 0ut= ,

0t > ), we find an analogous solution, given also by Boussinesq ( ()ut is a locally

integrable function)

(;) sin cos d

xct

uxt ut

λλ

∞

⎛⎞

=−+

⎜⎟

⎝⎠

∫

, 0t ≥ .

(12.2.53)

To determine the free vibrations of a bar of finite length

l, we assume that

13 3

(;) ()e

uxt Ux

= ,

[

]

0,xl∈

(12.2.54)

the homogeneous equation (12.2.47') leading to the differential equation

,3333

0UUλ−=,

= ;

(12.2.54')

on the same way, using the formula (1.1.51), we can write this equation in distributions

in the form (

333

() ()()Ux x Uxθ= )

,3333 3 3 ,333 3 ,33 ,3 3

() () (0)() (0)()Ux UxU xU xλδδ−= +

,3 ,33 3 ,333 3

(0) ( ) (0) ( )UxUxδδ++.

(12.2.54'')

Applying the Laplace transform and taking into account the formula (A.3.21), we

obtain

[]

3 ,3 ,33 ,333

L ( ) (0) (0) (0) (0)

Ux pU pU pU U

p λ

=+++

−

which leads to

313,323

() (0)( ) (0)( )Ux U f x U f x

λλ

,33 3 3 ,333 3

(0) ( ) (0) ( )Ufx U fx

λλ

++ ,

0x ≥ ,

(12.2.55)

where the functions

126

MECHANICAL SYSTEMS, CLASSICAL MODELS

13 3 3

( ) (cosh cos )

xxxλλλ=+,

23 3 3

( ) (sinh sin )

xxxλλλ=+,

33 3 3

() (cosh cos)

xxxλλλ=−,

333

( ) (sinh sin )

xxxλλλ=−,

(12.2.56)

verify the relations

1,3 3 3

() ()

xfxλλλ= ,

2,3 3 1 3

() ()

xfxλλλ= ,

3,3 3 2 3

() ()

xfxλλλ= ,

333

4,3

() ()

xfxλλλ= .

(12.2.56')

Taking into account (12.1.47''), we notice that

(0)U ,

,33

(0)U and

,333

(0)U ,

hence the quantities which appear in the Cauchy type conditions, are quantities in direct

proportion to the displacement, the rotation, the bending moment and the shearing

force, respectively, at the left end cross section of the bar. As a matter of fact, these

quantities can be easily calculated in any cross section of the bar (

[

]

0,xl∀∈ ) in the

form

,3 3 3 ,3 1 3

() (0)( ) (0)( )Ux U f x U f xλλ λ=+

,33 2 3 ,333 3 3

(0) ( ) (0) ( )Ufx U fx

λλ

++ ,

,33 3 3 3 ,3 3

() (0)( ) (0)( )Ux Ufx U fxλλλ λ=+

,33 1 3 ,333 2 3

(0) ( ) (0) ( )Ufx U fx

λλ

++ ,

,333 3 2 3 ,3 3 3

() (0)( ) (0)( )Ux Ufx U fxλλλ λ=+

,33 3 ,333 1 3

(0) ( ) (0) ( )UfxU fxλλ λ++.

(12.2.55')

Using bilocal conditions of the form (12.2.48 – 12.2.48''), two of the quantities

(0)U ,

,33

(0)U ,

,333

(0)U are equated to zero, while the relations (12.2.55), (12.2.55')

allow to calculate the other two quantities. One can thus obtain the frequency of the

proper vibrations and their corresponding modes. For instance, in case of a simply

supported bar we impose the conditions

,33 ,33

(0) (0) () () 0UU UlUl====,

wherefrom

,3 2 ,333

(0) ( ) (0) ( ) 0Ufl U fl

λλ

,3 ,333 2

(0) ( ) (0) ( ) 0Ufl U fl

λλ

;

this homogeneous system has non-trivial solutions if

() () 0

lflλλ−= leading to

sinh sin 0llλλ= or to

lnλπ= , n ∈ ` . With the notations (12.2.47'), (12.2.54') we

obtain the proper pulsations

= , 1, 2,...n = .

(12.2.57)

127

12 Dynamics of Continuous Mechanical Systems

In this case

[

]

,333 2 ,3 ,3

(0)/ ( )/ ( ) (0) (0)UflflUUλλλ=− =− , so that the modes of

the proper vibrations are sinusoidal

3,3 3 3

() (0)sin sin

nnnn

Ux U x C xλλ

(12.2.57')

where

const

C = for a certain mode of vibration. If the bar is built-in at the left end

cross section and simply supported at the right one (complex problem, the bar being

statically indeterminate), we put the conditions

(0) (0) 0UU==,

,33

() ()

Ul U l=

; we are thus led to the system of homogeneous equations

,33 3 ,333

(0) ( ) (0) ( ) 0Ufl U fl

λλ

+=,

,33 1 ,333 2

(0) ( ) (0) ( ) 0UflU fl

λλ

+=,

which admits non-trivial solutions if

32 1

()() ()() 0

lf l f lf lλλ λλ−=.

We obtain thus the characteristic equation

tanh tanllλλ= with the roots

3.927/lλ = ,

7.069/lλ = ,

10.210/lλ = ,

13.352/lλ = ,

16.493/lλ = ; for

5n > we can use the asymptotic formula (4 1) /4

nlλπ=+ . The proper pulsations

are thus given by

nn n

ωλ λ

== , 1,2,...n = .

(12.2.58)

The modes of vibrations are

333 3

()

() () ()

()

nn n

Ux Cf x f x

λλ

⎡⎤

=−

⎢⎥

⎣⎦

(12.2.58')

where

,33

(0)/

CU λ= for a certain mode of vibration. Other cases of support may be

analogously studied.

Considering also the particular solution of the equation (12.2.47') for

0p ≠

, we

obtain results which put in evidence the forced transverse vibrations of the straight bar.

128

MECHANICAL SYSTEMS, CLASSICAL MODELS

Chapter 13

Other Considerations on Dynamics of Mechanical Systems

In this chapter, we consider firstly the case of motions with discontinuity, putting in

evidence the phenomenon of collision in case of a discrete mechanical system. We deal

then with some problems concerning mechanical systems of variable mass.

13.1 Motions with Discontinuity

Using the results obtained in Chap. 5, Sect. 1.2.6 concerning the acceleration of a

particle, its motion with discontinuity has been considered in Chap. 10, Sect. 1; the

results thus obtained have been then applied to the study of the corresponding

phenomenon of collision. The relations of jump which have been put in evidence with

this occasion, as well as the relations of jump presented in Sect. 11.1.2, concerning the

discrete mechanical systems, are also useful in the study of the phenomenon of

collision. Starting from the case of only one particle, we pass to a finite system of

particles, modelling mathematically the phenomenon of collision and presenting the

corresponding general theorems. We make then a general study of elastic collisions,

while for the plastic collisions we introduce a space of plastic collisions.

13.1.1 Phenomenon of Collision in Case of a Discrete

Mechanical System

After some general considerations, we present various aspects of the phenomenon of

collision in case of a single particle; passing to the case of a discrete mechanical system,

a particular attention is given to the general theorems, including the Carnot and Kelvin

ones, as well as the principle of virtual work.

13.1.1.1 General Considerations

In general, during the motion of a mechanical system, the velocity (hence, the

momentum) of each particle has a continuous variation. If in the evolution of these

quantities appear discontinuities, then we say that the mechanical system is subjected to

a shock; the corresponding mechanical phenomenon is called collision. We mention

thus various cases of simple technique: nail beating, modelling by beating with a

hammer etc. or forging, pressing, boring, riveting, beating a pile with a drop hammer

etc., as well as the collision of a car with a wall (the velocity decreases from a finite

value to zero in a very short time). The phenomenon of collision appears also by

applying suddenly a rigid link upon a mechanical system in motion (the velocities and

the momenta have a sudden variation); but a sudden loss of a link does not lead to

129

P.P. Teodorescu, Mechanical Systems, Classical Models,

MECHANICAL SYSTEMS, CLASSICAL MODELS

130

collision, because the velocities and the momenta have a continuous variation. As well,

the sudden rigid linking of two mechanical systems, one of them being in motion and

the other at rest (the clutch of two mechanisms) is a collision; but the sudden separation

of those mechanical systems (the clutch release of two mechanisms) is a continuous

phenomenon from the point of view of velocities and momenta.

In the frame of the collision phenomenon, in a very short but finite interval of time

[

]

′′′

,tt

, called interval of collision, the velocities of the particles of the (discrete or

continuous) mechanical system have finite variations (in magnitude and direction), their

positions remaining practically unchanged. As a matter of fact, a deformation of the

bodies in collision takes place in case of continuous mechanical systems; this

deformation may be permanent (a plastic one) or not (an elastic one). On the other

hand, during the mechanical phenomenon, a growth of heat takes place, but it will be

neglected in what follows. The fundamental problem consists in the determination of

the velocities of the particles of a mechanical system after collision, assuming that the

corresponding velocities before the phenomenon are known.

In the interval of collision, between the bodies (in general, mechanical systems) in

contact are developed forces, the intensity of which increases quickly, reaching very

great values, and then decreases; under the action of these forces, the bodies are

deformed and we distinguish a compression phase and a relaxation phase, so that the

model of rigid solid is no more sufficient. It is extremely difficult to establish

theoretically or to determine experimentally the variation law of these forces in time.

We are thus led to the notion of percussion, as it was defined in Chap. 10, Sect. 1.2.3,

starting from the notions of generalized force and impulse of the generalized force.

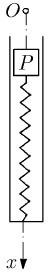
Fig. 13.1 Collision of a heavy body with an elastic spring

(model of a collision phenomenon)

We illustrate the above affirmations, together with R. Voinaroski, considering the

falling along the vertical of a heavy body (modelled as a particle P of mass m), which

comes in contact with a spring vertically guided; the spring is deformed till a position

for which the velocity vanishes and, due to its relaxation, the body is launched up, and

then is separated from the spring. The equation of motion of the particle P in contact

with the spring is

=−mx mg kx



, where > 0k is the elastic constant of the spring,

13 Other Considerations on Dynamics of Mechanical Systems

131

the Ox-axis being directed along the descendent vertical (Fig. 13.1). At the initial

moment

= 0t we impose the conditions =(0) 0x , ==

(0) 2xv gh



, h being the

height of free falling of the particle

P. Denoting =

/kmω , > 0ω , we obtain

=−+

() cos( )

xt a tωϕ

, =− −() sin( )vt a tωϕ,

(13.1.1)

where

222

agvω

⎛⎞

=−

⎜⎟

⎝⎠

arc tan

ϕ ;

(13.1.1')

the spring is compressed in the time interval beginning with

=−

arctan( / )/tvgωω

till

() 0xt



, and then it is relaxed till the moment

Δ=

2tt

for which Δ=−

()vt v,

the jump of the velocity being

Δ= − Δ =

(0) ( ) 2vv vt v. The maximal variation of the

position of the contact point between the body and the spring is given by

()

Δ= = + +

222 2

() /xxt g g vωω. Let be a helical cylindrical spring of elastic

constant

=⋅

310 daN/mk and a particle of mass = 10 kgm , which falls from 1 m

height; it results

−

=⋅

242

310 sω , ≅

4.429 m/sv (we have taken =

9.81 m/sg ),

Δ≅8.858 m/sv ,

−

≅⋅

9.142 10 st ,

−

Δ≅ ⋅

1.829 10 st ,

Δ≅0.026 mx

Δ=Δ= ⋅ = ⋅88.58 kg m/s 8.858 daN sHmv . The maximal intensity of the force by

which the spring acts upon the particle

P is given by =Δ≅

max

780 daNFkx , hence

much greater than the weight

≅ 9.81 daNmg of it. The percussions given by each of

these forces will be

=+ =Δ+Δ

∫

2()d2 2

kx t t mv mgt m v mg t

≅+ ⋅= ⋅= ⋅(88.58 1.79) kg m/s 90.37 kg m/s 9.037 daN s ,

==Δ= ⋅= ⋅

∫

2 d 2 1.79 kg m/s 0.179 daN s

mg t mgt mg t .

The above case can be considered to be a first modelling of a collision, in which some

aspects of this phenomenon (e.g., the mass of the spring, the variation of the unknown

intensity of the percussive force, modelled as an elastic force, the deformation of the

body modelled as a particle etc.) have been neglected; we mention also that the moment

of detachment of the particle from the spring (in calculation has been considered a

relaxation till the moment Δ

t) has not been specified. As well, replacing the spring by a

damper or by a model formed by a spring and a damper (in parallel or in series), we can

obtain a model closer to the reality for the considered mechanical phenomenon. But we

can obtain thus useful conclusions to set up a mathematical model with a general

character for this phenomenon. We notice thus that

≅0.179/9.037 0.020, hence the

percussion due to usual forces (which act permanently) may be neglected with respect

MECHANICAL SYSTEMS, CLASSICAL MODELS

to the percussion due to the forces which appear only in the interval of percussion (in

the considered case, the usual forces have a contribution of only

2% in what concerns

the percussion). We notice also that the interval of percussion Δ

t is small with respect to

the time in which the mechanical phenomenon takes place and that the displacement Δ

can be neglected with respect to the dimensions of the mechanical systems which

intervene; indeed, if we assume, e.g., that the body modelled by the particle

P is of

steel, then its volume would have approximately

0.001282 m , so that, for any of its

form, it would have dimensions much greater that Δ

x (moreover, the particle modelling

implies the neglecting of the dimensions of the body, so much the more the neglecting

of the displacement Δ

x).

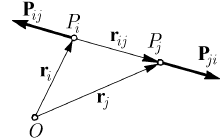
Fig. 13.2 Internal percussions of two particles

The interval of collision is called also interval of percussion. The forces which

appear in the interval of percussion and which have a great intensity (provoking great

variations of the momentum), in a very short interval of time (the percussion interval),

are called percussive forces, the other forces (the weight of bodies, the elastic forces,

the resistance of the air etc.) being usual (non-percussive) forces. Therefore, the

percussive forces as well as the percussion interval are thus difficult to estimate; we can

put better in evidence the mechanical phenomenon with the aid of the percussion

defined by the formula (10.1.40). Thus, the two quantities (a vector and a scalar one), of

very different order of magnitude, are replaced by a vector quantity of a mean order of

magnitude. Because the percussions are obtained starting from the percussive forces,

these ones can be classified analogously. We distinguish thus between given percussive

forces and constraint percussive forces, as well as between external percussive forces

and internal percussive forces. Obviously, in case of a mechanical system, starting from

the internal forces

and F

, which represent the actions of the particles

P and

P ,

respectively, one upon the other, having as support the straight line

PP and being

linked by the relation (1.1.81), we can define the internal percussions

of support

PP (

ij i j

PPλ





, λ scalar) and the internal percussion P

, respectively, both

percussions being linked by the relation (Fig. 13.2)

+=PP 0

ij ji

; (13.1.2)

corresponding to the relation (2.2.50), the above properties of the internal percussions

(either given or constraint ones) can be expressed in a concise form (the pole

O is

arbitrary)

132