Teodorescu P.P. Mechanical Systems, Classical Models Volume II: Mechanics of Discrete and Continuous Systems

Подождите немного. Документ загружается.

{

}

τ=PP 0,

ij ji

(13.1.2')

Before passing to limit in the formula (10.1.40), we can write the relation

corresponding to a mean percussion in the form

′′

′

∫

PF()d

tt;

(13.1.3)

is the mean percussive force in the percussion interval, then we have

′′ ′

=−=ΔPF F()

mm m

tt t.

(13.1.3')

Assuming that, till the moment

[

]

′′′

∈

,ttt takes place a compression phase (in the

interval

[

′

,t t ), a relaxation phase being then developed in the interval

]

′′

,tt , we

can define the corresponding mean percussions

′

∫

()d

tt,

′′

∫

()d

(13.1.4)

with the obvious relation

=+PP P

mcmrm

. (13.1.4')

Passing to limit in the sense of the theory of distributions (

F is a generalized force in the

sense defined in Chap. 10, Sect. 1.1.2), we obtain

′

−→+

∫

lim ( )d

tt,

′′

−→+

∫

lim ( )d

(13.1.5)

with the relation

=+PP P

. (13.1.5')

Together with the generalized force

F(t ) appears Dirac’s distribution too, so that we

can write

=−FP

() ( )tttδ ,

(13.1.6)

justifying thus the denomination of shock given to the respective phenomenon; the

percussive forces

F(t ) are thus temporal distributions.

In case of ideal constraints, the constraint percussions

are normal to the surface

elements of contact, while, in case of constraints with friction, the percussions have not

only a normal component

but also a tangential one P

, collinear with the sliding

velocity and opposite to the latter one; we can assume the Coulombian law

≤PP

Rt Rn

, f being a coefficient of sliding friction. The dimensional equation of

the percussion is

[]

−

FT LMTP .

13 Other Considerations on Dynamics of Mechanical Systems

133

] [

MECHANICAL SYSTEMS, CLASSICAL MODELS

We notice that the phenomenon of collision is characterized by the relative velocity

of two bodies and not by the absolute velocity of each one. If the support of the relative

velocity with which a body strikes another body (the collision line) is normal to the

surface of the latter one, then the collision is normal, while, otherwise, it is oblique (in

fact, the component of the relative velocity along the collision line intervenes); as well,

if the support of this velocity passes through the mass centre, then the collision is

central. For instance, the normal collision of two homogeneous spherical balls is a

central one.

For a mathematical modelling of the collision phenomenon, we make some

hypotheses which, taking into account the above considerations and the simplified

model studied, correspond sufficiently well to the physical reality. We assume thus that:

i) The principles of mechanics are applied in the conditions considered in Chap. 10,

Sect. 1.1.2; especially, the second principle of mechanics is applied in the form

(1.1.89), the differentiation being in the sense of the theory of distributions, using

generalized forces of the form (10.1.5), (10.1.5').

ii) The usual (non-percussive) forces are neglected with respect to the percussive

ones (as it was shown in Chap. 10, Sect. 1.2.3).

iii) It is assumed that, in the interval of percussion, the bodies have not rigid motions

(translation or rotation), but only deformations; the position vectors of the points of

contact are constant in this interval.

It is assumed that, for two given materials, the ratio between the magnitudes of the

normal components of relaxation and compression percussions, respectively, is constant

(13.1.7)

the constant

k being a restitution coefficient (coefficient of elasticity by collision).

Experimentally, it is seen that

<<01k , the magnitude of the normal collision in the

relaxation phase being smaller than the magnitude corresponding to the compression

phase; the respective collision is called elastic-plastic (natural) collision too. In the

ideal case

= 1k we have =

nr nc

PP, the collision being elastic (e.g., for steel, ivory

etc.), while for

= 0k it results = 0

P and we have to do with a plastic collision (the

bodies, e.g., wax, plasticine, clay etc., remain in contact also after the phenomenon of

collision).

13.1.1.2 Collision Phenomenon in Case of a Single Particle

In case of a particle subjected to collision, the corresponding mathematical model is

based on the hypotheses in the preceding subsection and the fundamental equation

which replaces Newton’s one is the jump relation (10.1.41); the Theorem 10.1.11 of the

momentum may be thus considered as a basic principle. Unlike Newton’s equation,

which is a differential equation, the relation (10.1.41) is an algebraic (finite) relation,

which implies the jump of the momentum (in fact, of the velocity) at the theoretic

moment

t of collision and the percussion at that moment. Assuming that the particle is

subjected to constraints too, we can write this relation (or the relation (10.1.43)) in the

form

134

′′ ′

Δ= = −=+

HvvvPP

() ( )

mm ,

(13.1.8)

where

′

is the velocity of the particle before collision,

′′

is the velocity of the same

particle after collision, while

P and P

are the resultants of the percussions

corresponding to the given and constraint forces, respectively, at the moment

t .

Starting from the equation

ddt=rv, taking into account the formula (13.1.8) and

integrating between the limits of the interval of percussion (we use a mean value

formula), we obtain (by

and P

and by P

and P

we mean the percussions in

the compression and relaxation phase, respectively, at a moment

′′ ′′

′′ ′

⎛⎞

′′ ′ ′

−= = + = +

⎜⎟

⎝⎠

∫∫∫∫

rr v v v v

ddd d

tttt

ttt t

()

′′

⎛⎞

⎡⎤

′′ ′ ′

+− =+ + −

⎜⎟

⎢⎥

⎣⎦

⎝⎠

∫

vvPP

d()

Rcm

ttt

()

⎡⎤

′′ ′′

+− + −

⎢⎥

⎣⎦

vPP

()

Rrm

;

we thus see that the displacement at the contact zone (the difference

′′ ′

−

rr) is of the

order of magnitude of the collision time. Passing to limit (

′

−→+

00tt and

′′

−→+

00tt ) and noting that the velocities and the percussions are finite

magnitudes, it results

′′ ′

−→

rr 0

; the hypothesis iii) is thus theoretically justified at

the limit (at the theoretically collision moment).

Analogously, the relation (10.1.42) leads to the relation

()

Δ=×Δ=×+KrHrPP

00 00

() ()

(13.1.8')

corresponding to the theorem of the moment of momentum.

Starting from the relations (10.1.45) and (10.1.45'), we obtain the theorem of kinetic

energy in the form (

/2Tmv

′′ ′ ′′ ′

Δ=−= −

() /2 /2TTTmv mv

)

()

′′

Δ+=+

⋅

PP v

()

TT ,

(13.1.9)

an analogue of the theorem of kinetic energy being given by

()

′

Δ−=+

⋅

PP v

()

TT .

(13.1.9')

In particular, if

()

′′

⋅=

PP v 0

, then we obtain Carnot’s theorem in the same form

(10.1.47), while if

()

′

⋅=

PP v 0

, then an analogue of this theorem is given by

(10.1.47'). As a matter of fact, Carnot’s theorem takes place at the moment of a sudden

apparition of a rigid constraint, corresponding to a plastic collision. As well, starting

from (10.1.48) to (10.1.48'), we can establish Kelvin’s theorem or an analogue of this

theorem, respectively, in the form

13 Other Considerations on Dynamics of Mechanical Systems

135

MECHANICAL SYSTEMS, CLASSICAL MODELS

()

′′′

Δ= + ⋅+

PP v v

() ( )

T ,

(13.1.10)

()

=+⋅PP v

T .

(13.1.10')

We notice that all the relations obtained above are algebraic (finite) relations, which–

obviously – influence (and simplify, in a great measure) the mathematical character of

the considered problems. Thus, in case of a free particle

P, the velocity

′

v before

collision and the percussion

P which appears in the interval of collision are considered

as known; the velocity of the particle after collision will be given, in this case, by the

relation (13.1.8) with

=P0

, in the form

′′ ′

vv P

(13.1.11)

Taking into account (13.1.11), the relations (13.1.10), (13.1.10') have the remarkable

form

′′′

Δ=⋅+ =⋅−

Pv P Pv P

()

(13.1.12)

= P

(13.1.12')

Let us suppose now that the particle

P is subjected to a unilateral holonomic (finite),

rheonomous constraint, of the form

≡≥r

123

(;) ( , , ;) 0

tfxxxt ;

(13.1.13)

hence, it can be on a surface

S or aside it, so as it was shown in Chap. 3, Sect. 2.2.5. We

consider, at the beginning, that the constraint is weak (it is a strict constraint), the

particle having a free motion given by the equation

=rr()t ,

[

)

∈∞0,t ; let

′

be the

smallest positive root of the equation

==rr 0

123

( ( ), ( ), ( ); )xtxtxtt , for which the

particle

P reaches the surface S with the velocity

′

. If the particle moves on the uni-

lateral constraint or leaves it at the moment

t, then its velocity must verify the

condition

≥d/d 0

t or

⋅+≥vgrad 0



(13.1.14)

corresponding to the results in Chap. 3, Sect. 2.2.5; the inequality appears when the

particle leaves the constraint. The condition verified by the velocity

′

v when the

particle reaches the surface

S is

′

=d/d 0

t . Let us suppose now that v

is thus

that

′

<d/d 0

t . To put this relation in concordance with the condition (13.1.14),

we must assume that, in the interval of percussion, appears a constraint percussive

136

force, which leads to a constraint percussion

; thus, arises a jump of the particle

velocity, which – at the end of the percussion interval – will be

′′

v , verifying the

condition (13.1.14). Hence, in the frame of the mathematical model previously set up,

we must have

′

′′

≥

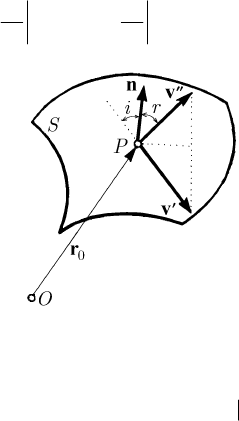
Fig. 13.3 Particle subjected to a unilateral constraint – phenomenon of collision

We can suppose that, at a moment

[

]

′′′

∈

,ttt, the particle reaches the surface S,

where it reaches a velocity

, so that to have

d/d 0

. The velocity

′

v by

which the particle reaches the surface is called incidental velocity, while the velocity

′′

v by which that one leaves it reflected velocity; the angles formed by these velocities

with the external normal to the surface, of unit vector

n, are the incidental angle i and

the reflected angle

r, respectively (Fig. 13.3). In case of ideal constraints, the constraint

percussion

is directed along the unit vector n (along grad f ), so that

=Pn

Rn Rn

The fundamental equation (13.1.8) allows to write

=vn

mP, the variation v

the velocity taking place along the unit vector

n, in its positive sense; it results that both

velocities

′

v and

′′

v are contained in a plane normal to the surface S, their projections

on the tangent plane being equal

′′ ′

sin sinvrvi.

(13.1.15)

The fundamental problem which is put consists in the determination of the reflected

velocity

′′

and of the constraint percussion P

if the incidental velocity

′

and the

position of the particle in the interval of collision (given by the position vector

) are

known. If

′

and

′′

are the constraint percussions corresponding to the first phase

and to the second phase of the collision phenomenon, respectively (in case of a

deformable body, they correspond to the compression phase and to the relaxation phase,

respectively), then we have

′′

−=

vv P

()

m ,

′′ ′′

−=

vv P

()

m ,

(13.1.16)

13 Other Considerations on Dynamics of Mechanical Systems

137

MECHANICAL SYSTEMS, CLASSICAL MODELS

as well as the obvious relation

′′ ′ ′ ′′

−= = +

vv P P P()

Rn Rn Rn

m .

(13.1.16')

The condition

=⋅+=v

d/d grad 0

tff



can be transcribed also in

one of the forms (we take into account the hypothesis iii)

′

⋅+ =v

grad 0



(13.1.17)

′′

⋅+ =v

grad 0



;

(13.1.17')

the unknown quantities are thus given by the equations (13.1.16) or (13.1.16') and

(13.1.17) or (13.1.17').

In the particular case of a plastic collision (the first phase of the collision

phenomenon), the problem to determine the velocity

and the constraint percussion

′

with the aid of the first equation (13.1.16) and of the equation (13.1.17) is put; by

eliminating the velocity

between these equations, one obtains

′′′

===

′

′′

−⋅−=−=⋅=vP

grad grad grad

Rn Rn

tt tt tt

mf mf m f fP



so that

′

=−P

grad

′

=−vv

grad

(13.1.18)

In general, if the second phase of the collision phenomenon takes place, then one

must determine the quantities

′′

and

′′

too, but we dispose only on the second

equation (13.1.16); we must add a supplementary relation of experimental nature to

complete the mathematical model of the collision phenomenon. Newton assumed that

the ratio

′′ ′

Rn Rn

PP does not depend on the incidental velocity but only on the physical

properties of the bodies in collision, so that one may write (corresponding to the

relation (13.1.7))

′′ ′

Rn Rn

PkP,

(13.1.19)

where

k is the restitution (damping) coefficient; in this case, taking into account

(13.1.16'), we obtain

′

=+ =−+PP

(1 ) (1 ) grad

grad

Rn Rn

kk f

(13.1.20)

138

′

′′ ′

=−+vv

(1 ) grad

grad

(13.1.20')

Starting from (13.1.16') to (13.1.17'), we get

′′

′

′′

grad

(13.1.20'')

too, so that, together with (13.1.18), (13.1.19), we are led to the remarkable relation

′′ ′

=−

tt tt

(13.1.21)

If, in particular, the constraint is scleronomic (the surface

S is fixed), then we have

= 0



and the relation (13.1.21) becomes

′′ ′

⋅=− ⋅vvgrad grad

tt tt

or, taking into account Fig. 13.3, has the form (we notice that

()

cos grad cos

′

⋅=−v

)

cos cosvrkvi

′′ ′

= ;

(13.1.21')

if we use also the relation (13.1.15), it results, finally,

cot tan

(13.1.21'')

With the aid of the first formula (13.1.10), where we make

P = 0, we may write (for

the two phases of the motion)

()

′′′

−= ⋅ +Pvv

TT ,

()

′′ ′′ ′′

−= ⋅ +Pvv

TT ,

where

T is the kinetic energy at the moment

t ; taking into account (13.1.17),

(13.1.17'), we can write

()

′

′′

⎛⎞

′′

−= ⋅ + = −

⎜⎟

⎝⎠

grad 2

2 grad 2 grad

Rn Rn

tt tt

TT f f



(13.1.22)

()

′

′′

′′ ′′

⎛⎞

′′ ′′

−= ⋅ + = −

⎜⎟

⎝⎠

grad 2

2 grad 2 grad

Rn Rn

tt tt

TT f f



13 Other Considerations on Dynamics of Mechanical Systems

139

MECHANICAL SYSTEMS, CLASSICAL MODELS

too. If, in the case of scleronomic constraints, we take into account (13.1.18),

(13.1.20''), then we obtain

′

−=−

′′

−=

;

(13.1.22')

using also the relations (13.1.19), (13.1.20), it results

()

−

′′ ′ ′ ′′

Δ=−=− − =−

22 2

111

() 1

221

Rn Rn

TTT kP P

mmk

(13.1.22'')

A relation of the form (13.1.12'), written for a constraint percussion, allows to write

−

Δ+ =

() 0

(13.1.23)

so that we can state

Theorem 13.1.1 (Carnot’s generalized theorem). In the motion of a particle subjected

to collision, due to a holonomic and scleronomic unilateral constraint, the sum of the

variation of the kinetic energy of that particle at the moment of discontinuity and the

kinetic energy of the lost velocity at the same moment, multiplied by the number

−+(1 )/(1 )kk, where k is the restitution coefficient, vanishes.

For

= 1k (elastic collision) we obtain Δ=

() 0T , hence

′′ ′

=TT

, so that a loss

of kinetic energy cannot take place, for

0 < k < 1 (elastic-plastic collision) we have

Δ<

() 0T (because

), hence the variation of the kinetic energy is negative. As

a matter of fact, we can replace the notion of variation of the kinetic energy

(

′′ ′

Δ=−

()TTT) by the loss of kinetic energy (

′′′

Δ=−Δ=−

() ()TTTT

), so

that

Δ>

() 0T (the loss of kinetic energy is positive, hence the kinetic energy

diminishes) and we may write

−

Δ=

()

(13.1.23')

corresponding to the generalized theorem of Carnot. Finally, in the limit case of a

plastic collision (

k = 0), we find again the Theorem 10.1.14 in the form (the loss of

kinetic energy is equal to the kinetic energy of the lost velocities)

Δ=

()TT.

(13.1.23'')

13.1.1.3 Collisions in Case of a Discrete Mechanical System

We consider a discrete mechanical system

S of particles

P , i = 1,2,...,n, in an inertial

frame of reference

R, subjected to the action of percussive and non-percussive, given

and constraint, external and internal forces. Corresponding to the hypotheses i) and ii)

of Sect. 11.1.1.1, we will use Newton’s law and will neglect the non-percussive forces

140

with respect to the percussive ones. Assuming that the time interval

[

]

,tt

′′′

contains

only one moment of discontinuity

t , so that

′′ ′

−<tt

ε , > 0ε arbitrary, and

passing to limit in the sense of the theory of distributions, as in Chap. 10, Sect. 1.2.3,

the relation (11.1.54) allows to write

′′ ′′

′′

′′ ′

−→+

⎡

⎤

Δ= +

⎢

⎥

⎣

⎦

∑

∫∫

HFR

( ) lim ( )d ( )d

tt tt

wherefrom

()

Δ= + =+

∑

HPP

()

(13.1.24)

so that we can state

Theorem 13.1.2 (theorem of momentum). The jump of a momentum of a discrete

mechanical system subjected to constraints, at a moment of discontinuity, is equal to the

resultant of the given and constraint external percussions which act upon that system at

the same moment.

Taking into account (11.1.19), we obtain

Δ=ΔHv

() ( )

M ,

(13.1.24')

so that we can write the relation (13.1.24) also in the form

()

′′ ′

Δ=Δ= − =+v

MMM



ρρρ ,

(13.1.24'')

putting thus in evidence the jump of the velocity of the mass centre and being led to the

Theorem 13.1.2' (theorem of motion of the mass centre). The product of the mass of a

discrete mechanical system subjected to constraints by the jump of the velocity of the

mass centre, at a moment of discontinuity, is equal to the resultant of the given and

constraint external percussions which act upon that system at the same moment.

Hence, the centre of mass of a discrete mechanical system subjected to constraints, at

a moment of discontinuity, moves as a particle at which would be concentrated the

whole mass of the system and which would be acted upon, at that moment, by the

resultant of the given and constraint percussions which act upon that system.

Taking into account the hypothesis iii) in Sect. 11.1.1.1, in conformity to which

=r const





in the interval of percussion, and passing to limit, as in the preceding case,

the relation (11.1.54') allows to write

′′ ′′

′′

′′ ′

−→+

⎡

⎤

Δ= × +×

⎢

⎥

⎣

⎦

∑

∫∫

KrFrR

( ) lim ( )d ( )d

ii i i

tt tt

′′ ′′

′′

′′ ′

−→+

⎡

⎤

=×+

⎢

⎥

⎣

⎦

∑

∫∫

rF R

lim ( )d ( )d

ii i

tt tt

wherefrom

13 Other Considerations on Dynamics of Mechanical Systems

141

MECHANICAL SYSTEMS, CLASSICAL MODELS

()

Δ=×+=+

∑

KrPP

()

ORiOO

MM,

(13.1.25)

so that we can state

Theorem 13.1.3 (theorem of moment of momentum). The jump of the moment of

momentum of a discrete mechanical system subjected to constraints, with respect to a

fixed pole, at a moment of discontinuity, is equal to the resultant moment of the given

and constraint external percussions which act upon that system, with respect to the

same pole, at that moment.

As well, the formula (11.1.54'') leads to

{} {}

{

}

Δτ = τ + τHPP

()

OOORi

(13.1.26)

and we can state

Theorem 13.1.4 (theorem of torsor). The jump of the torsor of a discrete mechanical

system subjected to constraints, with respect to a fixed pole, at a moment of

discontinuity, is equal to the torsor of the given and constraint external percussions

which act upon that system, with respect to the same pole, at that moment.

We notice that the theorem of moment of momentum and the theorem of torsor,

which depend on the fixed pole

O, maintain their form also with respect to another pole

Q, fixed with respect to the frame of reference R. If the pole Q is movable, the

calculation being made with respect to the same frame

R, then we start from the

formula (11.1.23); applying a mean value theorem and observing that under the integral

we have finite quantities, we can write

lim () ()d

ttt

′′

′

′′ ′

−→+

×=

∫

vH 0,

so that

Δ=+K

()

QQQ

MM,

(13.1.25')

the theorems of moment of momentum and of torsor maintaining their form with

respect to the movable pole

Q too.

Analogously, starting from the relations (11.1.66''), (11.1.67'') and (11.1.68'), we can

express the theorems of the dynamic resultant, of the dynamic moment and of the

dynamic torsor in the form

Δ=+A

() RR,

(13.1.27)

Δ=+D

()

OOO

MM,

(13.1.27')

{} {}

{

}

Δτ = τ + τAPP

()

OOORi

(13.1.27'')

respectively.

Let us suppose now that the frame of reference

R of pole O is a non-inertial one,

having a continuous motion (hence, the components

′

v and ω of the finite

142