Stewart J. College Algebra: Concepts and Contexts
Подождите немного. Документ загружается.

T34 ALGEBRA TOOLKIT B
■
Working with Expressions
2
■ Factoring Trinomials
To factor a trinomial of the form , we note that
so we need to choose numbers r and s so that and .rs = cr + s = b
1x + r21x + s2= x
2
+ 1r + s2x + rs
x
2
+ bx + c
example
3
Factoring a Trinomial
Factor .
Solution
We need to find two integers whose product is 12 and whose sum is 7. By trial and
error we find that the two integers are 3 and 4. Thus, the factorization is
Factors of 12
To check that this factorization is correct, we multiply.
✓
■ NOW TRY EXERCISE 17 ■
To factor a trinomial of the form with , we look for factors
of the form and :
Therefore, we try to find numbers p, q, r, and s such that , , and
. If these numbers are all integers, then we will have a limited number
of possibilities for p, q, r, and s.
ps + qr = b
rs = cpq = a
ax
2
+ bx + c = 1px + r21qx + s2= pqx
2
+ 1ps + qr2x + rs
qx + spx + r
a ⫽ 1ax
2
+ bx + c
1x + 3 21x + 4 2= x
2
+ 3x + 4x + 12 = x
2
+ 7x + 12
✓ CHECK
x
2
+ 7x + 12 = 1x + 321x + 42
x
2
+ 7x + 12
example
4
Factoring a Trinomial
Factor .
Solution
We can factor 6 as or , and we can factor as or . By trying
these possibilities, we arrive at the factorization
5
#
1- 12- 5
#
1- 53
#
26
#
1
6x
2
+ 7x - 5
example
2
Factoring Out Common Factors
Factor .
Solution
This expression has two terms, and each term contains the factor . So
Distributive Property
Simplify
■ NOW TRY EXERCISE 15 ■
= 12x - 121x - 32
12x + 421x - 32- 51x - 32= 312x + 42- 5 41x - 3 2
x - 3
12x + 4 21x - 3 2- 51x - 3 2
B.2
■
Factoring Algebraic Expressions T35
example
5
Recognizing the Form of an Expression
Factor each expression.
(a) (b)
Solution
(a) By trial and error we find that
(b) This expression is of the form
where represents . This is the same form as the expression in part
(a), so it will factor as .
■ NOW TRY EXERCISE 29 ■
= 15a - 2215a + 22
15a + 12
2
- 215a + 1 2- 3 = 315a + 12- 34315a + 1 2+ 14
1
ⵧ
- 321
ⵧ
+ 12
5a + 1
ⵧ
ⵧ
2
- 2
ⵧ
- 3
x
2
- 2x - 3 = 1x - 321x + 12
15a + 1 2
2
- 215a + 1 2- 3x
2
- 2x - 3
Factors of 6
Factors of
To check that this factorization is correct, we multiply.
✓
■ NOW TRY EXERCISE 23 ■
13x + 5 212x - 1 2= 6x
2
- 3x + 10x - 5 = 6x
2
+ 7x - 5
✓ CHECK
- 5
6x
2
+ 7x - 5 = 13x + 5212x - 12
2
■ Special Factoring Formulas
Some special algebraic expressions can be factored by using the following formulas.
These are simply Special Product Formulas written backward.
Special Product Formulas
If A and B are any real numbers or algebraic expressions, then
1. Difference of Squares
2. Perfect square
3. Perfect squareA
2
- 2AB + B
2
= 1A - B2
2
A
2
+ 2AB + B
2
= 1A + B2
2
A
2
- B
2
= 1A - B21A + B2
example
6
Factoring Differences of Squares
Factor each polynomial.
(a) (b) 1x + y 2
2
- z
2
4x
2
- 25

T36 ALGEBRA TOOLKIT B
■
Working with Expressions
example
7
Recognizing Perfect Squares
Factor each trinomial.
(a) (b)
Solution
(a) Here and , so . Since the middle term is 6x,
the trinomial is a perfect square. By the Perfect Square Formula we have
(b) Here and , so . Since the middle term is
, the trinomial is a perfect square. By the Perfect Square Formula we have
■ NOW TRY EXERCISES 41 AND 47 ■
When we factor an expression, the result can sometimes be factored further. In
general, we first factor out common factors, then inspect the result to see whether it
can be factored by any of the other methods of this section. We repeat this process
until we have factored the expression completely.
4x
2
- 4xy + y
2
= 12x - y2
2
- 4xy
2AB = 2
#
2x
#
y = 4xyB = yA = 2x
x
2
+ 6x + 9 = 1x + 32
2
2AB = 2
#
x
#
3 = 6xB = 3A = x
4x
2
- 4xy + y
2
x
2
+ 6x + 9
example
8
Factoring an Expression Completely
Factor each expression completely.
(a) (b)
Solution
(a) We first factor out the power of x with the smallest exponent.
Common factor is
Factor as a Difference of Squares
(b) We first factor out the powers of x and y with the smallest exponents.
Common factor is
Factor as a Difference of Squares
Factor as a Difference of Squares
■ NOW TRY EXERCISES 49 AND 53 ■
x
2
- y
2
= xy
2
1x
2
+ y
2
21x + y21x - y2
x
4
- y
4
= xy
2
1x
2
+ y
2
21x
2
- y
2
2
xy
2
x
5
y
2
- xy
6
= xy
2
1x
4
- y
4
2
x
2
- 4 = 2x
2
1x - 2 21x + 2 2
2x
2
2 x
4
- 8x
2
= 2x
2
1x
2
- 42
x
5
y
2
- xy
6
2x
4
- 8x
2
Solution
(a) Using the Difference of Squares Formula with and , we have
(b) We use the Difference of Squares Formula with and .
■ NOW TRY EXERCISES 33 AND 37 ■
1x + y2
2
- z
2
= 1x + y - z21x + y + z2
B = zA = x + y
A
2
- B
2
= 1A - B21A + B 2
4x
2
- 25 = 12x2
2
- 5
2
= 12x - 5212x + 52
B = 5A = 2x

B.2
■
Factoring Algebraic Expressions T37
2
■ Factoring by Grouping
Polynomials with at least four terms can sometimes be factored by grouping terms.
The following example illustrates the idea.
example
9
Factoring by Grouping
Factor each polynomial.
(a) (b)
Solution
(a)
Group terms
Factor out common factors
Factor out from each term
(b)
Group terms
Factor out common factors
Factor out from each term
■ NOW TRY EXERCISES 57 AND 63 ■
x - 2 = 1x
2
- 321x - 22
= x
2
1x - 2 2- 31x - 22
x
3
- 2x
2
- 3x + 6 = 1x
3
- 2x
2
2+ 1- 3x + 6 2
x + 1 = 1x
2
+ 421x + 12
= x
2
1x + 1 2+ 41x + 12
x
3
+ x
2
+ 4x + 4 = 1x
3
+ x
2
2+ 14x + 42
x
3
- 2x
2
- 3x + 6x
3
+ x
2
+ 4x + 4
B.2 Exercises
CONCEPTS
1. Consider the polynomial .
(a) How many terms does this polynomial have?
_______.
(b) List the terms:
_____________.
(c) What factor is common to each term?
_______.
(d) Factor the polynomial:
_____________.
2. To factor the trinomial , we look for two integers whose product is
_______ and whose sum is _______. These integers are _______ and _______, so
the trinomial factors as
_____________.
3. The Special Factoring Formula for the difference of squares is
_____________. So factors as _____________.
4. The Special Factoring Formula for a perfect square is
_____________. So factors as _____________.
5–16
■ Factor out the common factor.
5. 6.
7. 8.
9. 10. 6y
4
- 15y
3
- 2x
3
+ 16x
12x
3
+ 18x30x
3
+ 15x
4
- 3b + 125a - 20
x
2
+ 10x + 25A
2
+ 2AB + B
2
=
4x
2
- 25A
2
- B
2
=
x
2
+ 7x + 10
2x
5
+ 6x
4
+ 4x
3
=
2x
5
+ 6x
4
+ 4x
3
SKILLS

T38 ALGEBRA TOOLKIT B
■
Working with Expressions
11. 12.
13. 14.
15. 16.
17–28 ■ Factor the trinomial.
17. 18.
19. 20.
21. 22.
23. 24.
25. 26.
27. 28.
29–30
■ Factor each expression.
29. (a) (b)
30. (a) (b)
31–40 ■ Use the Difference of Squares formula to factor the expression.
31. 32.
33. 34.
35. 36.
37. 38.
39. 40.
41–48
■ Factor the perfect square.
41. 42.
43. 44.
45. 46.
47. 48.
49–56
■ Factor the expression completely.
49. 50.
51. 52.
53. 54.
55. 56.
57–66
■ Factor the expression by grouping terms.
57. 58.
59. 60.
61. 62.
63. 64.
65. 66. 3s
3
+ 5s
2
- 6s - 102t
3
+ 4t
2
+ t + 2
y
3
- y
2
+ y - 1y
3
- 3y
2
- 4y + 12
x
5
+ x
4
+ x + 1x
3
+ x
2
+ x + 1
- 9u
3
- 3u
2
+ 3u + 12r
3
+ r
2
- 6r - 3
3x
3
- x
2
+ 6x - 2x
3
+ 4x
2
+ x + 4
1x + 12
3
x - 21x + 12
2
x
2
+ 1x + 12x
3
1x - 121x + 2 2
2
- 1x - 12
2
1x + 22
18x
4
y - 2x
2
y
3
x
4
y
3
- x
2
y
5
2x
3
+ 8x
2
+ 8xx
4
+ 2x
3
- 3x
2
x
3
+ 2x
2
+ x3x
3
- 27x
r
2
- 6rs + 9s
2
4w
2
+ 4wy + y
2
25u
2
- 10u + 116z
2
- 24z + 9
t
2
+ 10t + 25t
2
- 6t + 9
y
2
+ 10y + 25x
2
+ 12x + 36
a1 +
1
x
b
2
- a1 -
1
x
b
2
1a + b 2
2
- 1a - b2
2
412x + 12
2
- 91 x + 3 2
2
- 4
4t
2
- 9s
2
49 - 4y
2
4x
2
- 259a
2
- 16
y
2
- 100x
2
- 36
21a + b2
2
+ 51a + b2- 32x
2
+ 5x - 3
13x + 22
2
+ 813x + 22+ 12x
2
+ 8x + 12
8x
2
+ 10x + 39x
2
- 36x - 45
2x
2
+ 7x - 45x
2
- 7x - 6
3x
2
- 16x + 52x
2
- 5x - 7
z
2
+ 6z - 16y
2
- 8y + 15
x
2
- 14x + 48x
2
+ 2x - 15
x
2
- 6x + 5x
2
+ 2x - 3
1z + 22
2
- 51z + 22y1y - 62+ 91y - 62
- 7x
4
y
2
+ 14xy
3
+ 21xy
4
2x
2
y - 6xy
2
+ 3xy
2x
4
+ 4x
3
- 14x
2
5ab - 8abc
B.3
■
Rational Expressions T39
2
B.3 Rational Expressions
■
Simplifying Rational Expressions
■
Multiplying and Dividing Rational Expressions
■
Adding and Subtracting Rational Expressions
■
Rationalizing the Denominator
■
Long Division
A rational expression is a fractional expression in which the numerator and de-
nominator are both polynomials. For example, the following are rational expressions:
In this section we learn how to perform algebraic operations on rational expressions.
2x
x - 1
x
x
2
+ 1
x
3
- x
x
2
- 5x + 6
2
■ Simplifying Rational Expressions
To simplify rational expressions, we factor both the numerator and denominator
and use the following property of fractions.
This allows us to cancel common factors from the numerator and denominator, as in
the case of the following fraction:
6
10
=
3
#
2
5
#
2
=
3
5
AC
BC
=
A
B
C ⫽ 0
example
1
Simplifying Rational Expressions by Canceling
Simplify .
Solution
Factor
Cancel common factors
■ NOW TRY EXERCISE 17 ■
=
x + 1
x + 2
x
2
- 1
x
2
+ x - 2
=
1x - 1 21x + 1 2
1x - 1 21x + 2 2
x
2
- 1
x
2
+ x - 2
T40 ALGEBRA TOOLKIT B
■
Working with Expressions
2
■ Multiplying and Dividing Rational Expressions
To multiply rational expressions, we use the following property of fractions:
This says that to multiply two fractions, we multiply their numerators and denom-
inators.
A
B
#
C
D
=
AC
BD
example
2
Multiplying Rational Expressions
Perform the indicated multiplication and simplify.
Solution
First we factor.
Factor
Multiply fractions
Cancel common factors
■ NOW TRY EXERCISE 23 ■
To divide rational expressions, we use the following property of fractions.
This says that to divide a fraction by another fraction, we invert the divisor and
multiply.
A
B
⫼
C
D
=
A
B
#
D
C
=
31x + 3 2
x + 4
=
31x - 1 21x + 3 21x + 4 2
1x - 121x + 42
2
x
2
+ 2x - 3
x
2
+ 8x + 16
#
3x + 12
x - 1
=
1x - 1 21x + 3 2
1x + 42
2
#
31x + 4 2
x - 1
x
2
+ 2x - 3
x
2
+ 8x + 16
#
3x + 12
x - 1
example
3
Dividing Rational Expressions
Perform the indicated division and simplify:
Solution
To divide these rational expressions, we invert the divisor and multiply.
x - 4
x
2
- 4
⫼
x
2
- 3x - 4
x
2
+ 5x + 6
B.3
■
Rational Expressions T41
■ NOW TRY EXERCISE 27 ■
=
x + 3
1x - 2 21x + 1 2
=
x - 4
1x - 2 21x + 2 2
#
1x + 2 21x + 3 2
1x - 4 21x + 1 2
x - 4
x
2
- 4
⫼
x
2
- 3x - 4
x
2
+ 5x + 6
=
x - 4
x
2
- 4
#
x
2
+ 5x + 6
x
2
- 3x - 4
Invert divisor and
multiply
Factor
Cancel common
factors
2
■ Adding and Subtracting Rational Expressions
To add or subtract rational expressions, we first find a common denominator and
then use the following property of fractions:
Although any common denominator will work, it is best to use the least common
denominator (LCD). The LCD is found by factoring each denominator and then
taking the product of the distinct factors, using the highest power that appears in any
of the factors.
A
C
+
B
C
=
A + B
C
example
4
Adding and Subtracting Rational Expressions
Perform the indicated operations and simplify.
(a)
(b)
Solution
(a) Here the LCD is simply the product .
Write fractions using LCD
Add fractions
Combine terms in numerator
(b) The denominators are and , so the LCD is
.1x - 121x + 1 2
2
1x + 1 2
2
x
2
- 1 = 1x - 121x + 12
=
x
2
+ 2x + 6
1x - 121x + 22
=
3x + 6 + x
2
- x
1x - 1 21x + 2 2
3
x - 1
+
x
x + 2
=
31x + 2 2
1x - 121x + 22
+
x1x - 1 2
1x + 2 21x - 1 2
1x - 1 21x + 2 2
1
x
2
- 1
-
2
1x + 1 2
2
3
x - 1
+
x
x + 2

T42 ALGEBRA TOOLKIT B
■
Working with Expressions
Factor
Combine fractions using LCD
Distributive Property
Combine terms in numerator
■ NOW TRY EXERCISES 35 AND 37 ■
=
3 - x
1x - 1 21x + 1 2
2
=
x + 1 - 2x + 2
1x - 1 21x + 1 2
2
=
1x + 1 2- 21x - 12
1x - 1 21x + 1 2
2
1
x
2
- 1
-
2
1x + 1 2
2
=
1
1x - 1 21x + 1 2
-
2
1x + 1 2
2
If a fraction has a denominator of the form , we may “rationalize the de-
nominator” by multiplying the numerator and denominator by the conjugate radi-
cal .A - B 1C
A + B1C
example
5
Rationalizing a Denominator
Rationalize the denominator: .
Solution
We multiply both the numerator and the denominator by the conjugate radical of
, which is .
■ NOW TRY EXERCISE 41 ■
=
1 - 12
1 - 2
=
1 - 12
- 1
= 12 - 1
=
1 - 12
1 - 1122
2
1
1 + 12
=
1
1 + 12
#
1 - 12
1 - 12
1 - 121 + 12
1
1 + 12
2
■ Long Division
2
■ Rationalizing the Denominator
Multiply numerator and denominator
by the conjugate radical
Product Formula 1:
Simplify
1a + b 21a - b 2= a
2
- b
2
Quotient
Divisor
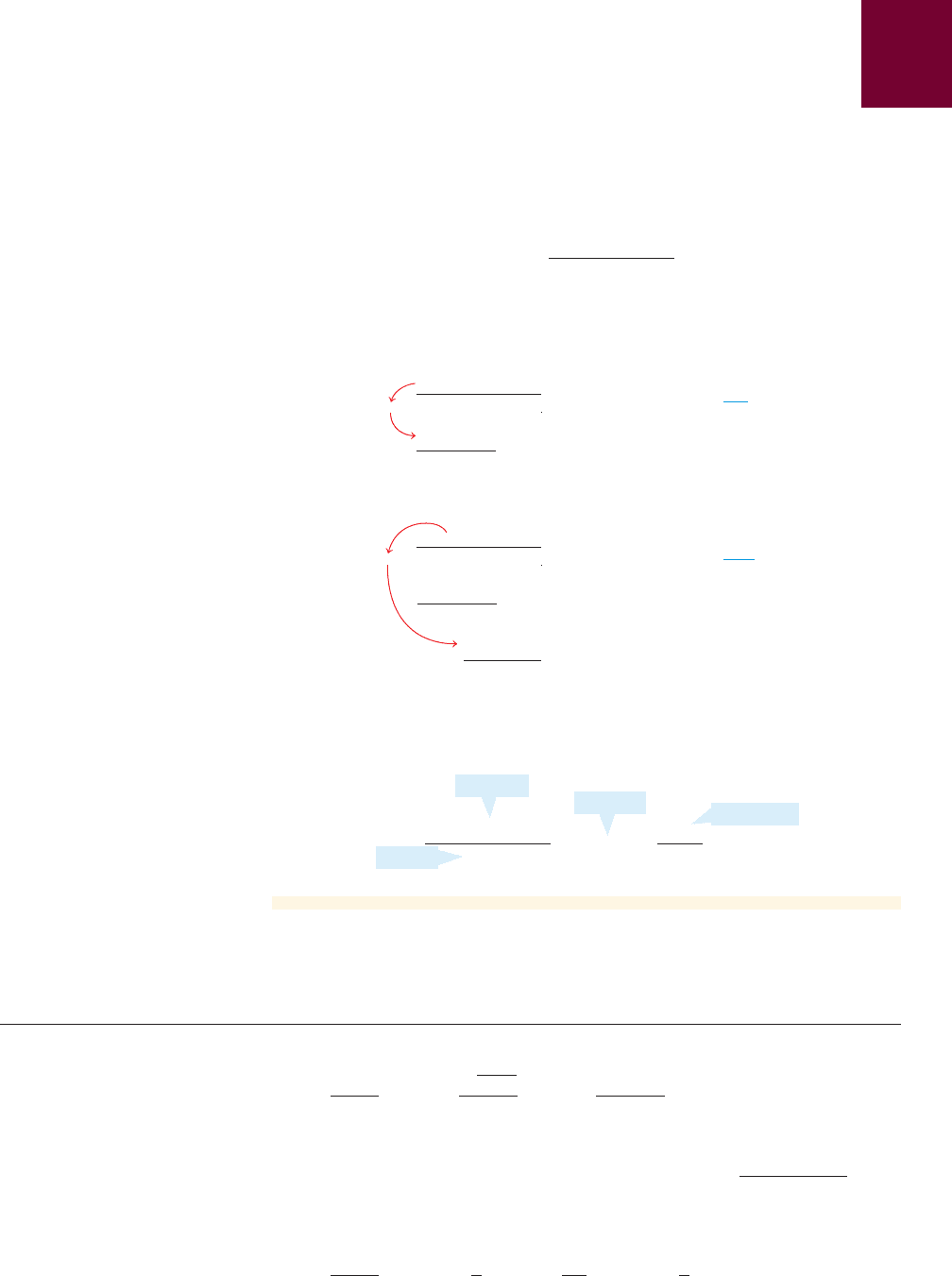
Another way to simplify a rational expression is to perform long division in much
the same way that we divide numbers. When we divide 38 by 7, the quotient is 5 and
the remainder is 3. We write
In the next example we divide rational expressions.
38
7
= 5 +
3
7
Remainder
Dividend
example
6
Long Division of a Rational Expression
Divide by .
Solution
We begin by arranging the expressions as follows.
Next we divide the leading term in the dividend by the leading term in the divisor to
get the first term of the quotient: . Then we multiply the divisor by and
subtract the result from the dividend.
6x
Multiply:
Subtract and “bring down” 12
We repeat the process using the last line as the dividend.
Multiply:
4 Subtract
The division process ends when the last line is of lesser degree than the divisor. The
last line then contains the remainder, and the top line contains the quotient. The re-
sult of the division can be interpreted in the following way:
■ NOW TRY EXERCISE 47 ■
6x
2
- 26x + 12
x - 4
= 6x - 2 +
4
x - 4
- 21x - 42=-2x + 8- 2x + 8
- 2x + 12
6x
2
- 24x
x - 4
冄
6x
2
- 26x + 12
6x - 2
- 2x + 12
- 2x + 12
6x1x - 42= 6x
2
- 24x6x
2
- 24x
x - 4
冄
6x
2
- 26x + 12
6x6x
2
>x = 6x
x - 4
冄
6x
2
- 26x + 12
x - 46x
2
- 26x + 12
B.3
■
Rational Expressions T43
Quotient
Divisor
Dividend
Remainder
B.3 Exercises
CONCEPTS
1. Which of the following are rational expressions?
(a) (b) (c)
2. To simplify a rational expression, we cancel factors that are common to the
_____________ and _____________. So the expression
simplifies to
_______.
3. True or false?
(a) simplifies to (b) simplifies to
3
5
3x
2
5x
2
3
5
x
2
+ 3
x
2
+ 5
1x + 121x + 2 2
1x + 321x + 2 2
x1x
2
- 12
x + 3
1x + 1
2x + 3
3x
x
2
- 1
Divide leading terms:
6x
2
x
= 6x
Divide leading terms:
- 2x
x
=-2
