Stewart J. College Algebra: Concepts and Contexts

Подождите немного. Документ загружается.

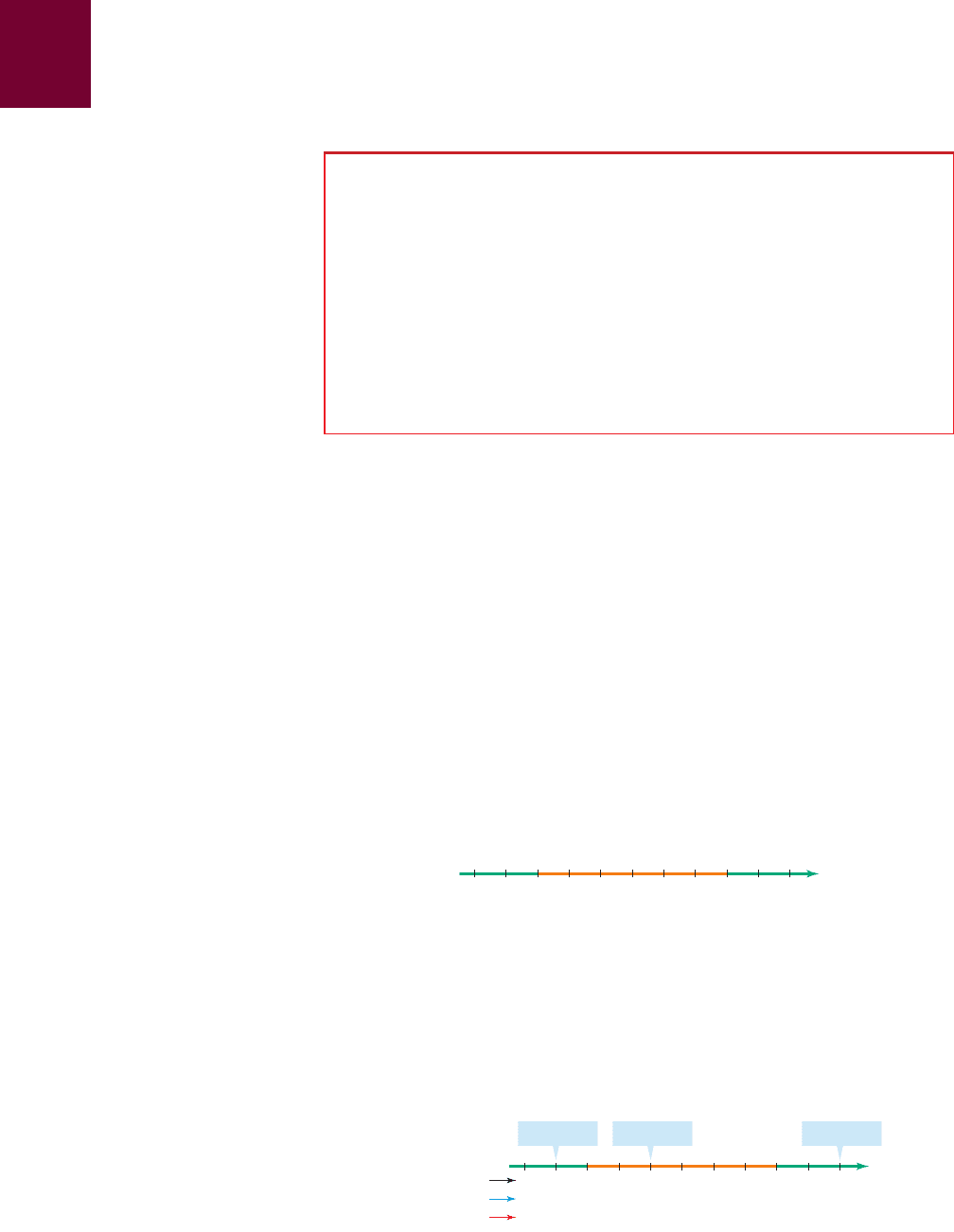
T64 ALGEBRA TOOLKIT C

■

Working with Equations

Solving Nonlinear Inequalities

In the next examples we illustrate the procedure.

1. Move all terms to one side. If necessary, rewrite the inequality so that

all nonzero terms appear on one side of the inequality symbol.

2. Solve the corresponding equation. Solve the equation you get by

replacing the inequality with an equal sign.

3. Find the intervals. The solutions from Step 2 divide the real line into

intervals. Use test values to decide whether the inequality is satisfied on

each interval.

4. Write down the solution. The solution consists of those intervals on

which the inequality is satisfied. Be sure to check the endpoints of each

interval to see whether they also satisfy the inequality.

example

Solving a Nonlinear Inequality Algebraically

Solve the inequality .

Solution

First we solve the equation . We do this by factoring the left-hand

side.

Equation

Factor

Solve

These solutions separate the real line into the three intervals , , and

, as shown in the following diagram.14, q 2

1- 2, 421- q, - 22

x = 4

orx = - 2

1x - 421x + 22= 0

- 2x - 8 = 0

- 2x - 8 … 0

0_2 4

The expression is either positive or negative on each of these intervals.

To decide which it is, we pick a test point in the interval and evaluate the expression.

For example, in the interval , let’s pick the test point . Evaluating the

expression at gives us . Since 7 is greater than 0, the ex-

pression is positive on the interval . In the diagram below we

choose test points in each of the three intervals and determine the sign of the ex-

pression on each interval.

1- q, - 22x

- 2x - 8

1- 32

- 21- 32- 8 = 7- 3

- 31- q, - 22

- 2x - 8

06_2 4_3x

16_87x™-2x-8

+-+Sign

Test pointTest pointTest point

Now that we know the sign of the expression in each interval, we can label the in-

tervals with “⫹” or “⫺” signs as in the following diagram.

SECTION C.3

■

Solving Inequalities T65

From this diagram we see that the solution of the inequality is the

interval . We have included the endpoints of the interval because the in-

equality requires that the expression be less than or equal to 0.

■ NOW TRY EXERCISE 25 ■

3- 2, 44

- 2x - 8 … 0

_2 40

______________________++++++++++++++

example

Solving a Nonlinear Inequality Algebraically

Solve the inequality .

Solution

First we bring all terms to one side of the inequality. Subtracting gives the

inequality

Next we solve the corresponding equation.

Equation

Factor

Solve

These solutions separate the real line into the three intervals

, as shown in the following diagram.and 13, q 2

1- q, - 12, 1- 1, 32,

x = 3

orx =-1

1x - 321x + 12= 0

- 2x - 3 = 0

- 2x - 3 7 0

2x + 3

7 2x + 3

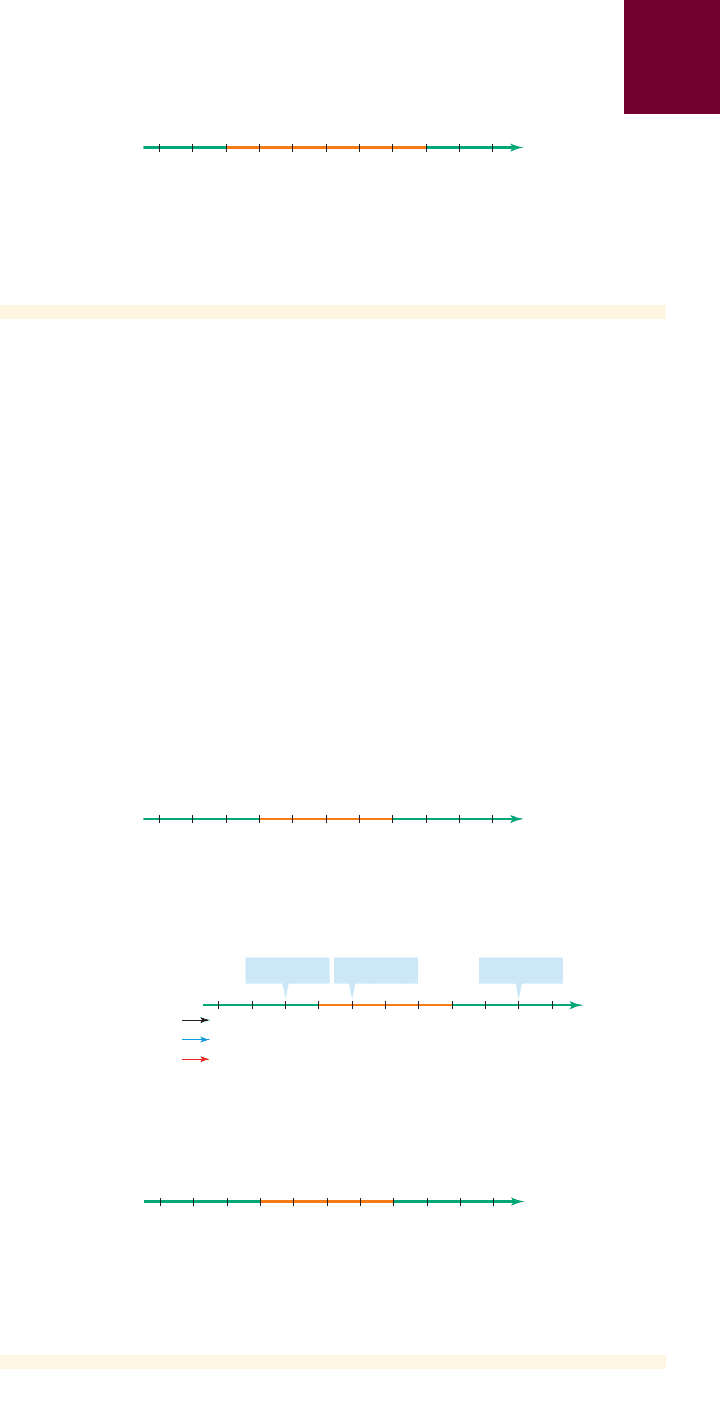
0_1 3

We use test points to determine the sign of the expression on each of

these intervals:

- 2x - 3

Now that we know the sign of the expression in each interval, we can label the in-

tervals with “+” or “-” signs as in the following diagram.

05_1 3_2x

12_35x™-2x-3

+-+Sign

Test pointTest pointTest point

From the diagram we see that the solution of the inequality con-

sists of all x-values in the set . We did not include the endpoints

and 3 because they do not satisfy the inequality.

■ NOW TRY EXERCISE 27 ■

- 1

1- q, - 12´ 13, q 2

- 2x - 3 7 0

_1 30

_______________++++++++++++++++++++

T66 ALGEBRA TOOLKIT C

■

Working with Equations

C.3 Exercises

1. Fill in the blank with an appropriate inequality sign.

(a) If , then

___ 2.

(b) If , then 3x

___ 15.

___ .

(d) If , then

___ 2.

2. True or false?

(a) If , then x and are either both positive or both negative.

(b) If , then x and are each greater than 5.

3–10

■ Let . Determine which elements of S satisfy the

inequality.

3. 4.

5. 6.

7. 8.

9. 10.

11–22

■ Solve the linear inequality. Express the solution using interval notation, and graph

the solution set.

11. 12.

13. 14.

15. 16.

17. 18.

19. 20.

21. 22.

23–34

■ Solve the nonlinear inequality. Express the solution using interval notation, and

graph the solution set.

23. 24.

25. 26.

27. 28.

29. 30.

31. 32.

33. 34. 1x + 3 2

1x + 127 01x - 421x + 22

6 0

1x - 521x - 2 21x + 1 27 01x + 221x - 1 21x - 3 2… 0

Ú 9x

6 4

6 x + 22x

+ x Ú 1

+ 5x + 6 7 0x

- 3x - 18 … 0

1x - 521x + 4 2Ú 01x + 221x - 3 26 0

1 6 3x + 4 … 16- 1 6 2x - 5 6 7

5 … 3x - 4 … 142 … x + 5 6 4

6 - x Ú 2x + 93x + 11 … 6x + 8

5 - 3x …-167 - x Ú 5

3x + 11 6 52x - 5 7 3

- 4x Ú 102x … 7

+ 2 6 4

…

- 2 … 3 - x 6 21 6 2x - 4 … 7

2x - 1 Ú x3 - 2x …

x + 1 6 2x - 3 7 0

S = 5- 2, - 1, 0,

, 1, 12, 2, 46

x + 1x1x + 127 5

x + 1x1x + 127 0

- xx 6-2

- 6- 3xx Ú 2

x … 5

x - 3x 6 5

CONCEPTS

SKILLS

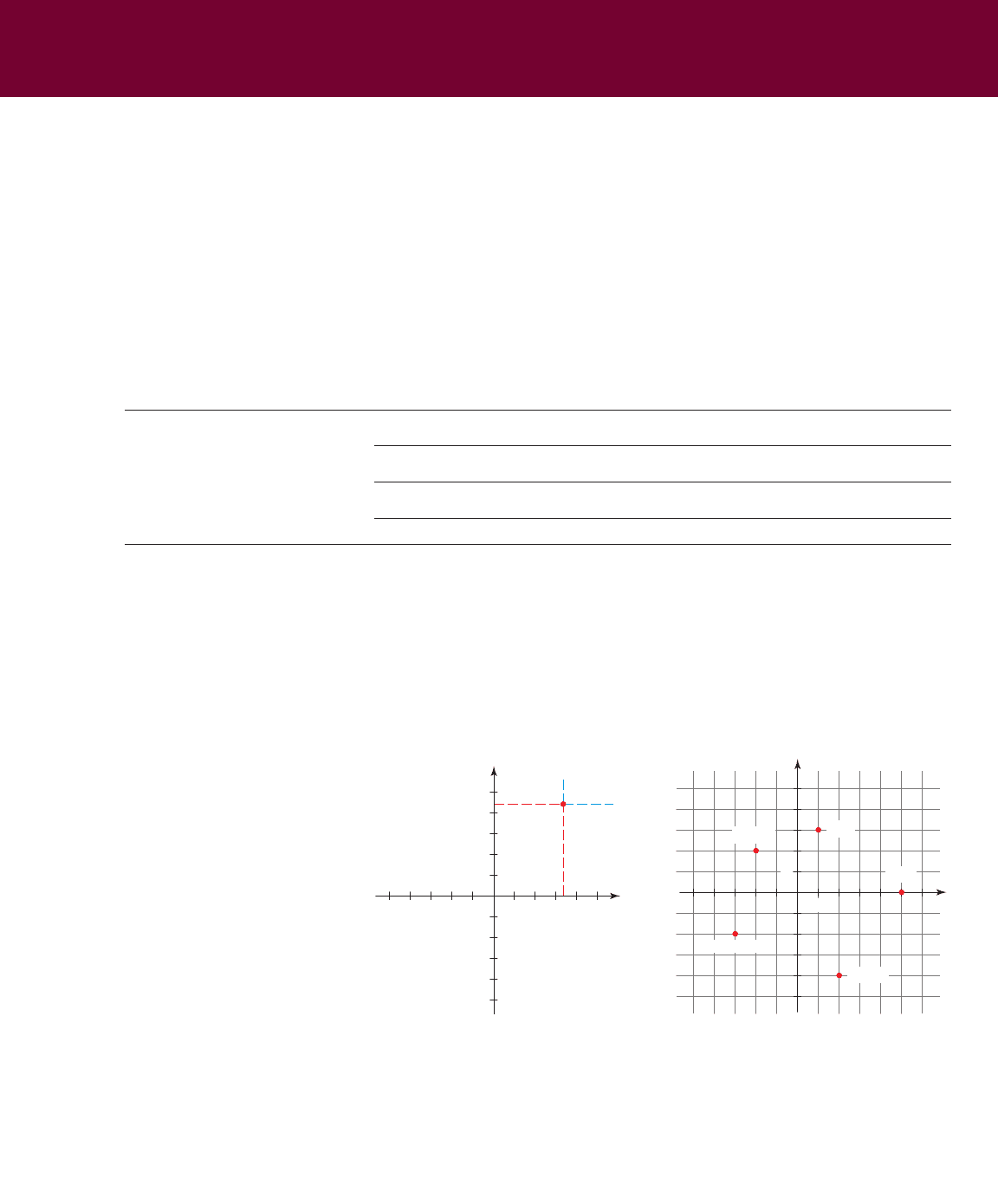
Any point P in the coordinate plane can be located by a unique ordered pair of

numbers (a, b). The first number a is called the x-coordinate of P; the second num-

ber b is called the y-coordinate of P. We can think of the coordinates of P as its “ad-

dress,” because they specify its location in the plane. Several points are labeled with

their coordinates in Figure 2.

T67

Algebra Toolkit D

Working with Graphs

D.1 The Coordinate Plane

D.2 Graphs of Two-Variable Equations

D.3 Using a Graphing Calculator

D.4 Solving Equations and Inequalities Graphically

D.1 The Coordinate Plane

■

The Coordinate Plane

■

The Distance Formula

■

The Midpoint Formula

■ The Coordinate Plane

Just as points on a line can be identified with real numbers to form the coordinate

line, points in a plane can be identified with ordered pairs of numbers to form the

Cartesian plane or the coordinate plane. To do this, we draw two perpendicular

real lines that intersect at 0 on each line. The horizontal line is the x-axis and the ver-

tical line is the y-axis. The point of intersection of the x-axis and the y-axis is the ori-

gin O. The two axes divide the plane into four quadrants, labeled I, II, III, and IV

in Figure 1. (The points on the coordinate axes are not assigned to any quadrant.)

P(a, b)

III

)

(_2, 2)

(5, 0)

(1, 3)

(2, _4)

(_3, _2)

figure 1 figure 2

T68 ALGEBRA TOOLKIT D

■

Working with Graphs

example

Graphing Points and Sets in the Plane

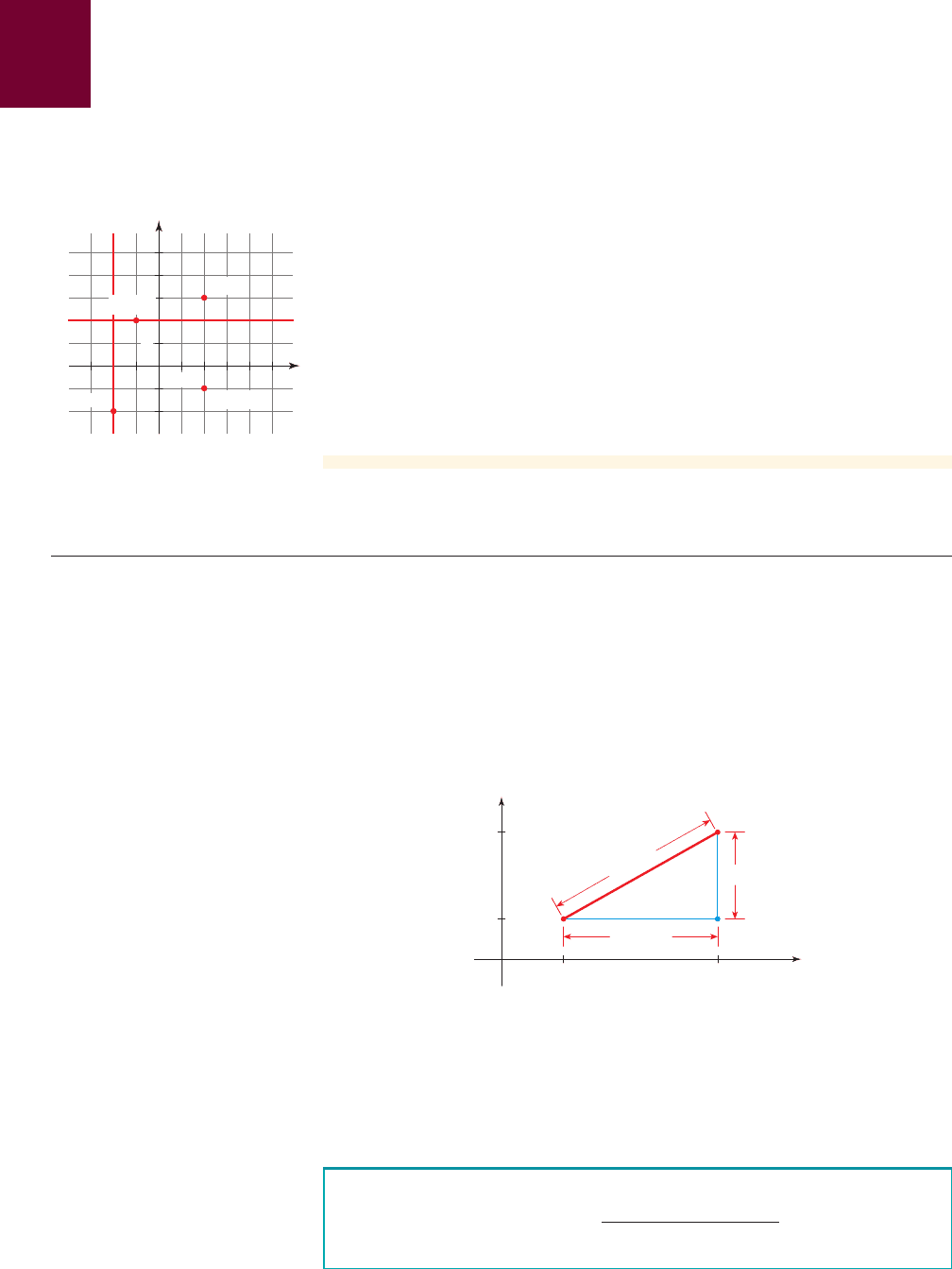
Graph the set of points in the coordinate plane.

(a)

(b)

(c)

Solution

(a) The points are graphed in Figure 3.

(b) This set consists of all points whose y-coordinate is 2. These points together

form a line parallel to the x-axis (see Figure 3).

form a line parallel to the y-axis (see Figure 3).

■ NOW TRY EXERCISES 5 AND 7 ■

- 2

51x, y20 x =-26

51x, y20 y = 26

512, 32, 1- 1, 22, 12, - 1 2, 1- 2, - 226

)(2, 3)

(2, _1)

(_2, _2)

(_1, 2)

figure 3 The coordinate plane

■ The Distance Formula

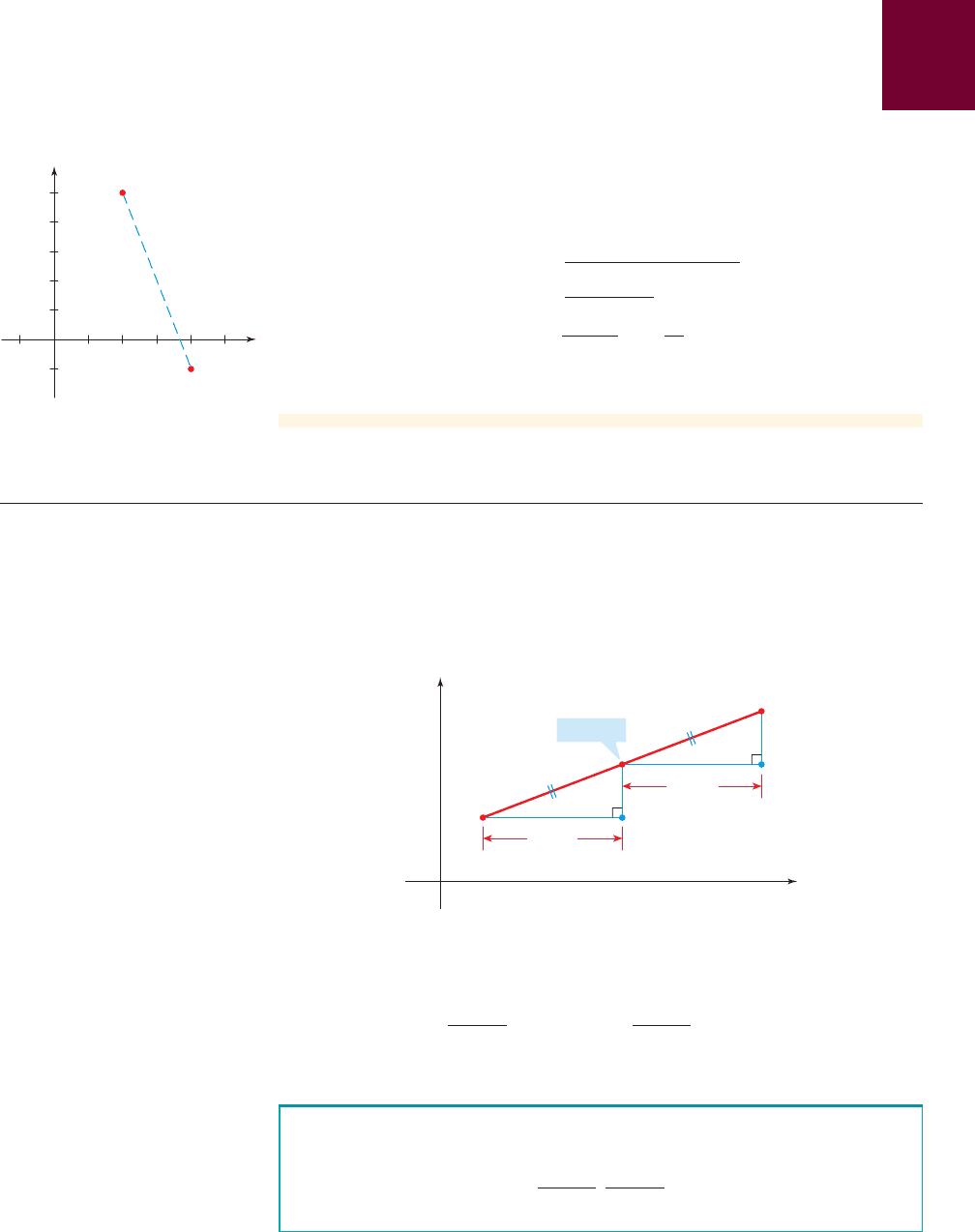
We now find a formula for the distance d(A, B) between two points and

in the plane. Recall from Algebra Toolkit A.2 that the distance between

points a and b on a number line is . So from Figure 4 we see that

the distance between the points and on a horizontal line must be

and that the distance between and on a vertical line must

be .0

- y

C1x

, y

2B1x

, y

20 x

- x

C1x

, y

2A1x

, y

d1a, b 2= 0 b - a 0

B1x

, y

A1x

, y

-x⁄

-y⁄

A(x⁄, y⁄)

B(x

, y

)

d (A, B)

C(x

, y⁄)

x⁄ x

y⁄

figure 4 Distance between A and B

Since triangle ABC is a right triangle, the Pythagorean Theorem gives the fol-

lowing Distance Formula.

The Distance Formula

The distance between the points and in the plane is

d1A, B 2= 21x

- x

+ 1y

- y

B1x

, y

2A1x

, y

SECTION D.1

■

The Coordinate Plane T69

The midpoint of the line segment from to is

+ x

+ y

B1x

, y

2A1x

, y

example

Finding the Distance Between Two Points

Find the distance between the points and .

Solution

Using the Distance Formula, we have

See Figure 5.

■ NOW TRY EXERCISE 15(b) ■

= 14 + 36 = 140 L 6.32

= 22

+ 1- 62

d1A, B 2= 214 - 22

+ 1- 1 - 52

B14, - 12A12, 52

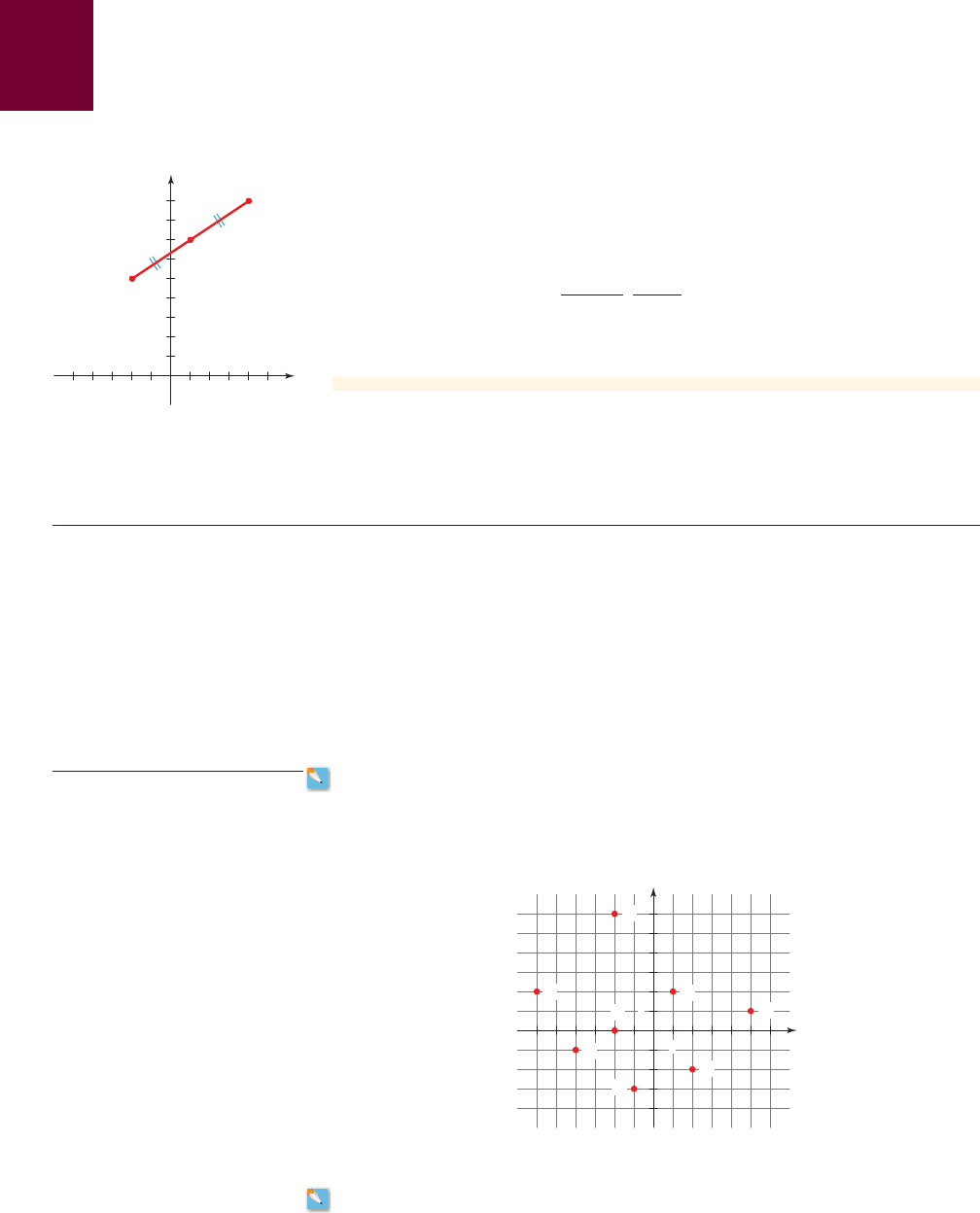
■ The Midpoint Formula

Now let’s find the coordinates (x, y) of the midpoint M of the line segment that joins

the point to the point . In Figure 6, notice that triangles APM and

MQB are congruent because and the corresponding angles are

equal.

d1A, M 2= d1M, B2

B1x

, y

2A1x

, y

A(2, 5)

B(4, _1)

d(A, B)Å6.32

23 4

figure 5

x-x⁄

x¤-x

A(x⁄, y⁄)

M(x, y)

B(x¤, y¤)

Midpoint

figure 6 Midpoint of the line segment AB

It follows that , so . Solving for x, we get

, so . Similarly, .

Midpoint Formula

y =

+ y

x =

+ x

2x = x

+ x

x - x

= x

- xd1A, P2= d1M, Q 2

So the coordinates of the midpoint are the averages of the coordinates of the

endpoints A and B.

T70 ALGEBRA TOOLKIT D

■

Working with Graphs

example

Finding the Midpoint

Find the midpoint of the line segment that joins the points and (4, 9).

Solution

Using the Midpoint Formula, we have

So the midpoint of the line segment is the point (1, 7). See Figure 7.

■ NOW TRY EXERCISE 15(c) ■

- 2 + 4

5 + 9

b= 11, 7 2

1- 2, 52

(4, 9)

(_2, 5)

(1, 7

)

figure 7

D.1 Exercises

CONCEPTS

1. The point that is 3 units to the right of the y-axis and 5 units below the x-axis has the

coordinates (

_______, _______).

2. If x is negative and y is positive, then the point (x, y) is in Quadrant

_______.

3. The distance between the points (a, b) and (c, d ) is

______________. So the distance

between (1, 2) and (7, 10) is

_______.

4. The point midway between (a, b) and (c, d ) is

______________. So the point

midway between (1, 2) and (7, 10) is

_______.

5. Plot the given points in a coordinate plane:

6. Find the coordinates of the points shown in the figure.

12, 3 2, 1- 2, 32, 14, 52, 14, - 52, 1- 4, 52, 1- 4, - 52

SKILLS

7–10 ■ Graph the given set of points in the coordinate plane.

7. 8.

9. 10.

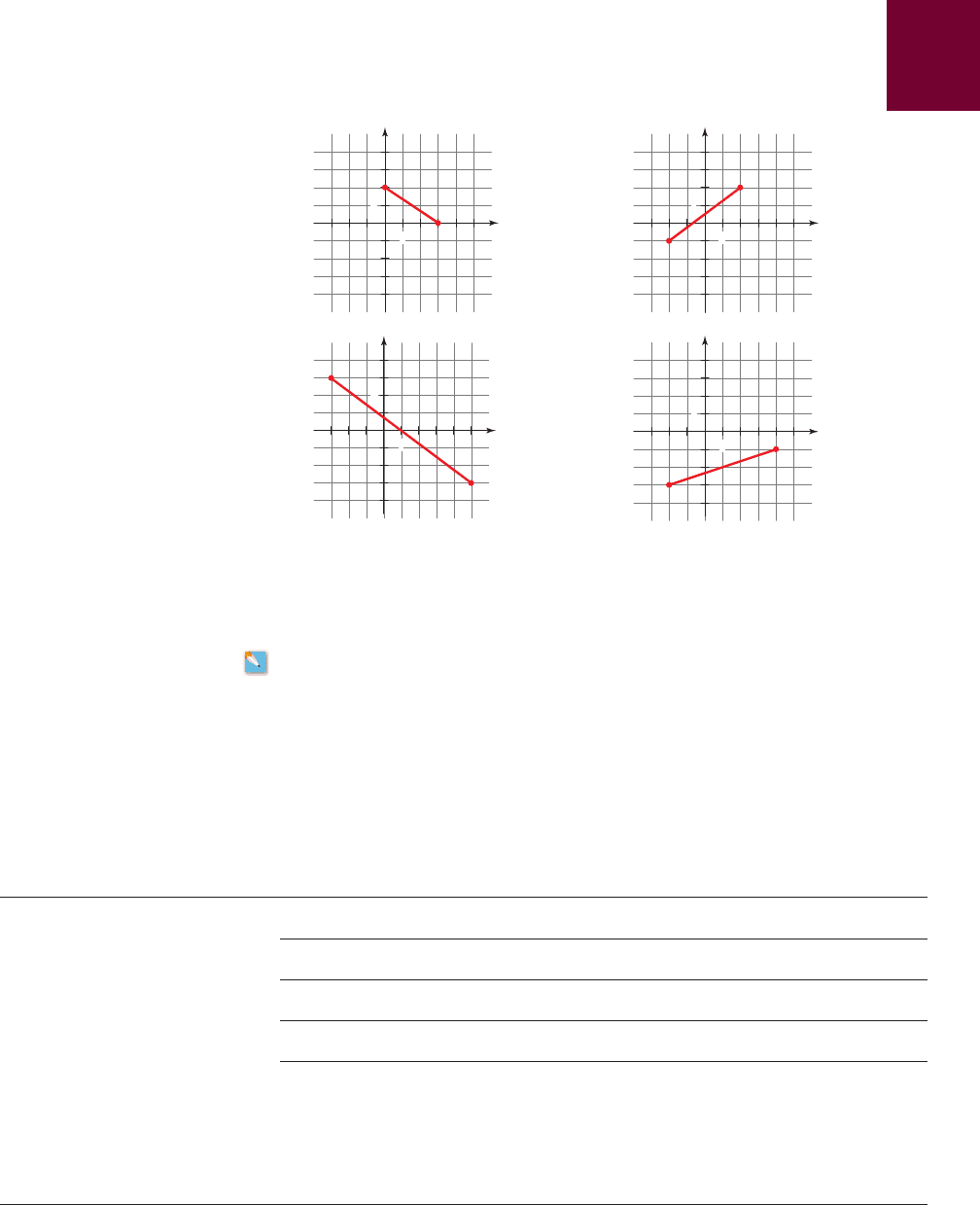
11–14 ■ A pair of points is graphed.

(a) Find the distance between the points.

(b) Find the midpoint of the line segment that joins the points.

51x, y20

x = 5651x, y20 y =-16

51x, y20

y =-2651x, y20 x = 36

SECTION D.2

■

Graphs of Two-Variable Equations T71

11. 12.

13. 14.

15–24 ■ A pair of points is given.

(a) Plot the points in a coordinate plane.

(b) Find the distance between the points.

15. (0, 8), (6, 16) 16. , (10, 0)

17. , (4, 18) 18. , (9, 9)

19. 20.

21. (7, 3), (11, 6) 22. (2, 13), (7, 1)

23. (3, 4), 24. (5, 0), (0, 6)1- 3, - 42

1- 1, 62, 1- 1, - 3216, - 22, 1- 1, 32

1- 1, - 121- 3, - 62

1- 2, 52

D.2 Graphs of Two-Variable Equations

■

Two-Variable Equations

■

Graphs of Two-Variable Equations

■

Finding Intercepts

■

Equations of Circles

The coordinate plane is the link between algebra and geometry because it allows us

to draw graphs of algebraic equations. The graphs, in turn, allow us to “see” the re-

lationship between the variables in the equation.

■ Two-Variable Equations

A two-variable equation is an equation that contains two variables. For example,

the equation

y = 3 + 2x

T72 ALGEBRA TOOLKIT D

■

Working with Graphs

example

Reading an Equation

Consider the two-variable equation .

(a) Give a verbal description of the relationship between the variables in the

equation.

(b) Is the ordered pair (2, 4) a solution of the equation? What about (7, 17)?

Solution

(a) The equation says that “subtracting two times y from five times x gives one.”

(b) To check whether (2, 4) is a solution, we replace x by 2 and y by 4 in the equation.

Equation

Replace x by 2 and y by 4

ⴛ False

So (2, 4) is not a solution. You can check that if we replace x by 7 and y by 17, we

get a true equation, so (7, 17) is a solution.

■ NOW TRY EXERCISE 5 ■

2 = 1

5 122- 2142= 1

5 x - 2y = 1

5x - 2y = 1

■ Graphs of Two-Variable Equations

We graph an equation by plotting all the solutions of the equation in a coordinate plane.

The Graph of an Equation

example

Equation, Table, Graph

Consider the equation .

(a) Make a table of the solutions (x, y) of the equation for .

(b) Sketch a graph of the equation.

x = 0, 1, 2, 3, 4, 5

y = 1 + 2x

To check whether (2, 4) is a

solution, replace x by 2 and y by 4.

5x - 2y = 1

is an equation in the two variables x and y. This equation expresses a relationship be-

tween the two variables; we can read the equation as saying

“y is twice x plus 3”

Expressing the equation in words helps us to understand what the equation is telling us.

An ordered pair (a, b) is a solution to an equation, or satisfies an equation, if

the equation is true when x is replaced by a and y is replaced by b. For example,

(1, 5) satisfies the equation because when we replace x by 1 and y by 5,

we get a true equation: .5 = 3 + 2112

y = 3 + 2x

Replace x by 2

and y by 4

The graph of an equation is a curve, so to graph the equation we plot as many points

as we can and then connect them by a smooth curve.

The graph of an equation in x and y is the set of all points (x, y) in the

coordinate plane that satisfy the equation.

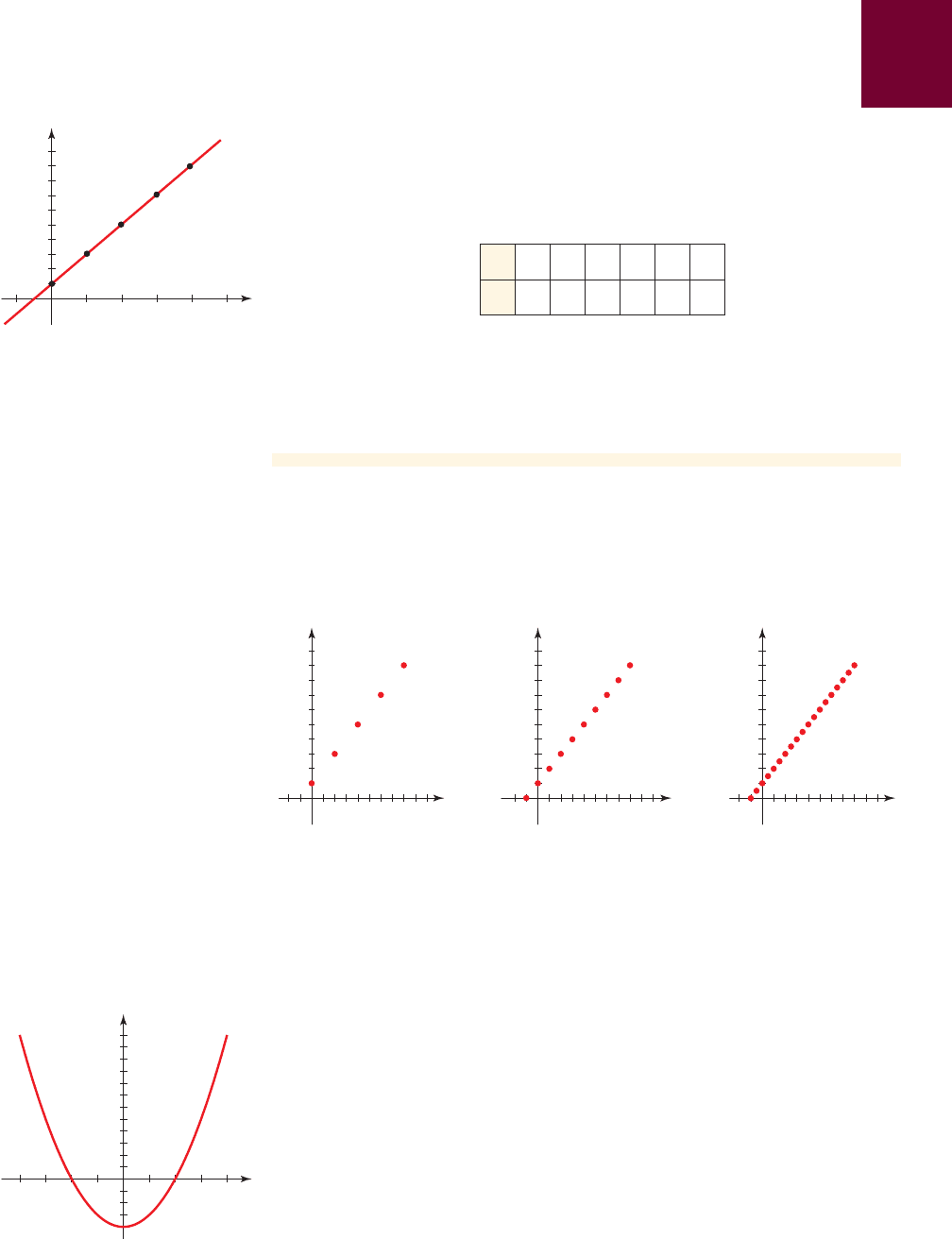
SECTION D.2

■

Graphs of Two-Variable Equations T73

(b) We graph the ordered pairs given in the table of part (a). Of course, the

equation has many more solutions than the ones in the table, so we connect

the points by a smooth curve that represents all the solutions of the equation as

in Figure 1.

■ NOW TRY EXERCISE 9 ■

In Example 1 we sketched the graph of the equation by plotting just

a few points. To check that connecting these points by a smooth curve is correct, we

can try graphing more and more points as in Figure 2. The more points we plot, the

more the graph looks like a line.

y = 1 + 2x

figure 1 Graph of y = 1 + 2x

figure 2 Steps in graphing y = 1 + 2x

x 0 1 2 3 4 5

y 1 3 5 7 9 11

The points on the graph of an equation are solutions of the equation, so we can

read solutions of an equation directly from the graph.

example

Reading the Graph of an Equation

The graph of the equation is shown in Figure 3. Answer the following ques-

tions about the solutions of this equation. Then use the equation to confirm your answer.

(a) Is (2, 2) a solution? What about (3, 5)?

(b) What are the value(s) of x when y is 5?

Solution

We answer these questions from the graph and then from the equation.

(a) From the graph: We see that the point (2, 2) is not on the graph but the point

(3, 5) is on the graph (see Figure 4(a) on the next page). So the ordered pair

(3, 5) is a solution, whereas (2, 2) is not.

y = x

- 4

Solution

(a) If x is 0, then , so (0, 1) is a solution. If x is 1, then

, so (1, 3) is a solution. The other entries in the table below

are calculated similarly.

y = 1 + 2112= 3

y = 1 + 2102= 1

figure 3 Graph of y = x

- 4