Roff D.A. Modeling Evolution: An Introduction to Numerical Methods

Подождите немного. Документ загружается.

rt

ðy

; y

; ...;y

; x

; ...; x

Þdt ¼ 1 ð2:5Þ

Thus the steps to follow are

1. Deﬁne the ﬁtness function. If the function can be integrated we will arrive

at a function in which ﬁtness, r, is on the left, for example,

r ¼ f ðy

; y

; ...;y

; x

; ...;x

Þ or a function in which ﬁtness cannot be sepa-

rated, for example, f ðy

; y

; ...;y

; x

; ...;x

; rÞ¼1:. In the former case the

model can be solved as discussed earlier. For the purposes of generality I shall

assume that r cannot be separated out and that integration of equation (2.5) is to

be done within the program. For clarity I shall consider the particular model

considered in detail in Scenario 5:

rt

16xe

ð10:5xÞt

dt ¼ 1 ð2:6Þ

2. The ﬁrst computer function we deﬁne is one which I shall call INTEGRAND

that gives the value of the function to be integrated, for example, in the

above equation the function would give the value of e

rt

16xe

ð10:5xÞt

, which

requires that we pass to it r, t, and x.InRt is a reserved word and so we

rename it as age:

INTEGRAND <- function(age,x,r) { exp(-r*age)*16*x/exp((1-0.5*x)

*age)}

3. The second function, INTEGRAL, is one that calls the R function integrate to

integrate the above with respect to age (the ﬁrst variable passed) and subtract

1 so that we can use a root-ﬁnding function to solve for r:

INTEGRAL <- function(r,x) {1-integrate(INTEGRAND(1 ,Inf,x,r)$value}

4. The third function, RCALC, calls the R function uniroot to ﬁnd the value of r

that satisﬁes equation (2.6) for a given value of x:

RCALC <- function(x){uniroot(INTEGRAL, interval¼c(1e-7,10),x)$root)}

5. All that now remains is to ﬁnd the value of x that maximizes r. Good practice

dictates that we ﬁrst iterate over values of x to ensure that r is a concave

function of x. Having satisﬁed ourselves that this is the case (see Scenario 5)

we use the R function optimize, which can locate either a minimum (the

default) or a maximum:

optimize(f¼RCALC, interval¼c(1.3,1.8), maximum¼TRUE)$maximum

68 MODELING EVOLUTION

2.2 Summary of scenarios (Table 2.1)

1. Scenario 1 illustrates the analysis of the simplest type of optimality model,

namely one in which the interaction of traits produce a concave function of

ﬁtness with the trait of interest. The example used is that in which body size,

the trait of interest, is a positive function of fecundity and a negative function

of survival. A semelparous life history is assumed, making analysis very sim-

ple. Scenarios 2–8 consider variants of Scenario 1.

2. In Scenario 2 age structure is added in such a manner that the analysis is

unaffected, illustrating the principle that additional complications to a model

may be mathematically neutral.

3. Scenario 3 also includes only the addition of age structure but in such a

manner that the optimum trait value is affected.

4. Scenario 4 is the same as Scenario 3 except that the ﬁtness function is a

continuous rather than a discrete function of the trait of interest.

5. In Scenarios 1–4 ﬁtness is measured by R

which makes analysis relatively

simple. Scenario 5 considers the analysis of the model with r as the ﬁtness

function.

6. Scenarios 1–5 assume that parameter values are constant. Scenarios 6–8 con-

sider models in which one or more of the parameters are variable. Scenario 6

assumes stochasticity in one or more parameters within but not among gen-

erations. In this case ﬁtness is the arithmetic mean ﬁtness. An important point

made by this example is that mean ﬁtness is not calculated simply using the

means of the parameters.

7. Scenarios 7–8 assume that parameter values are temporally variable, in which

case the ﬁtness measure is the geometric mean rather than the arithmetic.

Scenario 7 considers discrete temporal variation parameter values.

8. Scenario 8 examines the consequences of continuous temporal variation in

parameter values.

9. Scenarios 9–14 illustrate the analysis of models in which two traits are of

interest. In Scenario 9 the two traits are vigilance and foraging rate.

10. Scenario 10 illustrates that the two traits of interest may be independent even

though ﬁtness is a function of both.

11. Scenarios 11 and 12 examine a prominent problem in life history theory,

namely the coevolution of propagule and clutch size. Scenario 11 illustrates

a circumstance in which one trait is determined by the value of the second

and hence the problem is reduced to the analysis of a single trait.

12. Scenario 12 expands Scenario 11 such that the two traits (propagule size in

clutches 1 and 2 of a three-clutch life history) covary and cannot be reduced to

a single trait. An important feature of this analysis is the illustration of the

brute force method. It also illustrates the importance of plotting the ﬁtness

surface to determine if it is “smooth” or “rugged”.

FISHERIAN OPTIMALITY MODELS 69

Table 2.1 Summary of principle model assumptions in the scenarios (S) described in the text

S Focal trait(s) Constraining traits W Scenario feature

1 Body size Fecundity and

survival

Simplest model

...................................................................................................................................................

2 Body size Fecundity and

survival

No effect of age structure

...................................................................................................................................................

3 Body size Fecundity and

age‐speciﬁc survival

Discrete age structure and

optimum changed

...................................................................................................................................................

4 Body size Fecundity and age‐

speciﬁc survival

Continuous age function and

optimum changed

...................................................................................................................................................

5 Body size Fecundity and age‐

speciﬁc survival

Fitness cannot be isolated

on one side of ﬁtness

function

...................................................................................................................................................

6 Body size Fecundity and age‐

speciﬁc survival

Arithmetic

mean

Stochastic variation in

parameters

...................................................................................................................................................

7 Body size Fecundity and age‐

speciﬁc survival

Geometric

mean

Discrete temporal variation in

a parameter

...................................................................................................................................................

8 Body size Fecundity and age‐

speciﬁc survival

Geometric

mean

Continuous temporal

variation in a parameter

...................................................................................................................................................

9 Vigilance and

foraging rate

Survival Survival Simple two‐trait model

...................................................................................................................................................

10 Body size and

fecundity

Propagule size and

survival

Optima independent and

hence each trait analyzed

separately

...................................................................................................................................................

11 Clutch sizes 1 and 2 Reserves, fecundity,

and propagule size

Two traits but analysis

reduces to that of a

single trait

...................................................................................................................................................

12 Age‐speciﬁc clutch

sizes

Reserves, fecundity,

and propagule size

Importance of plotting to

examine ﬁtness surface and

illustration of a brute force

method

...................................................................................................................................................

13 Age at ﬁrst

reproduction and

single reproductive

allocation

Continuous growth,

weight‐speciﬁc

fecundity, and adult

mortality

Recursion dictates use of

brute force approach

...................................................................................................................................................

14 Age at ﬁrst

reproduction, age‐

speciﬁc

reproductive

allocation

Continuous growth,

weight‐speciﬁc

fecundity, and adult

mortality

Expansion of analysis to more

than two traits

70 MODELING EVOLUTION

13. Scenario 13 illustrates the analysis of models that involve recursion and which

may require a brute force approach. The speciﬁc problem considered is that of

ﬁnding the optimal age at maturity and allocation to reproduction in an

iteroparous organism.

14. Scenario 14 expands the problem considered in the forgoing scenario by

allowing the allocation to reproduction to change with age, thereby increas-

ing the number of traits to the number of mature age classes plus one (age at

maturity).

2.3 Scenario 1: A simple trade-off model

This scenario illustrates the analysis of the case in which there is no age structure

and ﬁtness is a simple quadratic function of the trait under study. Because of its

simplicity, its visualization and analysis is readily accomplished. Given the ease

with which this type of model can be analyzed it is worthwhile, if possible,

to commence with such a model. Following the analysis of this model further

biological assumptions, such as age structure as in Scenarios 2 and 3, can be added.

This approach allows one to assess the importance of particular assumptions.

The present model considers the case of a semelparous organism in which the

trait under investigation varies positively with one ﬁtness component and nega-

tively with another. A priori, one might be tempted to assume that this will

necessarily result in a function that has a maximum at some intermediate trait

value. This is not necessarily the case, suppose, for example the two ﬁtness

functions are

¼ e

2 ¼ e

bx

ð2:7Þ

where y

and y

are two traits such as fecundity and survival, a and b are constants,

and x is the trait under study (e.g., body size). Let us suppose that ﬁtness is the

product of y

and y

(which is quite reasonable): ﬁtness, W, is then given by

W ¼ y

¼ e

ðabÞx

ð2:8Þ

which has no intermediate optimum. In the scenario discussed below an interme-

diate optimum is not assured but does occur for particular parameter values.

2.3.1 General assumptions

1. The organism is semelparous.

2. Fecundity, F, increases with body size, x.

3. Survival, S, decreases with body size, x.

4. Fitness, W, is a function of fecundity and survival.

FISHERIAN OPTIMALITY MODELS 71

The above assumptions describe a very general situation. To make a prediction

we must convert these assumptions into mathematical expressions. But even

before doing this we can make a general statement. Because one ﬁtness compo-

nent, fecundity, increases with body size, whereas the second component, surviv-

al, decreases with body size then in many (but not all) circumstances we can

expect that there will be an optimum body size that maximizes ﬁtness. To

investigate this we shall give explicit mathematical expressions to the three

assumptions.

2.3.2 Mathematical assumptions

1. Fecundity increases linearly with body size:

F ¼ a

þ b

x ð2:9Þ

where a

and b

are constants.

2. Survival decreases linearly with body size:

S ¼ a

 b

x ð2:10Þ

3. Fitness, W, is the expected lifetime reproductive success, R

, given as the

product of fecundity and survival:

W ¼ R

¼ FS

¼ða

þ b

xÞða

 b

xÞ

¼ a

 b

þða

 a

Þx

ð2:11Þ

The above equation describes a parabola that is concave down, that is, has a

maximum value at some body size, say x*. Thus for this model we already know

that there is an optimal body size, though we do not know if this occurs at a

plausible body size. The ﬁrst step in the analysis is to plot W versus x to visually

conﬁrm that a maximum exists and show that it occurs at a value to be expected

for the species or taxa under study.

2.3.3 Plotting the ﬁtness function

It is usually a good idea to plot the function to visually examine its behavior. While

we know that in the present case the function is a quadratic that is concave down

we do not know if the optimum trait value is plausible given biologically plausible

parameter values. Thus we must assign parameter values. For the purposes of

illustration, let us suppose that reasonable values are a

¼ 0, b

¼ 4, a

¼ 1, b

¼ 0.5.

The ﬁtness equation can now be written as

72 MODELING EVOLUTION

W ¼ 0  1  4  0:5x

þð1  4  0  0:5Þx

¼2x

þ 4x

ð2:12Þ

R CODE:

rm(list¼ls()) # remove all objects from memory

x <- seq(0,2,length¼ 1000) # Create a vector of length 1000 be-

tween 0, 2

W <- (-2*x^2 þ 4*x) # Create a vector W using the fitness function

# Plot the data using ’l’ to designate a line

# las¼number orientation on axes, lwd ¼ line width

plot(x,W,type¼’l’,xlab¼’Body size, x’, ylab¼’Fitness, W’,

las¼ 1,lwd¼3)

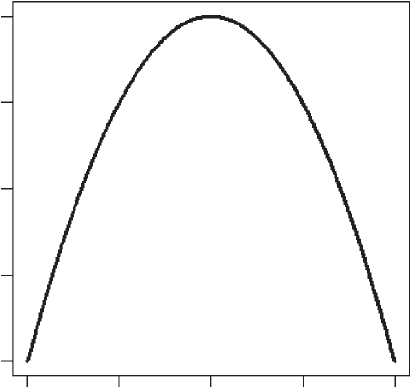
From the plot of the ﬁtness function (Figure 2.1) it can be seen that there is a

maximum at or around 1.

MATLAB CODE: See Section 2.18.1.

2.3.4 Finding the maximum using the calculus

We can now proceed in one of two ways:

1. Find the optimal body size by the calculus.

2. Use a numerical method without resorting to the calculus (Section 2.3.5).

To obtain x* using the calculus we differentiate the equation, set the result to zero

and ﬁnd the value of x that satisﬁes this condition. In the present case the

differentiation can be easily accomplished using the rules given in Appendix 2:

Body size, x

0.0

0.5

1.0

1.5

2.0

0.5 1.0 1.5 2.0

Fitness, W

Figure 2.1 Scenario 1: Fitness versus body size.

FISHERIAN OPTIMALITY MODELS 73

¼4x þ 4

¼ 0 when  4x þ 4 ¼ 0; i:e:; x ¼ 1

ð2:13Þ

R CODE:

Symbolic differentiation in R can be done using deriv:

y <- deriv(-2*x^2þ4*x,“x”) # Take the derivative and store in y

As discussed in the Introduction, the output is not particularly clear as it returns

the expression for the evaluation of the derivative:

y # Print y

OUTPUT:

expression({

.value <--2*x^2 þ 4*x

.grad <- array(0, c(length(.value), 1L), list(NULL, c(“x”)))

.grad[, “x”] <-4-2*(2*x)

attr(.value, “gradient”) <- .grad

.value

})

The value at which the derivative is zero can now be determined using the

function uniroot. We set the derivative as a separate function to be called by

uniroot:

rm(list¼ls()) # remove all objects from memory

# Function to obtain the gradient at a value w

FUNC <- function(w)

{

y <- deriv(2*x^2þ4*x,“x”) # Get the derivative

x <- w # Set x equal to w

z <- eval(y) # Evaluate the derivative at w

d <- attr(z,“gradient”) # Assign the gradient value to d

return(d) # Return d to the main program

}

# MAIN PROGRAM

# Root must be enclosed by the limits set by the user, here set at

2to4

B <- uniroot(FUNC, interval¼ c(2,4))

B$root # Print out the value found

MATLAB CODE: See Section 2.18.2.

74 MODELING EVOLUTION

2.3.5 Finding the maximum using a numerical approach

In many cases the function may not be easily differentiable: for example, the

function might consist of a simulation model or it may have discontinuities. We

shall encounter such cases shortly. The present model can be used to illustrate the

general approach.

The available routines typically locate the minimum value of a function. In our

case we wish to ﬁnd the maximum. To use the minimization routines we simply

take the negative value of our function. Thus, instead of seeking the maximum of

4x2x

we seek the minimum of 2x

4x. There are generally several routines that

ﬁnd minima. We shall, in this case, use nlm in R (an alternate is optimize) and

fminbnd in MATLAB.

R CODE:

# Create function to evaluate fitness function

FITNESS <- function(x) (2*x^2-4*x)

nlm(FITNESS, p¼2) # Call nlm with initial guess for x of 2

MATLAB CODE: See Section 2.18.3.

2.4 Scenario 2: Adding age structure may not affect the

optimum

We increase the complexity of the problem by introducing age-structure, which

we might expect to change the solution. In fact, they do not: Increasing complex-

ity need not change either the qualitative or quantitative conclusions.

2.4.1 General assumptions

1. The organism is iteroparous.

2. The following assumptions are the same as in Scenario 1.

3. Fecundity, F, increases with body size, x, which does not change after maturity

(e.g., as in insects).

4. Survival, S, decreases with body size, x.

5. Fitness, W, is a function of fecundity and survival.

2.4.2 Mathematical assumptions

1. Maturity occurs at age 1 after which no further growth occurs.

2. Fecundity increases linearly with size at maturity, resulting in fecundity being

a uniform function of age, t:

¼ a

þ b

x ð2:14Þ

FISHERIAN OPTIMALITY MODELS 75

3. Survival to the age at ﬁrst reproduction, here 1, decreases linearly with body

size and is thereafter constant per time unit and independent of body size.

Survival to age t is then given by

¼ða

 b

xÞe

Mðt1Þ

ð2:15Þ

where M is the instantaneous mortality rate that is independent of age.

4. Fitness, W, is the expected lifetime reproductive success, R

, given as the

cumulative product of survival and fecundity:

W ¼ R

t¼1

ða

þ b

xÞða

 b

xÞe

Mðt1Þ

ð2:16Þ

Although the above equation looks a lot more complicated than equation

(2.11) it actually contr ibutes no new information that changes the optimal

size, because those components that depend on size do not depend on

age and hence can be moved out of the summation sign. Consequently,

we have that the ﬁtness equation speciﬁed above is simply equation (2.11)

multiplied by a constant. Thus the optimal size remains unchanged.

2.5 Scenario 3: Adding age-speciﬁc mortality that affects

the optimum

We retain the same general assumptions as before but introduce a mathematical

change to the survival function that will alter the optimal size.

2.5.1 General assumptions

1. The organism is iteroparous.

2. Fecundity, F, increases with body size, x, which does not change after maturity

(e.g., as in insects).

3. Survival, S, decreases with body size, x.

4. Fitness, W, is a function of fecundity and survival.

2.5.2 Mathematical assumptions

1. Maturity occurs at age 1 after which no further growth occurs.

2. Fecundity increases linearly with size at maturity, resulting in fecundity being

a uniform function of age:

¼ a

þ b

x ð2:17Þ

76 MODELING EVOLUTION

3. The instantaneous rate of mortality increases linearly with the body size

attained at age 1 and is constant per time unit. Under this assumption, survival

to age t is given by

¼ e

ða

þb

xÞt

ð2:18Þ

Note that to make survival a declining function of body size, given the exponential

function, we replace the previous a

b

x with a

þb

4. Fitness, W, is the expected lifetime reproductive success, R

, given as the

cumulative product of survival and fecundity:

W ¼ R

t¼1

ða

þ b

xÞe

ða

þb

xÞt

ð2:19Þ

We cannot now factor out the age-dependent effects from body size and hence the

optimal body size will not be the same as found previously.

2.5.3 Plotting the ﬁtness function

Before seeking the turning point we ﬁrst plot the ﬁtness function to verify that it

has a turning point and the function is not oddly shaped such that the routines

locating the maximum will not home in on a single value, regardless of the

starting points. We shall assume the same parameter values as before (a

¼ 0,

¼ 4, a

¼ 1, b

¼ 0.5), and thus W ¼

4xe

ð1þ0:5xÞt¼1

R CODE (Figures 2.2 and 2.3):

The above summation is taken to inﬁnity, which is generally not an option with a

numerical analysis. Thus ﬁrst, we have to decide on how many ages, n, we need to

consider in the summation. To do this we set body size, x, at some arbitrary but

reasonable value, say x ¼ 1. The following commands do the summation and plot

the results as a function of the number of ages. The program consists of a separate

function called SUMMATION that calculates the value of equation (2.19) from 1 to n.

It does this by

1. Generating an integer sequence from 1 to n and assigning this to a vector called

Age.

2. It then creates another vector called Wt, which is the age-speciﬁc component of

equation (2.19), namely 4xe

ð1þ0:5xÞt

3. Finally it computes the sum of the vector Wt using the R function sum.

The main program is as follows:

1. First sets the maximum number of ages, nmax, to use at 20.

2. Creates a single column matrix called n with the integer sequence 1 to nmax.

FISHERIAN OPTIMALITY MODELS 77