Peube J-L. Fundamentals of fluid mechanics and transport phenomena
Подождите немного. Документ загружается.


Transport and Propagation 213
x
F
A
x
F
A
x
y
B
G
G
G
G
w
w
¸
¸
¹
·
¨
¨
©
§
[5.23]
From this we can calculate the characteristic determinant
yAxBQ
GG
and
the roots
xy
G
G
O
. Equation [5.10] can here be written as:
01
10
0
01
2
OOU
OU
OOU
O
vu
uv
uvQ
[5.24]
Equation [5.24] only has one real root
uvxy
G
G
O
which corresponds to
the differential equation of the trajectories. For this root, system [5.11] can be
written:
0
100
00
01
32
1
1
321
¸
¸
¸
¹
·
¨
¨
¨
©
§
¸
¸
¸
¹
·
¨
¨
¨
©
§
¸
¸
¹
·
¨
¨
©
§
t
LuvL
L
uvL
uv
uv
LLL
u
v
ABL
hence:
uvLLLL /10
321
.
Substituting into relation [5.12] gives:
0
00
10
001
10
¸
¸
¸
¹
·
¨
¨
¨
©
§
¸
¸
¸
¹
·
¨
¨
¨
©
§
pvvuu
p
v
u
u
uuvFLA
GGUGU
G
G
G
U
UG
[5.25]
The characteristic curves
uvxy
G
G
are the trajectories, and the variation of
the quantity pvvuu
G
G
U
G
U
is zero on these. We can recognize Bernoulli’s
first theorem for a perfect incompressible fluid (see section 4.3.2.3.1, relation [4.30])
which was obtained previously.
5.3.2.3.
Steady 2D flow of a inviscid compressible fluid
The equations for a steady 2D plane of perfect compressible fluid can be written
([4.6], [4.22] and [4.55]):

214 Fundamentals of Fluid Mechanics and Transport Phenomena
0.0
;0;0
22
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
w
y
v
c
x
u
c
y
p
v
x
p
u
y
p
y
v
v
x
v
u
x
p
y
u
v
x
u
u
y
v
x
u
y
v
x
u
UUUU
UUUU
UU
[5.26]
or, in matrix form [5.7]:
0
¸
¸
¹
·
¨
¨
©
§
w
w
w
w
F
y
B
x
A
22
00 0 0
001000
with: ; ; .
00 0 00 1
00 00
u v
u v
u
FA B
u v
v
p
cu cv
¬¬
¬
®
®®
Characteristic equation [5.10] can be written:
0
0
100
00
0
22
uvcc
uv
uv
uv
A
x
y
BQ
OUUO
OU
OOU
UOUO
G
G
or:
>
@
02
22222
2
2
cvuvcuuv
OOOU
[5.27]
It has the same real root
uv
O
as for the incompressible fluid, but here it is a
double root.
The two other roots satisfy a second degree equation whose discriminant can be
written:
>
@
22222222
if0' cvuVcvuc t t ' [5.28]
These are real only if the flow is supersonic (V > c). If the flow is subsonic, the
roots and the characteristic curves are imaginary, as in the case of the
incompressible fluid. The values of the slopes of the characteristic curves are:
Transport and Propagation 215
22
2222
])[(
cu
cvucuv
x
y
r
G
G
O
[5.29]
The velocity V
G
is the bisector of the characteristic curves; in effect, taking the
axis Ox parallel to the velocity V
G
(i.e. v = 0), slopes [5.29] of the characteristic
curves in the plane (x,y) are opposed:
1
1
2
22
2
r
r
M
cu
c
x
y
G
G
O
where
cVM designates the local Mach number defined using the local sound
speed c.
The reader can easily verify that the characteristic curves lie at an angle
T
with
respect to the velocity vector (or the trajectory) defined by:
M1sin
T
(we have
T
O
tan ); these curves are thus called Mach lines.
Identification of the relationship between the components of the differential
G
F
on each of the characteristic curves can be effected as previously by finding a vector
L which is a left solution of equation [5.11] and by substituting it into equation
[5.12]. We will here only show the calculation for the value
uv
O
:
0
00
1000
000
00
22
4321
¸
¸
¸
¸
¸
¸
¸
¸
¹
·
¨
¨
¨
¨
¨
¨
¨
¨
©
§
¸
¸
¹
·
¨
¨
©
§
cc
u
v
u
v
u
v
LLLL
u
v
ABL
UU
UU
or:
.0;0;0
32
2
41
2
41
L
u
v
LcLLcL
u
v
L
u
v
UUUU
From this we can calculate L
3
and L
4
as a function of the two arbitrary values of
L
1
and L
2
:
00
32
2
41
L
u
v
LcLL
216 Fundamentals of Fluid Mechanics and Transport Phenomena
Substituting into condition [5.12] gives:
0
2
2
1
¸
¸
¹
·
¨
¨
©
§
pvvuuL
c
p
uL
GGUGU
U
G
UG
As this equation is satisfied regardless of the values of L
1
and L
2
, we have the
two relations:
0;0
2
¸
¸
¹
·
¨
¨
©
§
pvvuu
c
p
GGUGU
U
G
UG
which describe the Lagrangian conservation of entropy and mechanical energy on
the trajectories (Bernoulli’s first theorem).
5.3.3. Cauchy problem with n unknowns and p variables
Now consider an n dimensional vector function F with components f
i
ni ,...,1, functions of p variables x
j
pj ,...,1 . The function F satisfies the n
first order quasi-linear partial differential equations:
¸
¸
¹
·
¨
¨
©
§
w
w
w
w
0
),...;1
;,...,1,(
0 G
x
F
A
pj
ni
g
x
f
A
i
j
ji
A
A
A
[5.30]
We will now solve the Cauchy problem, in other words we will calculate the
variation F
G
of the function F in the neighborhood of a point M of a hypersurface
S
0
of dimension p – 1 on which the values of F are given. This results in our
knowing p – 1 independent derivatives of the function F calculated following the
surface directions. The derivative of the vector function F following a transverse
direction S
0
is not given, but it can be calculated from system [5.30].
In order to individualize this direction, we will perform a change of coordinates
pkjxx
kjj
,...,1,
[
.
The Wronskian (functional determinant)
k
j
x
[
w
w
is assumed non-zero such that
we can also write:
Transport and Propagation 217
pkjx
jkk
,...,1,
[
[
This gives the following relationships between the partial derivatives of F with
respect to the two sets of variables:
¸
¸
¹
·
¨
¨
©
§
w
w
w
w
w
w
w
w
w
w
w
w
x
F
x
F
pkj
n
x
f
x
f
j
k
kj
[
[
[
[
.
),...1,
;,...,1(A
AA
[5.31]
System [5.30] can thus be written:
¸
¸
¹
·
¨
¨
©
§
w
w
w
w
w
w
w
w
0.
,...1,
;,...,1,
0
G
x
F
A
pkj
ni
g
x
f
A
i
j
k
k
ij
[
[
[
[
A
A
A
[5.32]
We will choose the coordinates
[
k
),...,3,2(
pk such that they describe the
surface (curvilinear coordinates on
S
0
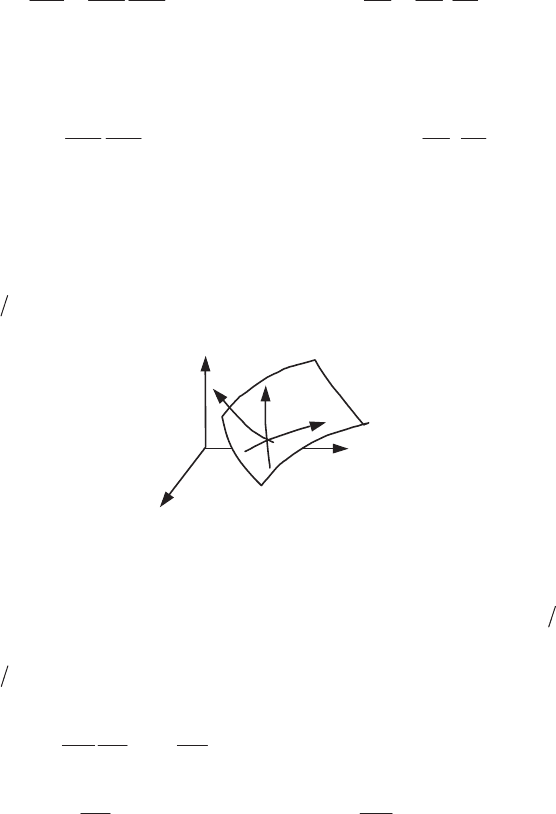
(Figure 5.2)). The curve of coordinate
[
1
crosses the surface
S
0
whose equation is
0,...
211
p
[[[[
. The derivatives
k
f ss
A
are known from the functions f
A
on the surface S
0
.
O
S
0
[
[
3
[
1
x
1
x
2
x
3
Figure 5.2. Initial surface of the Cauchy problem
All that remains is to determine the n unknown derivatives
1
[
ww
A
f in the
direction
[
1
by means of system [5.30] in which all the other terms in
1zww kf
k
[
A
are now known; system [5.30] can be written:
1
1
1
1
, 1,..., ;
,.. 0
1,... ; 2,...
or: 0 with:
ij i i
jk
ij
j
in
ff
A # g
j
pk p
x
F
AG AA
x
<
¬
ss s
ss s
®
¬
s
s
ss
®
AA
A
A
A
[5.33]

218 Fundamentals of Fluid Mechanics and Transport Phenomena
where the function
i
\
is known from the derivatives which are already given
1zww kf
k
[
A
.
If the determinant of the matrix
A
~
is non-zero, system [5.33] with unknowns
1
[
ww
A
f is of rank n and the n derivatives
1
[
ww
A
f can be uniquely determined.
The Cauchy problem thus possesses a unique solution.
If, on the other hand, we have
0
~
A , system [5.33] is of rank n – 1 and the
determination of the
n unknown derivatives
1
[
ww
A
f is no longer possible.
Equation
0
~
A is a partial differential equation for the scalar function
j
x
1
[
of
p variables:
0
~
1
w
w
j
ij
x
AA
[
A
[5.34]
We thus have a problem such as that described in section 5.2.4. The vector
j
xww
1
[
associated with the point M and satisfying equation [5.34] belongs to a
hypersurface (the cone C with summit M) of dimension
p – 1 of the geometric space
(
x
1
,…, x
p
). At the point M, this vector is the direction of the surface normal S
0
of the
equation
[
1
= 0; the tangent plane is normal to it, and the surface S
0
is surrounded by
tangent planes whose normals belong to the cone C
. This surface S
0
is itself a cone
of summit M to which the characteristic curves passing through M are tangent.
As before, in the case
0
~
A , there exists a non-zero vector L such that
0
1
w
w
j
iji
x
AL
[
A
. Equation [5.33] thus results in a linear relation between the partial
derivatives
1zww kf
ki
[
:
pknig
f
L
i
k
ii
,...2;,...,1,0,..
»
»
¼
º
«
«
¬
ª
¸
¸
¹
·
¨
¨
©
§
w
w
< A
A
[
5.3.4. Partial differential equations of order n
The same reasoning can be applied for an n
th
order quasi-linear partial
differential equation with p variables, or for a system of such equations. For
Transport and Propagation 219
example, consider the quasi-linear second order equation whose coefficients and the
right-hand side term g depend on the function f, on its first derivatives and on the
variables x
i
:
)1 (
2
,....,pi,jg
xx
f
A
ji
ij
ww
w
[5.35]
Suppose now that the values of the function f and of its first derivatives are given
at all points M of a hypersurface S
0
of dimension p – 1. The values of the second
derivatives taken on this surface are known. We can perform the change of
coordinates described in section 5.3.3, such that the second derivatives of the
function are known on the surface S
0
of equation
0,...
211
p
[
[
[
[
in the
reference frame
i
[
. The change of variables [5.31] allows the terms of equation
[5.35] to be written in the form:
22
...( , 1,..., )
km
ij km j i
ff
ij p
xx xx
ss
ss
ss ss s s
The calculation is performed as previously, and the equation thus transformed
should allow the calculation of the unknown value of
2
1
2
[
w
w f
at the point where we
seek a solution of the Cauchy problem. We obtain:
),...,2(
),...,1,(
0,..,,
2
2
1
2
11
pk
pji
g
ff
f
f
xx
A
kiiji
ij
¸
¸
¹
·
¨
¨
©
§
ww
w
w
w
w
w
w
w
w
w
[[[
\
[
[[
[5.36]
where the function
\
is a function of
f
, of its first derivatives and of its second
derivatives
1
2
z
ww
w
k
f
ki
[[
, which are known from the initial data given at all
points M of the hypersurface
S
0
. The coefficient of
2
1
2
[
ww f
must obviously be
non-zero for the calculation to be possible. If it is zero, we find a direction
[
1
corresponding to the characteristic surface
0,...
21
p
[[[
. These characteristic
directions
i
i
xw
w
1
[
D
satisfy the equation:

220 Fundamentals of Fluid Mechanics and Transport Phenomena
),...,1,(;0
11
pjiA
xx
A
jiij
ji
ij
w
w
w
w
DD
[[
[5.37]
The left-hand side of equation [5.37] is a quadratic form which represents the
equation of a second degree cone comprising the normals to the characteristic
surfaces. If it is imaginary, we have an
elliptic equation
. If on the other hand it is
real, the equation is
hyperbolic
. The preceding equation, which is easy to write,
allows us to reduce our study of the type of a differential equation to the
identification of the nature of a quadratic form. Instead of searching for the principal
directions and performing an orthogonal change of reference frame, we can follow
the simpler procedure of directly identifying a reduced form for this quadratic form.
We will look at some applications in the next section.
The compatibility relation of equation [5.36] can be written for the solutions of
[5.37]:
),...,2(
),...,1,(
0,..,,
2
pk
pji
g
ff
f
kii
¸
¸
¹
·
¨
¨
©
§
ww
w
w
w
[[[
\
5.3.5. Applications
5.3.5.1.
Quasi-linear partial differential equation
We will apply the preceding results to the flow of an inviscid fluid, limiting
ourselves to the identification of characteristic surfaces for the two-variable
examples already studied.
We immediately recover the results of section 5.2, by applying the preceding
results to equation [5.3]. Characteristic equation [5.37] can be written:
0
111
w
w
w
w
w
w
y
v
x
u
t
[[[
Its solutions
0,,
11
yxt
[
[
are equations of surfaces comprising the
trajectories (the equation shows in effect that the surface normals are normal to the
vector (1,
u
,
v
) which is thus situated in the tangent plane of the surface).
5.3.5.2.
System of partial differential equations with two independent variables
The characteristic curves obtained in section 5.3.1 can also be found using
characteristic equation [5.34]. This can be written as:
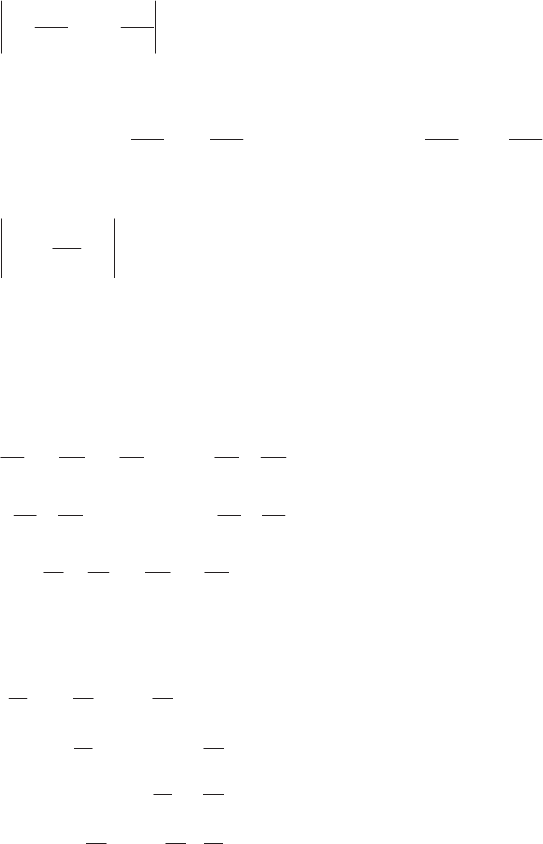
Transport and Propagation 221
ni
x
B
t
A
ii
,...,1,0
11
w
w
w
w
A
AA
[[
[5.38]
In two dimensions, the characteristic “surfaces” are the curves
0,
1
xt
[
on
which we have
0
11
1
w
w
w
w
x
x
t
t
G
[
G
[
[G
. Eliminating
tw
w
1
[
and
xw
w
1
[
between
this relation and [5.38], we recover equation [5.10] for the characteristic curves:
0
AA
ii
A
t
x
B
G
G
5.3.5.3.
Unsteady 2D flow of a perfect compressible fluid
Let us reconsider the study of section 5.4.2.3. with the two spatial coordinates
(
x,y
). The equations for an inviscid compressible fluid can be written ([4.6], [4.22]
and [4.54]):
2
0; 0
0; 0
with:
d uv dup
dt x y dt x
dv p d dp
c
dt y dt dt
d
uv
dt t x y
ss s
ss s
s
s
sss
sss
[5.39]
or in matrix form:
0
0
00
00
0
22
¸
¸
¸
¸
¸
¹
·
¨
¨
¨
¨
¨
©
§
¸
¸
¸
¸
¸
¸
¸
¸
¸
¸
¹
·
¨
¨
¨
¨
¨
¨
¨
¨
¨
¨
©
§
w
w
w
w
w
w
w
w
w
w
w
w
p
v
u
dt
d
y
c
x
c
ydt
d
xdt
d
yxdt
d
U
UU
U
U
UU
The unknown function
f
i
which has four components (
U
,
u
,
v
,
p
) is assumed to be
given on the surface
S
0
defined in the preceding section and characterized by the
coordinates
1
zk
k
[
. Setting
yxxxctx
321
,, , we can perform the
change of variables [5.31]:
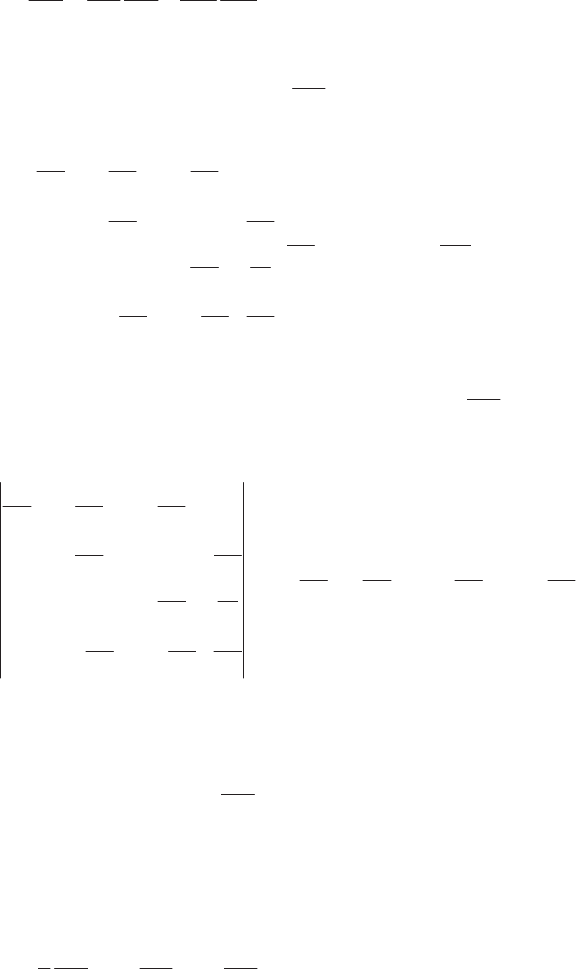
222 Fundamentals of Fluid Mechanics and Transport Phenomena
3,2;4,..,1
1
1
w
w
w
w
w
w
w
w
w
w
ki
x
f
x
f
x
f
j
k
k
i
j
i
j
i
[
[
[
[
Separating the unknown derivatives
1
[
w
w
i
f
we have:
3,20
(known)
similar to
terms
0
00
00
0
1
11
2
1
2
1
11
111
¸
¸
¸
¸
¸
¹
·
¨
¨
¨
¨
¨
©
§
w
w
¸
¸
¸
¸
¸
¹
·
¨
¨
¨
¨
¨
©
§
w
w
¸
¸
¸
¸
¸
¸
¸
¸
¸
¸
¹
·
¨
¨
¨
¨
¨
¨
¨
¨
¨
¨
©
§
w
w
w
w
w
w
w
w
w
w
w
w
k
p
v
u
p
v
u
dt
d
y
c
x
c
ydt
d
xdt
d
yxdt
d
k
U
[
U
[
[[
U
[
U
[
U
[[
U
[
U
[
U
[
This system of equations does not have a unique solution
1
[
w
w
i
f
if its determinant
is zero:
0
0
00
00
0
2
1
2
2
1
2
2
1
2
1
2
11
2
1
2
1
11
111
»
»
¼
º
«
«
¬
ª
¸
¸
¹
·
¨
¨
©
§
w
w
¸
¸
¹
·
¨
¨
©
§
w
w
¸
¸
¹
·
¨
¨
©
§
¸
¸
¹
·
¨
¨
©
§
w
w
w
w
w
w
w
w
w
w
w
w
x
c
y
c
dt
d
dt
d
dt
d
y
c
x
c
ydt
d
xdt
d
yxdt
d
[[[[
U
[[
U
[
U
[
U
[[
U
[
U
[
U
[
The characteristic equation can be decomposed into two first order partial
differential equations:
1) A quasi-linear equation
0
1
dt
d
[
whose solution we have already studied in
section 5.2.2. We have seen that the characteristic curves are the trajectories, the
corresponding characteristic surfaces being defined from these.
2) The partial differential equation:
0
1
2
1
2
1
2
1
¸
¸
¹
·
¨
¨
©
§
w
w
¸
¸
¹
·
¨
¨
©
§
w
w
¸
¸
¹
·
¨
¨
©
§
xydt
d
c
[[[
