Peube J-L. Fundamentals of fluid mechanics and transport phenomena
Подождите немного. Документ загружается.


Fluid Dynamics Equations 183
“physical” flux convective flux
¦ 6
w
w
³³ ³ ³
GGjj ii
DD
g
dv dv q n ds gu n ds
t
V
The left-hand side term corresponds to the possible accumulation of the quantity
G in
D
. This variation of the quantity G contained in this domain is the sum of:
the production of the sources
V
G
;
the fluxes of the quantity G due to transfers through matter;
the fluxes of quantity G associated in the matter which enters
D
by crossing the
boundary 6 (convective fluxes).
In the preceding equations, we can interpret the surface and volume integrals in
different ways:
– the surface integrals over 6 comprise inputs and outputs of the material system
contained in
D
, in other words we can evaluate them without knowing the internal
structure of the flow within the system;
– the volume integrals in the domain
D
clearly imply the internal variables of the
system for which a model is necessary.
IMPORTANT
NOTES
–
1) The global balance equations do not require the assumption of a continuous
medium. They can be applied to any domain D constituted by matter, continuous or
discontinuous: diverse media containing many different phases (liquid flows with
bubbles, fluidized beds for transport of pulverized media, etc.), avalanches of solid
substances (stones, snow, mud, etc.) and/or liquids (mud, etc.). The balance equation
can be directly written without assumptions concerning the differentiable properties
of the continuous medium. Only the assumption of integrability of the fluxes across
the surface 6 is necessary. Even the time differentiation assumption is not necessary:
it suffices to effect a balance of the amount of G contained in D between two
instants t
1
and t
2
:
21
12
andbetween
processother by
fromout come
in created
convectionby
fromout come
)()(
ofquantity
tt
DtGtG
G
DD
6
6
When the quantity G is a vector (momentum), the same balance can be
performed on its moments.
184 Fundamentals of Fluid Mechanics and Transport Phenomena
2) In all modeling we must have a global extensive variable G(t) internal to the
domain D, defined from the amount of G contained in D, such that
³
q
w
w
D
dv
t
g
is
equal to
dt
dG
; equation [4.63] can thus be written in the form:
¿
¾
½
¯
®
¿
¾
½
¯
®
DD
into
of inputs
inside
ofsource
GG
dt
dG
4.5.2. Equation of mass conservation
The expression of mass conservation for a fluid of density
U
inside the domain
D during its movement, can be written from [4.62], with
U
g :
0
w
w
6
³
m
qdv
t
D
U
where
³
6
6
mm
dqq is the mass flux leaving the surface 6.
4.5.3. Volume balance
The elementary volume flux dq
v
crossing the surface ds is: dsnVdq
v
G
G
. . The
volume flux leaving the domain D can be written:
dv
x
u
dvVdivdq
i
i
v
³³³
w
w
6 DD
G
4.5.4. The momentum flux theorem
Applying formulae [4.8] and [3.33] to the quantity Vg
G
U
, we obtain the linear
momentum theorem:
¦
³³
w
w
¦
ijji
D
i
Fedsnuudv
t
u
U
U
or, expressing the elementary mass flux: dq
m
=
U
u
j
n
j
ds:
Fluid Dynamics Equations 185
or
i
im ext
i
D
mext
D
u
dv u dq F
t
V
dv V dq F
t
U
U
¦
¦
w
w
w
w
¦
³³
¦
³³
G
GG
[4.64]
Note that in a steady flow the balance depends only on the input-output of the
domain. In unsteady flows, the first term corresponds to an eventual accumulation of
momentum in D.
Thus, as discussed in section 3.2.1, we can also perform a balance using the
moments (angular momentum). This gives:
¦
³³
w
w
¦
extm
D
MdqVOMdv
t
V
OM
G
G
G
U
[4.65]
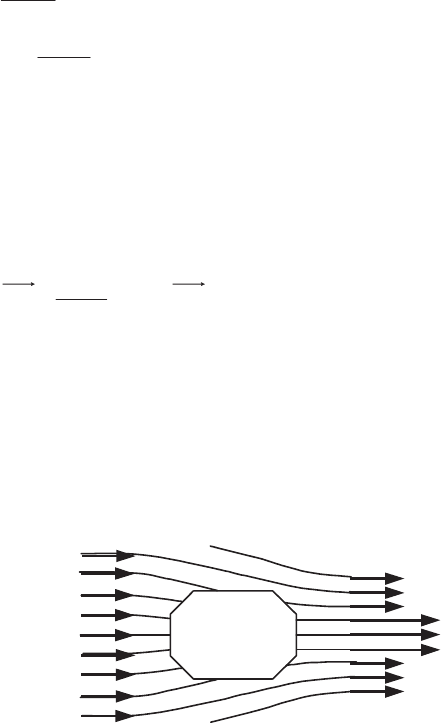
The momentum flux theorem is particularly useful in applications. Let us
consider as an example a propulsion device whose role is to increase the velocity of
the fluid which crosses it (a propeller, a turbine, a pump which produces a jet, etc.).
The reference frame is associated with the propulsion device, which is considered to
operate in a steady regime. We will ignore the details of the system and we will
consider the pressure to be constant on a surface 6 external to the device (Figure
4.5).
D
1
V
G
6
1
V
G
2
V
G
1
V
G
Figure 4.5.
Propeller thrust
Let q
m
be the mass flow rate passing through the propulsion device. The
momentum flow rate parallel to the upstream velocity
1
V
G
and leaving the surface 6
is equal to:
12
VVqdqV
mm
G
G
G
³
¦
186 Fundamentals of Fluid Mechanics and Transport Phenomena
The momentum flux is balanced by the thrust it generates, equal to
12
VVqP
m
G
G
.
Let us note that conservation of mass flow rate demands the existence of an
incoming mass flow through the lateral sides of surface
6
Very many practical problems can be treated in the same way, the changes in
momentum corresponding to the forces which can be calculated from a knowledge
of the mass flows and the velocities ([EVE 89], [GAR 06], [GUY 01], [SAG 66],
[SPU 97]).
4.5.5. Kinetic energy theorem
The global form can be obtained by integrating the local form [4.27] over the
domain
D
. Assuming a potential U which is independent of time, we obtain:
dv
x
udv
x
p
udsnuU
V
dv
V
t
dvU
V
dt
d
dvU
V
dt
d
j
ij
i
i
ijj
w
w
w
w
¸
¸
¹
·
¨
¨
©
§
¸
¸
¹
·
¨
¨
©
§
w
w
»
»
¼
º
«
«
¬
ª
¸
¸
¹
·
¨
¨
©
§
¸
¸
¹
·
¨
¨
©
§
³³³³
³³
6
W
U
U
UU
DDD
DD
22
22
22
22
We transform the terms of the right-hand side by introducing the divergence of a
vector, the corresponding integral being thus transformed into a surface integral
6
;
we obtain, after moving a pressure term to the left-hand side:
dv
x
u
dsnudv
x
u
p
dsnuU
V
p
dv
V
t
j
i
ij
j
j
ij
i
i
j
j
³³³
³³
w
w
w
w
»
»
¼
º
«
«
¬
ª
¹
·
¨
¨
©
§
¹
·
¨
¨
©
§
w
w
6
6
DD
D
W
W
U
U
22
22
The first term describes the accumulation of kinetic energy in D in the
transitional regime. The sum of this term and the following terms of mechanical
energy flux (kinetic and pressure) across
6
is equal to the sum of the power P
Q6
of
the external viscous stresses exerted on
6
of the power of pressure forces in D, and
of the power –P
Q
D
dissipated in D by viscosity (dissipation function [4.52]):

Fluid Dynamics Equations 187
D
D
D
vv
i
i
j
j
P
P
dv
x
u
p
dsnuU
V
p
dv
V
t
w
w
»
»
¼
º
«
«
¬
ª
¹
·
¨
¨
©
§
¹
·
¨
¨
©
§
w
w
6
6
³
³³
22
22
U
U
[4.66]
with: dsnuP
jjij
³
6
6
W
Q
the external power provided by the viscosity on
6
;
dv
x
u
P
j
i
ij
³
w
w
D
D
W
Q
the power dissipated in D by viscous friction.
In incompressible flows, we obtain:
D
D
vvjj
PPdsnu
V
ghpdv
V
t
¸
¸
¹
·
¨
¨
©
§
¸
¸
¹
·
¨
¨
©
§
w
w
6
6
³³
22
22
U
U
U
[4.67]
In incompressible inviscid flows, the sum of the temporal variation in the kinetic
energy of D and the flux of mechanical energy (kinetic and pressure) leaving
6
is
zero.
4.5.6. The energy equation
The integration of the energy equation over the domain D should be performed
using a form of the equation which allows flux integrals to be written. For a
compressible fluid, the form [4.48] with the total enthalpy is best suited. We obtain,
neglecting gravity:
³³³
³³
66
6
¸
¸
¹
·
¨
¨
©
§
»
»
¼
º
«
«
¬
ª
¸
¸
¹
·
¨
¨
©
§
w
w
dsnqdvdsnu
dsnu
V
hdv
V
e
t
jTjTjiij
jj
D
D
VW
UU
22
22
[4.68]
This equation has the inconvenience of not including the dissipation function
)
.
Using one of the forms [4.51] is preferable for thermodynamic balances, but internal
balances remain in the form of volume integrals:
dsnqdvdvdv
x
p
u
t
p
hdqdv
t
h
jTjG
j
jm
³³³³³³
¦
6
)
¸
¸
¹
·
¨
¨
©
§
w
w
w
w
w
w
qq
DDDD
V
U

188 Fundamentals of Fluid Mechanics and Transport Phenomena
However, these forms become perfectly adapted for the study of thermal transfer
at constant pressure or constant volume. In such conditions, we immediately obtain,
from [4.51]:
dsnqdvdv
g
dqdv
t
g
j
TjTm
³³³³³
¦
6
)
w
w
q
DDD
V
U
[4.69]
where for
g we must use either the specific internal energy e or the specific enthalpy
h. For the usual case of a fluid of constant specific heat, the preceding equation
becomes:
dsnqdvdvCTdqdv
t
CT
jTjTm
³³³³³
¦
6
)
w
w
q
DDD
VU
U
)(
[4.70]
where, depending on the case, we used either
C
p
or
C
v
for the specific heat C.
4.5.7. The balance equation for chemical species
Equation [4.58] can be immediately integrated to give:
dsnqdvdsnVdv
t
mimii
i
G
G
G
G
³³³³
¦6
w
w
q
DD
VU
U
. [4.71]
For a binary isothermal mixture, using [4.59] we obtain:
dsgradnDdvdsnVdv
t
i
mii
i
³³³³
¦
6
¸
¸
¹
·
¨
¨
©
§
w
w
q
U
U
UVU
U
..
GG
G
DD
[4.72]
In the case of weak concentrations, balance equation [4.60] gives:
dscgradnDdvdsnVcdv
t
c
icii
i
³³³³
¦6
w
w
q
..
G
G
G
DD
V
[4.73]
Homogenous or heterogeneous chemical reactions must be specified
accordingly, in the volume source term
dv
ci
³
D
V
or in the flux term
dscgradnD
i
³
¦
.
G
at the walls. In general, chemical reactions also lead to heat
release, which must be accounted for in a source term of the energy equation
([BIR 01]).
Fluid Dynamics Equations 189
4.6. Similarity and non-dimensional parameters
4.6.1. Principles
4.6.1.1. Invariance of physical laws
The fundamental physical laws are described by relations between real numbers
obtained as measures of physical quantities. A measurement is a comparison
between the quantity studied and a similar quantity considered as a unit. Most units
are “derived”, as they depend on physical laws: for example, the surface unit is a
square whose sides measure one unit of length. With such choices, physical laws are
relations which comprise either dimensional coefficients which have a physical
interpretation (the speed of sound for example) and which can be expressed using
the units of the system, or non-dimensional coefficients which are independent of
the system (for example,
S
for the surface of a circle). There are four fundamental
units which can be arbitrarily taken for mass, length, time and temperature, and
whose choice determines a coherent system of units. No specific physical
phenomenon is used to govern the particular quantities chosen as units, and for
practical reasons we can arbitrarily choose the four fundamental units of the
international metric system (the meter, the kilogram, the second, the Kelvin).
With these choices, the mathematical relations representing physical phenomena
are true regardless of the fundamental units which are used, which means that they
possess an invariance with respect to changes in the units which are used,
transformations which form a group. A given physical problem thus has an infinity
of equivalent numerical representations. Similarly, a numerical problem can
represent many different physical problems obtained using different systems of
units.
4.6.1.2.
Similar problems
A given problem must be repeatable, meaning that its definition must always
lead to identical results, within a certain margin of error. The problem is only
defined if analysis of the phenomena involved has been correct and complete, in
other words if we know the partial differential equations, and the boundary and
initial conditions which define the problem.
Two problems are similar if two systems of units exist, such that the
measurements of all the quantities of one of the problems, using a given system of
units, are equal to the measurements of the corresponding quantities of the other
problem using another system of units.
The conditions for similarity may be obtained by searching for conditions in
which the two problems will obey the same ensemble of equations and boundary
conditions after an appropriate change of the system of units. Because we can
190 Fundamentals of Fluid Mechanics and Transport Phenomena
choose the system of units, it is easier to write the equations and conditions of a
problem with a system of units which corresponds to the problem; the equations
obtained are non-dimensional, in other words they are independent of the units
chosen for the physical measurements: calculating the area of a circle using its
radius as a unit gives a result equal to
S
.
4.6.1.3.
Non-dimensional study of a problem
4.6.1.3.1.
Dynamic and thermal problem
We will now consider a flow problem involving a perfect gas with heat transfer
around a circular obstacle of diameter L. We will assume that the specific heat C
p
,
the viscosity
P
, and the thermal conductivity
O
are constant. The problem is posed in
the following manner:
– mass conservation:
0
w
w
w
w
i
i
x
u
t
UU
[4.74]
– Navier-Stokes equations:
)3,2,1,(
2
ww
w
w
w
w
w
ji
xx
u
x
z
g
x
p
dt
du
jj
i
ii
i
PUU
[4.75]
– energy equation (in enthalpic form) and the equation of state:
rT
p
xx
T
dt
dp
dt
dT
C
jj
ijp
ww
w
)
U
OHU
2
[4.76]
– dynamic boundary conditions:
- on the body surface, velocity equal to zero:
0
i
u ;
- at infinity, velocity
V
G
equal to U
G
;
- eventually a free surface condition on a horizontal surface;
– thermal boundary conditions:
- constant temperature
T equal to T
w
on the surface;
- constant temperature equal to
T
O
at infinity;
Fluid Dynamics Equations 191
– initial conditions:
- the fluid is assumed at rest at time
t=0.
The results of the problem are the velocity field, the pressure field and the
temperature field, in addition to the surface densities (friction stresses, pressure,
thermal flux density, etc.) or the global quantities (drag, lift, heat flux, etc.). These
are functions of the coordinates and the
n data of the problem (U, L,
U
0
, T
0
, T
w
,
P
,
O
, g, C
p
).
4.6.1.3.2.
Non-dimensional equations
For the fundamental units we will use the data of the problem such as the
velocity
U, the length L and the reference density
U
0
, and we will define the
following non-dimensional variables:
00
2
0
~
~
~
~~~
T
T
T
L
Ut
t
U
p
p
U
u
u
L
x
x
i
i
i
i
U
U
U
U
[4.77]
We have:
'
ww
w
ww
w
'
w
w
w
w
~
1
~~
1
;
~
;
~
1
2
2
2
2
L
xx
L
xxtd
d
L
U
dt
d
xLx
iijjii
Performing variable change [4.77] in equations [4.74] to [4.76] and dividing by
the dimensional coefficients of the left-hand side in each equation leads to the
following reduced equations:
T
U
rT
p
xx
T
ULCLTC
U
td
pd
TC
U
td
Td
ji
xx
u
ULx
z
U
gL
x
p
td
ud
x
u
t
jjp
ij
pp
jj
ij
ii
i
i
i
~
~
~
~~
~
~
~
~
~
~
~
~
)3,2,1,(
~~
~
~
~
~
~
~
~
~
~
0
~
~
~
~
~
2
0
2
000
2
2
0
2
ww
w
)
ww
w
w
w
w
w
w
w
w
w
U
U
O
H
U
P
U
U
P
UU
U
U
[4.78]
192 Fundamentals of Fluid Mechanics and Transport Phenomena
where the dissipation function
ij
H
)
(which characterizes kinetic heating) is given
by [4.56]:
ijij
L
U
H
P
H
~
~
2
2
) ) .
The preceding equations contain the following (non-dimensional) similarity
parameters:
0
00
p
22
p0 0
Reynolds number: Re
Froude number: Fr U
Mach number: M U
Prandtl number: Pr C
Eckert number: Ec U C 1 M
A
UL μ
g
c
μ
T
which allows us to write [4.78] in the form:
T
p
xx
u
td
pd
td
Td
ji
xx
u
Rex
z
x
p
td
ud
x
u
t
jj
ij
ij
jj
ij
ii
i
i
i
~
M
1
~
~
~~
~
Re.Pr
1
~
~
Re
Ec
~
~
Ec
~
~
~
)3,2,1,(
~~
~
1
~
~
Fr
1
~
~
~
~
~
~
0
~
~
~
~
~
2
0
2
2
2
J
U
HU
UU
U
U
ww
w
)
ww
w
w
w
w
w
w
w
w
w
[4.79]
The Eckert number and the Mach number are related by the perfect gas relation
between C
p
and r (
p
Cr 1
JJ
). We also define the Péclet number Pe = Re. Pr.
These similarity parameters characterize the importance of the non-dimensional
terms by reference to the terms of the left-hand side of the non-dimensional
equations. For example, we can write:
