Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations

Подождите немного. Документ загружается.

118 HYPERBOLIC EQUATIONS

*4.5.3

The telegraph equation

By the use of the exponential transformation mentioned at the end of §4.2.3, the

telegraph equation can always be considered in the form

202u 82u

8x2

8t2 -

_ 0,

(4.50)

where c > 0 in practical problems, and the Cauchy problem with u and 8u/8t

prescribed for all x at t = 0 is suitable for a Fourier transform. When u is zero

initially, the analogue of (4.36) is

u = v0(k)

sin (

aok2 + c

)

t, (4.51)

aok2 + c

\\\\

the apparent branch points at k = ±if /ao being illusory (removable). The details

of the identification of the Fourier inversion of (4.51) with the Riemann function

representation of the solution derived in §4.2.3 are given in Exercise 4.12, but we

can give a quicker way of removing the guesswork used in deriving (4.15). Taking

= q = 0, we seek a Riemann function directly, satisfying

y + R = a(x)b(y)

with R non-zero only in x 5 0, y < 0. Then the Fourier transform of R with

respect to x satisfies

and so

O +

= ka(y)

iv/k

where H is the Heaviside function. Hence

R(x, y)

iH(-y)

e-ikx-iv/k

27r

a k=constant<0

the inversion contour being chosen as described in §4.4. This integral clearly van-

ishes when x > 0, by closing the contour in !a k < 0, but if x < 0 we can write it

iH(-y) f

-; =vik'+l/k'l We

21r

k'=constant<O

Now we deform the contour into the unit circle around the origin so that, when x

and y are both negative,

x, y) =

f2r

-Zi

Ja (2v

R(x

) --

It is interesting at this stage to recall our discussion at the end of §4.2.2 about

the propagation of discontinuities in the Riemann function. For the wave equation,

APPLICATIONS TO WAVE EQUATIONS 119

R = 1 in x <, 0, y < 0 which, by differentiation, means that solutions are possible

in which u is precisely a sum of delta functions on x = 0 and y = 0. For the

telegraph equation, however, such delta functions can only propagate if there is a

non-zero wave field between the characteristics x = 0 and y = 0. We will discuss

this point further in the next section and in Exercise 4.12.

*4.5.4

Waves in periodic media

The study of the solutions of

02U ,

8t2 =

ao(x)(92U

(4.52)

where as is periodic in x, is important for many practical problems, for example

electromagnetic wave propagation in crystalline solids such as semiconductors. The

relevant spatial eigenvalue problem, when u is harmonic in time with frequency w,

dx2d+ awo(x)

k=0,

(4.53)

with w real, so there are few explicit solutions for such problems. Nonetheless,

(4.53) is susceptible to Floquet theory (2], which roughly speaking says that as w

increases the qualitative behaviour of X alternates; when w lies in some intervals,

called stop bands, the two independent solutions of (4.53) either grow or decay

as jxI -+ oo, but when w lies in the complementary `pass band' intervals these

eigensolutions are all quasiperiodic in x, and are hence physically acceptable for

problems in which (4.52) holds in -oo < x < oo. It is only at the boundaries

between the pass and stop bands that X can be periodic. Hence, even without

an explicit solution in front of us, we can see a new phenomenon that can occur

for hyperbolic equations: waves may be able to propagate along characteristics

but their spatial penetration may be much greater when frequencies lie in certain

bands than in others. In fact, such dispersive behaviour is quite general, because,

even for the telegraph equation (4.50), which has constant coefficients, waves with

frequency w are such that the wavenumber

k =

2 -c,

*4.5.5 General remarks

Continuing to catalogue examples like this would entrain us ever deeper into

problem-specific technicalities. However, all the examples we could have displayed

would reveal conformity with the predictions of the Riemann function represen-

tation (4.14). They all have domains of dependence and regions of influence, the

only example that might have given us pause for thought in this respect being the

telegraph equation (4.50). This equation does admit solutions of the form

u =

Rek(z-v:),

120

HYPERBOLIC EQUATIONS

where

(4.54)

and, in the physically realisable case c > 0, this appears to predict the propagation

of waves travelling faster than the characteristic speed ao. However, what happens

in any practical initial value problem where, say, Cauchy data at t = 0 has compact

support (i.e. vanishes outside a finite range of x) is that the Fourier components

with wavenumber k can disperse, i.e. rearrange themselves within the region of

influence of the Cauchy data at a speed greater than ao; the representation (4.14)

shows that none of this rearrangement is felt outside this region of influence.

Another way of looking at this is to say that the discontinuities that inevitably

occur on the boundary of the region of influence are described by very large values

of k and, from (4.54), this boundary has speed ao.

This raises the general question of the way in which the solution sorts itself

out within the region of influence of the Cauchy data. We can see from Exer-

cise 4.22 that, for the one-dimensional wave equation (4.1) (and hence the three-

dimensional radially-symmetric equation (4.49)), any initially localised data for u

with 8u/8t (x, 0) = 0 gives a response that varies just in the vicinity of the char-

acteristics x = tact emanating from the source. Our region of influence argument

led us to anticipate that there is no disturbance in x > aot or x < -aot, but it

did not reveal that in between these characteristics there is a `zone of silence' in

which u is zero (in three dimensions, u vanishes in 0 < r < aot). However, when

we consider the circularly-symmetric wave equation (4.42) or the telegraph equa-

tion (4.50) with localised Cauchy data, we can show that the solution is non-zero

(almost) everywhere in the region 0 < r < aot (and this is borne out by the mo-

tion of a leaf floating on a pond when a stone is thrown nearby). We have already

hinted at this at the end of §4.5.3, and we will have more to say about it in the

next section.

4.6

Wave equations with more than two independent variables

Turning to wave equations with three or more independent variables, there are two

related ideas that we have already touched upon but wish to discuss in greater

detail in this section before we frame our notion of hyperbolicity in this more

general case.

4.6.1 The method of descent and Huygens' principle

The ideas of this section are best illustrated by the now-familiar acoustic model

8ta = a0Vzu (4.55)

in three space dimensions, for which we have shown via (4.49) that the general

radially-symmetric incoming and outgoing solutions are

u = I F(r ± aot),

(4.56)

MORE THAN TWO INDEPENDENT VARIABLES 121

respectively, when r is a spherical polar coordinate. These solutions all decrease as

r increases with r ± aot fixed, which reflects the merciful spreading out of sound

waves in three-dimensional space; they are all also singular at r = 0, representing

the possibility of focusing, which is absent in one-dimensional problems. Alas, no

representation as simple as (4.56) exists in two space dimensions, for reasons which

will soon become apparent. However, we can use (4.56) to generate the explicit

solution of the general Cauchy problem45 in which

u = uo(x), at = vo(x) at t = 0, (4.57)

where x = (x, y, z). As in (4.41), we proceed by superimposing weighted solutions

of the form (4.56) in the case where F is localised and, by causality, we only take

outgoing waves; in other words we write

u(x) y, z, t) =

r f r b(r r aot) f(x

, y, z') dx' dy' dz', (4.58)

where r2 = (x-x')2+(y-y')2

J+J(z-z')2,

which clearly satisfies (4.55) for arbitrary

weight functions f. The presence of the delta function in the integral ensures that

we need only account for values of f on the surface of a sphere with the centre

(x, y, z) and radius aot. Hence, changing to spherical polar coordinates (r, 0, 0)

and integrating with respect to r, we obtain a surface integral over the sphere of

radius aot. Remembering that the surface element is aot2 sin 9 dO d¢, we obtain

u = 4rraot Cf,

where

Cf= 1 f(x+aotsin0cosO,y+aotsin0sinO,z+aotcos0)sin9d9do.

(4.59)

For this retarded potential solution, it is clear that

u=0 att=0 and

8uI

= 47raoG f Jt_o = 4aaof(x,y,z),

t=o

by direct integration. Hence, setting f = vo/(47rao) gives us the solution to (4.57)

when uo = 0. However, we can solve the complete Cauchy problem by making the

crafty observation that if u satisfies (4.55) then so does Ou/8t. Thus, 818t (tC f )

is a solution of (4.55) for all t > 0 and, by the argument above, its value at

t = 0 is 4aaof; simply taking f = (1/41rao)uo enables the first part of (4.57) to

be satisfied. Finally, we note that 02/&2 (t C f) = as t V 2 C f

, since t C f satisfies

(4.55), and hence vanishes at t = 0. Thus, we have solved the Cauchy problem in

the form

u=tCvo+&(tCuo).

45This problem can be shown to have a unique solution by an energy argument similar to that

in Exercise 4.13.

122

HYPERBOLIC EQUATIONS

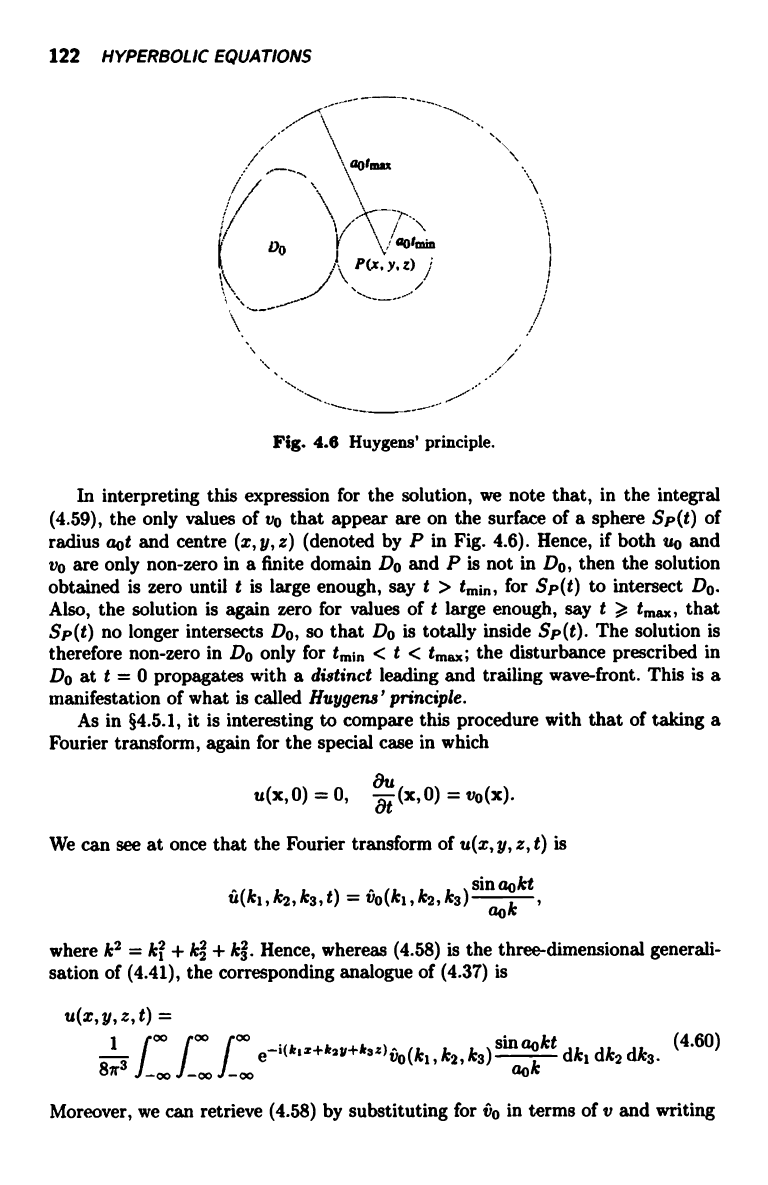
Fig. 4.6 Huygens' principle.

In interpreting this expression for the solution, we note that, in the integral

(4.59), the only values of vo that appear are on the surface of a sphere Sp(t) of

radius aot and centre (x, y, z) (denoted by P in Fig. 4.6). Hence, if both uo and

vo are only non-zero in a finite domain Do and P is not in Do, then the solution

obtained is zero until t is large enough, say t > t,, ,,, for Sp(t) to intersect Do.

Also, the solution is again zero for values of t large enough, say t > t,", that

Sp(t) no longer intersects Do, so that Do is totally inside Sp(t). The solution is

therefore non-zero in Do only for tmin < t < tmax; the disturbance prescribed in

Do at t = 0 propagates with a distinct leading and trailing wave-front. This is a

manifestation of what is called Huygens' principle.

As in §4.5.1, it is interesting to compare this procedure with that of taking a

Fourier transform, again for the special case in which

u(X,0) = 0, 8t(X,0) = vo(X)

We can see at once that the Fourier transform of u(x, y, z, t) is

u(ki, k2, k3, t) = vo(ki, k2, ka)

sin aokt

aok

where k2 = kl + k2 + k3. Hence, whereas (4.58) is the three-dimensional generali-

sation of (4.41), the corresponding analogue of (4.37) is

u(x,y,z,t) =

I. f

-,(k,.+k2y+k,z)

sin akkt

(4.60)

vo(kl, k2, k3)

dkl dk2 dks.

Moreover, we can retrieve (4.58) by substituting for vo in terms of v and writing

MORE THAN TWO INDEPENDENT VARIABLES 123

u(x,y,z,t) _

8x3ao

vo(x',y',z') (JJf

sinaokta-'k''COseksin6d9dOdk) dx'dy'dz',

where r2 = (x-x')2+(y-y')2+(z-z')2 and (k, 0, 0) are spherical polar coordinates

centred at k1 = k2 = k3 = 0. Hence, integrating with respect to 9 and 0,

in ao Tt sin kr

1 , , ,

s4x1

/ / / , , ,

(f-,

u(x, y, z, t) =

J f f vo(x , y , z)

dk) dx dy dz

JJJ

(x',y',z')6(r

rant)dx'dy'dz'.

To obtain the solution for a two-dimensional initial value problem we may

use the method of descent on the result (4.59). If we assume that uo and vo are

independent of z and Do is an infinite cylinder with generators in the z direction,

then

102x

Gvo =

vo(x+aotsin0cos¢, y+aotsin9sin0)sin9d0dO.

With the substitution p = aotsin0, so that IpI < a0t,

/2a

aot

pdpdO

Gvo =

Zxaot

vo(x + p

y + pain 0) (a8t2 -e)112'

and p and 0 are two-dimensional polar coordinates. Hence, in Cartesian coordi-

nates = p cos t¢ + x and , = p sin 0 + y, this reduces to

,CVO _

f r

r!) dk dri

(4.61)

21raotJJ (a02t2-(x-f)2-(y-q)2)'/2,

where the integration is now over IpI < aot. If the initial data is defined and non-

zero on a region A0 (the two-dimensional cross-section of Do) then, for all t > train,

the interior of the circle of radius aot intersects A0, and the integral (4.61) is non-

zero. This ties in with our remark at the end of §4.5.5 about leaves floating on

the surface of a shallow pond, and it is consistent with the prolonged rumbling

heard after the initial clap of thunder caused by lightning strikes which, although

jagged, are sufficiently elongated to generate an approximately two-dimensional

sound field.

A further application of the method of descent reduces (4.61) to the d'Alembert

formula and both leading and trailing wave-fronts occur In one space dimension

for initial data with compact support, i.e. vanishing outside a finite interval.

We remark that the ideas above can also be used on inhomogeneous wave

equations of the form

8t2 -

aoV2u = f (X, t). (4.62)

As observed by Duhamel, if we let v(x, t, r) be the solution of the homogeneous

equation (f =- 0) with v = 0 and Ov/8t = f (x, r) at t = r, solve for v using (4.59),

124

HYPERBOLIC EQUATIONS

and set u = fo v(x, t, z) dr, we satisfy (4.62) with zero Cauchy data. This simply

reflects the fact that the source term f is equivalent to a superposition of `pulsed'

initial value problems.

It is interesting to compare the ease with which (4.61) can be obtained by the

method of descent with the difficulty that confronts a Fourier analysis. Suppose,

for example, that we consider the problem in two space dimensions with u = 0

and 8u/8t = vo at t = 0, and use the two-dimensional Fourier transform

u(x, y, t)ei(k1:+k2y) dx dy.u(ki , k2, t) =

(4.63)

As in the three-dimensional case, we find that

u(k,t) =

vo(k,t)smkkt,

(4.64)

where k2 = kl +k2. Following (4.36) and Exercise 4.8, and (4.60), we can write u in

the

form

convolution

integral if

can invert the

function

sin(aokt)/aok. This immediately forces us into delicate convergence questions of

the type mentioned in §4.4. One hair-raising possibility is to use the imaginary

part of the following blind assertion: with p2 = x2 + y2,

froo foo eiaokte i(k,s+kZy)

foo

dkt dk2 = -

eik(aot-pcog0) dk d9

00 00

aok

ao 0

c2w

- ao Jo aot - pcos9

f2,ri(aov1at2_p2)',

p < aot,

0, p > aot.

(4.65)

Squeamish readers may prefer to work backwards from the answer and check that

the double Fourier transform of (4.65) is

21ri

2wfaot

peikpcoa9

dpdB, (4.66)

aot2 - p2

which is an integral that can, with the use of tables [19], be reduced to eiaokt/aok

The discussion above, like that at the end of the preceding section, raises the

general question of identifying how much of the region of influence of any com-

pactly supported Cauchy data is actually excited when we are simply considering

the wave equation (4.55). It is tempting to conjecture that in one and three dimen-

sions the solution always has a sharp `beginning' on the outgoing characteristic

surfaces through the boundary of the support, and a sharp `conclusion' according

to Fig. 4.6; we get no such sharp conclusions in two dimensions. While, strictly

speaking, this statement is true, it is important to remember that it only applies

MORE THAN TWO INDEPENDENT VARIABLES 125

to the constant-coefficient wave equation (4.55). Any inhomogeneity such as a

spatial variation in ao probably destroys the property that the conclusion of the

disturbance is sharp.

We conclude this section by mentioning how Fourier transforms lead to yet

another interesting representation of solutions of the wave equation. In all the

cases we have considered with Cauchy data u = 0 and Ou/8t = vo at t = 0,46 we

have found that the Fourier transform of u is a = vo(sin aokt)/(aok). As described

after (4.36) and in Exercise 4.8, this gives the explicit one-dimensional solution as

iks -

sin a0kt

u(x,t) e-

vo(k)

! oo

a0k

= 2ao (Vo(x + aot) - 4o(x - aot)), (4.67)

where Vo(x) = vo(x).

However, in two dimensions, instead of (4.65), we can write

(x, y, t) =

foo

(e-"'(' coa O+Vsin ¢)-iaokt

-e-ik(scosm+Vsin43)+iaokt)

F(k,0)dkdo,

where kl = k cos ¢, k2 = k sin O and F = vo/(47r2aok). Thus, when we integrate

with respect to k, we have a Fourier inversion with arguments z cos '+y sin 0f aot.

Hence,

u(x,y,t) = f (F(xcos0+ysin0+aot,¢) -F(xcos0+ysin¢-aot,O))do.

(4.68)

While this 'plane-wave' superposition is less useful than (4.61) for solving initial

value problems, it is interesting that it involves just one integral of an arbitrary

function of two variables.

Finally, in three dimensions, the obvious generalisation of (4.68) is the plane-

wave superposition

fo2w

r2w

u(x,y,z,t)=

(F(xsinocos9+ysin¢sin9+zcos0+aot,0,

- F(x sin 0 cos 0 + y sin 0 sin 0 + z cos 0 - aot, 0)) do d9.

(4.69)

4.6.2 Hyperbolicity and time-likeness

In §4.3 we have already made some remarks about the way in which the idea

of time-like directions can be introduced mathematically into the theory of ini-

tial/boundary value problems in one space dimension in order to suggest well-

posed data. In more space dimensions, such considerations have greater physical

46Remember we can consider more general initial value problems using the ideas of p. 121.

126

HYPERBOLIC EQUATIONS

(a)

Vy2

++ z2

(b)

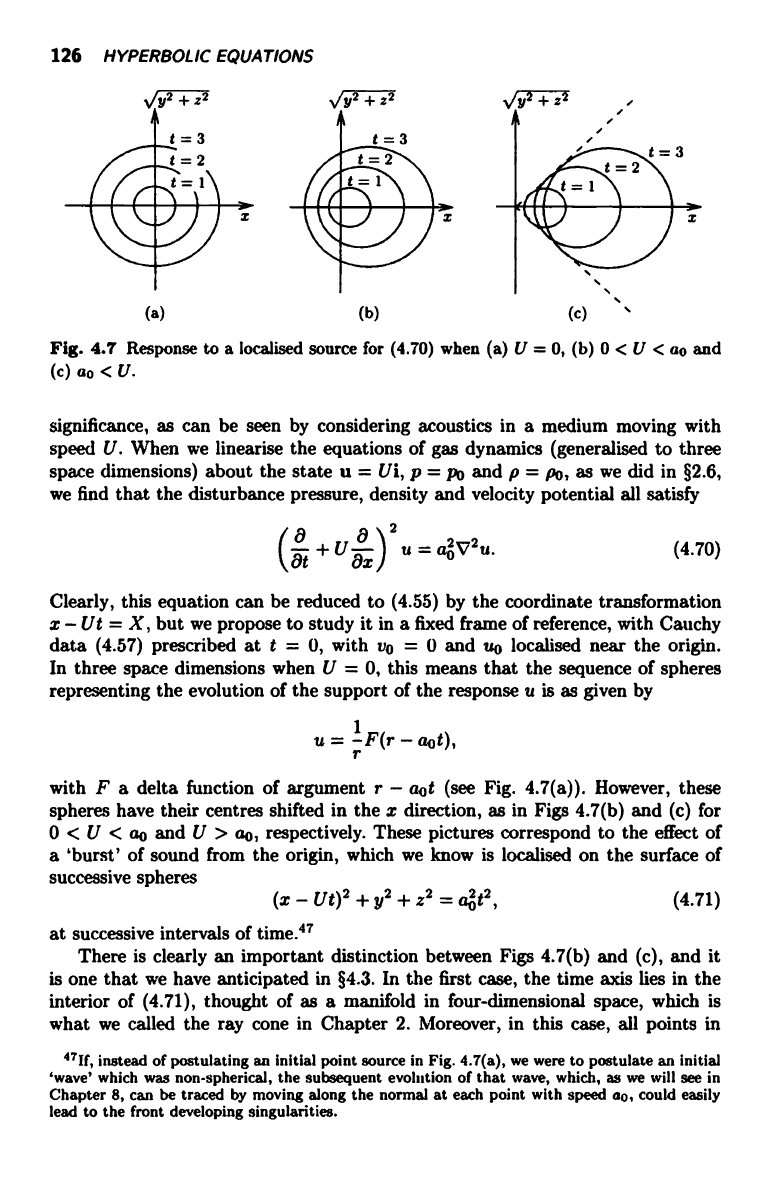
Fig. 4.7 Response to a localised source for (4.70) when (a) U = 0, (b) 0 < U < ao and

significance, as can be seen by considering acoustics in a medium moving with

speed U. When we linearise the equations of gas dynamics (generalised to three

space dimensions) about the state u = Ui, p = po and p = po, as we did in §2.6,

we find that the disturbance pressure, density and velocity potential all satisfy

)2U

Uax = aov2u.

(4.70)

Clearly, this equation can be reduced to (4.55) by the coordinate transformation

x - Ut = X, but we propose to study it in a fixed frame of reference, with Cauchy

data (4.57) prescribed at t = 0, with vo = 0 and uo localised near the origin.

In three space dimensions when U = 0, this means that the sequence of spheres

representing the evolution of the support of the response u is as given by

u = 1 F(r

- aot),

with F a delta function of argument r - aot (see Fig. 4.7(a)). However, these

spheres have their centres shifted in the x direction, as in Figs 4.7(b) and (c) for

0 < U < ao and U > ao, respectively. These pictures correspond to the effect of

a `burst' of sound from the origin, which we know is localised on the surface of

successive spheres

(x - Ut)2 + y2 + z2 = aot2, (4.71)

at successive intervals of time.47

There is clearly an important distinction between Figs 4.7(b) and (c), and it

is one that we have anticipated in §4.3. In the first case, the time axis lies in the

interior of (4.71), thought of as a manifold in four-dimensional space, which is

what we called the ray cone in Chapter 2. Moreover, in this case, all points in

47If, instead of postulating an initial point source in Fig. 4.7(a), we were to postulate an initial

'wave' which was non-spherical, the subsequent evolution of that wave, which, as we will see in

Chapter 8, can be traced by moving along the normal at each point with speed ao, could easily

lead to the front developing singularities.

MORE THAN TWO INDEPENDENT VARIABLES 127

(a) (b)

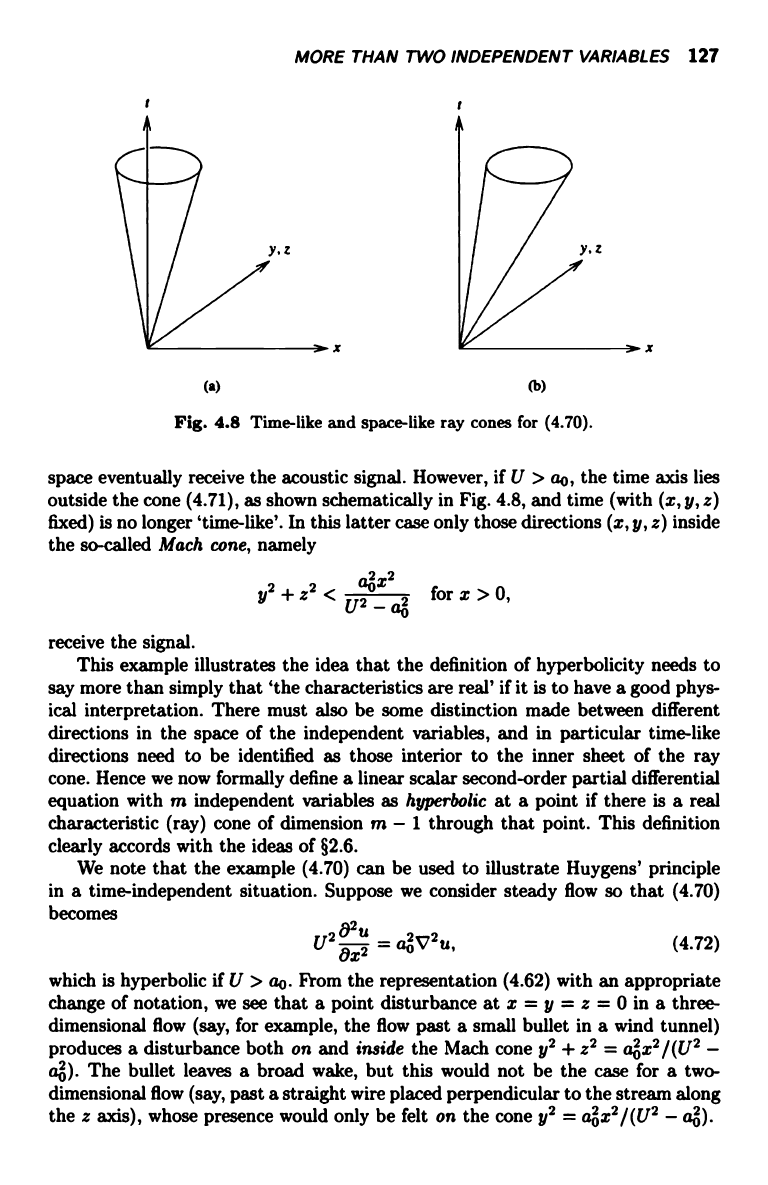
Fig. 4.8 Time-like and space-like ray cones for (4.70).

space eventually receive the acoustic signal. However, if U > ao, the time axis lies

outside the cone (4.71), as shown schematically in Fig. 4.8, and time (with (x, y, z)

fixed) is no longer 'time-like'. In this latter case only those directions (x, y, z) inside

the so-called Mach cone, namely

2x2

+ z

< (p

forx>0,

receive the signal.

This example illustrates the idea that the definition of hyperbolicity needs to

say more than simply that `the characteristics are real' if it is to have a good phys-

ical interpretation. There must also be some distinction made between different

directions in the space of the independent variables, and in particular time-like

directions need to be identified as those interior to the inner sheet of the ray

cone. Hence we now formally define a linear scalar second-order partial differential

equation with m independent variables as hyperbolic at a point if there is a real

characteristic (ray) cone of dimension m - 1 through that point. This definition

clearly accords with the ideas of §2.6.

We note that the example (4.70) can be used to illustrate Huygens' principle

in a time-independent situation. Suppose we consider steady flow so that (4.70)

becomes

U202"

= a4V2u,

(4.72)

which is hyperbolic if U > ao. From the representation (4.62) with an appropriate

change of notation, we see that a point disturbance at x = y = z = 0 in a three-

dimensional flow (say, for example, the flow past a small bullet in a wind tunnel)

produces a disturbance both on and inside the Mach cone y2 + z2 = aox2/(U2 -

ao). The bullet leaves a broad wake, but this would not be the case for a two-

dimensional flow (say, past a straight wire placed perpendicular to the stream along

the z axis), whose presence would only be felt on the cone y2 = aox2/(U2 - ao).