Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations

Подождите немного. Документ загружается.

108

HYPERBOLIC EQUATIONS

the equation is to be solved. This can be proved when the eigenvalue problem

is set on a finite interval and there are no singularities in the coefficients in

,C; this is Sturm-Liouville theory. Moreover, when the interval is infinite or

semi-infinite, or when C has singular coefficients and has eigenvalues Ak labelled

by the continuous parameter k and corresponding orthonormal eigenfunctions

O(x, k), we need to consider the continuous transform

j (k) = / f (x)b(x, k) dx, f (x) = f

f (k)c(x, k) dk,

(4.26)

in which the range of the k integration is not always obvious. (Note that, when

we normalise the exponential in (4.22) by multiplying by 1/

27r, and corre-

spondingly replace the factor 1/27r in the inversion in (4.24) by 1/

27r, (4.21)

and (4.24) are almost special cases of (4.25) and (4.26), the only discrepancy

being in the sign of the arguments of the exponentials. We will resolve this

shortly.) When we come to use (4.25) and (4.26) to describe the solution of any

particular partial differential equation, we cannot overemphasise how important

it is that the x derivatives form a self-adjoint operator G. It is only when this

is the case that multiplying the equation L f = Akf by O(x, k) and integrating

over x gives the inner product relation

(O,Cf) = (Cm,f) = ak(0,f),

(4.27)

so that the transform of C f can be written in terms of the transform of f . In

the Fourier transform example above, Ak = -k2, each eigenvalue being double,

and the orthogonal eigenfunctions are a z.

Complex eigenfunctions. The operator C = d2/dx2 is the simplest example of

a self-adjoint operator, and this is reflected in the fact that its eigenvalues,

-072 /L2 in the discrete case and -k2 in the continuous case, are real. Also,

with our periodic boundary conditions, its eigenfunctions are orthogonal sine

and cosine functions and the Fourier series (4.21) is often written in terms of

these real functions; however, combining them into complex exponentials not

only saves space but also helps conceptually. We can now see why the normalised

versions of (4.21) and (4.24) referred to above do not quite agree with (4.25)

and (4.26). The difference results from the fact that (4.25) and (4.26) only apply

when ¢ (x) and O (x, k) are real; when they are complex, the inner products

need to be generalised to (f, g) = f f§ dx, where g is the complex conjugate of

g. Also, we need to normalise so that f

dx or f O(x, k) dx is

replaced by

unity, and (4.25) and (4.26) are then correspondinglyJf(x)(x)dx,

f (x) =

cn.O.(x)

with

c =

f (z) = f f (k)0(x, k) dk

with

1(k) = J f

k) dx.

The Laplace transform. Bearing in mind our encounter with causality in §4.3,

it is useful to be able to represent functions that are zero for negative time

TRANSFORMS AND EIGENFUNCTION EXPANSIONS 109

(here represented by x < 0). In that case it is convenient to write k = ip and

j (k) = j (p) to obtain, formally, the Laplace transform and its inverse,

1(p) = f ,* f

(x)e-v= dx,

f (x) =

tai

f f (p)egs dp.

(4.28)

We have deliberately left the range of integration in p undefined for reasons

which result from the serious convergence questions associated with the Fourier

transform and its inverse. Indeed, the former does not even work for f (x) = 1.

This is the most difficult and confusing aspect of Fourier analysis and it can be

approached in the following two ways.

The complex Fourier transform. The first procedure applies to the Fourier trans-

form in its standard form (4.22) and is expounded in [8]. As in (4.28), we begin

by considering functions that vanish in x < 0, and then we assume that they

grow like a°s, a > 0, as x -4 +oo (but not like

e0z'+`

fore > 0). Instead of try-

ing to find f (k), the basic idea is to consider the Fourier transform of f (x)e-`3s,

Q > a. The effect of introducing ,6 is exactly the same as that of complexifying

k in (4.22) and working on a contour !3 k > a, and this involves careful contour

integration (see Exercise 4.9). The principal result is that the inversion needs

to be taken along a contour which lies above all the singularities of j (k) in the

complex k plane. When the consequences are incorporated into the Laplace in-

version formula, the integral needs to be taken from y - ioo to y + ioo, where

the real number y is large enough for all the singularities of j (p) to lie to its left

in the complex p plane.

This procedure can equally be applied to functions that grow exponentially as

x -4 -oo and vanish in x > 0, allowing more general functions to be considered

by extending the argument of Exercise 4.9. However, functions that grow faster

than eos as (xj -+ oo can only be treated by more radical changes in the contour

integrals in (4.22). When faced with, say, f (x) = es', we define its transform as

F(C) = f e4ses3 dx,

where r is any contour starting at infinity in 5a/4 < argx < 7a/4 and ending

at infinity in a/4 < argx < 3a/4. We soon find that F(C) = i fe-('/°, and the

inversion formula is

1 f ive-('/°e-CZ d(,

TV-71

where f is again chosen so that the integral exists. Indeed, the representation

Ax) =

tai

I a-1Z F(C) dC

is a useful general technique for solving certain ordinary differential equations

(see Exercise 4.10).

110

HYPERBOLIC EQUATIONS

Fourier transforms and distributions. The second procedure is simply to permit

series such as (4.21) and integrals such as (4.22) to diverge in the usual sense

but to interpret them as generalised functions or distributions, as discussed on

p. 98. For example, the periodically extended function

f(x) _

-L < x < 0,

j1, O<x<L,

has the Fourier series

f (x) =

4 sin

(2n + 1)7rx

n=O

(2n 11),

Assuming that f'(x) = 25(x) in -L < x < L (the delta function comes from

the derivative of the jump at x = 0), it is tempting to write

00 4 (2n + 1)irx _

fl(X) _

L cos L

= 2

(-1)nb(x - n4

n=-00

Fortunately, this outrageous-looking statement is easy to justify. A series E Dn

of distributions is said to converge if the series of numbers E(Dn, 0) converges

in the usual sense whenever 0 is a test function. Term-by-term differentiation

is justified because E(D;,, ') = - E,(Dn,1("), and if 1' is a test function then

so is ii', so the right-hand side converges. All the difficulties associated with

the 'usual' convergence of series disappear here, because the test functions are

assumed to be so smooth.

This idea of defining properties of generalised functions by transferring them to

the test functions can be carried further to define the Fourier transform of a

distribution. The key here is the formal observation that, for functions f(z) and

9(x),

(f, 9) = foo V'0*0 f

(x)e'k: dx)

9(k) dk

I dx

CIO

= 1100 f (x)

9(k)edk\

= (f,9),

as long as the orders of integration can be changed (this is called Parseval's

formula).-This suggests that we define the Fourier transform of a distribution

D to be D(k), where

(b,,O) = (D, ),

with the corresponding formula

(D, 0) = (D, )

TRANSFORMS AND EIGENFUNCTION EXPANSIONS 111

for the inverse D(x), where j is the inverse Fourier transform of 0 as given

by (4.24). When this is done, a beautiful theory emerges in which most use-

ful distributions have suitably defined Fourier transforms that are themselves

distributions. This theory enables us easily to confirm results such as

b(k) = j00e'ozdx = 1, 1 =

e'dx

= 2rb(k),

and hence that the inversion formula for j(k)§(k) is

f (k)9(k)e

ik:

27r

1-00 Yoo

(y)eikv dy)

(L.

9(z)edzl

e= dk

f (y)9(z)6(x - y - z) dy dz

f(y)9(x - y) dy, (4.29)

the so-called convolution formula; the last integral is often written as f * 9.

Multidimensional Fourier transforms. We can formally generalise (4.22) to func-

tions f(x,y) by defining

0o ao

= f

1 f (x,

y)e'(k,:+k,v) dx

dy,

(4.30)

f (ki, k2)

ac a0

so that (4.23) suggests that

1-00

00 00

f (ki,

dkl dk2,

(4.31)

and the extension to more variables is obvious. Cases where the inversion can be

done explicitly are even rarer than for functions of one variable, and the question

of convergence becomes even more complicated. Nevertheless, we will see that

quite simple changes of variable in the integrals in (4.30) or (4.31) can sometimes

give us valuable insights. A spectacular example occurs when we consider f as

a function of k and 0, where kl = k cos 9 and k2 = k sin 0, so that f is equal to

f00

f (z, y)e'k(: cos a+v sin e) dx dy.

When we rotate the axes by writing

x = r cos 9 - t sin 9,

y = r sin 9 + t cos 9,

this becomes

112

HYPERBOLIC EQUATIONS

00 /00

e"k''f(rcos8-tsin9,rsin0+tcos8)drdt.

Hence, as a function of k and 0, f is equal to

J_0eikrfR(*,

0) dr,

where

f R (r, 0) = f f (r cos 0 - t sin 0, r sin 0 + t cos 0) dt. (4.32)

This is called the Radon transform of f (x, y) and it is fundamental to the anal-

ysis of CAT scanning tomography. Suppose we shine a thin beam of X-rays

through a two-dimensional body at an angle 0; the transmitted intensity is then

determined by the integral of the absorption coefficient f (x, y) along the beam,

that is by the Radon transform of f in this direction. Repeating the scan at

the same angle 0 along parallel beams for each value of the lateral coordinate

r, and then scanning again for all 0 < 0 < rr, we find fR(r, 8). Then, in order

to retrieve f (x, y), all we need to do is to take the Fourier transform in r of

fR(r,0), transform into Cartesian coordinates, and invert f.

We must emphasise one severe limitation on the use of distributions in multiple

Fourier transforms. This is that, while we can perfectly easily define the Fourier

transform of 6(x)6(y) as a product of two one-dimensional transforms (which

is hence equal to unity), there is no satisfactory definition of, say, a(x)a(x).

This remark applies to any product of distributions with the same `independent

variable' unless, of course, they are functions in the usual sense.

The transform integral regarded as an integral equation. We might consider an-

other approach to the problem of recovering a function from its transform: in-

stead of using the inversion formula, we might try to find f (x), given its trans-

form j (k), by solving

ff(x)cb(x,k)dz = 1(k)

as an integral equation for f (x). Now readers familiar with the theory of integral

equations will be aware that Fredholm equations of the first kind, in which a

function f(x) has to be determined such that

K(f](t) = f K(z, t)f (z) dx = 9(t),

(4.33)

for given K(z, t) and g(t), are usually ill-posed. One simple-minded reason for

this is that, if K is separable, so that K(x, t) =

a.(x)fl (t), where N < oo,

then (4.33) can never have a solution unless g(t) is a linear combination of 8, (t);

moreover, if any functions fe (x) exist such that fa K(z, t) f, (x) dx = 0, then,

even if f (z) exists, it is indeterminate to within a linear combination of these

functions. More generally, if K is not separable and fQ f6 IK(x,t)1adxdt < oo,

APPLICATIONS TO WAVE EQUATIONS 113

it is a standard result that, as long as a and b are finite, there exists an infinite

sequence of eigenvalues A. and a complete sequence of eigenfunctions f (x) such

that

An f K(x, t)ff(x) dx = fn(t),

and, moreover, A,, -p oo as n -+ oc. Because these eigenfunctions are complete,

every well-behaved function can be expanded as a generalised Fourier series in

terms of f,,. Now suppose that f (x) = F, a f,, (x); then the operator K is such

that

K[f](t) = E ! fn(t),

which is a series whose coefficients are ultimately smaller in modulus than the

original ones and thus represents a `smaller' function than the input f (x) (for

this reason such operators are called compact). Hence, if we try to solve (4.33) for

a compact operator IC by expanding g(t) = E b f (t) and equating coefficients,

we find a,, = A b,,. That is, f is less smooth than g, and so we can only hope

to find a solution if we restrict the class of possible right-hand sides g(t); this is

another indication of ill-posedness.

These observations might cause us to doubt the value of (4.24); the last thing we

want is for Fourier inversion to be ill-posed! However, we are reassured by the

facts that the kernel O(x, k) = e'lcx is not separable, the corresponding operator

IC has no null space and, most importantly, IC is not compact (to see this,

consider the `small' function f (x) =

ee_E!=l,

which converges uniformly to zero

as e --- 0; its Fourier transform is j (k) = 2e2/(e2 + k2) which, however, does not

tend uniformly to zero).

4.5 Applications to wave equations

From the practical point of view, the message that emerges from the discussion

of the previous section is that linear partial differential equations (hyperbolic or

otherwise) that are `symmetric' enough to have a spectrum of solutions (4.19)

can be solved by generalised Fourier methods, that the particular method will

depend on the problem in hand, and that technical pitfalls may well be encountered

concerning questions of convergence. With this in mind let us now consider some

simple hyperbolic problems.

4.5.1

The wave equation in one space dimension

Let us consider

02u

"2u

fort > 0.

8t2

8x2

(4.34)

We have already given the d'Alembert solution to the Cauchy problem with

data at t = 0 in (3.31) and Exercise 3.8, but the constancy of the coefficients in

(4.34) also makes it suitable for Fourier analysis. If, for example,

u=0,

8t =vo(x) att=0,-oo<x<oo,

114

HYPERBOLIC EQUATIONS

the range of the respective independent variables indicates that the problem is

amenable to a Fourier transform in x or a Laplace transform in t (or both). The

result of the former is

and so

d2 + aok2u = 0,

u(k,t) =

f(k)e'kaot

&)e-ikaot

(4.35)

for some f and y; incorporating the initial data we find

u(k, t) _

fo(k)

sin aokt, (4.36)

from which

u(x, t)

= 1

0o vo(k)

(e1k(aot-=)

e-ik(aot+s)) dk,

2aao

2ik `

(4.37)

with suitable assumptions about the behaviour of vo and vo. We can write this as

r00 0o

(aot-z) ik(aot)

u(x't)

v°() (1100

- e

2ik

and use the result from contour integration that, when v is real,

f00 eivk

dk = fir

for v > 0 and v < 0, respectively (no matter which way we indent the contour at

k = 0), to show that the integral with respect to k vanishes, unless x - aot < a <

x + aot when it is ar. Hence (4.37) is in accordance with the d'Alembert solution

stool

u(x,t) = 12(uo(x

- aot) + uo(x + aot)) +

vo(a)da,

(4.38)

Tao

-aot

which applies to this problem when u(x, 0) = no as well as Ou/Ot(x, 0) = vo. Thus,

we have effectively retrieved (3.31), albeit by a more long-winded but more pow-

erful method. However, (4.37) illustrates how concepts such as regions of influence

and domains of dependence can emerge naturally from Fourier representations;

outside a region of influence the solution is represented by an integral round a

contour containing no interior singularities, and is thus zero.

We also remark that (4.37) is a very typical result in the application of Fourier

transforms. In the case of this Cauchy problem, the method effectively retrieves

the general solution of the wave equation in terms of the arbitrary functions uo

and vo Of course, Fourier representations such as (4.37) satisfy the wave equa-

tion for arbitrary initial and boundary conditions, but often it is not so easy to

relate the arbitrary functions of k that enter into the Fourier representation to the

initial/boundary data for any particular problem.

APPLICATIONS TO WAVE EQUATIONS 115

The alternative Laplace transform approach is even more messy: we obtain

2d2u 2-

ao dx2 -

p u = -vo(x),

for which the appropriate solution is

uo(x,p) = 2

(foo

f e-W-=)/aovo(t) di;) ,

2p > 0.

A formal reverse of the order of integration in the Laplace inversion gives the

d'Alembert solution (4.38) with u0 = 0 when we remember that

1 en(E-:)/aoe

dp = J0,

x - e; > apt,

27ri

7-ioo

1, x -' < aot,

when Ry>0.

The initial/boundary value problem in which

u = 0, & = vo(x),

for 0 < x < 1,

(4.39)

with, say, it = 0 at x = 0 and x = 1, which is the two-boundary version of the

problem we presented in Fig. 4.3, can be attempted by either a Fourier series

in x (because X(x,.1) = sin (V1rx)) or a Laplace transform in t. Using the

specialisation of (4.21) to odd functions, so as to satisfy the boundary conditions,43

we find

u = E b sin n7rx sin niraot,

(4.40)

n=1

where

bn = vo (x) sin nzrz dx,

n rao

which is sometimes a more convenient description of the solution than would be

the equivalent of (4.38), encompassing as it does all the possible discontinuities

running along the `zig-zag' characteristics in Fig. 4.5. The bottom left-hand corner

of Fig. 4.5 is Fig. 4.3.

We also remark that we can find the general solution of (4.34) by superposing

elementary solutions of the form 6(x - aot) and 6(x + aot). Instead of (4.35), we

obtain

u(x,t) =

foo

(6(x - x' - aot)f(x') + 6(x - x' + aot)g(x')) dx'

= f(x - aot) + g(x + aot),

(4.41)

where f and g are arbitrary; another route to this formula is given in Exercise 4.8,

and we will return to this observation below.

43This

can be systematised by saying that we seek f. and X as in (4.20), with X(0) = X(1) = 0.

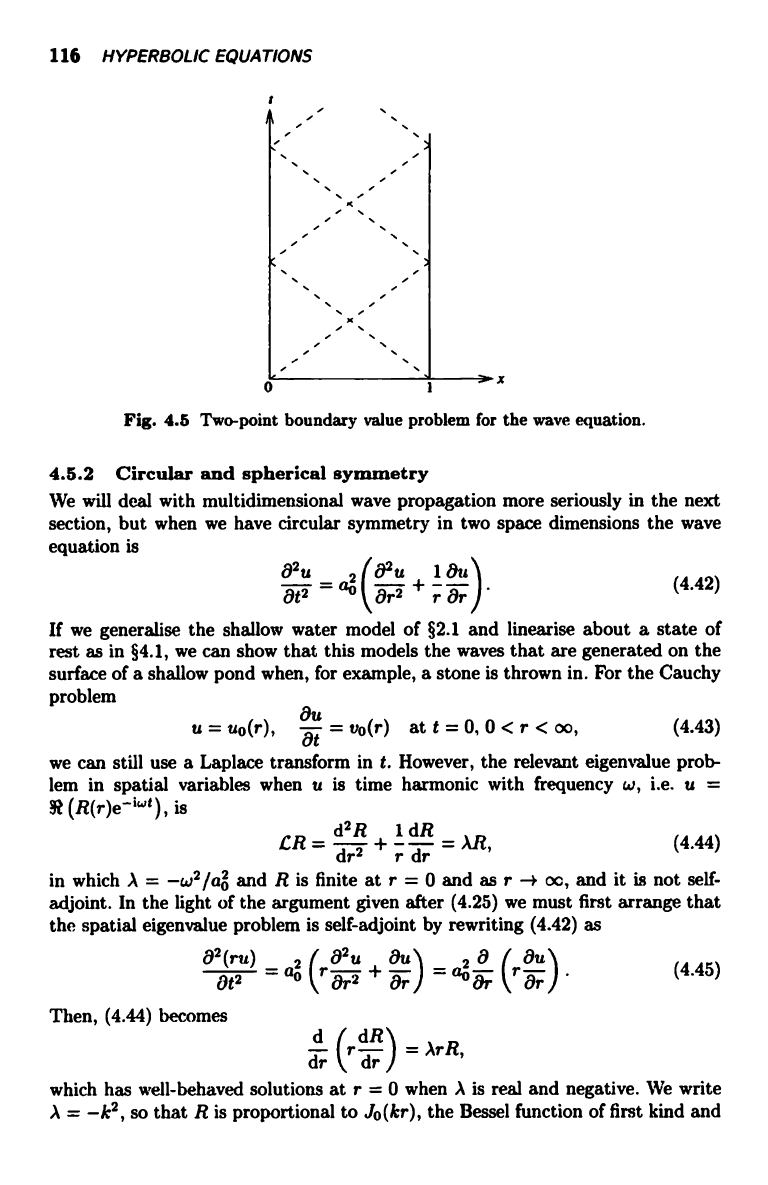
116 HYPERBOLIC EQUATIONS

F-1,

0 1

Fig. 4.5 Two-point boundary value problem for the wave equation.

4.5.2

Circular and spherical symmetry

We will deal with multidimensional wave propagation more seriously in the next

section, but when we have circular symmetry in two space dimensions the wave

equation is

02u

_ ao

(82u 18u

8t2

8r2 +

(4.42)

If we generalise the shallow water model of §2.1 and linearise about a state of

rest as in §4.1, we can show that this models the waves that are generated on the

surface of a shallow pond when, for example, a stone is thrown in. For the Cauchy

problem

u = uo(r), at = vo(r)

we can still use a Laplace transform in t.

lem in spatial variables when u is time

'lt (R(r)e-'"i), is

at t = 0, 0 < r < oo,

(4.43)

However, the relevant eigenvalue prob-

harmonic with frequency w, i.e. u =

d2R 1 dR

CR =

dr2 + r dr

AR,

(4.44)

in which A = -w2/ao and R is finite at r = 0 and as r -+ oc, and it is not self-

adjoint. In the light of the argument given after (4.25) we must first arrange that

the spatial eigenvalue problem is self-adjoint by rewriting (4.42) as

) = a0 (r

8r2 +

8r)

f r8r

(4.45)

Then, (4.44) becomes

aR )

= ArR,

which has well-behaved solutions at r = 0 when A is real and negative. We write

A = -k, so that R is proportional to Jo(kr), the Bessel function of first kind and

APPLICATIONS TO WAVE EQUATIONS 117

zeroth order. From (4.26) the appropriate spatial transformation is the so-called

Hankel transform

u(k, t) = frti(rt)Jo(kr)dr.

The inversion formula for this transform is found most easily by taking the double

Fourier transform of a function U(x, y, t) with respect to x and y, namely

U(kl, k2i t)

(

oc .l

f c eu(k,r+k2V)U(x,

y, t) dx dy,

(4.46)

l oc

for which (4.31) gives

r00

U(x, y, t)

e-i(k,=+k2V)U(ki, k2, t) dki dk2.

47r

When U(x, y, t) = u(r, t), (4.46) becomes

00 2x

U(kl,k2it) =

ru(r,t)

f e'kr`os0dOdr,

where

k2 = ki +l4,

= 27ru(k,t),

since, as can be verified by direct differentiation,

(4.47)

Similarly,

and so44

u(r, t) =

f00

ku(k, t)Jo(kr) dk.

If, for simplicity, we take uo = 0, we find that

roo

u(r, t) = 1

vo(k)Jo(kr) sin aokt dk;

(4.48)

ao o

this formula has important repercussions to which we will return later.

In three space dimensions, (4.42) is replaced by

82u

_ 2

(02u

2 8u1

(4.49)

8t2 -

8r2 + r 8r J'

and now a surprising 'symmetry' occurs. By writing u = rv we find

82v_ 282v

8t2 =

,2'

and we are back in one space dimension. Thus, for hyperbolic problems, it is

sometimes easier to proceed when there are more independent variables than when

there are fewer, as we shall also discuss further in the next section.

44We will derive this 'transform pair' by another method in Chapter 5.

ku(k, t)

a-ikr cos 0 do) dk

u(r, t) =

fo"o