Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations

Подождите немного. Документ загружается.

138 HYPERBOLIC EQUATIONS

as (xo, yo) vary with uo = u(xo, yo). Hence, writing p = 8u/8x and q = au/8y in

order to save ink, if we think of x and y as functions of p and q and define

w(p,q) = px(p,q) + qy(p,q) - u(x(p,q),y(p,q)),

(4.86)

then this tangent plane is

w = wo = p(xo, yo)xo + q(xo, yo)yo - uo.

Thus, with w defined by (4.86), the surface w = w(p,q) defined pointwise in

(w, p, q) space is the same surface as u = u(x, y) in (u, x, y) space, and w is the

variable that we should work with.

Equation (4.86) is called the Legendre transform of u and it means that the

formula for the tangent planes to u = u(x, y) in (x, y, u) space involves no differ-

entiations in w space, and vice versa. Partial differential equations whose solution

surfaces possess this property are clearly promising candidates for explicit solutions

if we formulate them correctly. To do this we need the identity

Spa

aq e

Y X_ 8xOp+exOq'

so that

aw ax

8u 8w

= x + p

+ q

= x,

= y

Now let us carry this further. Since

8p 82w

Oq 02w _ Sp 02w 8q 82w

1 _ 8x 8p2 + 8x Op 8q'

8x 8p 8q + 8x 8q2 '

we have the easy relationships

02u

1 02w

02u _ 1 02w 82u

1 02w

8x2

O 8q2 ' 8x 8y 0 8p 8q' 82y 0 8p2 '

where the discriminant

82w 82w

82w 2 _ 82u 82u 82u

8p2 8q2 8pOq) - (8x2 8y2 - (8x

8y)2)-l

(4.87)

Equations such as (4.85) are eminently suitable for this kind of change of vari-

ables. Indeed, this is the reason that the method is so-called because in mechanics

the hodograph is the plane of velocities. The ensuing linear equation is

F(p, q) aqs - G(p, q)

8p 8q

+ H (p, q) Spa = 0,

and various further simplifications are sometimes possible.

The most important realisation provoked by this gasdynamic example is that

A, as defined by (4.87), is infinite in the following cases.

NONLINEARITY 139

1. In a uniform stream, the whole of the regions above and to the left of OA in

Figs 4.12 and 4.13 map into a single point (U,,,, 0) in the hodograph plane.

2. In a simple wave, such a region maps into the curve in the hodograph plane

corresponding to the constancy of the relevant Riemann invariant.

These observations prompt us to ask the question `when can the hodograph trans-

formation go wrong?' Trouble will only come if A is zero or infinite, preventing

the map from (x, y) to (p, q) from being one-to-one, and we can make two remarks

about this. First, the equation A = oo has the geometric interpretation that the

surface u = u(x, y) (w = w(p, q)) is developable; this means that it is generated

by the tangent lines to some curve 1' or, more technically, it has zero Gaussian

curvature.b" Hence, when A = oo, it is not surprising that there are many points

on the developable where the normal vectors (p, q, -1) are identical, because the

tangent plane to u = u(x, y) everywhere along a generator of the developable is

just the `osculating plane' at the point where the generator touches r. Second,

the equation A = 0 is itself an interesting non-quasilinear equation for w(p, q), to

which we will return in Chapter 8.

Although the hodograph method can be applied equally to elliptic and parabolic

equations, the remarks above indicate that it must be used with caution. We must

also draw the reader's attention to the sad fact that, in practice, the dependence

of the physical boundary conditions on (x, y) often makes it impossible to find

appropriate boundary conditions for w. The principal exception in fluid dynamics

is when the flow direction is known to be constant on the boundary, i.e. when the

boundary comprises only straight segments, and we will return to this situation in

Chapter 7.

4.8.3 Liouville's equation

There is one other device that we cannot resist mentioning here because of its ap-

plicability to an elliptic equation as well as a hyperbolic one. It concerns Liouville's

equation

02u

- eL8x 8y

for which the apparently retrograde step of differentiating with respect to x un-

covers an exact derivative with respect to y, namely

82u

8x2 2 (8x )

We are amply repaid because we see that 8u/8x satisfies the ordinary differential

(Ricatti) equation

82u 2 - f(x)

8x2

(8x8u)

51A developable is a special

case of a ruled surface, i.e. one formed by glueing together a

one-parameter family of straight lines.

140 HYPERBOLIC EQUATIONS

for some function f. Hence, by writing52

2 Ov

v 8x'

so that u(x, y) = 2 log (F(y)/v(x, y)) for an arbitrary function F(y), we have

reduced Liouville's equation to the linear problem

02V 1

+ 2 f (z)v = 0.

Although this gives the general solution, containing as it does the two arbitrary

functions f (x) and F(y), the dependence of u on f and F is far from transparent.

However, we can make more progress by noting that we could equally well have

differentiated first with respect to y, giving that u(z, y) = 2log (G(z)/w(x, y)),

where

02W

8y2 +

29(y)w = 0,

for two more arbitrary functions g(y) and G(x). The final coup is to exploit the

structure of solutions of second-order linear equations, writing

v(x,y) = a,(y)vl(z) + a2(y)v2(x),

w(x,y) = bi(x)wi(y) + b2(x)w2(y),

so that, since F(y)/v(x, y) = G(x)/w(x, y),

G(x)vi(x)

F(y)wj(y)

for i = 1, 2,

bi(z)

al(y)

where y is constant. But the constancy of the Wronskians of the equations for v

and w shows that we can take

v2 (x) = VI (Z)

f ;

(S)

w2 (y) = wi (y) f y

from which there are functions X (x) and Y (y) such that

1 X

1 Y

V1 = X,, V2 = X,, wl = Y,, W2 = Y,,

and so

F _ 7

X1(x)Y1(y)

wlvl

+ w2v2 ='y y 1+X(x)Y(y)

The final step is to take -y = f, so that the general solution of Liouville's equation

is given in terms of two arbitrary real functions X(x) and Y(y) as

u(x,y) = log

(_2X'(x)Y'(y)

(1 + X(x)Y(y)

Other choices of y give the general solution of 02u/Ox Oy = ce" for c # 1.

52The rationale for this transformation will be explained in Chapter 6.

EXERCISES 141

*4.8.4

Another method

Apart from the many other ingenious tricks that exist in the literature, but are

too specialised to list here, there is one other `heavy gun' that can sometimes be

wheeled out when dealing with nonlinear hyperbolic equations, and that is soliton

theory [15]. This method is complicated technically and, since some of its most

famous successes concern non-hyperbolic equations, we will defer discussion until

Chapter 9. Meanwhile, readers might like to consider what they would do if faced

with the Sine-Gordon equation

82u 82u

8x2 - 8t2 =

sin u,

which arises in superconductivity modelling.

Exercises

4.1. Verify the result in footnote 39 by showing that, if t = ax + by and n =

cx + dy, then

f e2+V2h/'

dds1= det

a b

1-00

\c dJ

4.2. Show that, if C = 82/8t2 - 82/8x2 and homogeneous Cauchy data u =

8u/8t = 0 is given on t = 0, then D in Fig. 4.1 is the triangle with vertices

at (x, t), (x - t, 0) and (x + t, 0), and R = 1/2. Hence show that the solution

of Cu =

e-x-i

in t > 0 is

-e-r d dr =

((2t +

1)e-x-e - e-:+t)

u(x, t) =

J 1fD e

82u

_ 82u

= 1

8y2

8x2

almost everywhere in x > 0, y > 0, with u(x, 0) = 8u/8y (x, 0) = 0 for

x > 0, and u(O,y) = 0 for y > 0.

By extending u to be an odd function in x, so that u(-x, y) = -u(x, y), and

using a Riemann function, or otherwise, show that

fy2/2,

x > y,

x(2y - x)/2, y 3 x.

Verify that u and its first derivatives are continuous and evaluate the jumps

in its second derivatives across the characteristic y = x.

Why did we say that the equation is satisfied only `almost everywhere' and

not everywhere?

142 HYPERBOLIC EQUATIONS

4.4. Show that, if

02u 02u_

8x2 8y2 = 0'

then the Riemann invariants

8u 8u

are constant on the characteristics y ± z = constant.

Suppose u satisfies Cauchy data

(1 - 2xy), = 1 - 2xy

on the semicircle r2 = x2 + y2 = 1, y < 0. Show that a solution exists in the

form a(x-y)2+b(x+y)2+c, where a, b and c are constant and that, for this

solution, Ou/Ox + 8u/8y = 0 everywhere and 8u/8x - Ou/Oy = constant on

x - y = constant. However, the problem is ill-posed because the boundary

is tangent to characteristics at (f1/f, -1/f). Show non-existence when

the data is perturbed so that

8x -

iii j4 2(x-y)

on the arc between (1/f,-1/f) and (1,0).

4.5. Suppose that

82u 82u

= 0

8x2

8y2

almost everywhere in y > 0, with

u=ou=0 ony=0,x>0,

u=0,

Ou=1 ony=0,x<0.

Use a Riemann function to show that

u=-0 inx>y>0,

u=-y in-x>y>0.

By computing the Riemann invariants show that

8u 8u

8y 8x

in -y < x < y and check that u, but not its first derivatives, is continuous

across x = ±y. Confirm these results using d'Alembert's solution.

EXERCISES 143

4.6. Suppose that

Ou Ou

8t + 8x =

b(x - 2 t2)

for t > 0, with u(x, 0) = 0. Show that putting £ = x -

t2 gives

(1-t)8C+

=aW,

with 0) = 0. Deduce that, for t < 1, u = 0 for x > t and x < It'. Show

further that

1 t2

)

u(x,

as x

and hence that

( -

1+2(x-t)

for

t <z<t<1.

By considering the characteristics in the

t) plane, show that this solution

holds for t < 2 and that, for t > 2,

_ 0,

x<tandx> Zt2,

u(x, t)

-1/

1 + 2(x - t),

Zt2

< x < t.

Remark. Note that it would be easy to get the wrong answer to this question

without the substitution = x- s t2. It is good technique to use the argument

of a delta function as an independent variable where possible.

* 4.7. A problem in the dynamics of the overhead power wire for an electric loco-

motive leads to the model

&28x2 =0 for xiO X(t),t>0,

where X is a prescribed smooth function with 0 < X'(t) < 1 (t is time, X (t)

is the locomotive position and u is the displacement of the wire). Across

x = X, there are prescribed discontinuities

x+O

jX_o = -V(X(t),t), [u]x-O = 0.

Suppose u = Ou/8t = 0 at t = 0. By modifying the argument leading to (4.8)

to account for the discontinuities across x = X, show that

u(x,t) = I

IVV,r)

(I -

(X'(r))2) dr,

where the integral is taken along the part of £ = X (r) that lies within

r - t < C - x < t - r, r > 0. If X = t2/2 and V = 1, show that, for

0 < x < t <

2x,

Ss\ !

where x-

=t-l;,

and comment on the well-posedness of this situation.

144

HYPERBOLIC EQUATIONS

4.8. Show that the Fourier transform in x of 8(x ± aot) is a*'*Qt. Now recall that

the Fourier transform of the general solution of the one-dimensional wave

equation (4.35) on p.114 is

u(k, t) = f (k)eikaot +

9(k)e-fkoot

Use the convolution theorem to derive the general solution itself in the form

u(x, t) = f(x + aot) + g(x - aot).

4.9.

(i) Suppose that f (x) = 0 in x < 0 and F(x) = f

(x)e-131

-> 0 as x -+ +oo

for some # > 0. Define

°O

F(k) T

j F(x)elk:

J ao

and, assuming F can be suitably analytically continued, define f by

j (k) = F(k - is).

Show that the inversion formula

F(x) = 2; r P(k)e-'kr

J 00

becomes

oo+iR

f (x) _

(rc)e-I"s dr..

2 !

00+i$

Show also that this implies that the Laplace inversion formula is

7+i00

f(x) =

2ri

, 1_i0o

f(p)evx dp,

where ry is such that all the singularities of f lie in R p < y.

Suppose that

for t > 0, with

02u

_ 82u

8t2 8x2

u(x,0) = 0,

8t(x,0) 0,

le0z,

x<0,

x>0,

for some a > 0. Show that

u(x,t)

1 r"0+'p

(- sin

rct)e-"" dre

21r -3°+io

rc(a +

for 8 > a > 0, and hence use the calculus of residues to show that

0, x < -t,

u(x, t) _

(e°(x+t) - 1)/2a,

-t < x < t,

(e°(:+0

- e°(z-t))/2a,

t < x.

EXERCISES 145

4.10. Suppose that f (x) satisfies Airy's equation

ax+xf

=0.

Verify that a solution is

P x) =

e-C*F(() d(,

where F(C) = e8' and I' is such that the integral exists and the change

e-(e/3-Zc from

one end of f to the other is zero. Find two choices of t

that cannot be deformed into each other.

4.11.

(i) Take the Laplace transform of Bessel's equation of order zero,

rdao+

dr°+rJo=0,

and use the facts that Jo(r) is analytic at r = 0 and f O° Jo(r) dr = 1 to

show that the Laplace transform of Jo is

1+p2-

(ii) Show that, if r is such that differentiation under the integral sign is

permissible, then

satisfies Bessel's equation of order v,

ddrV +r dr

+1r2)Jv=O.

Deduce that the Laplace transform of J,, is proportional to

1+p2 (p+

1+p2)'

* 4.12. (i) Show that, if

1 + k2 is defined so that it asymptotes to k as Ik) -+ oo in

all directions in the Argand diagram, a branch cut must be introduced

between k = ±i.

(ii) When we take ao = c = 1, the inversion of (4.51) is

U(x,

t) =

2z i_:

(J'

vo(()eikf dC)

sill ((t

1 + k2

a tkx dk.

,° J l+k

With the definition of 1 + k given above, reverse the order of integra-

tion and consider

Ioo eik(( -:)fit 1+

°° 1+k

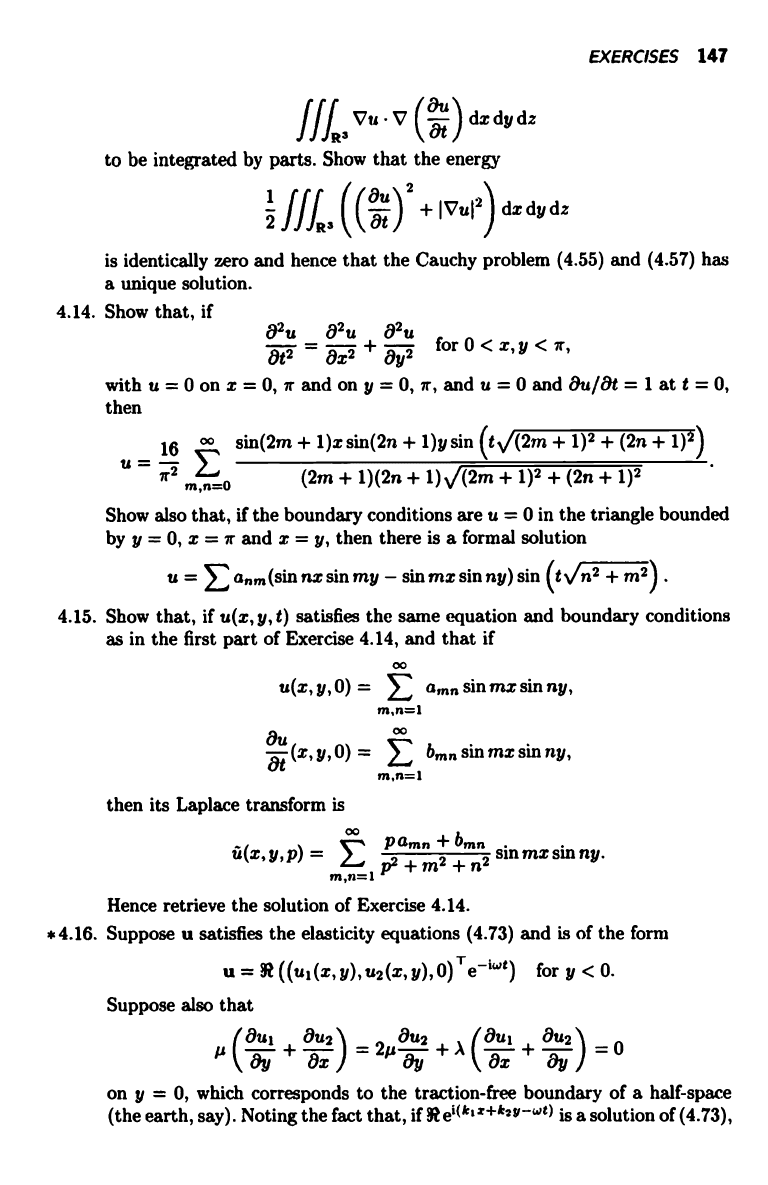
along the contour of Fig. 4.14, inside which there are no singularities.

146

HYPERBOLIC EQUATIONS

Show that

Fig. 4.14 Contour for Exercise 4.12.

r sin (t 1 + k

eik((-x)

1+k2

1 cosh(s (x - t)) Cos (t

1 - s2

= 2

ds, x-t<<z+t,

1-s

z+t <

with the non-zero contribution coming from the integrals along either

side of the branch cut. Finally, quote the result that

os1scosh(as)

1 - s

d8 =

A (

62 - a2) ,

which follows from (4.47) on p. 117, to retrieve the Riemann function

solution

x+t

u(x, t) = 1 J o ( t2 - (x - f)2) vo(t) df

2 z-t

Check that, if we had defined

1 + k to tend to k if R k > 0 and -k if

It k < 0 as IkI -> co, we would obtain a domain of dependence of (x, t)

that included points ((,0) such that it - xj > r. Why would this be

physically unrealistic?

4.13. Suppose that

82u

_ 02u 82u 82u

8t2 872

8y2 + 8Z2

in all space with u = 8u/8t = 0 at t = 0, and that u decays sufficiently fast

at infinity for

EXERCISES 147

fJJR

()dxdYdz

tobe integrated by parts. Show that the energy

2Iff, (()2

+ IVuI2)

axdyaz

is identically zero and hence

that the Cauchy problem (4.55) and (4.57) has

a unique solution.

4.14. Show that, if

2 2

t2 - 8x2 + 8y2

for

0 < x, y < 7r,

with u=Oonx=O,irandony=0,ir,andu=OandOu/8t=1 att=0,

then

O°

sin(2m + 1)xsin(2n + 1)y sin (t,/-(2m+ 1)2 + (2n + 1) )

u_x2 Eo

(2m + 1)(2n + 1) (2m +-1)2+ (2n + 1)2

Show also that, if the boundary conditions are u = 0 in the triangle bounded

by y = 0, x = 7r and x = y, then there is a formal solution

u =

anm (sin nx sin my - sin mx sin ny) sin (t

n2 + m2) .

4.15. Show that, if u(x, y, t) satisfies the same equation and boundary conditions

as in the first part of Exercise 4.14, and that if

u(x, y, 0) = E a,nn sin mx sin ny,

m,n=1

(x, y, 0) = E bmn sin mx sin ny,

then its Laplace transform is

m,n=1

u(x, y, P) _ F

P amn + b"'n

sin mx sin ny.

2+m2+n2

Hence retrieve the solution of Exercise 4.14.

* 4.16. Suppose u satisfies the elasticity equations (4.73) and is of the form

u = lrt ((u

l(x,y),u2(x,y),0)Te-1wt)

for y < 0.

Suppose also that

(Out

8u2

_ 8u2 Out 8u2 _

8J +

8x)

2P a1/

+ 8x + ay) 0

on y = 0, which corresponds to the traction-free boundary of a half-space

(the earth, say). Noting the fact that, if

e0':+k2Y_

?t) is

a solution of (4.73),