Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations

Подождите немного. Документ загружается.

148 HYPERBOLIC EQUATIONS

(kl

, k2 i w) lies in the normal cone

ki + k2 = w2/c2, use (4.75) to check that,

for any constants A and B,

u1 = kAe'kz+dr

k2-w2/cps

k2 -

e'kz+y

k -m cp

and

ul = iB

k2 -

w2 eikr+u

k72-w2/c;

u2 =

kBeikz+y k W2/c;

are both candidate solutions of (4.73). Show further that the boundary con-

ditions are satisfied if k = w/c, where

(2_)2_4(1_)

('1) =0.

(It can be shown that this equation has a root in which c > c, > c; it

corresponds to the famous Rayleigh wave of seismometry.)

* 4.17. According to Maxwell's equations, the static magnetic field H = (Hi, H2i

H3 )T in an inhomogeneous medium satisfies

VAH=O,

where µ is a function of position. Suppose the interface z = 0 separates a

medium in which µ = µ+ = constant from one in which µ = µ- = constant.

By using Green's and Stokes' theorems show that a weak solution satisfies

[PH3]:=00± = 0,

[H1]z O- _ [H2]z=o± = 0.

* 4.18. Consider a time-varying electromagnetic field in a homogeneous conducting

medium in which Ohm's law relates the current density and electric field by

j = aE, where a is the conductivity, and suppose there is no charge density.

Show that

02E

a 8E

C2 V2 E,

8t2 8t

where c2 = 1/(eµ). Hence show that, if E = E(x, t), its components can be

found in terms of solutions to the telegraph equation.

* 4.19. As shown in Chapter 2, if the normal cone is Q(to, t1, t2, ...) = 0, where

t; = 8tio/8x, are the components of the normal to the characteristics (wave-

fronts) <p = constant, the ray cone is given by F, x,fr = 0, where xi =

p 8Q/8t;. Take xo = t, xi = x and x2 = y and show that, if the normal cone

(i)

at, + bf2 - to = 0,

2 2

(ii) a2 + - to = 0,

( )

6 2-q2=0,

EXERCISES 149

then the ray cone is

= 6 = -t,

(ii) a2x2 + b2y2 = t2,

(iii)

4xy = t2.

Draw a typical cross-section o = constant, t = constant in each case, in-

cluding the case a = b = e, a -> 0 in (ii). Use the acquired knowledge to

relate the local geometry near a point on a cross-section to = constant of

the normal cone to the geometry of a cross-section t = constant of the ray

cone, and hence verify Fig. 4.11.

4.20. Suppose that

82u

8x2 =

8y2.

Show that, if u(x, y) = v(p, q), where p = Ou/ax and q = Ou/sy, then

82v a2v

.9p

8p2

q2 ,

long as

# ay-

4.2 1.

(i) Following (4.13), define V2 log r in two dimensions by the identity

2a 00

r2,,

IOV2(log r)rdrd9 = /

(log r)(V2V') rdrd9

0 0 0 0

in polar coordinates. By showing that

2a a

( bIr_, +eloge

I _

f dO

1 I

OV2(log r)rdrd9

= fo

0 c

aT re/

2a r00

+ 1

(log r) r dr dO

(be careful about the direction of the normal and the orientation of the

contour!) and taking the limit as a -+ 0, show that

\72 (log r)

= 2ir6(x)6(y).

(ii) Show that, if u = 0 in x < 0 and y < 0 and

= a(x)a(y),

then u = H(x)H(y). Does

82u 02u

OX2 - i =

6(x)a(y)

have a solution that vanishes in x < 0 and y < 0?

150

HYPERBOLIC EQUATIONS

* 4.22. The displacement u(x, t) of an elastic string satisfies

82u 82u

= 0

for -oo < x < oo.

8t2 - 8x2

Show that, if u(x, 0) = 0 and 8u/8t (x, 0) vanishes except for jxj < c, where

it is a constant vo, then u = evo in e - t < x < -e + t, t > e. By taking

a suitable limit show that, when u(x, 0) = 0 and 8u/8t (x, 0) = 25(x), then

u(x, -t) is the Riemann function for the wave equation written in this form,

in the domain t < 0 and with

37) = (0, 0). (This Riemann function is

defined to satisfy

82u 82u_

_- 25(x)5(t);

8t2 8x2

as in Exercise 4.2 the factor 2 is introduced because the equation is not in

canonical form.) Show also that, if u(x, 0) = 5(x) and 8u/8t (x, 0) = 0, then

u(x,t) = 2(5(x + t) + 5(x - t)).

Elliptic equations

In this chapter we will, as usual, begin by discussing some physical situations

that are modelled by elliptic equations, as defined in a rather unfocused way in

Chapter 3. Most of the examples involve scalar second-order equations, several of

which are special cases, such as steady states, of evolution models discussed in

Chapters 4 and 6.

The methods we will use in the subsequent analysis of these models are more

ad hoc than those used on hyperbolic equations, for the simple reason that we

have no general well-posedness statement analogous to that for the Cauchy prob-

lem for hyperbolic equations. Moreover, we will find that the influence of the data

for elliptic problems, especially singularities in the boundary data, is much less

localised and `coherent' than it is for hyperbolic equations, where we recall that

many kinds of singularities merely propagate along characteristics. These are the

reasons why this chapter is longer than its predecessor; however, the most powerful

tools we develop are Green's functions, which are direct analogues of the Riemann

functions of Chapter 4, and eigenfunction expansions. Whereas most of the illus-

trations of Chapter 4 revolved around the wave equation, here the paradigm is

Laplace's equation.

The range of applicability of elliptic equations is vast, as will be apparent

from the following section where we will present models arising in gravitation,

electromagnetism, mechanics, heat flow, chemical reactions and acoustics.

5.1

Models

5.1.1 Gravitation

Some of the most famous models in the history of applied mathematics lead to

elliptic equations, the most revered perhaps being that of Newtonian gravitation.

This is based on the observation that `point' masses attract each other along the

line joining them with a force proportional to the inverse square of the distance

between them. Hence, with a suitable normalisation, the force field of a unit point

mass (soon to be related to a Green's function) is the gradient of a potential

0=1

(5.1)

in spherical polar coordinates; 0 is unique up to the addition of a constant. Sum-

ming over a distribution of such masses, the potential of a general density distri-

bution p(x) is

151

152 ELLIPTIC EQUATIONS

O(x) = 1J

p(x') IX -

X11

(5.2)

and it is a simple calculation to show that, away from any matter, 0 satisfies the

famous Laplace's equation

V20=0.

(5.3)

Functions satisfying this equation are said to be harmonic functions. However, it

is less easy to show that, in the presence of matter, 0 satisfies Poisson's equation

in the form

V20

= -47rp.

(5.4)

The most elementary procedure is to consider the integral in (5.2) with a small

sphere around x excised, write down its Laplacian and use Green's theorem, but

we will see this in a more general setting in §5.5.

Presented this way, the theory of gravitation does not seem to be a problem in

differential equations, because the solution of Poisson's equation (5.4) is known to

be (5.2). But often this formula is tedious to compute and we will soon see that

the partial differential equation formulation is of inestimable conceptual value.

5.1.2 Electromagnetism

Almost equally venerable are models for electrostatics and magnetostatics, which

can be derived at a glance from §4.7.2. In the absence of any charge p and current

j, and with 8/8t = 0 in a steady state, we find

VAH=VAE=O. (5.5)

Hence both the magnetic and electric fields are the gradients of potentials, which,

like (5.2), are only defined to within an additive constant; but they both satisfy

Laplace's equation because, by (4.83) and (4.84), V H = V E = 0. Now we have a

more clear-cut partial differential equation situation because these electromagnetic

fields can be modelled as if they are created at boundaries, or at infinity, where

E and H satisfy certain conditions. We will not give these conditions here except

to say that the commonly occurring problem of finding the electric field outside a

charged perfect conductor demands that the electric potential 0, where E = -0t,

is constant on the conductor. The charge, which is confined to an exceedingly thin

layer near the surface of the conductor, turns out to have a density proportional to

the normal derivative 80/8n, a fact that can be inferred by reinstating the charge

density p, so that V20 = -p/e, where a is the permittivity, and using (5.2). The

problem of designing a lightning conductor involves solving Laplace's equation

with 0 constant on the conductor and calculating where 8c6/8n is largest, because

this is where the lightning will strike.

Indeed, many everyday situations involve charge distributed on a long thin

straight wire and it is easy to see that in such cases the electric potential in plane

polar coordinates is proportional to log r. Only the field, not the potential, goes

to zero as we move away from the wire, in distinction to the potential (5.1) which

decays as we move away from a point charge.

MODELS 153

More commonly, we have to model currents flowing in electrically conducting

materials. Then, if the current flow is steady, we can often use the experimentally

observed Ohm's law

j = QE, (5.6)

where a > 0 is the conductivity, taken to be constant for simplicity. When we take

this in conjunction with Maxwell's equations (5.5), we have that j = -aVo, where

yet again 0 satisfies V20 = 0. Note that if we then needed to find the magnetic

field we would have to solve

VAH=j,

(5.7)

we can take the curl of the first equation to obtain a vector version of Poisson's

equation, but (5.7) itself is an as-yet-unclassified system for H to which we will

return in Chapter 9.

There are many, many other interesting situations involving Maxwell's equa-

tions and Ohm's law which we do not have space to mention in detail here. For

example, it is often possible to reduce the model to ordinary differential equa-

tions when currents only flow in wires, and to two-dimensional partial differential

equations for flow in metal sheets.

5.1.3

Heat transfer

We will use this even more familiar discipline to begin to write down some concrete

problems for elliptic equations. Suppose heat is being conducted in a medium D

whose temperature is T (x) and in which there is a volumetric heat source f (x),

as in the element of an electric fire, or in food cooked in a microwave oven, or in

the radioactive decay process in the core of the earth. Now Fourier's law of heat

conduction states that the heat flux is given, analogously to (5.6), by

q = -kVT, (5.8)

where k > 0 is the thermal conductivity. Hence conservation of energy requires

that, for any region D in the material,

-k8nd5

- ffj V (kVT)dx= Jff

v fdx (5.9)

in a steady state; here, as usual, 8/0n denotes the derivative along the outward

normal to the boundary OR This is true over any region in the conductor so that,

when k is constant, we again retrieve Poisson's equation

kV2T = -f (x). (5.10)

We are almost always given a boundary value problem for T, there being three

very common situations.

The Dirichlet problem

Suppose the boundary is an excellent thermal conductor (e.g. a metal). Then its

temperature is nearly constant, or at least a prescribed function of position on the

boundary:

T = To(x)

on OD.

(5.11)

154 ELLIPTIC EQUATIONS

The Neumann problem

Suppose the region outside D is a very bad conductor, such as air. Then the normal

heat flux is zero or, more generally, a prescribed function of position:

-k = q(x)

on 8D.

(5.12)

The Robin problem

The Robin problem arises when the heat flux at the boundary is proportional to

the difference between the boundary temperature and some ambient temperature.

This might be the case if D is a solid (such as your skin) around which a relatively

hot (or cold, usually) fluid at temperature To(x) flows, and heat transfer takes

place across a thin `boundary layer' in the fluid. There are then experimental and

theoretical reasons to write down

= h (T - To (x))

on OD,

-k

(5.13)

where It is called a heat transfer coefficient. Thermodynamics requires that h > 0,

so that heat flows from hotter regions to colder ones.

On physical grounds (5.12) is suspicious because, assuming D is bounded, we

could not expect to be able both to prescribe the heat flux on OD and yet have a

steady state in which T is independent of time. We can quantify this suspicion by

integrating (5.10) over D and using Green's theorem to yield

fffl.f(x)dx_fJ1.qdS=0

(5.14)

for the Neumann problem. Here we have inserted the factors of unity to highlight

that this is simply a statement of the Fredholm Alternative; the `right-hand side'

of the Neumann problem, which is a combination of f and q, must be `orthogonal'

to any relevant eigenfunctions, in this case constants. Moreover, when (5.14) is

satisfied, even though T exists, it is undetermined to within the addition of any

eigenfunction. We will see later that this non-existence/non-uniqueness does not

happen with (5.13) if h > 0.

There are countless other variations and applications of the Laplace and Pois-

son equations, and we will cite only three very briefly. First, since Fick's law of

molecular diffusion, namely that mass flux is proportional to concentration gradi-

ent, is mathematically identical to Fourier's law, the mathematics of beat transfer

by conduction and of mass transfer by diffusion are identical.53 Second, there are

numerous situations where heat and mass transfer are intimately coupled, as in

the case of many commonly occurring chemical reactions. We will study these in

more detail in Chapter 6 but for now we simply note that the temperature in a

steady-state reaction might be modelled by the uncoupled thermal model (5.10) in

331n fact, they are both based on the microscopic models of Brownian motion, which will be

discussed further in a simple-minded way in §6.1.

MODELS 155

regions where the source term is a function, possibly nonlinear, of T. This function

represents local heat generation near the reacting regions and is positive or nega-

tive depending on whether the reaction is exothermic or endothermic, respectively.

Finally, it is often important to consider convective and radiative heat transfer as

well as conduction. The former is relatively easy to incorporate because we simply

have to include a term pc f f f v VT in (5.9), where v is the velocity, p is the

density and c the specific heat54 This leads to the convection-difusion elliptic

equation

pcv VT = kV2T + f (x),

(5.15)

where, if we are lucky, v is prescribed independently as the result of some un-

coupled dynamics model. Alas, the dynamics and heat transfer are all too often

coupled.

Radiation is much more difficult to model, as we shall see in Chapter 6, but

a simple case is that in which a `black body' radiates from its boundary, and

all the beat transfer in the interior is by conduction alone. On experimental and

theoretical grounds we can then write down the Stefan-Boltzmann law

q(x) = e(T4 - To)

(5.16)

in (5.12), where, again, To is an ambient temperature (now measured in absolute

terms) and a is a constant called the emissivity (see [41] and §6.6.1.2); note that,

when T is close to To, (5.16) can be approximated by (5.13) with h = 4ET0 3.

All discussion of such nonlinear effects, be they from chemical reactions or

radiation, is deferred to the end of the chapter.

5.1.4

Mechanics

5.1.4.1

Inviscid fluids

Continuum mechanics provides another justifiably famous source of elliptic equa-

tions. One of the most familiar is that of inviscid incompressible hydrodynam-

ics [27]. The fact that the fluid is incompressible means that the velocity field

v(x, t) satisfies

V.v=0, (5.17)

but it is a long story to prove that many inviscid flows can be well approximated

by writing down the condition that the vorticity V A v satisfies

VAv=0.

and thus that v is the gradient of a potential 0. We will discuss this point further

in Chapter 9, but, for the moment, let us assume that 0 exists, although it is only

defined to within addition of an arbitrary function of time, and is harmonic from

(5.17). Typical boundary conditions now take the form of homogeneous Neumann

conditions because the relative velocity is tangential to any stationary impermeable

34 However, if the material is a gas we must be much more careful about our definition of

specific heat and also to incorporate the `work done against compression'.

156 ELLIPTIC EQUATIONS

boundary;SS this condition is associated with the fact that, for these inviscid flows,

we only have Laplace's equation to solve, and hence in `streaming flows' we have a

Neumann problem in an infinite domain exterior to an obstacle. A more interesting

model is that for a vortex, as may occur, approximately, in emptying the bath,

where we could seek a two-dimensional flow in which the velocity is azimuthal,

i.e. in the 8 direction in plane polar coordinates (r, 8), getting faster and faster

as we approach the `core' of the vortex r = 0. An inspection of (5.3) shows that

a suitable potential, indeed the only one, is proportional to 0. We also note that

this flow provides a non-constant, albeit multiple-valued, solution of the Neumann

problem with homogeneous boundary conditions in a circular annulus centred

at the origin, and it gives an early warning that the connectivity of D may be

important in considering the uniqueness of solutions of elliptic equations.

5.1.4.2 Membranes

The easiest way to visualise solutions of Laplace's equation is by looking at the

shape of a membrane such as a drum, whose boundary is deformed slightly out of

plane. Like a soap film, the membrane is assumed to be capable of supporting a

tension T which is assumed to be isotropic, i.e. the same in all directions. Then a

normal force balance reveals that the displacement w satisfies 82w/8t2 = a2eV2w,

where ap = T/p, p being the surface density of the membrane; this is because

the components of the tension forces out of the plane add up to T multiplied by

the small mean curvature, which is approximately V2w. (In one dimension, this

model reduces to that for waves on an elastic string, which is just the familiar wave

equation (4.1).) Thus the equilibrium displacement is indeed a two-dimensional

harmonic function with Dirichlet boundary conditions, assuming the perimeter

is prescribed and nearly planar. Also, it is easy to see that the application of

a pressure difference p across the membrane enables us to visualise solutions of

Poisson's equation with p as the right-hand side.

5.1.4.3

Torsion

A less well-known, but no less important, model leading to the Laplace equation in

two dimensions arises in the study of torsion in linear elasticity. Torsion bars are

often used in motor car suspensions, and, in the simplest configuration, they are

metal circular cylinders which are stress free except for equal and opposite torques

about the axis which are applied at each end of the cylinder. To describe this we

will again eschew the conventional tensorial formulation of elasticity theory and

proceed on the justifiable assumption that the displacement from the unstressed

state in any cross-section of the torsion bar aligned along the z direction is a rigid

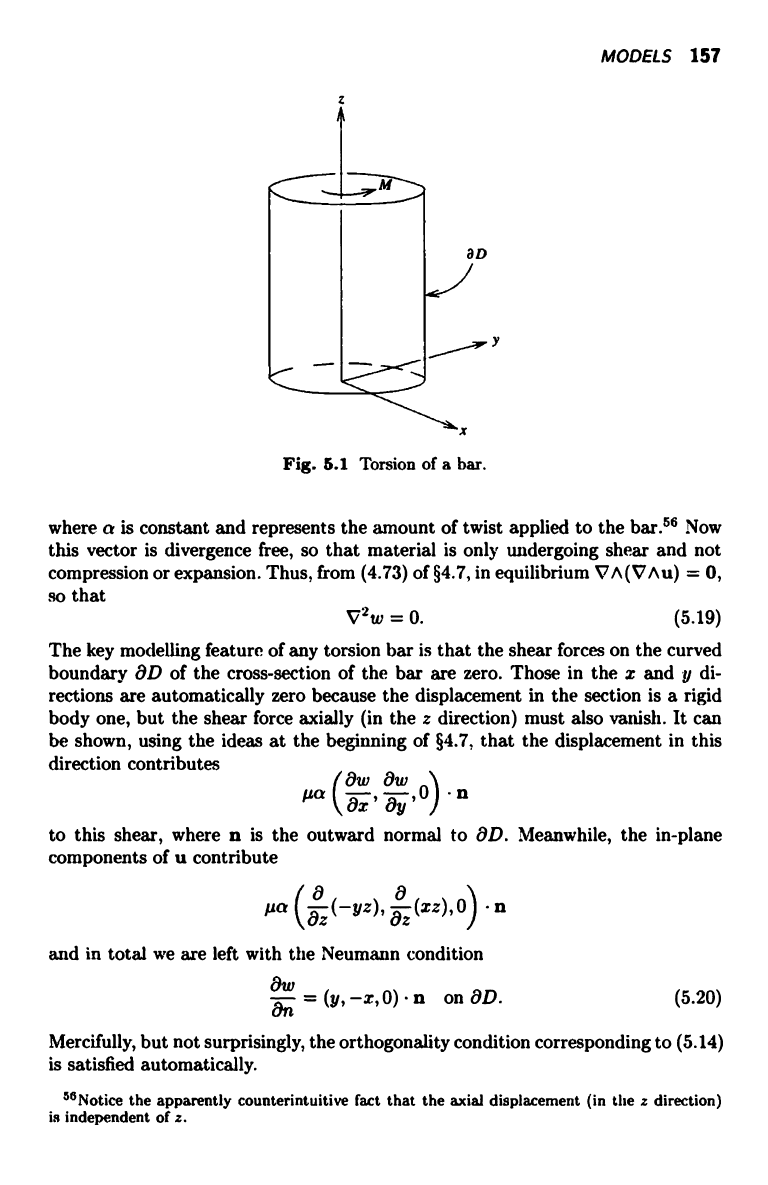
body rotation about the z axis which varies linearly in z (Fig. 5.1).

Hence we write the displacement as

u = a (-yz, xz, w(x, Y)), (5.18)

"This is in contrast to the paint flow of §1.1, where the paint adheres to the wall.

MODELS 157

Fig. 5.1 Torsion of a bar.

where a is constant and represents the amount of twist applied to the bar.56 Now

this vector is divergence free, so that material is only undergoing shear and not

compression or expansion. Thus, from (4.73) of §4.7, in equilibrium VA(VAu) = 0,

so that

V2w=0.

(5.19)

The key modelling feature of any torsion bar is that the shear forces on the curved

boundary OD of the cross-section of the bar are zero. Those in the x and y di-

rections are automatically zero because the displacement in the section is a rigid

body one, but the shear force axially (in the z direction) must also vanish. It can

be shown, using the ideas at the beginning of §4.7, that the displacement in this

direction contributes

8w 8w

8x ,

ey , 0

to this shear, where n is the outward normal to 8D. Meanwhile, the in-plane

components of u contribute

pa f 8z (-yz), z (xz),0) n

and in total we are left with the Neumann condition

= (y, -x, 0) - n on OD.

(5.20)

Mercifully, but not surprisingly, the orthogonality condition corresponding to (5.14)

is satisfied automatically.

58Notice the apparently counterintuitive fact that the axial displacement (in the z direction)

is independent of z.