Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations

Подождите немного. Документ загружается.

168 ELLIPTIC EQUATIONS

Continuous dependence on the data

This is a trivial extension of the comparison theorem when we let 91 -4 92 (from

above and below). A similar result describes the dependence of u on f.

This last result is the first real evidence that the Dirichlet problem for Poisson's

equation is in fact well posed. But we must remember that we have not yet proved

existence!

In this book we will not delve much further into the vast number of uses to

which the maximum principle and its generalisations can be put, although we note

that the principle can also be used when the dependent variable u satisfies suitable

differential inequalities, as we will see in §5.11, or more general elliptic equations.

The question of existence is the hardest of all and requires a lengthy chain of

arguments, of which the starting point is often the following methodology.

5.4

Variational principles

In certain circumstances, which occur almost as frequently as those under which

the maximum principle applies, it may be possible that the solutions of elliptic

equations are minima or other stationary points of a variational integral. Indeed,

in subjects such as thermodynamics and nonlinear elasticity, it is common to

model processes in terms of such minimisation procedures and only consider the

associated Euler--Lagrange equations as partial differential equation problems a

posteriori. This strategy has great advantages in the computer age, when discrete

approximations to an integral (as, for example, in the finite element method) are

very easy and convenient compared to discrete approximations of a differential

equation. However, in this book we always take the viewpoint that the partial

differential equation is the fundamental model.

As an example, supposing f is given and u minimises

E(u) = IL 12 (Vu12 + f (x, Y)u I dx dy, (5.42)

with or without boundary conditions, we would vary u to find

vn . Vu + qf) dx dy + Q(, 2)

E(u + n) - E(u) = IL

(

IL2u

dx dy + Q(r2 ),

neglecting boundary conditions. Because n is arbitrary, E can only attain a sta-

tionary value when u is a solution of Poisson's equation (5.35).

The advantages of working with mine E(u) are not confined to numerical algo-

rithms. For example, we could consider using any one of a number of optimisation

algorithms analytically to construct sequences such that E (un+1) < E (un).

Then, if we could prove that {un}, or at least a subsequence thereof, converges,

the limit would, in many circumstances, be a'weak solution' of Poisson's equation,

i.e. a function which could be proved to satisfy Poisson's equation when multiplied

GREENS FUNCTIONS 169

by an arbitrary test function and integrated by parts. If we could prove enough

about its regularity, we might then even be able to prove that this weak solution

was a classical one in that the left- and right-hand sides of Poisson's equation were

equal everywhere in D. This is not so unlikely because we know from Chapter

3 that elliptic equations cannot `propagate' singularities; all the singularities we

have encountered make their `presence' felt everywhere, but their effect away from

the boundary has always been very smooth, in fact analytic. Further details of

this procedure can be found in [12], where iterations other than those suggested

by E(u) are used as starting points."

We remark that variational principles have one of their most important prac-

tical applications in the study of eigenvalue problems for elliptic equations, as we

shall see briefly in §5.7.1.

5.5

Green's functions

Green's functions provide the most important technique for gaining insight into the

structure of solutions of linear elliptic equations. These functions are the analogues

of Riemann functions for hyperbolic equations, but whereas Rientann functions

are multidimensional generalisations of Green's functions for initial value prob-

lems for second-order ordinary differential equations, Green's functions for elliptic

equations extend the theory of two-point boundary value problems for ordinary

differential equations.

As usual, we begin with Poisson equations and, as in §4.2, we can proceed in two

ways, both of which reach the same conclusion. Either we can go through a fairly

lengthy analysis using classical functions, or we can take a short cut, requiring

more `infrastructure', by using generalised functions.

5.5.1

The classical formulation

We begin with the Dirichlet problem

V2u = f in D,

u = g

on OD,

(5.43)

where D is a smooth, bounded, simply-connected domain in R2, and we write

x = (z, y).

Our starting point is to recall that the two-point boundary value problem for

the ordinary differential equation

L 1

0 44)5

) = u(

u = )

= f(z), u( = , ( .

is formally solved by

uW = f f (x)G(x, E) dx,t

(5.45)

where

ebA common one is that resulting from time-stepping in an evolution equation of the type

considered in Chapter 6.

170 ELLIPTIC EQUATIONS

,CG = dal = 0

for x 5 £,

with G(0, i;) = G(1, i;) = 0, G continuous and

(5.46)

(5.47)

Equation (5.45) states that u is the integral of f over (0, 1) weighted by a Green's

function G which has a slope discontinuity at x = i; of just the right size to ensure

that

d2 1

I f (x)G(x, ) dx = f (C)

We can see that this is the case by simply multiplying (5.44) by G and (5.46) by

u, subtracting, and integrating over 0 < x <

1.66

Motivated by this result for ordinary differential equations, we seek a function

G(x, 4) such that, for each E E D,

V2G=0 forx#t. (5.48)

Now, as above, we multiply this equation by u and Poisson's equation (5.43)

by G, subtract, and integrate over D to try to `pick out' u(4). To do this we need

G to satisfy the following conditions.

1. We must have G = 0 on OD, or else we would be left with unwanted boundary

contributions involving Ou/On$&D.

2. We need G to have a suitable singularity at x =

analogous to the `kink'

in (5.47).

The second condition is difficult to motivate, but if we guess that this singularity

is isotropic, i.e. the behaviour of G is independent of the direction87 of x - E, we

are forced to try

G(x,4) = constant log lx - E1 + 0(1) as x - 4. (5.49)

With hindsight, we choose the constant to be 1/2r and now we apply Green's

theorem in the form

(u_c)ds=ff

(uV2G - GV2u) dx,

e(D_D,)

D-v,

"Precisely the same argument applies when

dz2

+P(x)_ +9(x),

as long as we replace CO in (5.46) by

C'G

= dx2 - dx

(p(x)G) + 4(x)G,

(5.50)

C' being the operator adjoint to C.

87This is unlike the situation in §4.2, where the characteristic directions were vital.

GREEN'S FUNCTIONS 171

where D, is a circle with centre E and radius a that has been excised from D.

Noting that 8/BnJ8D. _ -8/8r in polar coordinates centred at E, we find

,) (l

f) +0(1)) ed8

Cu- -

ds =

/p2 (u(4) (-2

5;i

(

27r

(5.51)

as e -> 0, for some Z such that It -

I < e. Substituting into (5.50) and using

(5.43), (5.48) and the data on OD, and taking the limit a -> 0, we end up with our

desired formula

uW = ff

G(x,

)f (x) dx +

(5.52)

18D

There are two immediate remarks to be made about this result.

It is easy to see that an equation similar to (5.52) applies to the Robin problem,

as long as G satisfies the homogeneous form of the Robin boundary condition.

Also it is easy to modify the argument to cater for other second-order elliptic

operators, except that when the operator is not self-adjoint, say when

we have to work with the adjoint operator defined by

VG = V2G - 8x (aG) -

(bG).

Although we have defined Green's functions in a framework analogous to that

for Riemann functions, their properties are very different: G depends on the

domain D, whereas R is independent of the initial curve. Moreover, G can only

vanish at isolated points, whereas R is zero for all x > £ and all y > ',. The heart

of the analogy is revealed by the `short-cut' approach, which we now describe.

5.5.2 Generalised function formulation

Precisely in line with §4.2.2, we could, instead of writing (5.49), define G to be

such that

V2G = 8x2 + e GG = 6(x - 4)

for 4 E D, (5.53)

where we recall 6(x -

= 6(x - C)6(y - i) is zero except at x = and is such

that f fD 6(x - 4) dx = 1. Then, assuming that G = 0 on 8D, we can derive (5.52)

in one line by assuming Green's theorem holds for ffD (uV2G - GV2u) dx. As

usual, we simply multiply (5.53) by u, Poisson's equation V2U = f by G, integrate

over D and subtract to get

172

ELLIPTIC EQUATIONS

IID (uV2G - GV2u) dx =

(u Gam)

ds =

fg(x)

f (u(x)a(x - ) - G(x, 4)f (x)) dx

(x,

)f (x) dx.

= u(f) - fL G

As discussed in §4.2.2, the justification for the use of Green's theorem is the nub of

the matter and further reassurance concerning its validity is given in Exercise 5.9.

We can now set out our general theoretical framework for an elliptic operator

G with adjoint C. Everything relies on the fact that, if, as functions of x,

CG(x) = d(x - 4) and Cu(x) = f (x),

then, with homogeneous boundary conditions, the identity

implies that

(G(x, t)Cu(x) - u(x)CsG(x, t)) dx = 0

(x, 4) f (x) dx.

uW = AD G

We can now make some more remarks.

Equation (5.53) emphasises the ancestry of all Green's functions that we outlined

in §4.4. They are such that a linear operator annihilates them at all points

except one. Moreover, if the operator C is self-adjoint with appropriate boundary

conditions, then G is symmetric, i.e.

G(x, C) = G(C, x).

To prove this simply set

CG(x,7I) = 6(x - t])

and use the fact thIL

(G(x, n)CG(x, ) - G(x, )C`G(x, ri)) dx = 0.

This fact is important when reconciling the views of people who boldly assert

that the solution of Poisson's equation with zero Dirichlet boundary data is

u(x) =

(5.54)

just aslong as G2G(x,

= 8(x-t), by simply differentiating under the integral

sign. Of course, their G is simply the `transpose' of G defined by (5.53) and,

for any self-adjoint operator, there is no confusion. Indeed, this argument shows

that when C iA C the Green's function satisfies CG = 6(x -

as a function of

4, as well as CG = 8(x - 4) as a function of x.

GREEN'S FUNCTIONS 173

When we extend (5.53) to Rm, we simply find that the singular part of (5.49)

is replaced by

-rm_2

form > 3,

where wm is the surface area of the unit sphere in R1, namely68

f mir"/2/(m/2)!,

m even,

wm = lx(m-1)/22m

((m

- 1)/2)!/(m - 1)!, m odd.

(5.55)

If D is infinite and u -+ 0 at infinity, G is precisely this function and it models

a point `charge' or point `mass'; this is just our comment before (5.1). When

m = 2, a physical interpretation of (5.49) is as the electrostatic field due to a

line charge as mentioned in §5.1.2. We also remark that the singularity in the

Green's function of an arbitrary elliptic operator takes the form above after the

equation has been put into canonical form.

The whole question of the existence of G is as vexed as that of the existence of

solutions for general elliptic equations, discussed briefly at the end of the last

section 89 Of course, if G does exist, formulae such as (5.52) provide a ready tool

to demonstrate the continuous dependence of the solution on the data. However,

there is one commonly occurring situation where G definitely does not exist and

the procedures above must be modified. Suppose we try to solve the Neumann

problem for Poisson's equation V2u = / as in (5.35), with 8u/8n = g(x) on

8D, and we know that (5.39) is satisfied. We know at once that G only exists

if the term that forces G to be non-zero, namely the right-hand side 6(x - 4)

of (5.53), is orthogonal to the constant eigenfunction. But 6(x - f) has a unit

integral so this can never happen. Fortunately, there are at least two ways out

of this difficulty. One is to give G an extra degree of freedom by choosing

V2GM

= 6(x - r;) + cb(x - +I),

pick c = -1 to satisfy orthogonality, and arrive at

uW - u(rl) = lID

IOD

(5.56)

he right-hand side is easily seen to be the sum of a function of t and a function

of q. Another way is to choose

"To prove this quickly, note that

,,m/2

= fm e"2 dx = w,n I

r'"-le-'2 dr,

where r = lxi.

'^ 0

69We remark that, if we have proved the existence of the solution of

a boundary value prob-

lem for an elliptic equation, we can use this information to deduce the existence of a Green's

function. Fbr example, for the Dirichlet problem for Laplace's equation in two dimensions,

G = (1/2a) log ix - 4 + GR(x,C), where GR, the regular part of the Green's function, satis-

fies the boundary value problem G2CR = 0 in D and CR = -(I /2w) log Ix - 41 on 8D.

174 ELLIPTIC EQUATIONS

V2Gtif = b(x - l;) + c 1,

(5.57)

using the fact that 1 is an eigensolutiou. Now pick -1/c to be the area of D to

ensure orthogonality and a formula similar to (5.56) emerges.

As mentioned after (5.52), our definition of G easily generalises to the Robin

problem where (5.36) holds with neither fi nor a equal to zero. If u satisfies

Poisson's equation with this condition, we simply set V2G = 8(x - f) with

aG +,6 OG/On = 0 on OD to ensure that fan (G Ou/On - uOG/On)ds can be

written in terms of G and g alone.

There is one other very important remark to be made about Green's functions

from the computational viewpoint. This is that, if we relax the boundary condi-

tions that we have imposed on G, we can always use (5.50) to relate the values of

u in D to integrals involving both u and Ou/On around 8D. Hence, by taking a

suitable limiting process, as in Exercise 5.13, we can derive a linear integral equa-

tion for the values of u or Ou/On on OD for any of the boundary value problems

involving (5.36). The form of this integral equation depends on the problem and

the way in which we manipulate (5.50), but it is the basis of the so-called boundary

integral method [6] for solving Laplace's equation and many other elliptic equa-

tions. Discretising the integral equation over the whole of OD gives fewer linear

algebraic equations to he solved than would be necessary with a conventional finite

difference or finite element discretisation, but the other side of the coin is that the

matrices resulting from the boundary integral discretisation are `full', in that they

have few zero entries.

It is with sadness that we must tell the reader that explicit formulas for Green's

functions rarely exist (see [34] for a catalogue). However, things seem a little better

than they are for Riemann functions; although we can only cope with a small

handful of equations, there are quite a few geometries that have enough symmetry

for us to be able to find G.

5.6

Explicit representations of Green's functions

5.6.1

Laplace's equation and Poisson's equation

We have seen that the Green's functions that are as well-behaved as possible at

infinity in Rl" are (1/2ir) logr and -1/4zrr for in = 2 and m = 3, respectively.

Things rapidly become more complicated when boundaries are introduced, as we

now see.

5.6.1.1

Circles and spheres: Dirichlet and Neumann conditions

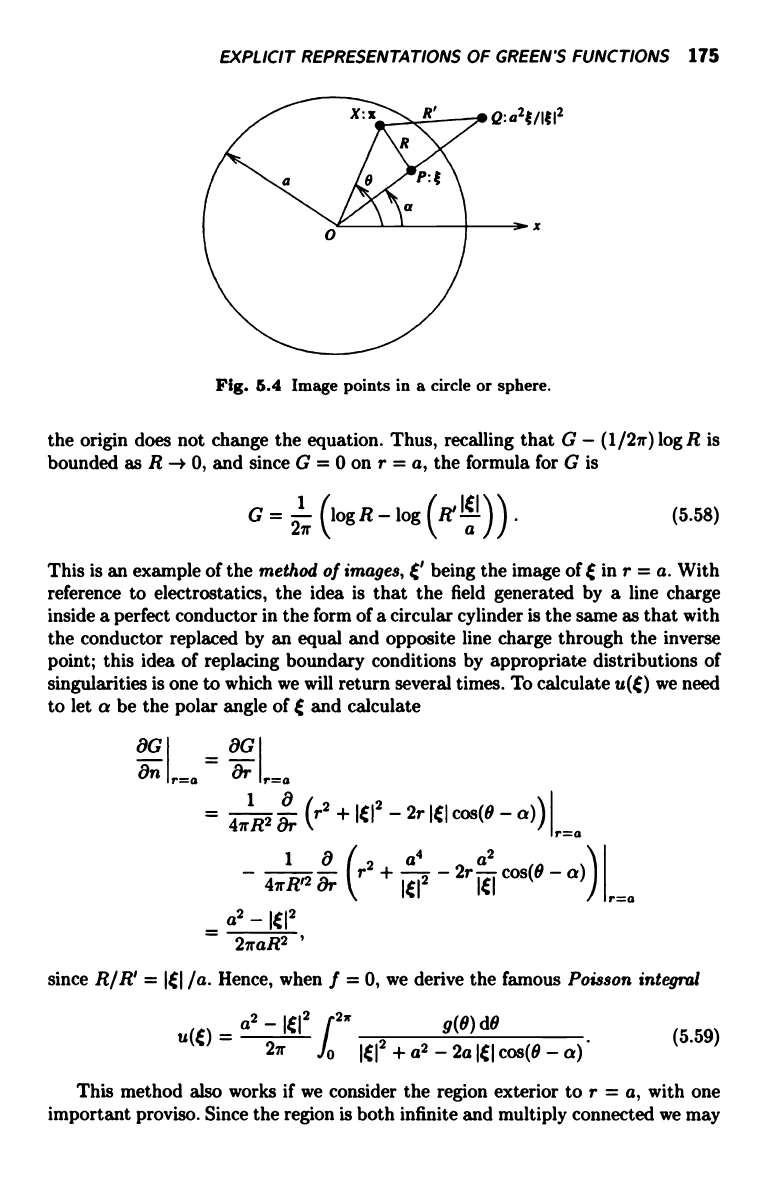
Suppose D is a circle with centre x = 0 and radius a in R. For the geometrically

minded, we can proceed by defining the point inverse tot by r;' = all;/412 and set

R = Ix - j and R' = Ix - 4'I, as in Fig. 5.4.70 Then R/R' = 141 /a when r = lxi =

a, and log R and log R' are both solutions of Laplace's equation since changing

"When X lies on the circle, triangles XOP and QOX are similar because they have a common

angle a-a between sides in the same ratio, as OQ/OX = OX/OP by the image condition; hence,

R = R'1tl/a when jxj = a.

EXPLICIT REPRESENTATIONS OF GREEN'S FUNCTIONS 175

Fig. 5.4 Image points in a circle or sphere.

the origin does not change the equation. Thus, recalling that G - (1/27r) log R is

bounded as R -+ 0, and since G = 0 on r = a, the formula for G is

G = 2_-

(logR-log(R'!i)). (5.58)

This is an example of the method of images,

' being the image of t in r = a. With

reference to electrostatics, the idea is that the field generated by a line charge

inside a perfect conductor in the form of a circular cylinder is the same as that with

the conductor replaced by an equal and opposite line charge through the inverse

point; this idea of replacing boundary conditions by appropriate distributions of

singularities is one to which we will return several times. To calculate u(t) we need

to let a be the polar angle off and calculate

- 8G)

r=a

47rR28r

(r2+IEI2-2rItlcos(9-a))

r=a

(r2+2_2rf1c059_)

4rrR'2 8r

a2_1 4 1 2

2,raR2

r=a

since R/R' = IEI /a. Hence, when f = 0, we derive the famous Poisson integral

u(f) =

a2 -

IFI2

9(B) dO

(5.59)

2'r

ICI + a2 - 2a ItI cos(9 - a)

This method also works if we consider the region exterior to r = a, with one

important proviso. Since the region is both infinite and multiply connected we may

176 ELLIPTIC EQUATIONS

need to take especial care to exclude eigensolutions. For the Dirichlet problem, it

is sufficient to say that u = o (log r) as r -4 oo so as to exclude the eigensolutions

log

and

(rm -

I (A cos nO + B sin nO),

wheren is an integer greater than zero, and A and B are constants.

No formula such as (5.59) is available for the Robin condition (5.13), but the

Green's function for the Neumann problem can still be represented by images.

We will leave the details to Exercise 5.17, where it is shown that when Its > a

the image system comprises two line charges inside the circle when we exclude

eigensolutions for which u = constant 9 + o(1) as r -+ oo. On the other hand, if

Its < a, we know there is no usual Green's function and we have to resort to (5.56);

the formula quoted in Exercise 5.18 is an example of this.

Since Fig. 5.4 could equally well be drawn in R1, the ideas of inversion can

often be used for spheres as well as for circles, with G = -1/47rr + 0(1) near the

singularity in spherical polar coordinates.71

For the next class of problems, Green's functions can be found by `turning a

handle' (in fact, by taking Fourier transforms).

5.6.1.2

Half-spaces

Suppose we have to solve Laplace's equation in y > 0 in R2, with Dirichlet data

u = gp(x) on y = 0 and suitable behaviour at infinity to ensure uniqueness. Then

we can either use images directly, or take the limit of (5.58), to find that

- 27r log IX - 4'1

so that the solution, which we write as7L uD(x, y), is

UD(X,Y)

9D(,)d

(5.60)

(x - l;)2 + y2

Note that uD (x, y), as defined by (5.60), also satisfies Laplace's equation for y < 0;

its normal derivative is continuous across y = 0, but UD itself has a jump of 29D

there. It also shows that the behaviour at infinity that we need is u = O(1/r) as

r2 = z2 + y2 -, oo.

We could have derived this result more methodically by introducing the Fourier

transform of uD,

uD(k,y) =

fuDxYei

91 We will not pursue this here, but there is another mysterious-looking connection between

inversion and Laplace operators in IR"'. This is that, if u(r, B1, ... , B,"_ 1) is a harmonic function

in R", with r being distance from the origin and being polar angles, then so is

r2-mu(n2/r,B1,...,8m_1). This result basically comes from separating the variables in polar

coordinates and noting that powers of r always appear as pairs of the form ra and

r-a-"+2.

721n this section, for ease of presentation, we use the Green's function to find u(x) rather than

u(4) as hitherto. There should be no confusion as G is symmetric.

EXPLICIT REPRESENTATIONS OF

GREEN'S

FUNCTIONS 177

using the postulated decay at infinity, to obtain

d2uD

- k2uD = 0,

dye

with

(5.61)

UD(k, 0) = 9D(k) = f

9D(x)e'kz dx.

Hence, assuming we restrict ourselves to real k,

UD(k, y) =

9D(k)e-Ikly

= 9D(k)H(k, y), (5.62)

say. Now the Fourier inversion of H can be

performed on the real axis to give

H(x,y)

r00

a ikZ-lklar dk =

a-ka' cos kx dk =

A z2 +

_ -

J ( y

2).

Hence (5.60) follows by the convolution theorem{; clearly

OG ,7=0

This method has the advantage of working for Neumann and Robin as well as

Dirichlet data, and in principle it can be used for any constant-coefficient elliptic

equation in a half-space.

If UN(x, y) is the solution of the Neumann problem in y > 0, in which

OUN/ay (x, 0) = gN(x) is given, it is easy to show that a Green's function is

G- 2a

(logIx - C + loglx-0)

so that

1 '00

1N(x,Y) =

9 N

+ y2)

(5.63)

/ o0

and an arbitrary constant can be added. Note that UN (X, y) can only be bounded

at infinity if f°°00 gN (t) dt = 0, which is the analogue of the usual solvability

condition in a finite domain. Note also that UN(x, y) satisfies Laplace's equation

for y < 0 and is continuous across y = 0, but that its normal derivative has a jump

of 29N(x). Moreover, this solution, which is a superposition of Green's functions

that are singular on the x axis, has a simple interpretation as a'source distribution'

in fluid mechanics, gravitation or electromagnetism.

Still in connection with the half-plane geometry, there is one very useful piece

of jargon that can be introduced. It is easy to see that the Fourier transform of

UN is

a Ikly,

UN(k,y) =

-LL

Jkl

and hence the Dirichlet and Neumann data for u are related by

§N (k) = - IkI9D(k).

(5.64)

This is an example of a so-called Dirichlet-to-Neumann map and it sheds further

light on the ill-posedness of the Cauchy problem for Laplace's equation in y > 0.