Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations

Подождите немного. Документ загружается.

188 ELLIPTIC EQUATIONS

products: with reference to (5.81), if G is self-adjoint and £4 = Aq(x)¢,a, where

0a is real, we simply find that, in one dimension, our basic Fourier transform

formula must be changed to

fa =

ff(x)q(x)A(x)dx,

f (x) = f

faoa (x) dA,

(5.88)

assuming now that Ox are normalised so that f q¢2 dx = 1. In terms of generalised

functions, this equation merely reflects the fact that (5.85) has become

f cba(x)ci (x')q(x') dA = 6(x' - x). (5.89)

For (5.87), the eigenfunctions are 0 = Jo(kr) and Yo(kr), where A = -k2,

0 < k < oo, and Jo and 'a are Bessel functions of zero order. The latter is

discarded because it is unbounded as r -+ 0 and we require the solution of (5.86) in

the region r >, 0. To conform with conventional usage, we label the eigenfunctions

with k = instead of A and, as in §4.5.2, write the Hankel transform

Ii(k, z) =

rJo(kr)u(r, z) dr;

we thereby obtainso

with

k2u = 0 for r > 0,

(5.90)

(5.91)

u(k,0) = g(k).

In order to write down the inversion formula for (5.90) from the recipe in §5.7.2,

we must first normalise Jo(kr). We have effectively done this in (4.48), but a

more direct procedure is to revert to a limit of the type considered in §4.4 and

consider functions v(r) defined on 0 < r < R, with v(0) bounded and v(R) = 0,

subsequently letting R -+ oc. The `Fourier series' corresponding to the operator

d/dr(r d/dr) is

v(r) =

EanJo(knr), (5.92)

where kn is such that Jo(kR) = 0 and

an =

f rv(r)Jo(knr)

/LR

(kr) dr .rJ

Now, letting R -4 oc and using the famous result that Jo(x) is approximately

2/ix cos(x - it/4) for large x, we find that (1/R) f rJo dr -+ 1/irk,,.

801f you prefer not to write this down at once from §5.7, simply evaluate

or or

S Jo(kr) ( (r )

r' 2 ) - u 1 ' (r B_ Jo(kr))

k2rJo(kr))1 dr = 0.

TRANSFORM SOLUTIONS OF ELLIPTIC PROBLEMS 189

Thus, since, for large k, the k are almost separated by x/R, the sum (5.92) can

be thought of as a Riemann integral, giving

v(r) -+ f °° kv(k)Jo(kr) dk,

(5.93)

as R -4 oo, where

rv(r)Jo(kr)dr;

(5.94)

v(k) = f

this is just the formula before (4.48). The asymptotic behaviour of Jo(x) for large

x means that no normalising factor needs to be introduced into (5.93), in the same

way that none would have been needed for Fourier transforms had we worked with

(1/ 2ir)e'kx instead of

elk,. Thus we can assert that81

u(r, z) =

OO kJo(kr)u(k, z) dk

and we can now solve our problem by substituting u = g(k)e

ka into this inversion

formula.

Reverting to our discussion at the beginning of this section, we could have

adopted a full-blooded approach and tackled the problem in r and z simultaneously

as a brute-force application of the ideas of §5.7. The eigenfunctions would have

been 4(r, z; kl , k2) = e'k't Jo(k2r), with kt and k2 real and positive, and writing

ci=

(r,z;ki,k2)u(r,z)rdrdz

would have given (ki - kz )Q, = 0. The only possibility would then be for ¢ to be

a generalised function82 with support at kt = k2 and this would also have led,

eventually, to the same result.

We also point out that there is another important method for many prac-

tical problems for Laplace's equation with axial symmetry. In (5.63), we super-

imposed two-dimensional Green's functions, but for (5.86) we can superimpose

three-dimensional ones to write the general solution as

(r, z)

r2 + (z - {)

(5.95)

for some suitable weight function g (we also note that this would be reminiscent

of the general solution (4.61) for the wave equation, were we to boldly replace aot

by iz). It is fairly easy to see (as in Exercise 5.33) that 0 decays at infinity and

that 0 = -2g(z) logr + O(1) as r -a 0.

"The equation (5.89) reads

O r'kJo(kr')Jo(kr) dk = B(r' - r);

anyone puzzled by this can see the explanation about normalisation after (5.98).

"The interested reader should verify that the only distribution f(x) that satisfies xf(x) = 0

for all x is a constant multiple of the delta function.

190

ELLIPTIC EQUATIONS

5.8.2

Laplace's equation in a wedge geometry; the Mellin transform

When the separation of variables strategy is applied to, say, Laplace's equation in

a wedge with data on 9 = 0 and 9 = a in polar coordinates, it leads us to the

eigenvalue problem

d20a

1 dO

dr2 + r dr = r2

for the function 0a describing the r dependence of u, because the only term involv-

ing a 0 derivative is (1/r2) 82/862. As in (5.87), we write this in the self-adjoint

form

(r d2) =rya,

(5.96)

with eigenfunctions rfk, where A = V. Suppose we require u to be bounded as

r -+ 0 and as r -+ oo. It is tempting to define u(k, 0) = fo rk-lu(r, 0) dr in line

with (5.88), but we could never define an inversion formula because of the difficulty

of normalising r*. This is an all-too-common situation in transform theory but it

can be overcome by the idea introduced in §4.4 of 'complexifying' the transform

as a function of k. In Chapter 4 we found that we could only obtain bounded

eigenfunctions of d2/dx2 as (xi -+ oo if A is real and negative; here we only get.

boundedness as r -> 0, oo if A in (5.96) is real and negative, i.e. if k is purely

imaginary. Hence, we define the Mellin transform by

u(k, 6) =

jr1_1u(r,0)dr

(5.97)

for suitable complex values of k. Instead of an inversion involving an integral like

fa rku(k, 6) dk, we expect

fn(k)r_cuk,9)dk;

u(r, 0) = (5.98)

here n(k) is another annoying normalising factor and the sign change in rk _+

r-k comes about because the fact that k is imaginary requires us to work with

conjugate inner products, as in Fourier transforms. We hope that by now the

reader is comfortable enough with generalised functions to say that

ioo

W)k-lr k dk = i

r°o (r,)-'eiklog(r'/r)

100

= 21rib(r' - r);

the penultimate step comes from (5.89) and the discussion before (4.29), and the

final one from the fact that, if f (x) is monotone and f (0) = 0, then b (f (x)) _

f'(0)I-16(x). Hence n(k) = 1/21ri. We remark that, in the same way that Fourier

transforms can be applied to functions that are not square-integrable by 'shifting'

TRANSFORM SOLUTIONS OF ELLIPTIC PROBLEMS 191

k up and down in the complex plane, so can (5.98) be made more widely applicable

by taking the inversion contour from c - ice to c + ioo for some appropriate real

constant c.

We can make our usual statement that, although the Mellin transform is usually

defined as (5.97) with k replaced by s, it would really be more self-consistent to

replace k by is (the same remark applies to the Laplace transform).

As an example, suppose that

2 2

52 +r8r+r 892 =0,

with

u=uo(r) onO=a

and

=0 onO=0,0<r<oo.

When we proceed as with the Hankel transform, we find that

2 -k2u=0,

with

and so

Thus

If, say,

u=uo(k) on 9=a,

dB =0 on8=O,

u(k,9) = uo(k)

cos k8

coska

(5.99)

u(r,O)

r_kuo(k)coskadk. (5.100)

uo =

f1, 0<r<1,

10, 1<r<oc,

we need to take ltk > 0 in (5.97), so that uo = 1/k and then, with k = irc,

1 r

cosh

sin(iclogr)d

the first term coming from the residue at k = 0.

*5.8.3 Helmholtz' equation

For this section only, we replace the k in Helmholtz' equation by unity, in order

to avoid a notational clash. In a half-plane, transform methods for Helmholtz'

equation, now written (V2 + 1) u = 0, are cursed by the presence of branch points

in the transform plane (which we still denote by k). For example, when Dirichlet

192 ELLIPTIC EQUATIONS

data u(x, 0) = g(x) is given on y = 0, the simple formula (5.62) for Laplace's

equation is replaced by

u(k,y) = g(k)e`y k `' (5.101)

and great care has to be taken over the definition of

k --1 and the appropriate

choice of inversion contour (remember we have to consider complex functions u).

We will content ourselves with just one transform solution which will be useful

later in this chapter and in Chapter 8. This concerns scattering of radiation by a

half-line and is called the Sommerfeld problem.

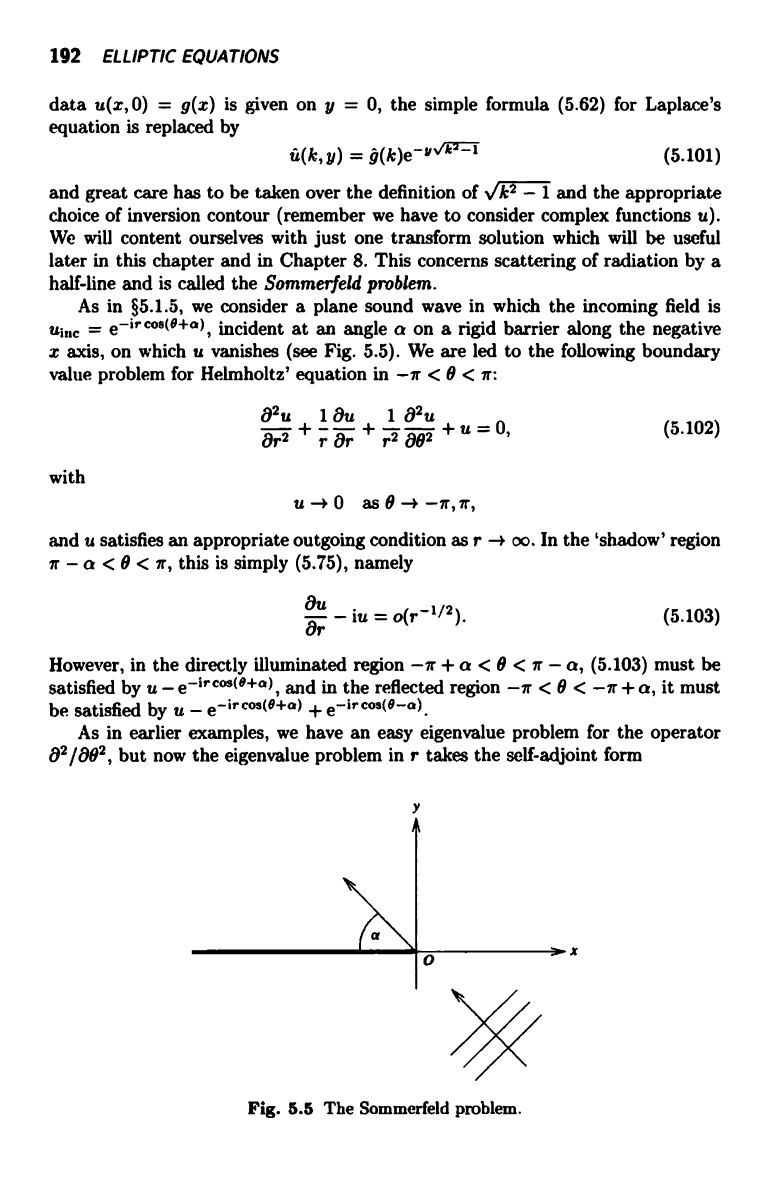
As in §5.1.5, we consider a plane sound wave in which the incoming field is

u4114c =

e-ircoe(e+a) incident at an angle a on a rigid barrier along the negative

x axis, on which u vanishes (see Fig. 5.5). We are led to the following boundary

value problem for Helmholtz' equation in -7r < 9 < 7r:

492U

Ore + r Or

r2 092

+ u = 0,

with

(5.102)

u->0 as8->-n,a,

and u satisfies an appropriate outgoing condition as r - oo. In the `shadow' region

it - a < 9 < ir, this is simply (5.75), namely

- iu = o(r-1/2).

(5.103)

However, in the directly illuminated region -lr + a < 9 < 1r - a, (5.103) must be

satisfied by u - e ircos(O+a), and in the reflected region -ar < 9 < -ir + a, it must

be satisfied by u - e-ircos(e+a) +

e-ircos(6-a)

As in earlier examples, we have an easy eigenvalue problem for the operator

02/092, but now the eigenvalue problem in r takes the self-adjoint form

Fig. 5.6 The Sommerfeld problem.

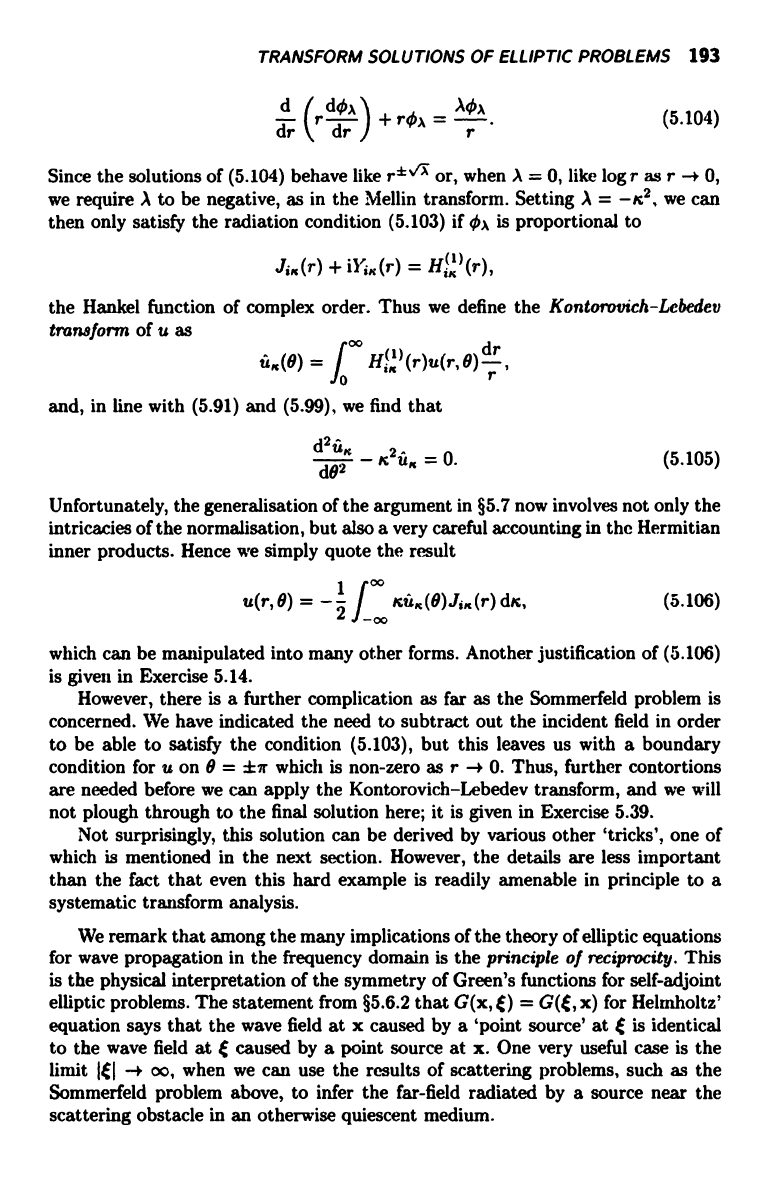
TRANSFORM SOLUTIONS OF ELLIPTIC PROBLEMS 193

dr (rddr) + rQA =

Ana

(5.104)

Since the solutions of (5.104) behave like

rt"a

or, when A = 0, like log r as r -+ 0,

we require A to be negative, as in the Mellin transform. Setting A = -K2, we can

then only satisfy the radiation condition (5.103) if ¢a is proportional to

JiK (r) + iY,, (r) = H 1 l (r),

the Hankel function of complex order. Thus we define the Kontorovich-Lebedev

transform of u as

U'K(e) =

(r)u(r,0)dr,

and, in line with (5.91) and (5.99), we find that

d2iK

d92 -

K2i1" = 0. (5.105)

Unfortunately, the generalisation of the argument in §5.7 now involves not only the

intricacies of the normalisation, but also a very careful accounting in the Hermitian

inner products. Hence we simply quote the result

u(r, 0) = - 2

Kfi,e (9)Ji, (r) dK, (5.106)

which can be manipulated into many other forms. Another justification of (5.106)

is given in Exercise 5.14.

However, there is a further complication as far as the Sommerfeld problem is

concerned. We have indicated the need to subtract out the incident field in order

to be able to satisfy the condition (5.103), but this leaves us with a boundary

condition for u on 9 = frr which is non-zero as r -+ 0. Thus, further contortions

are needed before we can apply the Kontorovich-Lebedev transform, and we will

not plough through to the final solution here; it is given in Exercise 5.39.

Not surprisingly, this solution can be derived by various other `tricks', one of

which is mentioned in the next section. However, the details are less important

than the fact that even this hard example is readily amenable in principle to a

systematic transform analysis.

We remark that among the many implications of the theory of elliptic equations

for wave propagation in the frequency domain is the principle of reciprocity. This

is the physical interpretation of the symmetry of Green's functions for self-adjoint

elliptic problems. The statement from §5.6.2 that G(x, 4) = G(4, x) for Helmholtz'

equation says that the wave field at x caused by a `point source' at 4 is identical

to the wave field at f caused by a point source at x. One very useful case is the

limit 141 -+ oo, when we can use the results of scattering problems, such as the

Sommerfeld problem above, to infer the far-field radiated by a source near the

scattering obstacle in an otherwise quiescent medium.

194

ELLIPTIC EQUATIONS

*5.8.4

Higher-order problems

We conclude this section with a brief discussion of a few points that may need to be

borne in mind when Green's functions and transforms are applied to higher-order

equations. The first concerns the biharmonic equation

V4u=0

for which the Green's function in two dimensions satisfies

VG = b(x - {)b(y -,I). (5.107)

One way to proceed in the whole of R2 is to invert the double Fourier transform

(kl + 2k? ki + k2) _', but it is more straightforward to look for radially-symmetric

solutions of

(-T2 +rdr) G=0 forr>O,

(5.108)

where r2 = (x - t)2 + (y - q)2. Hence G is a linear combination of r2, r2 log r,

log r and a constant, and we might then guess that, since

z2 +

r d

) (rz log r - r2) = 2a

log r'

we might have

8s'

(r2logr - r2) . (5.109)

However, to reassure ourselves that there should be no additional log r terms

in G, we need to compute V4 log r in accordance with the procedure described in

Exercises 4.13 and 4.21, where it was shown that V2(log r) =

We sim-

ply look at (V40,logr) = lime_,o f fr>E logrV4Odxdy for suitable test functions

vii. Since V2 log r = 0 when r > 0, repeated use of Green's theorem gives

(fir,V4logr) = limJJ

j'V4logrdxdy

e-+0

r>e

V40logrdxdy+1

_ (V21,)logeds

r>e

r_e

f _V2

ds)

r=e e

The second term on the right-hand side tends to zero and the last to -2nv2rk(0, 0)

as a - 0, which is a term we do not want, and hence there is no log r term in G.

The biharmonic equation may look to be a relatively benign generalisation of

Laplace's equation. Indeed, because

4 16V =

8z2 8z2'

its 'general solution' can be written down in two dimensions in terms of com-

plex variables as 3t(2f(z) + g(z)), where z = x + iy, 2 = x - iy and f and g

COMPLEX VARIABLE METHODS 195

are arbitrary analytic functions. However, it admits no maximum principle, and

the fact that it is fourth order often destroys the possibility of easily finding ex-

plicit eigenfunction representations. Also, the fact that most practical, and prob-

ably well-posed, boundary value problems only have two pieces of data on the

boundary of a closed domain D means that we can rarely expect to prove that

f fp uV4v dx dy = f fn vV4u dx dy; hence, even if we have a complete set of eigen-

functions in which to expand, they are not usually orthonormal (see Exercise 5.28).

Another kind of complexity arises when we consider vector systems of equa-

tions. One that we have encountered in §4.7.2 concerns the steady case of Maxwell's

equations in the form

VAH=j,

(5.110)

It turns out that, in practice, these equations often have to be solved for H given

both j and some physically relevant boundary conditions. Although we do not wish

to present a systematic discussion of vectorial Green's functions until Chapter 9,

we can point out a famous way of solving (5.110) using scalar Green's functions.

We remove the constraint V H = 0 by writing H = V A A, where A is called the

magnetic vector potential, and now we see that if we satisfy the `gauge condition'

V A = 0 we are simply left with a vector Poisson equation

V2A = -j.

Our by-now-familiar Green's function argument gives the solution as

fll

Cdx

A(4)

a x(x)

(5.111)

in Te, and, taking the curl, we find

H(F)

j(x) A (f $ x)

dx,

(5.112)

- F1

which is the famous Biot-Savart law of electromagnetism. If j is simply a current

in a wire, the calculation of H reduces to a single curvilinear integral.

5.9 Complex variable methods

At one level, it could be said that complex variable theory solves all problems for

Laplace's equation in two dimensions, because the general solution is the real part

of an analytic function of x + iy (or x - iy). Indeed, we can formalise this statement

by writing the solution of Vu = 0 in y > 0, with u(x, 0) = f (x), as a Fourier

inversion

from which we find

u(x, y) =

27r J

e-Ikz-Ikly f(k) dk,

u(x, y) = f+ (z - iy) + f_ (x + iy), (5.113)

where

196 ELLIPTIC EQUATIONS

f- (k) =

f(k),

k > 0,

f- (k) =

0, k > 0,

0, k < 0, f (k),

k<0.

It is interesting to compare this result with (4.67) for the wave equation; in the

same vein, we can write the solution of the three-dimensional Laplace's equation

in z > 0, with u(x, y, 0) = f (x, y), as

°°

u(x, y, z) =

e-'(k1Z+k2V)-k: f(ki, kz) dk1 dk2,

°°

foo

where k2 = k2 + kz and k > 0. Transforming to polar coordinates, we obtain

2a °°

u(x y z) =

ik(..0+vginb-iz)F(k

¢)dkdo,

o o

where F = 21rk j. Making the observation that led to (4.68), we derive the repre-

sentation

`2w

u(x, y, z) =

F(x cos Ji + y sin o - iz, i) do.

(5.114)

When complemented by a similar formula with z replaced by -z (or, equivalently,

one in which 0 is shifted by 7r), we retrieve the general solution of Laplace's

equation in three dimensions as a single integral. We also make the following

remarks.

If u is axisyminetric about the z axis, we can write

F(x cos o + y sin ii - iz, o) = G(z + it cos(9 - o)),

where x = r cos 0 and y = r sin 0, and hence that

u(x, y, z) =

fG(z+ircos)d;

(5.115)

clearly 7rG(z) = u(0, 0, z).

We could relate these ideas to the three-dimensional generalisation of (5.95) on

p. 189, namely

n) d dq

(x, y, z) =

f 00

+(x-S)2+(y"'I)2.

However, this representation involves convolution integrals with functions that

are complex versions of those in (4.65). Here we simply mention that (5.95) is

singular at the singularity distribution along x = y = 0. If we required an ax-

isymmetric potential function that was analytic on the z axis, we could consider

fs+ir

9(f) dl;

f-ir

r2 + (z - t)2

which would immediately retrieve (5.115) above. Note that this approach does

not work for source distributions in two dimensions, such as (5.63) on p. 177.

But, in two dimensions, we can simply resort to (5.113).

COMPLEX VARIABLE METHODS 197

These observations are intriguing but, alas, they are usually of little help in

solving practical boundary value problems. Instead, we must usually resort to the

Green's function and transform techniques of the previous sections. Nevertheless,

as we now explain, there are some powerful constructive complex variable methods

that are especially valuable for Laplace's equation in two dimensions.

5.9.1

Conformal maps

Three of the most distinctive features of an analytic function f (z) of a complex

variable z = x + iy are as follows.

1. Its real and imaginary parts are harmonic functions, he. they satisfy Laplace's

equation in x and y.

2. As long as f'(z) 54 0, f (z) maps regions from the z plane into the plane of

(= f (z) such that mapped curves intersect at an angle equal to that between

the original curves.

3. Laplace's equation in R2 is `conformally invariant', because when we write

(_ (+ill= f(z) = f(x+iy)

we find

axe+ay =If'(z)!2(g

+al

These facts are of profound significance to the solution of Laplace's equation and

its `square', the biharmonic equation. We have already mentioned that, if a partial

differential equation is invariant under certain classes of transformations, we may

be able to perform some simplifications, but here the fact that the transformations

involve functions of a complex variable makes things especially interesting. One

consequence is that we can conformally map Laplace's equation problems into

other Laplace's equation problems, although the boundary conditions may not

be invariant under the map. However, one of the most sensational implications is

that, if we have to solve Laplace's equation in a simply-connected closed domain D

with prescribed Dirichlet, Robin or Neumann data, and we can explicitly map D

conformally into the interior or exterior of the unit circle, or a half-plane, then we

can use our knowledge of Green's functions and/or transforms to solve the mapped

problem. The catch here is that we must find the conformal map explicitly; its

existence is known from the Riemann mapping theorem,83 although extra care

must be taken when using this theorem for multiply-connected regions.

One immediate corollary is that Green's functions for Dirichlet and Neumann

problems map into Green's functions. Considering the Dirichlet problem for sim-

plicity, suppose ( = f (z, zo) maps the domain D into the unit circle in the ( plane,

with z = zo going into

0, so that the ( plane Green's function is (1/27r) log

Then we claim

G(z, zo) =

log If (z, zo) I

(5.116)

83 We will say more about this in Chapter 7.