Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations

Подождите немного. Документ загружается.

218

ELLIPTIC EQUATIONS

the 'time-dependent' and 'energy' concepts can be made by using the energy as a

Lyapunov function, as in the theory of ordinary differential equations.

This discussion provokes two other questions. The first concerns the possibility

of `spontaneous' singularities appearing in the solution domain.

5.11.3.2 Singularities

We know from our general discussion in Chapter 3 that singularities in the solution

of elliptic problems cannot propagate, in that they cannot exist on manifolds of

dimension one fewer than the space of independent variables, but there is nothing

to prevent more isolated singularities occurring. Indeed, for m > 3 in (5.159) and

(5.160), it can be shown that there is a value A.. such that there are solutions u

and parameters A with u tending to infinity at some interior point of D as A -+ Ate.

Additionally, the singular solutions U are weak

in the sense that

V' DU dx = \'e' dx

for all test functions tii in some suitable space. We can see this explicitly for the

symmetric case98 (5.161), when A,,. = 2(m - 2) and U = -2logr.

The discussion above raises the question of the relationship between the pos-

sible forms of the response diagram when umax is large and the growth of f for

equations of the form

V2u + \f(U) = 0, (5.162)

with, say, zero Dirichlet data. In particular, if f grows as a power as u -a oo, how

does the response depend on this power and the dimension m? It is easily seen

by direct integration that, when m = 1 and f (u) /u -4 oo as u - oo, then there

are large solutions for small A, as in Fig. 5.7(a). More generally, and motivated by

the analysis of (5.161), it can be shown that, for smooth, bounded, m-dimensional

regions D, the following scenarios are possible.

1. If f grows linearly, with f (u)/u -; K > 0 as u -+ oo, there are large solutions

for A close to P/K, where p is the principal eigenvalue for

V2¢ + 110 = 0

in D

with

0 = 0 on OD.

(5.163)

2. If the growth is sublinear, so that f (u)/u -> 0 as u -+ co, and f (u) > 0, then

u exists and umaz is large for large values of A.

3. If f (u) is identically a power, say f (u) = uV with p > 1, the form of the response

diagram depends crucially upon the relation between p and m. In this case, there

is always the trivial solution u _ 0. For m = 1 or 2, or p < (m+2)/(m-2) with

m > 3, there is a non-trivial solution for all A. For p > (m+2)/(m-2) with m >

3, there is no non-trivial solution. The special value, p = pc _ (m+2)/(m-2), is

called the critical Sobolev exponent. This case is distinguished in that it allows

981t can be shown that A 0 < A' for 3 < m <, 9, and Ao, = A' for m >, 10. In two dimensions

there is an apparently similar situation, where there is a solution satisfying the equation for

0 < r < I and the condition at r = 1, and having logarithmic growth as r -, 0. This singular

solution is not a weak solution.

NONLINEAR PROBLEMS 219

us to proceed more easily in solving the equation that corresponds to (5.161)

via a phase-plane analysis (see Exercise 5.48).

5.11.3.3

Non-uniqueness and bifurcations

The discussion above has revealed how easy it is for solution branches of nonlinear

elliptic equations to cease to exist or tend to infinity at critical values of the control

parameter A. Another kind of pathology is that of bifurcation, by which we mean

the branching of a new solution from another `reference' solution as the parameter

is varied.

These kinds of behaviour can be approached systematically by careful exami-

nation of the local dependence of the solution on the parameter. Let us begin with

the simplest case of the nonlinear eigenvalue problem

V2u+Af(u) = 0

in D

with

u = 0 on OD,

(5.164)

in which f(0) = 0 and f'(0) 96 0, and let us see whether any solutions can exist

near the trivial solution u = 099 The key step is to search in the vicinity of a

particular value of A, say Ao, by writing A - Ao = e and, in the first instance,

expanding u in the form

u = UO + EUj +F

U2+ ... ;

(5.165)

thus we are effectively seeking the derivative of u with respect to A. Equating the

coefficients of like powers of c, we soon see that

V2ut + Ao f'(0)u1 = 0 in D

with Ut = 0

on OD,

so that ut can only be non-zero if -Ao f'(0) is an eigenvalue of the Laplacian in

D, with corresponding normed eigenfunction 0. Writing ul = ao, where a 96 0,

we find that u2 satisfies

OZU2 + Ao f'(0)u2 + 2 Aoa2 f"(0)02 + a f'(0)o = 0.

Hence, by the Fredholm Alternative, U2 can only exist if a satisfies

(5.166)

dx = 0. (5.167)aAo f"(0)

¢3 dx + f'(0)

IDIIf

fD 03 dx # 0, we then have what is called a transcritical bifurcation from the

zero solution. The bifurcation solution is locally, for A near the eigenvalue Ao, an

eigenfunction aqs of known amplitude. Moreover, the discussion of §5.7.1 shows that

bifurcation at the principal eigenvalue leads to one-signed bifurcation solutions (see

Exercise 5.49).

When 1(u) = sin u, (5.164) is a model for the buckling of an elastic strut (the 'Euler strut'),

where u is the transverse displacement and a is the compressive load applied along the strut.

220 ELLIPTIC EQUATIONS

Now let us look at cases of (5.164) where we have a non-trivial reference solution

uo(x, A). Adopting the same notation as in (5.165), we now find

`2u1 + Aof' (uo(x)) ul = _f (tza(r))

in D

with

ul = 0

on 8D.

(5.168)

Hence there is a unique solution for uo unless Ao is an eigenvalue of the (less trivial,

because of the x dependence in the coefficient of ul) eigenvalue problem in which

the right-hand side of (5.168) is set equal to zero. If A0 is such an eigenvalue, there

are two possibilities.

The most likely is that f (uo) is not orthogonal to the eigenfunction 0 corre-

sponding to Ao. In this case ul does not exist and any solutions that are `close' to

uo cannot be found by the prescription (5.165). What this means is that we must

seek a more general representation for u, say in the form

u=up+IEI112u'1 +E u2+

, (5.169)

and this is precisely the behaviour we saw near the turnover points A = A* in the

previous section. Locally the solution depends on IA - A011/2, and no bifurcation

occurs. Indeed, we could say that A - A0 is a locally smooth function of maxD uo.

The second possibility is that

O(x) f (uo(x)) dx = 0.

In this case, by the Fredholm Alternative, there is a continuum of solutions of

(5.168). Noting that the derivative of (5.164) with respect to A gives that

00 + Af'(uo)

_ -f (uo),

we see that 8uo/8A is a particular integral of (5.168) which vanishes on 8D, and

hence that

Ao + a¢,

where a is again an arbitrary constant. The problem for u2 is now

02u2 + Aof '(uo)u2 + (8A

CIO)

(f'(uo) + Aof"(uo)

co)

However, by differentiating (5.164) twice with respect to .\ and using the Fredholm

Alternative in reverse, we find that (8uo/8A) f'(uo) + (Ao/2)(8u0/8A)2 f"(uo) is

orthogonal to ¢, and hence a third application of the Alternative to (5.166) shows

that either a = 0 or

02 (f'(llo) +Aof"(uo) 8A) dx/ ID 1 AoO3 f"(uo) dx. (5.170)

The case a = 0 just

means that (5.165) is the Taylor expansion of uo (x, A) about

A = Ao, and is analogous to the smooth continuation of the zero solution in the

case f (0) = 0. Hence (5.170) corresponds to another transcritical bifurcation.

LIOUVILLE S EQUATION AGAIN 221

We notice that either (5.167) or (5.170) fails when certain integrals vanish

(fD (a f"(uo) dx for (5.170)), and in this case we again have to revert to a repre-

sentation like (5.169). However, this will not now lead to a turnover in the response

curve, but rather a `transverse' bifurcation in which the bifurcating solution branch

is perpendicular to the reference branch; this is called a pitchfork bifurcation.

We conclude with the observation that sometimes it is possible to show that

at most one solution exists to (5.164). We illustrate this by showing that there is

at most one positive solution if f (u)/u is a strictly decreasing function. We must

make the assumption that all solutions are smooth, and, if there are two solutions,

then they intersect at a reasonably regular surface. We suppose that u and v are

two distinct, positive solutions. Then u - v is positive in some region D+ and

vanishes on aD+. Integration by parts over D+ yields

f (vf(u)

- uf(v)) dx = faD+ u

(av - aud8.

\ an

an J

Since the left-hand side is negative while the right-hand side is non-negative, the

assumption is contradicted.

5.11.3.4

Other irregular behaviour

We have already noted, in §5.11.3.2, that solutions to nonlinear equations may be

infinite at some points whose position is not known in advance, unlike solutions of

linear problems whose interior singularities are determined by the coefficients in

the equations. However, two weaker types of irregularity can arise. For example,

if we look at the radially-symmetric solution of V V. (IVulVu) - 1 = 0, then IDul =

O(r3/2) for r -+ 0. The solution, although differentiable everywhere, is not twice

differentiable at the origin. Another type of behaviour, which will be considered

further in Chapter 7, is exhibited by semilinear problems in which f (u) is not

Lipschitz continuous, such as

V2u=up inD

with

u=g>0 on8D

and 0 < p < 1. If the region D is big enough, it can be shown (see Exercise 5.50)

that there is a `dead core', defined to be a region contained in D, say Do, in which

u = 0. Outside Do, u is positive. On the boundary BDo, 8u/8n as well as u is

zero, and clearly this solution is not analytic on BDo.

5.12 Liouville's equation again

We conclude this chapter by pointing out a remarkable relationship between the

elliptic version of Liouville's equation in two dimensions and Green's functions.

Suppose we return to §5.9.1, and consider the problem for the Green's function

G(z, zo) for Laplace's equation in a closed region with Dirichlet boundary data.

Let us assume that the region can be mapped conformally onto (J

1 by the map

( = P4

We assumed earlier that the point zo is mapped onto ( = 0, so the mapping is

different for each choice of zo. Here, we will let zo vary, so we assume that this

222 ELLIPTIC EQUATIONS

point is mapped to Co and keep f fixed. Then, using the mapping of (5.117) to

map the unit disc onto itself, the Green's function is simply

G(z' zo) =

log

1 -

(coo

= 2a (log Iz - zol + H(z, zo)),

say. Now set H(z, z) = T(z) so that,10° taking the limit z -> zo,

T(z) = log

If'(z)I

l {5.171)

1-If(x)12/

Then it is easy to show that

V2T(z) = -V2log(1

- If(z)I2)

= -4If'(z)I2

log(1- CC)

a( a

= 4e2T,

which, with a trivial change of variable, is Liouville's equation (5.159). Clearly,

the argument above suggests that (5.171) is the general solution to this nonlinear

equation, but we knew this anyway from the discussion in 0.8.3. Writing u =

2T+log2 and

V2u = 4eu

82u

= eu

8z02

and formally setting X = if (z) and Y = if (z) and ry = iv, we find that

u = 2log

21f'(z)I 1

1-If(z)I2

which is (5.171)!

5.13 Postscript: V2 or -0?

Many textbooks, especially the more theoretical ones, use the symbol A for the

Laplace operator, and write Laplace's equation as

-Au=0

rather than V2u = 0. Apart from the different notations for the Laplacian, there

are theoretical reasons for introducing the minus sign, as it automatically ensures

100The function H(z, zo), which can be loosely called the 'regular part of the Green's function',

has many important applications (see Exercise 5.52). The function T(z) Is known as the Bergman

kernel function.

EXERCISES 223

positivity of many quantities of central importance. For example, when Poisson's

equation is written -Du = f, with u = 0 on OD, positive data f gives a positive

solution u. Likewise, the Green's function for the operator -A with homogeneous

boundary data is positive. as are its eigenvalues and 'Fourier symbol' Jk12. It is

also often natural to think of an elliptic equation as arising out of the large-time

behaviour of an evolution problem such as the parabolic equation au/at - V2u =

f, which again leads to -Au = f. Set against this, the vast majority of 'end-

users' of partial differential equations conventionally write V2 and think of Green's

and Riemann functions as the solutions of 'Lu = 6', and we have followed that

convention in this book.

Exercises

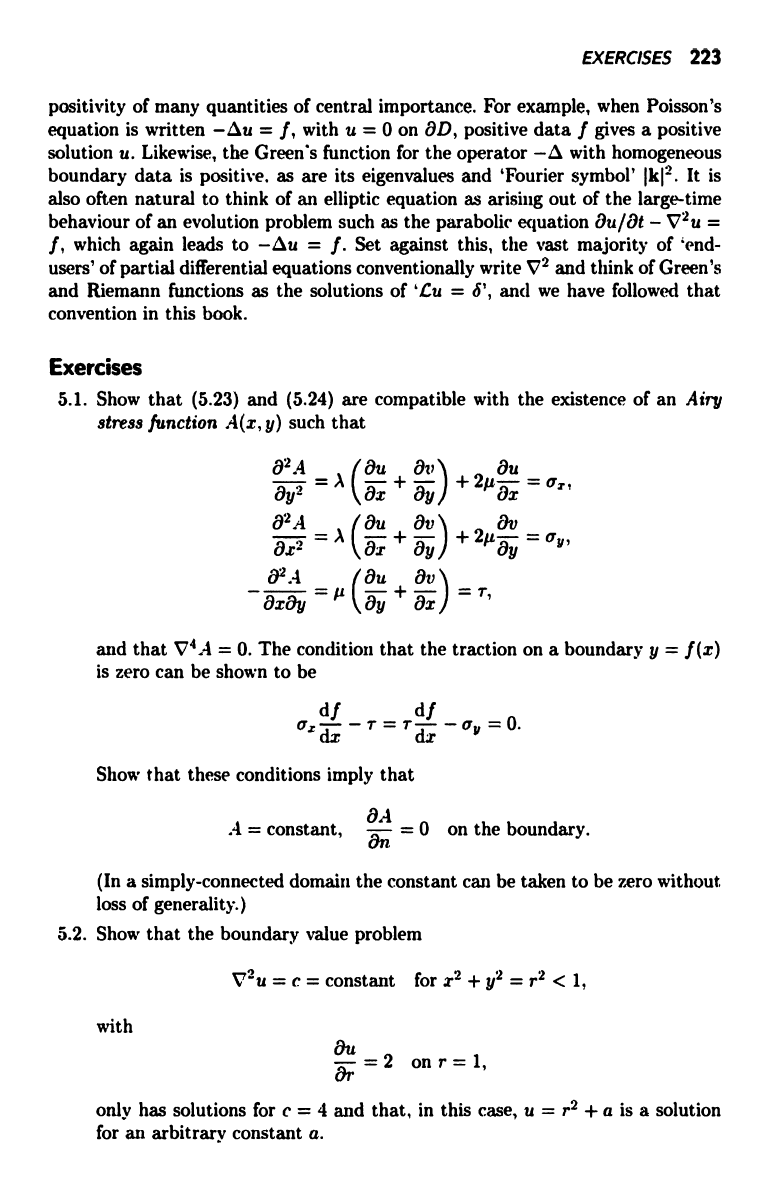
5.1. Show that (5.23) and (5.24) are compatible with the existence of an Airy

stress function A(x, y) such that

'u 9u

\(ax+ay)+2p-

o:,

62A

av 1 av

7x-

ax+ay+2,uay

a2L

(aY

av 1

ax a + ax

= r,

= ay,

and that V4A = 0. The condition that the traction on a boundary y = f (x)

is zero can be shown to be

asLf-T=rLf-sy=0.

Show that these conditions imply that

4 = constant,

= 0

on the boundary.

(In a simply-connected domain the constant can be taken to be zero without

loss of generality.)

5.2. Show that the boundary value problem

V2u=c=constant forx2+y2=r2 <1,

with

5T=2 onr=1,

only has solutions for c = 4 and that, in this case, u = r2 + a is a solution

for an arbitrary constant a.

224

ELLIPTIC EQUATIONS

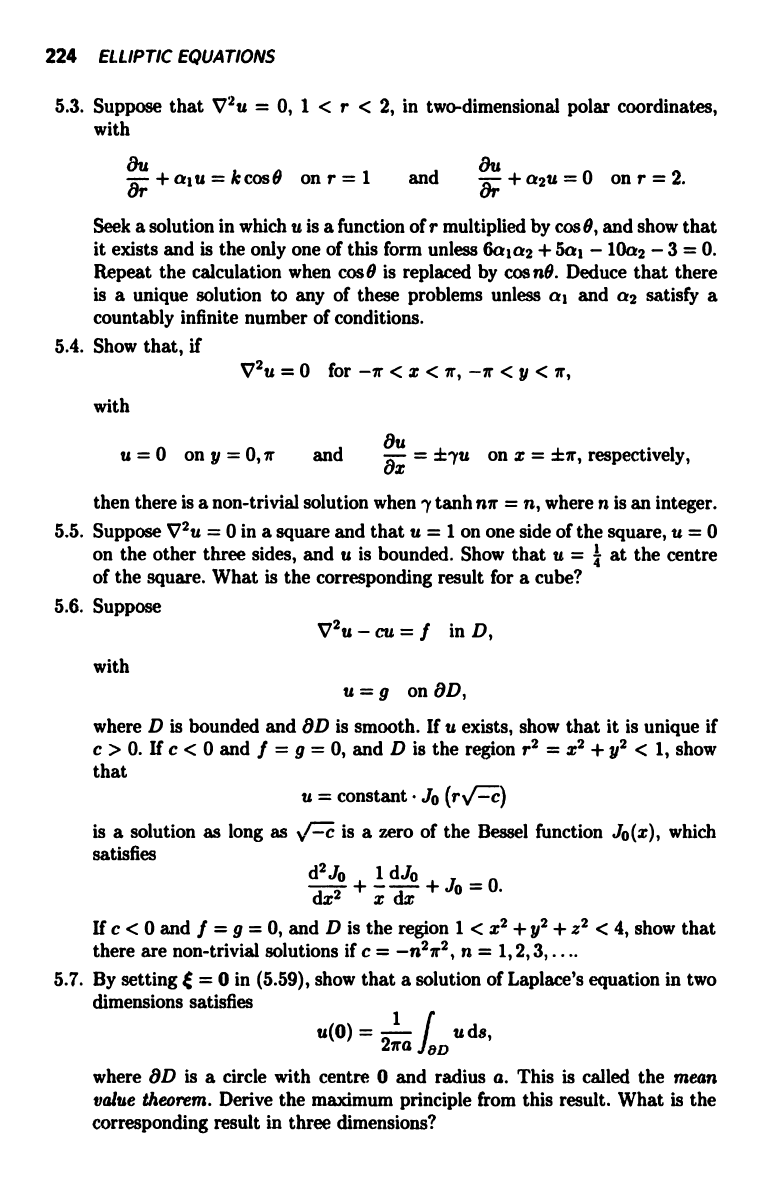
5.3. Suppose that V2u = 0, 1 < r < 2, in two-dimensional polar coordinates,

with

+alu=kcos9 onr=1

and

&+a2u=0

onr=2.

Seek a solution in which u is a function of r multiplied by cos 9, and show that

it exists and is the only one of this form unless 6a1a2 + 5a1 -10x2 - 3 = 0.

Repeat the calculation when cosO is replaced by cos n9. Deduce that there

is a unique solution to any of these problems unless al and a2 satisfy a

countably infinite number of conditions.

5.4. Show that, if

V2u=0 for-ar<x<ar,-ar<y<ar,

with

u=0 on y = 0, ar

and 8x = ±yu on x = far, respectively,

then there is a non-trivial solution when ry tanh nar = n, where n is an integer.

5.5. Suppose V2u = 0 in a square and that u = 1 on one side of the square, u = 0

on the other three sides, and u is bounded. Show that u = a at the centre

of the square. What is the corresponding result for a cube?

5.6. Suppose

V2u-cu= f

in D,

with

u=g on OD,

where D is bounded and OD is smooth. If u exists, show that it is unique if

c > 0. If c < 0 and f = g = 0, and D is the region r2 = x2 + y2 < 1, show

that

u = constant Jo (rte)

is a solution as long as is a zero of the Bessel function Jo(x), which

satisfies

d2Jo

1 dJo

dx2 + x dx

+ Jo =

If c < 0 and 1=9 = 0, and D is the region 1 <x2 + y2 + z2 <4, show that

there are non-trivial solutions if c = -n2ar2, n = 1,2,3,....

5.7. By setting E = 0 in (5.59), show that a solution of Laplace's equation in two

dimensions satisfies

u(0)=2

uds,

where 8D is a circle with centre 0 and radius a. This is called the mean

value theorem. Derive the maximum principle from this result. What is the

corresponding result in three dimensions?

EXERCISES 225

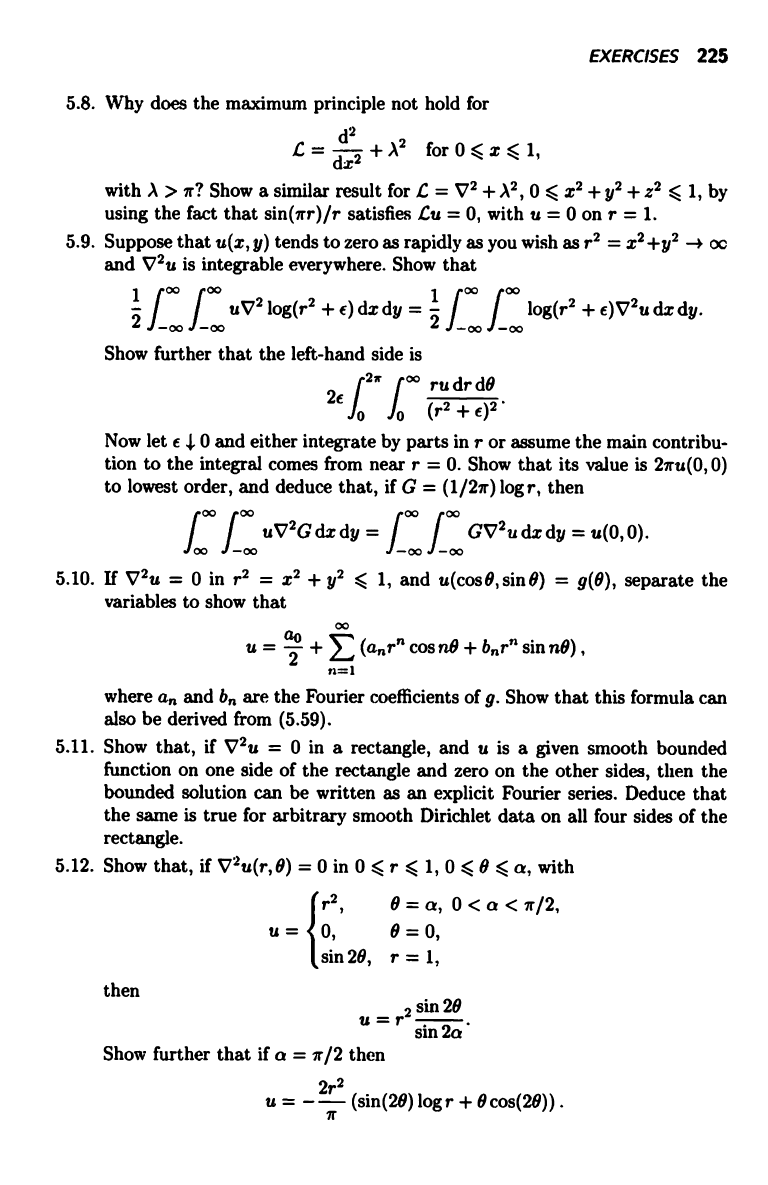
5.8. Why does the maximum principle not hold for

G=dx2+A2 for0<, x<, 1,

with A > 7r? Show a similar result for C = V2 + Al, 0

x2 + y2 + z2 '< 1, by

using the fact that sin(irr)/r satisfies Cu = 0, with u = 0 on r = 1.

5.9. Suppose that u(x, y) tends to zero as rapidly as you wish as r2 = x2+y2 -4 00

and V22u isrintegrable everywhere. Show that

u02 log(r2 + E) dx dy = 1 f I

log(r2 + E) V2u dx dy.

2 J

o0 00

0o J oa

Show further that the left-hand side is

ru dr dB[00

J r2+ E2

0 ( )

Now let e 10 and either integrate by parts in r or assume the main contribu-

tion to the integral comes from near r = 0. Show that its value is 27ru(0, 0)

to lowest order, and deduce that, if G = (1/27r) log r, then

LL:

uV2Gdxdy =

L:cV2udxdy

5.10. If V2u = 0 in r2 = x2 + y2 < 1, and u(cos8, sin 9) = g(O), separate the

variables to show that

u = 2 + 1: (anr" cos n8 + bnr" sin n9)

n=1

where an and b" are the Fourier coefficients of g. Show that this formula can

also be derived from (5.59).

5.11. Show that, if Vu = 0 in a rectangle, and u is a given smooth bounded

function on one side of the rectangle and zero on the other sides, then the

bounded solution can be written as an explicit Fourier series. Deduce that

the same is true for arbitrary smooth Dirichlet data on all four sides of the

rectangle.

5.12. Show that, if V2u(r, 9) = 0 in 0 <, r < 1, 0 <, 0 < a, with

r2,

0 = a, 0 < a < 7r/2,

0, 8=0,

sin 20,

r = 1,

then

2 sin 20

u = r

sin 2a

Show further that if a = a/2 then

u = -

(sin(20) log r + 0 cos(20)) .

226

ELLIPTIC EQUATIONS

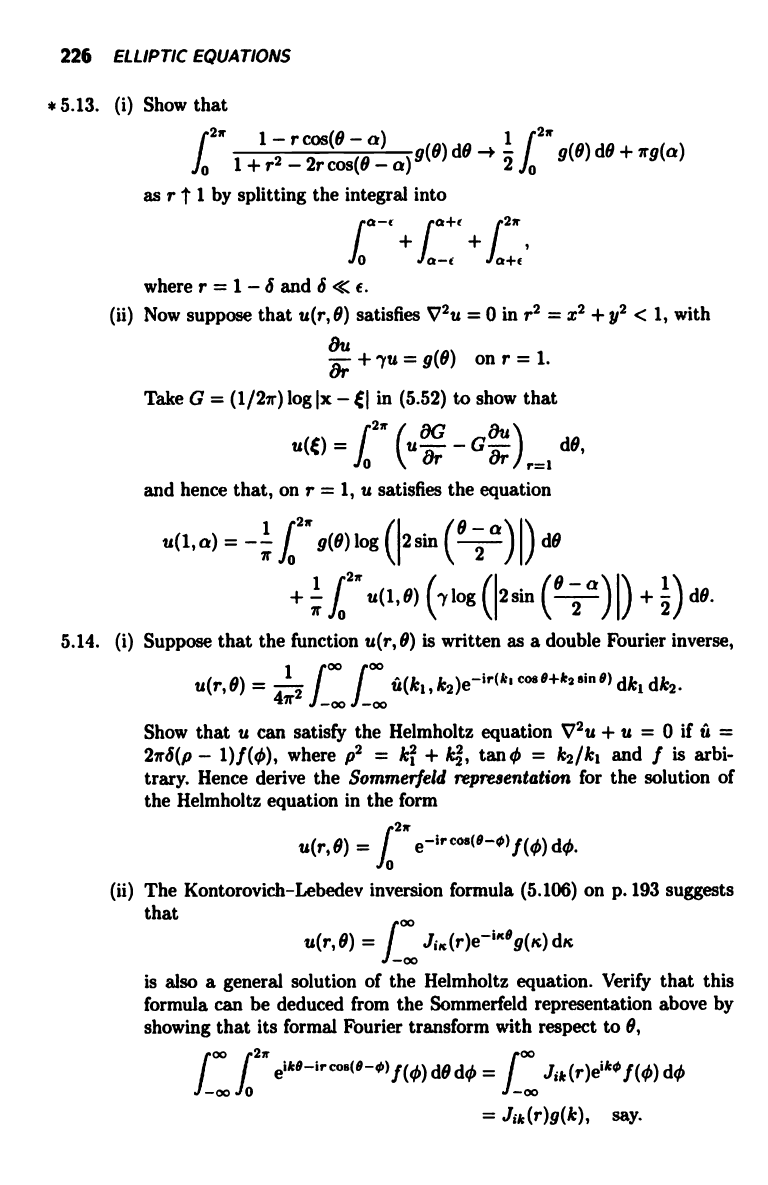
* 5.13. (i) Show that

f 21r

1- r cos(9 - a)

g(8) dO ->

1 J

2,r

g(O) dO + lrg(a)

1+r2-2rcos(O-a)

as r 1 1 by splitting the integral into

J0Q-r+I at(+1+E,

where r=1-6and 8«E.

(ii) Now suppose that u(r, 9) satisfies V2u = 0 in r2 = a2 + y2 < 1, with

5r + yu = g(B)

on r = 1.

Take G = (1/2a)log Ix - 41 in (5.52) to show that

u(E) =

(uG

- G )

do,

o Or

r=11

and hence that, on r = 1, u satisfies the equation

U(1' a)

_ -! f g(o) log (I2sin (0

a)1)

j2wu(1,o)

(log (2sin (0

) )

d9.

5.14.

(i) Suppose that the function u(r, 0) is written as a double Fourier inverse,

-(kcos 9+k2

9dki

dk2.

u(r, 8) =

i_: f

k2)e

Show that u can satisfy the Helmholtz equation V2Uu + u = 0 if u =

2ir8(p - 1) f (0), where p2 = k1 + k4, tan ¢ = k2/k1 and f is arbi-

trary. Hence derive the Sommerfeld representation for the solution of

the Helmholtz equation in the form

-ircos(9-0)f(0)d,

u(r,O)

(ii) The Kontorovich-Lebedev inversion formula (5.106) on p. 193 suggests

that

u(r,O) = f JIK(r)e

iK9g(rc)dlc

is also a general solution of the Helmholtz equation. Verify that this

formula can be deduced from the Sommerfeld representation above by

showing that its formal Fourier transform with respect to 8,

f00 2a 00

.f eik9-r-e(9-0)f(0) d8d0

= f Jik(r)ekm

o 00

f(r) dm

= J,k(r)g(k),

say.

EXERCISES 227

5.15. Show that, if the real symmetric matrix A has real eigenvalues Aj and or-

thogonal eigenvectors x;, so that x; Ax; = Aix; xi, then, for any vector

y = E c,x the smallest eigenvalue AO satisfies

TAy,\O

yTy

Show that the eigenfunctions 0 and eigenvalues -A of the problem

V20 +,\o

= 0

in a region D,

with

8n + a¢ = 0 on 8D,

where 8/8n is the outward normal derivative, satisfy

,\L02

dx=J ,712 dx+a fq ds.

Deduce, as in the matrix case, that the smallest, or principal, eigenvalue

satisfies

A0 < (fD1vv12dx+ajDv2d8)/Lv2dx

for any smooth v.

5.16. Suppose that the eigenvalues An and normalised eigenfunctions ¢n(x) of the

Dirichlet problem for the Laplacian in a domain D are known: that is,

V2.0a

= Anon

in D with

¢n = 0 on OD.

Multiply by 0n and use Green's theorem to show that A. < 0. Show further

that the Green's function G(x, 4) for the modified Helmholtz equation, which

satisfies

V2G-k2G=6(x-C)

in D

with

G = 0 on 8D,

has the expansion

G(x,4) _

On(x)O. W

n_0

An -

Show that the expansion is also valid for the Helmholtz equation provided

that -k2 is not equal to any of the A. When -k2 = An for some n, show

how to construct a modified Green's function using the arguments of p. 173,

by solving

(V2 - An) G(x, F,) = 6(x

- F) + c4n(x)On(0

via an eigenfunction expansion with a suitable choice of c.