Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations
Подождите немного. Документ загружается.

88
INTRODUCTION TO SECOND-ORDER SCALAR EQUATIONS
data given on
t=lpandr
E
0t
to
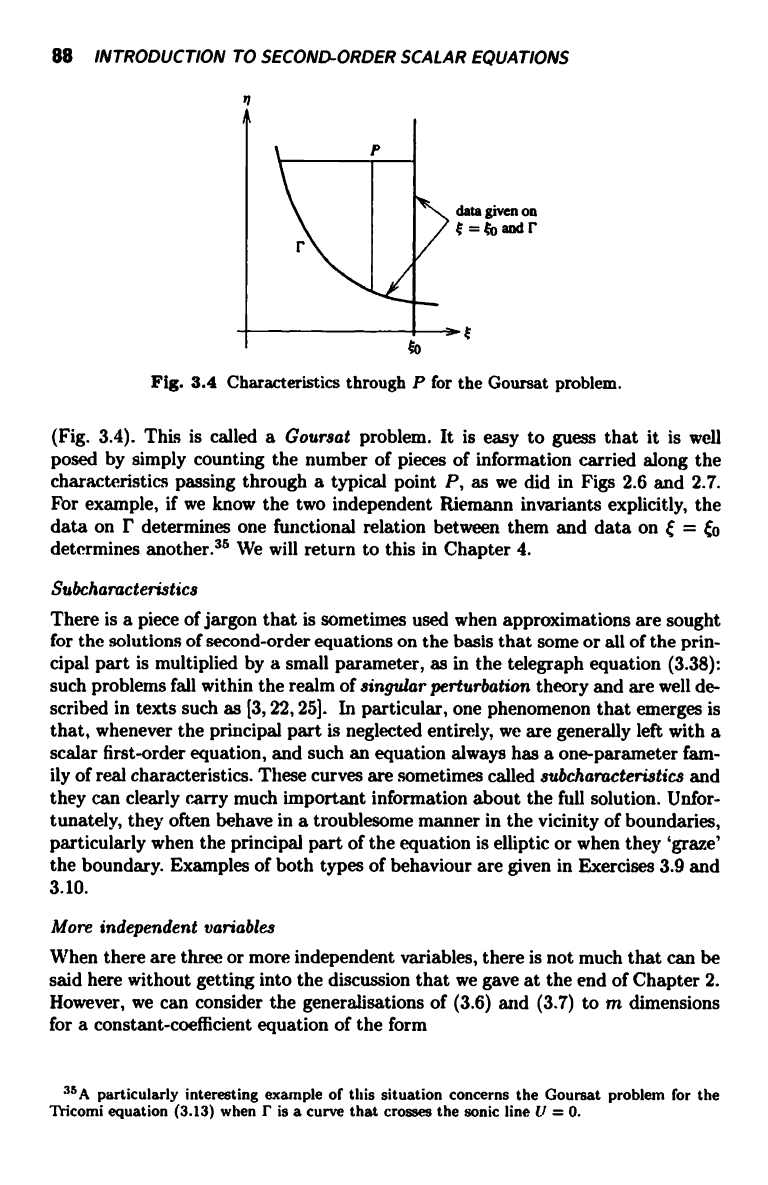
Fig. 3.4 Characteristics through P for the Goursat problem.
(Fig. 3.4). This is called a Goursat problem. It is easy to guess that it is well
posed by simply counting the number of pieces of information carried along the
characteristics passing through a typical point P, as we did in Figs 2.6 and 2.7.
For example, if we know the two independent Riemann invariants explicitly, the
data on r determines one functional relation between them and data on
= Co
determines another.35 We will return to this in Chapter 4.
Subcharacteristics
There is a piece of jargon that is sometimes used when approximations are sought
for the solutions of second-order equations on the basis that some or all of the prin-
cipal part is multiplied by a small parameter, as in the telegraph equation (3.38):
such problems fall within the realm of singular perturbation theory and are well de-
scribed in texts such as [3,22, 25]. In particular, one phenomenon that emerges is
that, whenever the principal part is neglected entirely, we are generally left with a
scalar first-order equation, and such an equation always has a one-parameter fam-
ily of real characteristics. These curves are sometimes called subcharacteristics and
they can clearly carry much important information about the full solution. Unfor-
tunately, they often behave in a troublesome manner in the vicinity of boundaries,
particularly when the principal part of the equation is elliptic or when they `graze'
the boundary. Examples of both types of behaviour are given in Exercises 3.9 and
3.10.
More independent variables
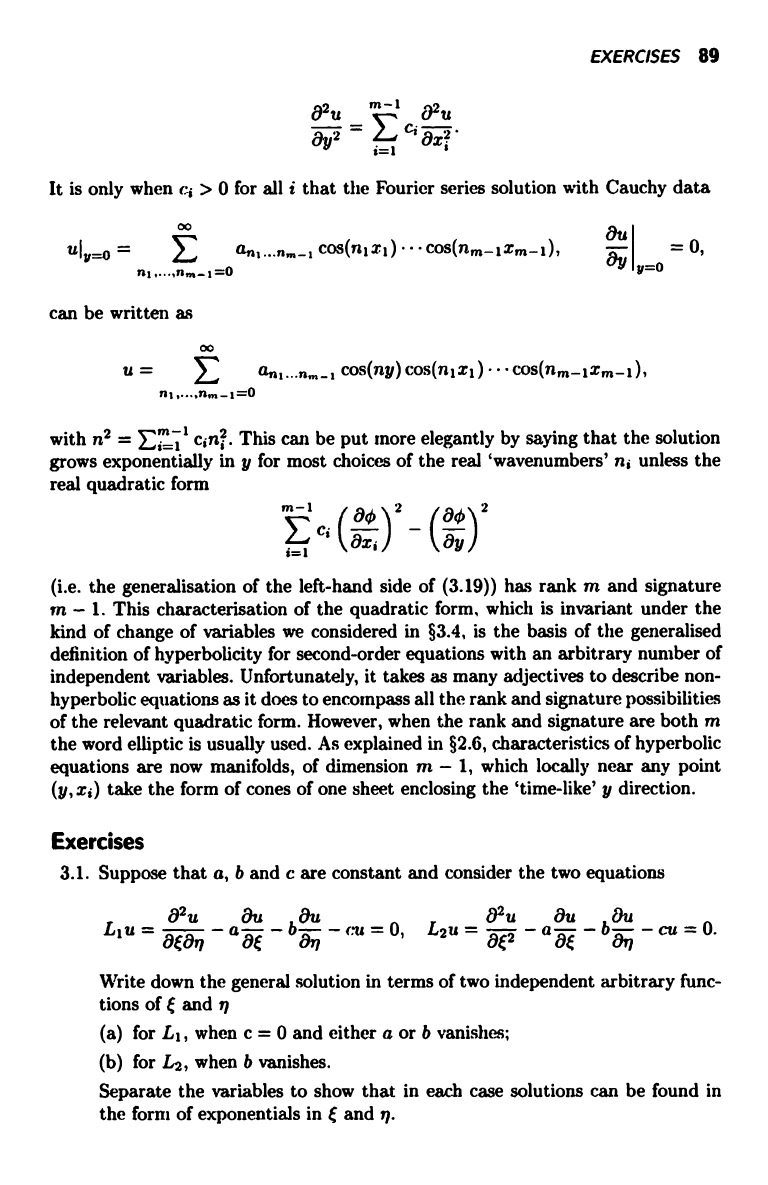
When there are three or more independent variables, there is not much that can be
said here without getting into the discussion that we gave at the end of Chapter 2.
However, we can consider the generalisations of (3.6) and (3.7) to m dimensions
for a constant-coefficient equation of the form
35A particularly interesting example of this situation concerns the Coursat problem for the
Tticomi equation (3.13) when r is a curve that crosses the sonic line U = 0.
EXERCISES 89
02U
m-'
C72u
aye
C572
00
u1Y=0 =
an,...n.,,-i cos(nlxl) ... COS(nm-lxm-l),
can be written as
8u
y-0
- 0,
00
u =
an,...nm_, cOs(ny) cOS(njx1) ... coS(nm-lxm-1),
with n2 = E' 1 cin; . This can be put more elegantly by saying that the solution
i__ I
grows exponentially in y for most choices of the real `wavenumbers' ni unless the
real quadratic form
uc8x)z-(9Y
(i.e. the generalisation of the left-hand side of (3.19)) has rank m and signature
m - 1. This characterisation of the quadratic form, which is invariant under the
kind of change of variables we considered in §3.4, is the basis of the generalised
definition of hyperbolicity for second-order equations with an arbitrary number of
independent variables. Unfortunately, it takes as many adjectives to describe non-
hyperbolic equations as it does to encompass all the rank and signature possibilities
of the relevant quadratic form. However, when the rank and signature are both m
the word elliptic is usually used. As explained in §2.6, characteristics of hyperbolic
equations are now manifolds, of dimension m - 1, which locally near any point
(y,xi) take the form of cones of one sheet enclosing the 'time-like' y direction.
Exercises
3.1. Suppose that a, b and c are constant and consider the two equations
L1u=J02U
--a8 -b--eu=0,
L2U=8 -a8,--b
cu=0.
Write down the general solution in terms of two independent arbitrary func-
tions off and rl
(a) for L1, when c = 0 and either a or b vanishes;
(b) for L2, when b vanishes.
Separate the variables to show that in each case solutions can be found in
the form of exponentials in C and rl.
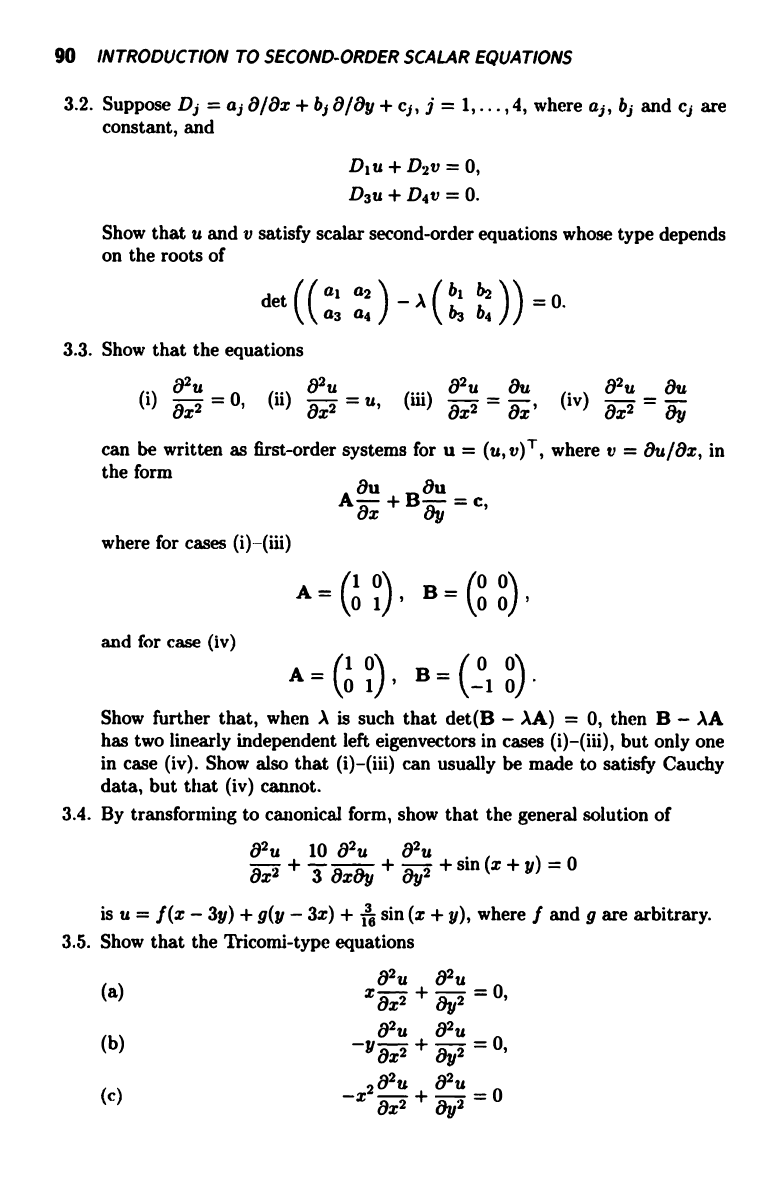
90
INTRODUCTION TO SECOND-ORDER SCALAR EQUATIONS
3.2. Suppose Dj = aj 8/8x + bj 8/8y + cj, j = 1,
... , 4, where aj, bj and ej are
constant, and
Dju + D2v = 0,
D3u+D4v=0.
Show that u and v satisfy scalar second-order equations whose type depends
on the roots of
det (I aa,
a2 b, b2
3 a4
b3 b4
) )
0.
3.3. Show that the equations
02u 82u
02u 8u 82u 8u
(i)
8x2
= 0,
(ii)
8x2
= u,
(ill)
8x2 = 8x'
(iv)
8xz = aU
can be written as first-order systems for u = (u,v)T, where v = 8u/8x, in
the form
A8x
+B5
=c,
8u
where for cases (i)-(iii)
A
- (0
0),
' B= (0 0),
and for case (iv)
A=(0 0), B=(O1 Q).
Show further that, when A is such that det(B - AA) = 0, then B - AA
has two linearly independent left eigenvectors in cases (i)-(iii), but only one
in case (iv). Show also that (i)-(iii) can usually be made to satisfy Cauchy
data, but that (iv) cannot.
3.4. By transforming to canonical form, show that the general solution of
02U
10
z z
8x22 + 3 8x8y + ayz
+ sin
(x + y) = 0
is u = f (x - 3y) + g(y - 3x) + 18 sin (x + y), where f and g are arbitrary.
3.5. Show that the Ticomi-type equations
z z
( ) + 0a
x
8x2 8y2 '
02u 02u
(b)
-y8x2 + 8y2 = 0,
( )
- z
0
c
x
8xz
+
W
EXERCISES 91
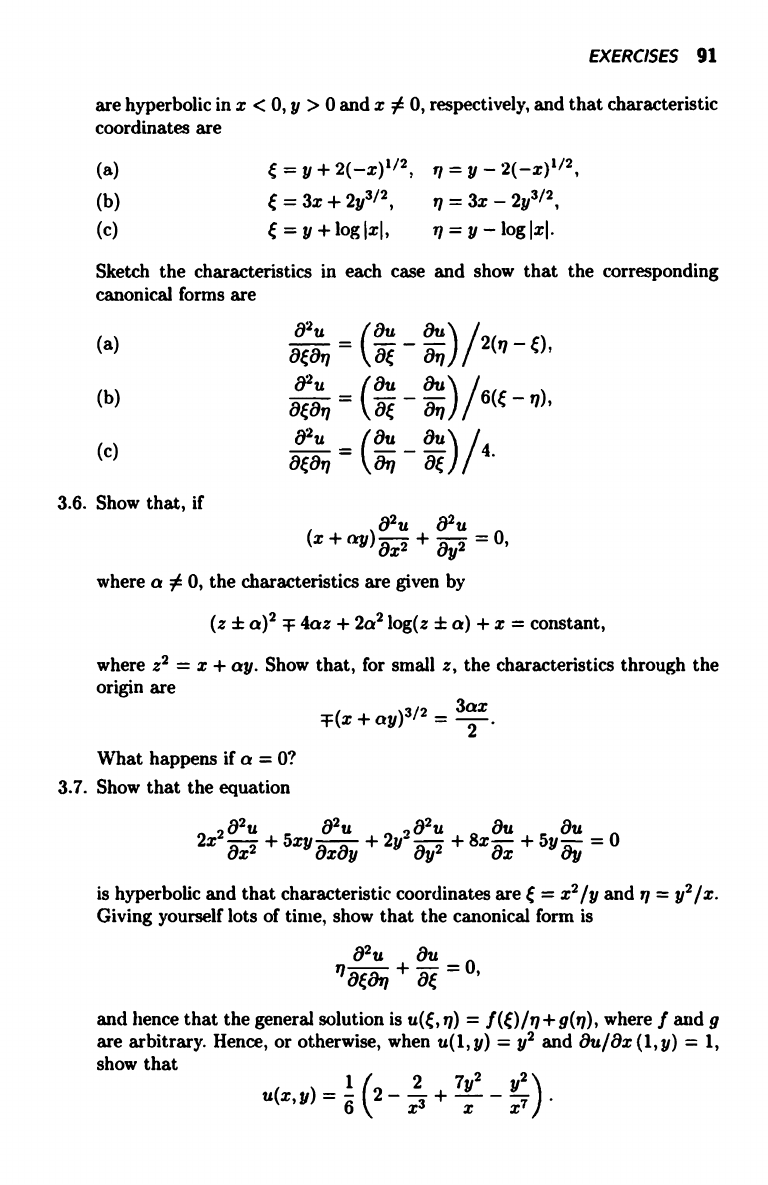
are hyperbolic in x < 0, y > 0 and x # 0, respectively, and that characteristic
coordinates are
(a)
= y +
2(-x)12,
*I = y -
2(-x)112,
(b)
=3x+2y312,
n=3x-2y312,
(c)
=y+loglxl, 7i=y-loglxl.
Sketch the characteristics in each case and show that the corresponding
canonical forms are
(a)
(b)
(c)
3.6. Show that, if
i
(L-
) /2(q -
/6(t- n),
02U
OU au
=
-
- -)/4.
8017 0% at
82u 82u
(x + ay) axe + aye = 0,
where a i4 0, the characteristics are given by
(z ± a)2 4az + 2a2 log(z ± a) + x = constant,
where z2 = x + ay. Show that, for small z, the characteristics through the
origin are
(x + ay)3/2 =
3ax
2
What happens if a = 0?
3.7. Show that the equation
u
2x2
axe
+ 5xy
8x.y +
2y2 8-2 + 8x 8- + 5y
= 0
is hyperbolic and that characteristic coordinates are = x2/y and q = y2/x.
Giving yourself lots of time, show that the canonical form is
02U
rl
8t
= 0,
and hence that the general solution is u(t, q) = f (t)/q+g(iI), where f and g
are arbitrary. Hence, or otherwise, when u(1, y) = y2 and 8u/8x (1, y) = 1,
show that
1 (2
2 +
7y2
y2
U(-T'
TT
;77
92 INTRODUCTION TO SECOND-ORDER SCALAR EQUATIONS
3.8. Show that, when u(x,0) = uo(x) and Ou/Ot (x, 0) = vo(x), the d'Alembert
representation (3.31) gives
x+aot
u(x, t) = 2 (uo(x - aot) + uo(x + aot)) +
-
f_
vo(s) d8.
t Opt
3.9. Show that constants a and b can be chosen so that u = ay +
bye-y''
satisfies
/
02U
49U
+-p+-=0 for-1<x<1,
e1 -
OX2 9y2
OX
with u(1, y) = y and u(-1, y) = -y. Check that, as a -4 0+, u becomes
constant on the subcharacteristics y = constant and satisfies one boundary
condition, but not the other. What happens when a -+ 0-?
3.10. Suppose that u(x, y, t) satisfies the elliptic equation
O2 +f2
02U
0 for0<y<1,
with
ay (x, 0, t) = 0, a (x, 1, t) + e2 5i2 (x, 1, t) = 0.
Show that a formal power series in which
u = uo(x,y,t) + e2u1(x,y,t) + ...
satisfies the equation and boundary condition up to terms of O(e2) if
uo = uo(x,t),
where uo satisfies the hyperbolic equation
0-2U0
O2uo
= 0
ax2 &2
This example, which is a simple model for the tides, shows that the solution
of an elliptic equation can sometimes be consistently approximated by that
of a hyperbolic equation.
4
Hyperbolic equations
4.1 Introduction
Hyperbolic equations are the easiest scalar second-order equations to classify from
the point of view of the Cauchy problem. They occur commonly in practical appli-
cations, as is evident from studying the models of Chapter 2. Take, for example,
fluid dynamics. We have already remarked in Chapter 3 that a large class of
steady two-dimensional supersonic gas flows can be modelled by the hyperbolic
equation (3.11), (1- U2/ao)820/8x2 + 820/8y2 = 0. However, the requisite cross-
differentiation is not possible for evolutionary models such as the shallow water
model (2.1) and (2.2), or the unsteady gas dynamics model (2.3) and (2.4), except
in the frequently occurring acoustic limit in which the fluid is nearly in a state
of rest or uniform motion. Then, a linearisation procedure can be carried out as
on p. 65. For example, when we assume that u and h - ho in (2.1) and (2.2), or
u, p - po and p - po in (2.3) and (2.4), are small enough for their squares to be
negligible, we obtain the hyperbolic one-dimensional wave equation
820 2820
8t2 = ao 8x2
(4.1)
where as = gho or ao = rypa/po, respectively. Here ¢ is any one of the variables
u or h - ho in (2.1) and (2.2), or u, p - po or p - po in (2.3) and (2.4), the
remaining variables being related to 0 by simple linear transformations. Equally,
the regenerator and fluidised bed models (2.17)-(2.20) can be cross-differentiated
to give a constant-coefficient equation of the form
(
a
+
aTx
+
b) I i
t +
cTx
+ d l u = eu, (4.2)
which is clearly hyperbolic and is a version of the telegraph equation mentioned
in §3.4.3; to see this set u = e°:+dev for suitable n and Q.
Multi-space-dimensional versions of such linear scalar second-order equations
are even more relevant for practical problems. Indeed, much of linear acoustics is
governed by
ae
= aS V2ut
93

94
HYPERBOLIC EQUATIONS
in two or three space dimensions,36 and we will discuss how the solutions of such
hyperbolic equations depend on dimensionality in §4.6. Still more interesting is
the appearance of vector versions of (4.3), which should really be classified using
the methods of Chapter 2. However, a generalisation of the discussion of §3.4 will
give us enough of a basis to be able to discuss systems such as Maxwell's equations
and the equations of linear elasticity in §4.7.
Our first task in this chapter is to see how the theory for linear scalar second-
order equations in two independent variables can be put to good use. Since we know
that the Cauchy problem for such equations is well posed, we can immediately
set about finding representations of solutions, confident that such representations
make sense and depend continuously on the data. This is the first time in the book
that a general strategy for representing solutions is presented, and it is not the last,
so a careful preamble about the possible procedures and methodology is given in
§4.2. Moreover, there is one analytical technique, that of eigenfunction expansions,
which we review in §4.4 because it is of such importance in this and subsequent
chapters. Most of the rest of this chapter is devoted to the explicit solution of
linear equations, and we leave those few representations that are available for the
solution of nonlinear hyperbolic problems to the brief §4.8.
4.2 Linear equations: the solution to the Cauchy problem
We begin by considering linear equations that have been transformed to charac-
teristic variables (x, y), so that we have to solve
82u
8u
8u
Gu=8x8y+pex+qay+ru=f,
where p, q, r and f are functions of x and y, but not of u or its derivatives. We
take u and Ou/8n (or, equivalently up to a constant, 8u/8x and 8u/8y) to be
prescribed on some open curve r that is nowhere parallel to the characteristics,
which are the x and y axes. For definiteness we only look for the solution on one
side of this curve, say y increasing, as in Fig. 4.1.
The prescription for the representation for the solution of this general Cauchy
problem can be presented at two different levels and we leave it to the reader to
decide which route to take; they converge at the end of §4.2.2.
4.2.1 An ad hoc approach to Riemann functions
Motivated by §§3.3 and 3.4.1, we ask whether the d'Alembert formula (3.31) could
not be generalised to apply to (4.4). Now the key step in deriving (3.31) was
direct integration with respect to first x and then y, which is one of the many
ways of evaluating a double integral over the region D of Fig. 4.1. In the light of
36Here and henceforth we will use the applied mathematician's notation
_ 82 82
82
V2
8s2 + 8y2 + 8z2'
where z, y, z denote spatial coordinates.
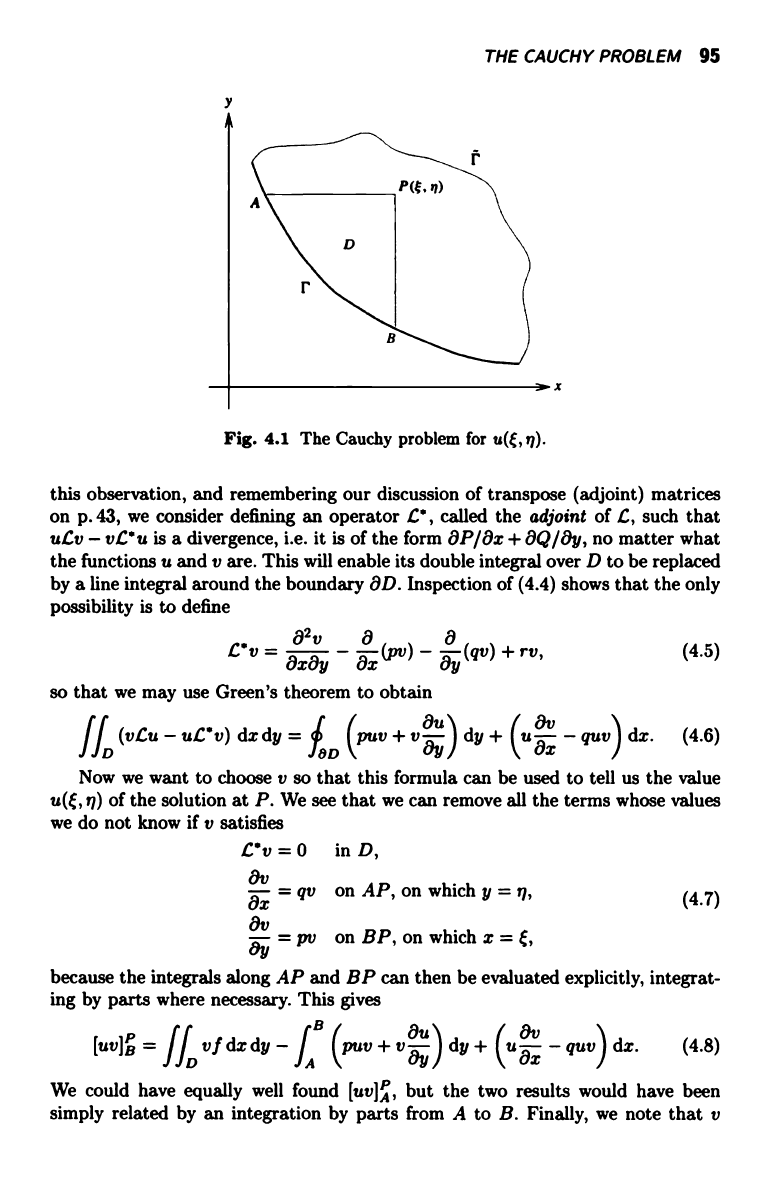
THE CAUCHY PROBLEM 95
y
x
Fig. 4.1 The Cauchy problem for u(C, n).
this observation, and remembering our discussion of transpose (adjoint) matrices
on p. 43, we consider defining an operator C', called the adjoint of C, such that
urv - vC'u is a divergence, i.e. it is of the form OP/8x + 8Q/8y, no matter what
the functions u and v are. This will enable its double integral over D to be replaced
by a line integral around the boundary W. Inspection of (4.4) shows that the only
possibility is to define
z
Cv =
8 v
-
8
(pv) -
8
(qv) + rv, (4.5)
8x8y ax ay
so that we may use Green's theorem to obtain
\ \
I'D (vCu - uVv) dx dy = illD
+ v
2u-)
dy +
(us!
- quv I dx. (4.6)
Now we want to choose v so that this formula can be used to tell us the value
u(t, p) of the solution at P. We see that we can remove all the terms whose values
we do not know if v satisfies
Vv = 0
in D,
8v
= qv
on AP, on which y = q,
8x
(4.7)
8v
= pv on BP, on which x =
8y
because the integrals along AP and BP can then be evaluated explicitly, integrat-
ing by parts where necessary. This gives
1
+
dy +
(u 8-
quv 1 dx. (4.8)
[uv]
= ffD' dx dy -
a
We could have equally well found [uv]A, but the two results would have been
simply related by an integration by parts from A to B. Finally, we note that v

96
HYPERBOLIC EQUATIONS
is undefined to within a multiplicative constant so that, if v exists, it can be set
equal to unity at P.
The function v is called the Ricmann function for our problem and, if we can
convince ourselves that it exists, (4.8) gives us our desired representation of the
solution of the Cauchy problem. In fact, existence is no problem when (4.5) can
be formally converted to a linear Volterra integral equation; this happens, for
example, if p = q = 0, when
y
v(x, y) = 1 - f
f:r
r(x', y')v(x', y') dx' dy';
n
th
e convergence of a sequence satisfying
1 - f f
to a unique v(x, y)
is easy to establish, as in the proof of Picard's theorem for ordinary differential
equations.
Even in the absence of any explicit formula for the Riemann function v, our
basic result (4.8) contains a wealth of valuable information about hyperbolic equa-
tions, to which we will return at the end of this section. However, we first present
an alternative, more complicated, but more systematic, story of the Riemann func-
tion which may be skipped by those anxious to get to explicit situations as quickly
as possible. We nonetheless urge those who wish to see a coherence between this
chapter and its successors to read on.
4.2.2 The rationale for Riemann functions
We begin this rather lengthy tale by recalling the Fredholm Alternative that we
encountered in §2.2 for the matrix equation
Ax = b,
(4.9)
where x and b are column vectors, and A is an n x n matrix. If the solution exists,
we can simply write it as x = A-'b. However, we can write this in an especially
convenient way if we observe that, if yk is a vector such that37
Yk A = ek ,
i.e.
ATYk = ek,
(4.10)
where ek is the kth standard basis vector, then the kth component of x, which is
equal to ek x, can be written
(Yk A)x = YT(AX) = Ykb-
Thus we have found the kth component of x simply by taking the `inner product'
of (4.9) with Yk and of (4.10) with x and subtracting; all we needed was the
identity
XTATYk = Yk Ax.
This offers us a layout that can be used repeatedly for defining the inverse of all
kinds of linear differential operators. For example, suppose we consider the initial
value problem for a second-order linear ordinary differential equation in the form
370f course, yk is just the kth row of A.
THE CAUCHY PROBLEM 97
2
Cu = f(x), C =
i +
P(x)aj + q(x)
for x > 0, (4.11)
with u(0) = du/dx (0) = 0 without loss of generality. We exploit the argument
above by identifying C with A it with x and f with b. We now try to define C,
b, R and an inner product ( , ) so that we can draw the analogies
Ax = b
<-+
Cu(x) = f (x),
u(0) = dZ (0) = 0;
ATYk = ek
++ b(x - l;);
Yk Ax
=
xTATYk
H
(R(x,C), Cu(x)) = (u(x), C'R(x,C));
yk b = e,k x H
(R(x, C), f (x)) = (b(x - l), u(x)).
The last line in the left-hand column gives x, component by component, and the
last line in the right-hand column is supposed to give u(t) at all possible values of
C. These analogies will work if we proceed as follows.
1. We define (u(x), v(x)) = f
x
u(x)v(z) dx for some suitable X > . The motiva-
tion for this is as follows: if we divide the range of integration into N subintervals
of width h, and approximate u(x) and v(z) b y step functions on each subin-
terval, the functions are represented by vectors of values u = (ui , ... , UN) and
v = (vi, ... , vN). The integral of uv is approximated by r uivih = u v h
which, under suitable conditions, becomes f uv dx as N -4 oc.
2. We define
C R = d R - dx (pR) + qR,
which makes uC R - RCu into an exact differential, and in addition we specify
that R(X) = dR/dx (X) = 0.
3. We define b(x - t) to be the so-called delta function, i.e. the limit of a sequence
of well-behaved functions that tend to zero except for very small values of x -1;,
but whose integral over any interval containing x =
is unity. The sequence
could, for example, be defined by
1 _2
b(x) = lim -e =
eto F
r
(4.12)
By taking limits of integrals of members of this approximating sequence, the
vital result (b(x - {), u(x)) = u(t) in the last line of the analogy can be justified
as long as X >
and, strikingly, it can be shown to follow from limits of any
reasonable sequence of approximating sequences like (4.12).
This last idea is one of the principal motivations for the axiomatic definition of
distributions or generalised functions [37, 42]. In this approach all the `epsilonology'
is swept away by defining the delta function and its relatives as linear functionals
that map a class of suitably smooth test functions to the real numbers 38 We define
The whole philosophy is closely related to that of the theory of weak solutions of hyperbolic
equations in the presence of shock waves, as discussed in §1.7.
