Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations

Подождите немного. Документ загружается.

FIRST-ORDER QUASILINEAR SYSTEMS

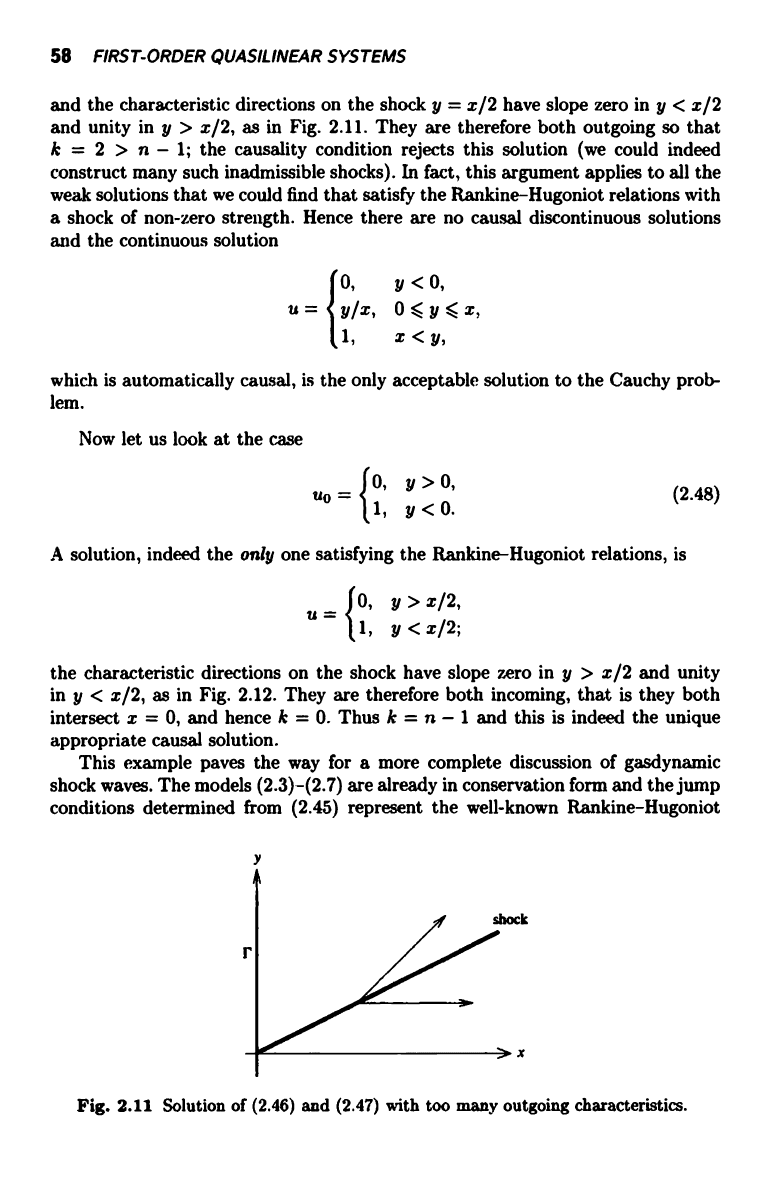
and the characteristic directions on the shock y = x/2 have slope zero in y < x/2

and unity in y > x/2, as in Fig. 2.11. They are therefore both outgoing so that

k = 2 > n - 1; the causality condition rejects this solution (we could indeed

construct many such inadmissible shocks). In fact, this argument applies to all the

weak solutions that we could find that satisfy the Rankine-Hugoniot relations with

a shock of non-zero strength. Hence there are no causal discontinuous solutions

and the continuous solution

0, y < 0,

y/x,

0'< y<x,

x < y,

which is automatically causal, is the only acceptable solution to the Cauchy prob-

lem.

Now let us look at the case

Un =

0, y > 0,

I, y <0

(2.48)

A solution, indeed the only one satisfying the Rankine-Hugoniot relations, is

0, y > x/2,

1, /2V < x ;

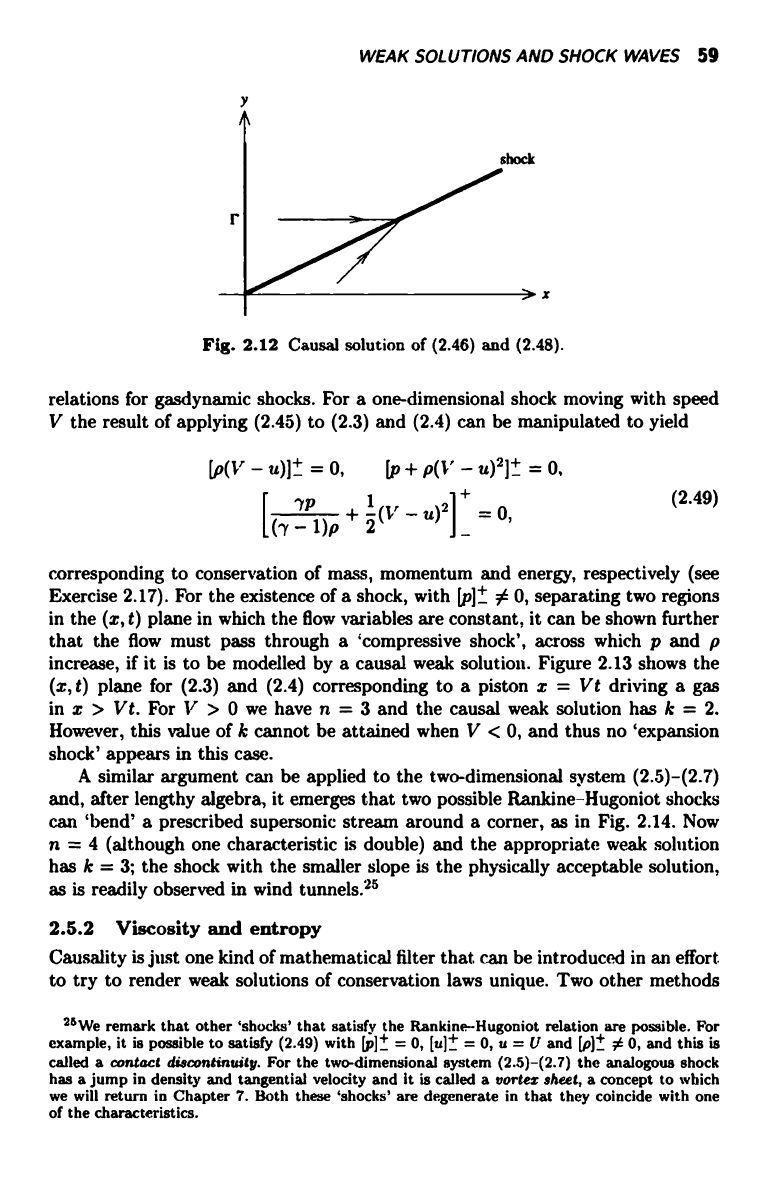
the characteristic directions on the shock have slope zero in y > x/2 and unity

in y < x/2, as in Fig. 2.12. They are therefore both incoming, that is they both

intersect x = 0, and hence k = 0. Thus k = n - 1 and this is indeed the unique

appropriate causal solution.

This example paves the way for a more complete discussion of gasdynamic

shock waves. The models (2.3)-(2.7) are already in conservation form and the jump

conditions determined from (2.45) represent the well-known Rankine-Hugoniot

Fig. 2.11 Solution of (2.46) and (2.47) with too many outgoing characteristics.

WEAK SOLUTIONS AND SHOCK WAVES 59

-> r

Fig. 2.12 Causal solution of (2.46) and (2.48).

relations for gasdynamic shocks. For a one-dimensional shock moving with speed

V the result of applying (2.45) to (2.3) and (2.4) can be manipulated to yield

[p(V - u)]± = 0,

[p + p(V - U)2]1 = 0,

[_-p

-1)p +

2(`'

u)2J o,

(2.49)

corresponding to conservation of mass, momentum and energy, respectively (see

Exercise 2.17). For the existence of a shock, with [p]± 36 0, separating two regions

in the (z, t) plane in which the flow variables are constant, it can be shown further

that the flow must pass through a `compressive shock', across which p and p

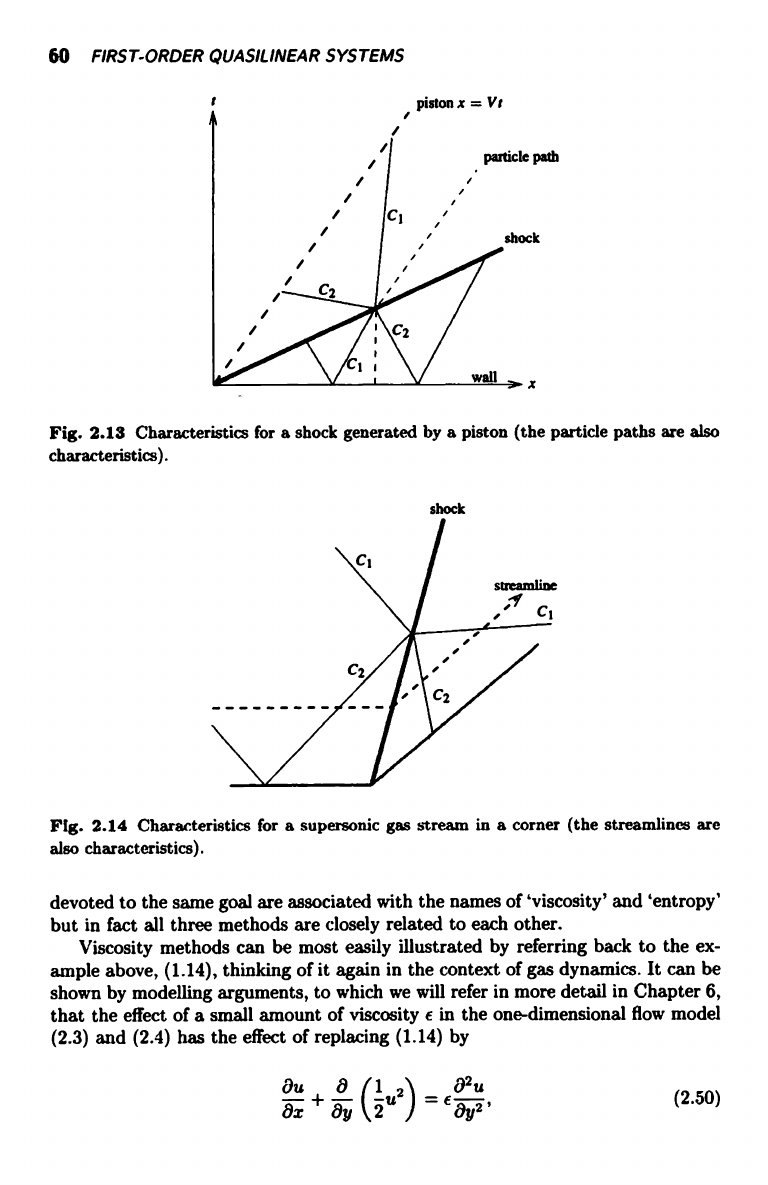
increase, if it is to be modelled by a causal weak solution. Figure 2.13 shows the

(z, t) plane for (2.3) and (2.4) corresponding to a piston z = Vt driving a gas

in x > Vt. For V > 0 we have n = 3 and the causal weak solution has k = 2.

However, this value of k cannot be attained when V < 0, and thus no 'expansion

shock' appears in this case.

A similar argument can be applied to the two-dimensional system (2.5)-(2.7)

and, after lengthy algebra, it emerges that two possible Rankine-Hugoniot shocks

can 'bend' a prescribed supersonic stream around a corner, as in Fig. 2.14. Now

n = 4 (although one characteristic is double) and the appropriate weak solution

has k = 3; the shock with the smaller slope is the physically acceptable solution,

as is readily observed in wind tunnels.25

2.5.2 Viscosity and entropy

Causality is just one kind of mathematical filter that can be introduced in an effort

to try to render weak solutions of conservation laws unique. Two other methods

25We remark that other `shocks' that satisfy the Rankine-Hugoniot relation are possible. For

example, it is possible to satisfy (2.49) with [p]± = 0, [u)± = 0, u = U and [p)± # 0, and this is

called a contact discontinuity. For the two-dimensional system (2.5)-(2.7) the analogous shock

has a jump in density and tangential velocity and it is called a vortex sheet, a concept to which

we will return in Chapter 7. Both these `shocks' are degenerate in that they coincide with one

of the characteristics.

FIRST-ORDER QUASILINEAR SYSTEMS

Fig. 2.13 Characteristics for a shock generated by a piston (the particle paths are also

characteristics).

Fig. 2.14 Characteristics for a supersonic gas stream in a corner (the streamlines are

also characteristics).

devoted to the same goal are associated with the names of `viscosity' and `entropy'

but in fact all three methods are closely related to each other.

Viscosity methods can be most easily illustrated by referring back to the ex-

ample above, (1.14), thinking of it again in the context of gas dynamics. It can be

shown by modelling arguments, to which we will refer in more detail in Chapter 6,

that the effect of a small amount of viscosity e in the one-dimensional flow model

(2.3) and (2.4) has the effect of replacing (1.14) by

8x + 8y

C2u2J - E8y2'

(2.50)

WEAK SOLUTIONS AND SHOCK WAVES 61

U-00

-4

U00

y - V.r

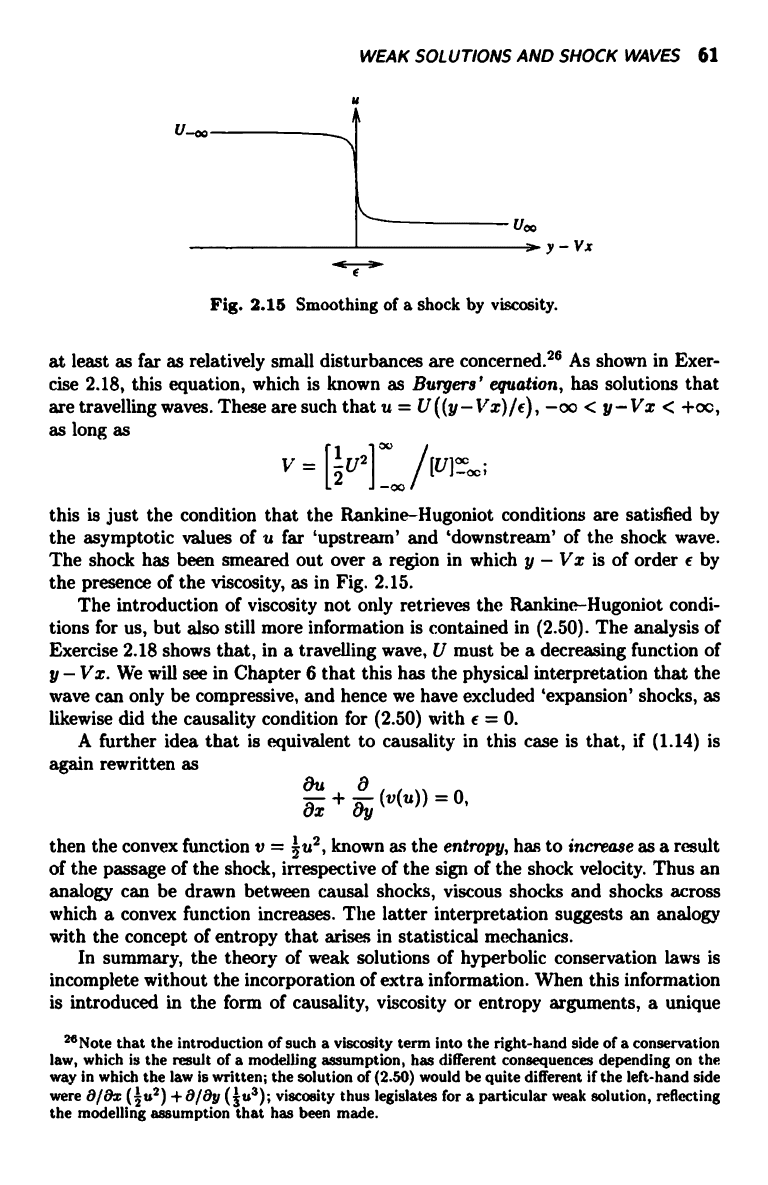
Fig. 2.15 Smoothing of a shock by viscosity.

at least as far as relatively small disturbances are concerned.26 As shown in Exer-

cise 2.18, this equation, which is known as Bangers' equation, has solutions that

are travelling waves. These are such that u = U((y-Vx)/e), -co < y-Vx < +oo,

as long as

V =

{U2]:/EU];

this is just the condition that the Rankine-Hugoniot conditions are satisfied by

the asymptotic values of u far `upstream' and `downstream' of the shock wave.

The shock has been smeared out over a region in which y - V x is of order a by

the presence of the viscosity, as in Fig. 2.15.

The introduction of viscosity not only retrieves the Rankine-Hugoniot condi-

tions for us, but also still more information is contained in (2.50). The analysis of

Exercise 2.18 shows that, in a travelling wave, U must be a decreasing function of

y - Vx. We will see in Chapter 6 that this has the physical interpretation that the

wave can only be compressive, and hence we have excluded `expansion' shocks, as

likewise did the causality condition for (2.50) with e = 0.

A further idea that is equivalent to causality in this case is that, if (1.14) is

again rewritten as

8 + F (v(u)) = 0,

then the convex function v = Ju2, known as the entropy, has to increase as a result

of the passage of the shock, irrespective of the sign of the shock velocity. Thus an

analogy can be drawn between causal shocks, viscous shocks and shocks across

which a convex function increases. The latter interpretation suggests an analogy

with the concept of entropy that arises in statistical mechanics.

In summary, the theory of weak solutions of hyperbolic conservation laws is

incomplete without the incorporation of extra information. When this information

is introduced in the form of causality, viscosity or entropy arguments, a unique

26Note that the introduction of such a viscosity term into the right-hand side of a conservation

law, which is the result of a modelling assumption, has different consequences depending on the

way in which the law is written; the solution of (2.50) would be quite different if the left-hand side

were 8/8x (Zu2) + 8/ay (.u3); viscosity thus legislates for a particular weak solution, reflecting

the modelling assumption that has been made.

FIRST-ORDER QUASILINEAR SYSTEMS

weak solution emerges in which the Rankine-Hugoniot conditions are satisfied

across any shock waves that are present. The concept of viscosity is a stronger

one than those of causality or entropy because it even allows us to predict the

Rankine-Hugoniot conditions as well as selecting a unique limiting solution as the

viscosity tends to zero.

2.5.3 Other discontinuities

A much more general discussion of discontinuous solutions of partial differential

equations will be given in Chapter 7. That chapter is rather lengthy, mostly because

of the delicate nature of the subject, and there could be no better example of this

than the modelling of gasdynamic shock waves in the presence of chemical reactions

which are localised near the discontinuity, which is then often called a detonation.

In this case, the right-hand side of (2.4) is replaced by a localised source term,

which means that the Ranldne-Hugoniot relation for the energy changes from

(2.49) to

+1(V-u)2l+=E,

('Y-1)P 2 J-

where E is the prescribed constant energy per unit mass released during the reac-

tion. When E is zero, we can, as mentioned above, derive non-unique weak solu-

tions in which [p)+-, [p]± and [uJ+ satisfy the Rankine-Hugoniot relations (2.49).

It can be shown that we can rearrange these relations into

ry + 1 - (ry - 1)/p

(7 +

1)/3 - (7 _ 1)'

where p and p are the ratios of the downstream to the upstream pressures and

densities, respectively (upstream referring to gas that has not yet traversed the

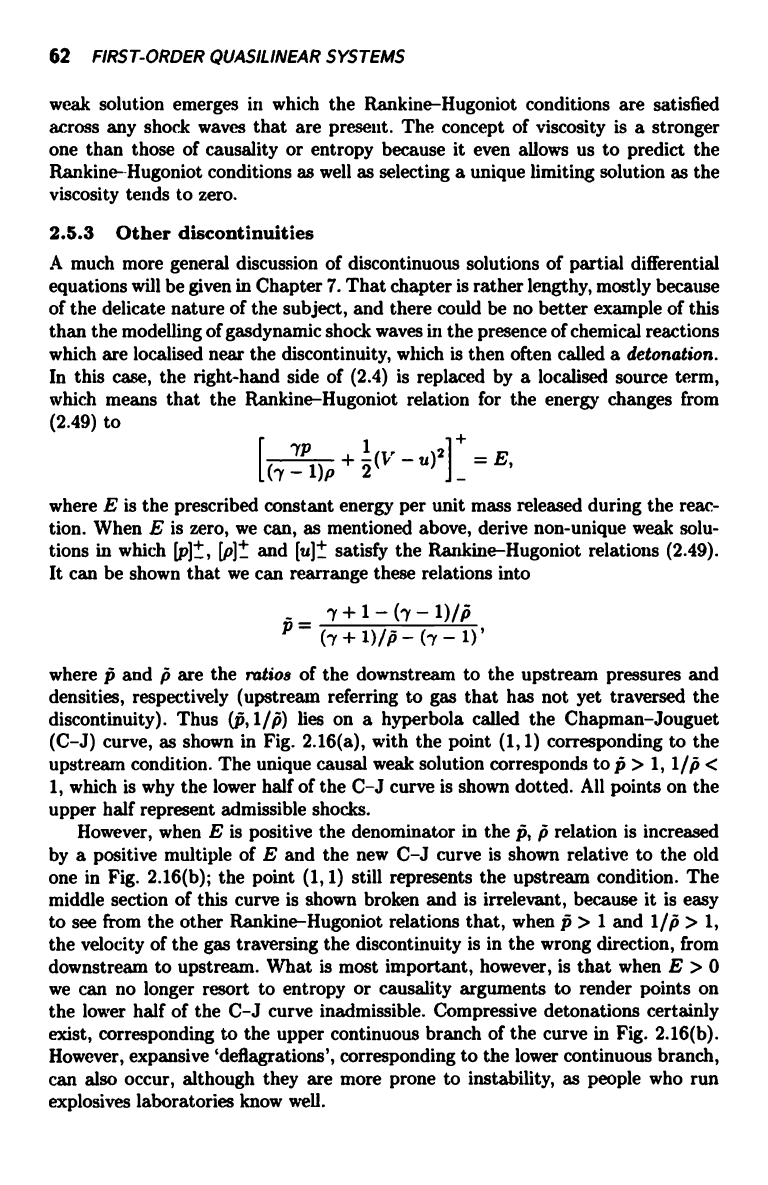
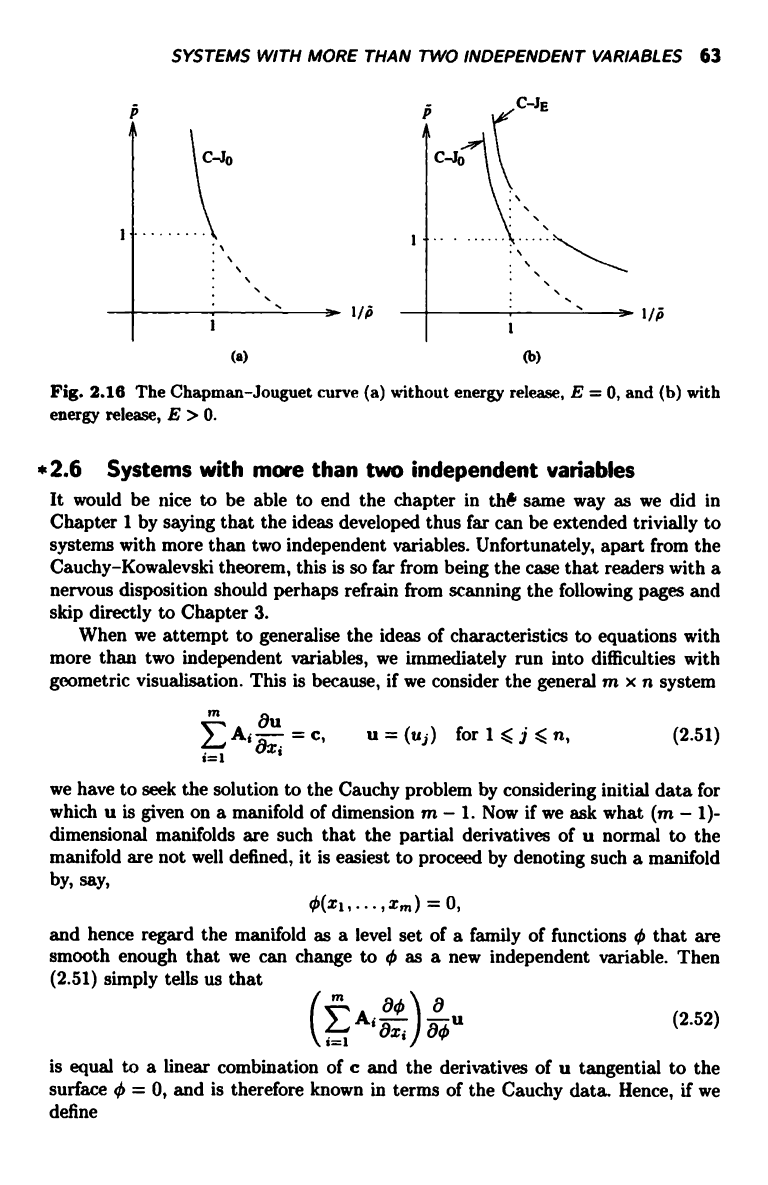
discontinuity). Thus (p, 1/p) lies on a hyperbola called the Chapman-Jouguet

(C-J) curve, as shown in Fig. 2.16(a), with the point (1,1) corresponding to the

upstream condition. The unique causal weak solution corresponds to p > 1, 1/0 <

1, which is why the lower half of the C-J curve is shown dotted. All points on the

upper half represent admissible shocks.

However, when E is positive the denominator in the p, p relation is increased

by a positive multiple of E and the new C-J curve is shown relative to the old

one in Fig. 2.16(b); the point (1, 1) still represents the upstream condition. The

middle section of this curve is shown broken and is irrelevant, because it is easy

to see from the other Rankine-Hugoniot relations that, when p > 1 and 1/p > 1,

the velocity of the gas traversing the discontinuity is in the wrong direction, from

downstream to upstream. What is most important, however, is that when E > 0

we can no longer resort to entropy or causality arguments to render points on

the lower half of the C-J curve inadmissible. Compressive detonations certainly

exist, corresponding to the upper continuous branch of the curve in Fig. 2.16(b).

However, expansive `deflagration', corresponding to the lower continuous branch,

can also occur, although they are more prone to instability, as people who run

explosives laboratories know well.

SYSTEMS WITH MORE THAN TWO INDEPENDENT VARIABLES 63

C-lo

I/P

1/0

Fig. 2.16 The Chapman-Jouguet curve (a) without energy release, E = 0, and (b) with

energy release, E > 0.

*2.6 Systems with more than two independent variables

It would be nice to be able to end the chapter in the' same way as we did in

Chapter 1 by saying that the ideas developed thus far can be extended trivially to

systems with more than two independent variables. Unfortunately, apart from the

Cauchy-Kowalevski theorem, this is so far from being the case that readers with a

nervous disposition should perhaps refrain from scanning the following pages and

skip directly to Chapter 3.

When we attempt to generalise the ideas of characteristics to equations with

more than two independent variables, we immediately run into difficulties with

geometric visualisation. This is because, if we consider the general m x n system

A,Lu =c, u=(u,) for1<j<, n, (2.51)

we have to seek the solution to the Cauchy problem by considering initial data for

which u is given on a manifold of dimension m - 1. Now if we ask what (m - 1)-

dimensional manifolds are such that the partial derivatives of u normal to the

manifold are not well defined, it is easiest to proceed by denoting such a manifold

by, say,

0(x1,... ,x,,,) = 01

and hence regard the manifold as a level set of a family of functions ¢ that are

smooth enough that we can change to 0 as a new independent variable. Then

(2.51) simply tells us that

(m8)O

u (2.52)

8x; Fo

is equal to a linear combination of c and the derivatives of u tangential to the

surface

= 0, and is therefore known in terms of the Cauchy data. Hence, if we

define

FIRST-ORDER QUASILINEAR SYSTEMS

= det

Aj-q0--)

Q axt ..., axm

ox;

we are led to define a characteristic surface 4' = 0 as one on which

0.0

ail

axt

, ... , axm) = 0.

(2.53)

This clearly reduces to the first two of (1.7) and (2.26) when m = 2 and n = 1,

2, respectively. Equation (2.53) also reduces to (1.32) in the scalar case, and our

remarks about the interpretation of (1.32) as a partial differential equation apply

equally to (2.53).

In principle, it is easy to generalise the Cauchy-Kowalevski theorem to prove

the existence and uniqueness of solutions to the Cauchy problem when the data

is given on a non-characteristic manifold, given the usual crucial requirements of

locality and analyticity.

On the other hand, when m > 2, not only do we have the irksome task of

solving a partial differential equation for 0, but also it is more difficult to visualise

what (2.53) means for the surface 0 = 0. However, bearing in mind that the

normal to the surface is always (a¢/ax;) = (£;), say, we can see that (2.53), being

a homogeneous expression of degree n in these quantities, states that at any point

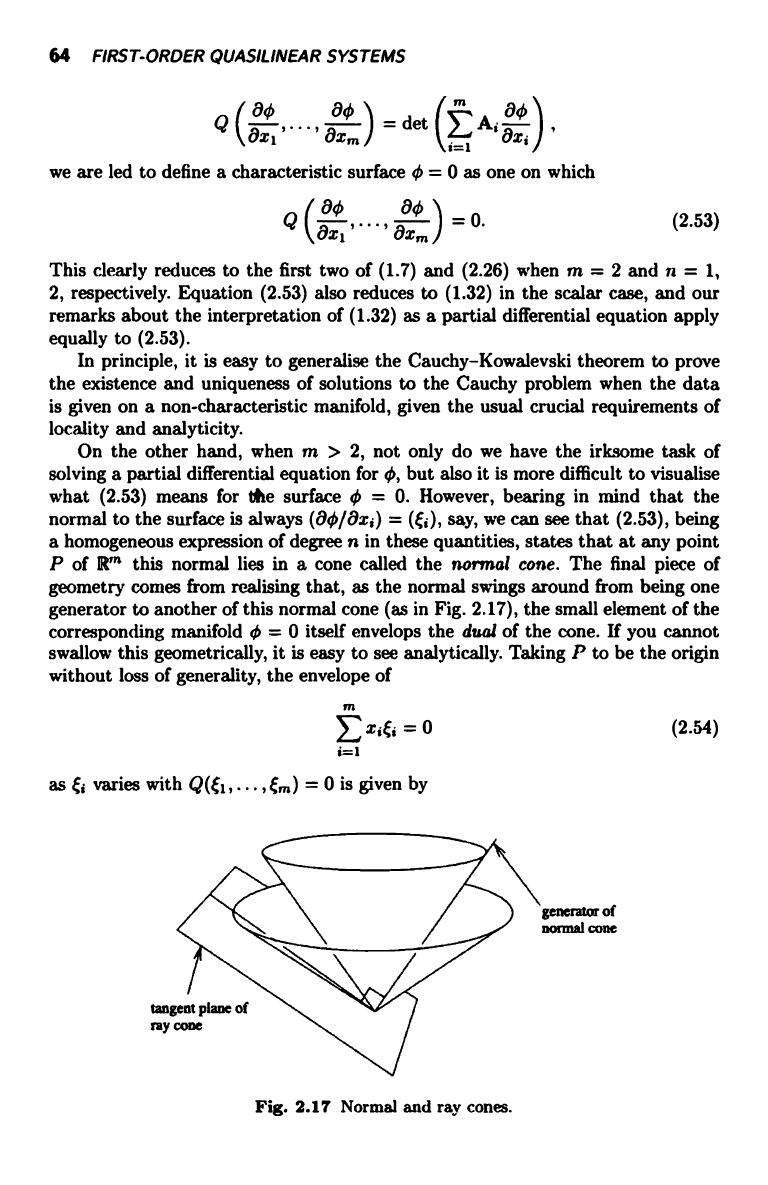
P of R1 this normal lies in a cone called the normal cone. The final piece of

geometry comes from realising that, as the normal swings around from being one

generator to another of this normal cone (as in Fig. 2.17), the small element of the

corresponding manifold 0 = 0 itself envelops the dual of the cone. If you cannot

swallow this geometrically, it is easy to see analytically. Taking P to be the origin

without loss of generality, the envelope of

;.t

as {; varies with Q(£1i ... , m) = 0 is given by

generator of

normal cone

(2.54)

Fig. 2.17 Normal and ray cones.

SYSTEMS WITH MORE THAN TWO INDEPENDENT VARIABLES 65

(xi&i)

- p = 0

for i = 1, ... , m, (2.55)

i=1

where p is a Lagrange multiplier. Hence we obtain

=,ULQI

Q(61...It.,,) = 0

(2.56)

as the parametric representation of the envelope. It is clearly a homogeneous ex-

pression of degree n, this time in our base coordinates xi, and thus also represents

a cone, called the nzy cone. To visualise the characteristic surface, all we have to

think of is the ray cones dotted around in Rm; a surface is characteristic if it is

tangent to each ray cone at every point. For scalar equations, in which n = 1, the

ray cones all collapse into lines, as in Fig. 1.2.

Once this picture has emerged, the concept of hyperbolicity takes on an entirely

new flavour, because we no longer have a slope A whose reality or otherwise could

be used as a criterion. Instead, we are motivated to consider the possible geometry

of the normal and ray cones and ask how degenerate they are. For example, if

Q(ti,... ,

is positive definite, the null vector is the only generator of the normal

cone. We hope it is clear that the cones that have the greatest `structure' are those

for which there are n sheets all separated from each other; of course, some of these

sheets might be flat (hyperplanes) or lines (one-dimensional).

The surprising result, which can only be verified by obtaining certain integral

estimates on the bounds for u, is that the Cauchy problem for the system (2.51)

only turns out to be well posed in the sense defined after (2.24) when the normal

and ray cones have the maximum structure they could have. To be precise, the

Cauchy data must be prescribed on what is called a space-like hypersurface. Such

hypersurfaces only exist if the ray cone has the maximal number of sheets that

it could have and, in addition, all the sheets are `nested' inside each other. Then

the space-like hypersurfaces lie outside the `outer' sheet of the ray cone; directions

pointing inside this outer sheet are called time-like vectors.

We can illustrate this idea by combining two gasdynamics models, the unsteady

one-dimensional model (2.3) and (2.4), and the steady two-dimensional model

(2.5)-(2.7) and, as in the example on p.42, linearising about a uniform velocity

(U, 0) to give

Oil 8u

_ _ao 8p

+ U

8v OF)

ao 8p

8t 8x

po 8x'

8x po 8y'

8p 8p

eu 8v

(2.57)

8t +U

8x + pogo 8x

+ 8y

= 0.

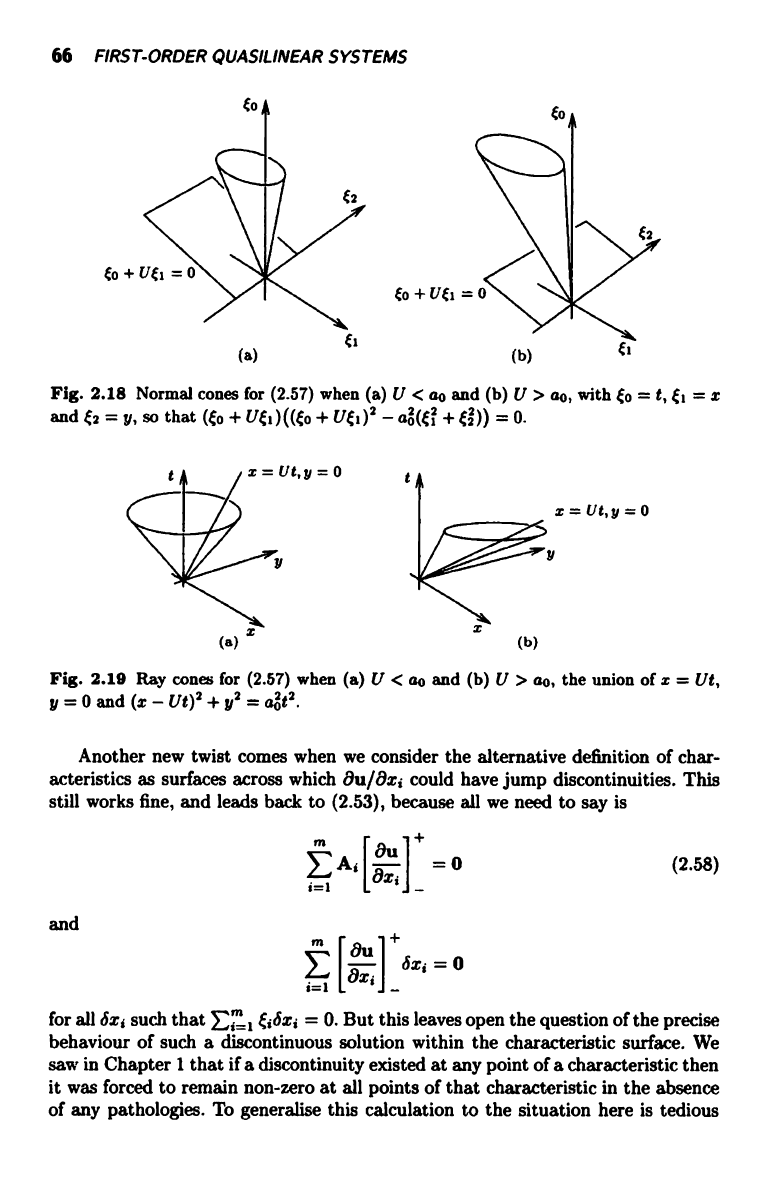
When U > 0, the cones in (x, y, t) space are as in Figs 2.18 and 2.19; the (x, y)

plane is always space-like but, when U > ao, the time axis is no longer time-liken

27The same phenomenon occurs in the theory of relativity, when a particle travels faster than

the speed of light in the material through which it is moving; it emits what is called Zerenkov

radiation.

FIRST-ORDER QUASILINEAR SYSTEMS

to+Uti =

(a)

(b)

Fig. 2.18 Normal cones for (2.57) when (a) U < ao and (b) U > ao, with to = t, ti = x

and t2 = y, so that ({o + U{,) (({o + Uti )2 - a2 (E + ai )) = 0.

x=Ut,y=0

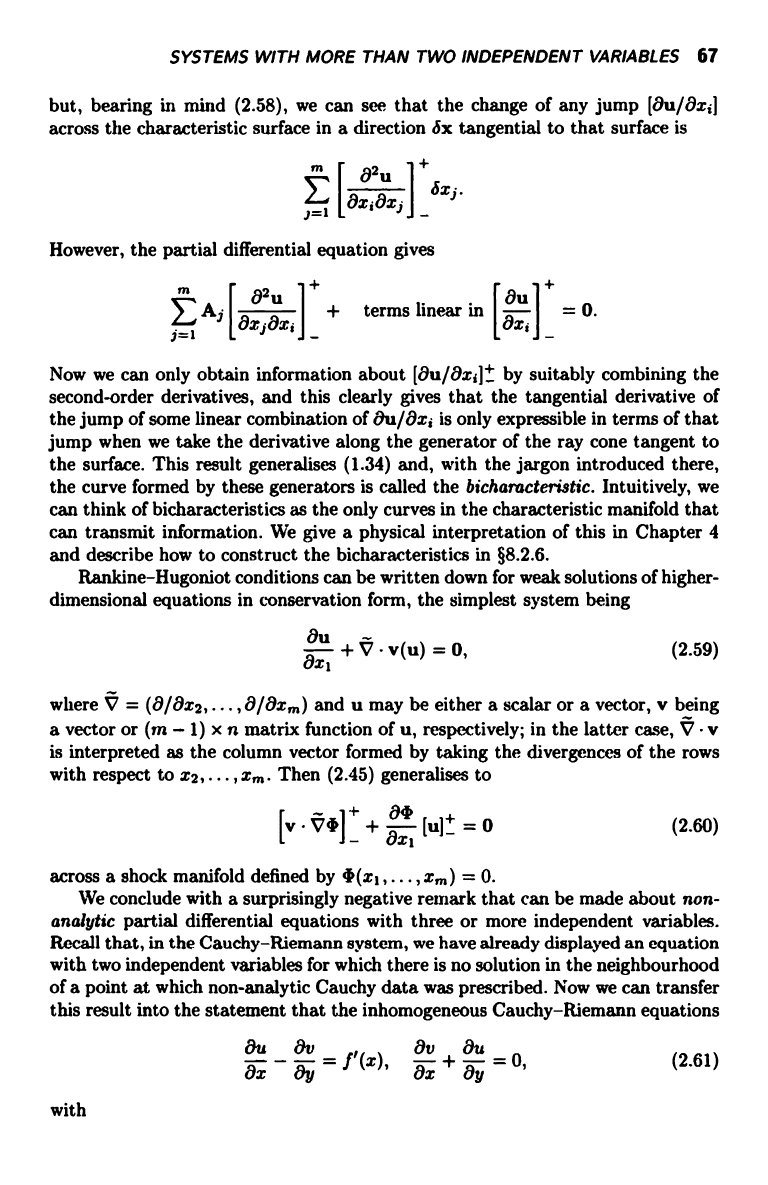
Fig. 2.19 Ray cones for (2.57) when (a) U < ao and (b) U > ao, the union of x = Ut,

y = 0 and (x - Ut)2 + y2 = apt2.

Another new twist comes when we consider the alternative definition of char-

acteristics as surfaces across which 8u/8x; could have jump discontinuities. This

still works fine, and leads back to (2.53), because all we need to say is

(2.58)

and

[i9u1+6.T,=0

t=i

for all dxi such that E', {6x{ = 0. But this leaves open the question of the precise

behaviour of such a discontinuous solution within the characteristic surface. We

saw in Chapter 1 that if a discontinuity existed at any point of a characteristic then

it was forced to remain non-zero at all points of that characteristic in the absence

of any pathologies. To generalise this calculation to the situation here is tedious

SYSTEMS WITH MORE THAN TWO INDEPENDENT VARIABLES 67

but, bearing in mind (2.58), we can see that the change of any jump [Ou/8x11

across the characteristic surface in a direction bx tangential to that surface is

x; x; -

However, the partial differential equation gives

ax;ax;

j_1

dx3.

terms linear in

Now we can only obtain information about [8u/ax;]± by suitably combining the

second-order derivatives, and this clearly gives that the tangential derivative of

the jump of some linear combination of Ou/8x, is only expressible in terms of that

jump when we take the derivative along the generator of the ray cone tangent to

the surface. This result generalises (1.34) and, with the jargon introduced there,

the curve formed by these generators is called the bicharacteristic. Intuitively, we

can think of bicharacteristics as the only curves in the characteristic manifold that

can transmit information. We give a physical interpretation of this in Chapter 4

and describe how to construct the bicharacteristics in §8.2.6.

Rankine-Hugoniot conditions can be written down for weak solutions of higher-

dimensional equations in conservation form, the simplest system being

v(u) = 0,

(2.59)

where V _ (0/8x2, ... , 010x,,,) and u may be either a scalar or a vector, v being

a vector or (m - 1) x n matrix function of u, respectively; in the latter case, t v

is interpreted as the column vector formed by taking the divergences of the rows

with respect to x2, ... , x,,,. Then (2.45) generalises to

[ U ]

(2.60)

across a shock manifold defined by

xm) = 0.

We conclude with a surprisingly negative remark that can be made about non-

analytic partial differential equations with three or more independent variables.

Recall that, in the Cauchy-Riemann system, we have already displayed an equation

with two independent variables for which there is no solution in the neighbourhood

of a point at which non-analytic Cauchy data was prescribed. Now we can transfer

this result into the statement that the inhomogeneous Cauchy-Riemann equations

au av ,

8x-8y=f(x), 8x+8y=0,

02u

]

[

(2.61)

with