Morris & Fan. Reservoir Sedimentation Handbook
Подождите немного. Документ загружается.

SEDIMENT DEPOSITS IN RESERVOIRS 10.21
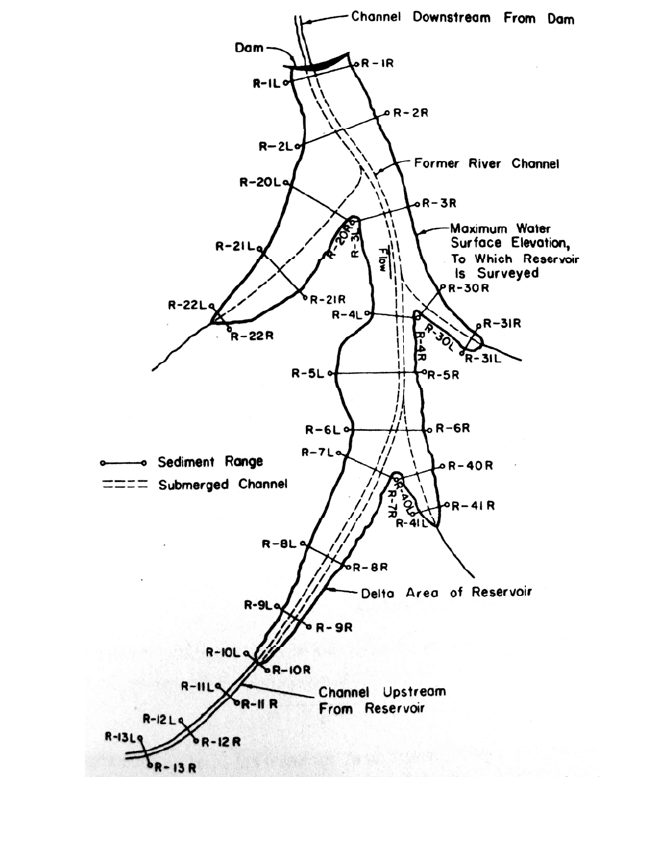
FIGURE 10.13 Location of ranges for measurement of reservoir sedimentation (Borland,
1971)
Estab
lish range lines perpendicular to the reservoir or tributary axis at locations such
that average conditions in the intervening reach will be accurately represented by the
average of the two bounding ranges.
Locate ranges across the mouth of each principal arm or tributary, with additional
ranges extending upstream as along the main stem.
The most downstream reservoir range should be located immediately upstream of the toe
of the dam.
Spacing between ranges may be closer in the delta or areas of special interest, and
ranges should also be located at structures or other features that are particularly sensitive
to sediment impacts.
Range lines should extend to the anticipated aggradation limit above the pool level, and
downstream degradation limit. By convention, range lines are normally assigned numbers
SEDIMENT DEPOSITS IN RESERVOIRS 10.22
or stations that increase moving upstream, and range lines should be plotted from left to
right when looking downstream.
The number of ranges depends on reservoir size and geometry, with a minimum of
three ranges required for even the smallest impoundment. As a rough guide, the average
number of ranges used by the Bureau of Reclamation in 57 reservoirs from about 30 to
15,000 ha in surface area is given by
Number of ranges = 2.942 A
0.3652
(10.3)
where A = reservoir surface area, ha. The exponent value is 0.2875 for A in acres. This
relationship provides only a very approximate guideline, as there is considerable scatter
in the data. For instance, reservoirs with a surface area around 500 ha had from 12 to 60
ranges (Blanton, 1982).
10.7.2 General Considerations for Computing Volumes from Ranges
Several methods are available to compute volumes from ranges. Each of these methods
may be applied to range end areas for either water depth or sediment thickness, to
respectively compute capacity or sediment accumulation within each reach. Because each
computational method will produce different results, it is essential that identical
computational methods be used along each reach for each survey so that the changes in the
computed volume represent sedimentation rather than differences in computational technique.
Automated surveying techniques used for contour surveys are also applicable to range
surveys and can largely eliminate errors due to displacement of range lines during data
collection.
10.7.3 Computing Range End Areas
The end area between adjacent sounding points along a range line is computed by
E
LD
1
D
2
2
(10.4)
where D
1
and D
2
are the depths (or sediment thickness) at adjacent sounding points
separated by interval length L. The total end area of a range line is the sum of the
segment end areas so computed. When soundings are approximately equally spaced, the
following formula may be used to compute the total end area of the entire range, or
along a segment of a range having equally spaced sounding intervals
E L
D
1
2
D
2
D
3
...
D
n
2
(10.5)
where L is the equally spaced sounding interval along the range. As is evident,
computations are greatly simplified when the spacing between sounding points is
uniform.
10.7.4 Average End Area
The simplest volume computation method is the average end-area method:
Volume
LE
1
E
2
2
(10.6)

SEDIMENT DEPOSITS IN RESERVOIRS 10.23
where L = length between ranges and E
1
and E
2
are the cross-sectional end areas of the ranges
bounding the downstream and upstream limits of the reach. To obtain accurate results with
this method requires that the cross sections extend perpendicular across the pool and tributary
arms at points representative of the reservoir cross-section area. Because reservoirs
commonly have an irregular shape and range lines may not be located in areas
representative of the average reservoir cross section, large inaccuracies may result by
multiplying the average range end area by the reach length.
10.7.5 Surface Area—Average End Area
A more accurate estimate of the volume bounded by ranges may be obtained by using
average end areas and top widths to determine the mean water depth below normal pool, and
multiplying mean depth by the intervening surface area. It is assumed that the average
depth at the ranges is representative of the average depth across the intervening pool area. The
equation is
Volume
A
2
E
1
W
1
E
2
W
2
(10.7)
where A = pool surface area between ranges with end areas E
1
and E
2
and top widths W
1I
and W
2
.
If there is only one range in a segment, such as will occur above the most upstream
range along each tributary, locate a point at the upstream limit of the tributary and treat it
as a range line having zero cross-sectional area (E
2
= 0 and W
2
= 0). For the reach
between the most downstream cross section and the sloping face of an embankment dam,
compute the volume by:
V A
E
W
V
0
(10.8)
where V
0
is the volume displaced by the upstream face of the dam.
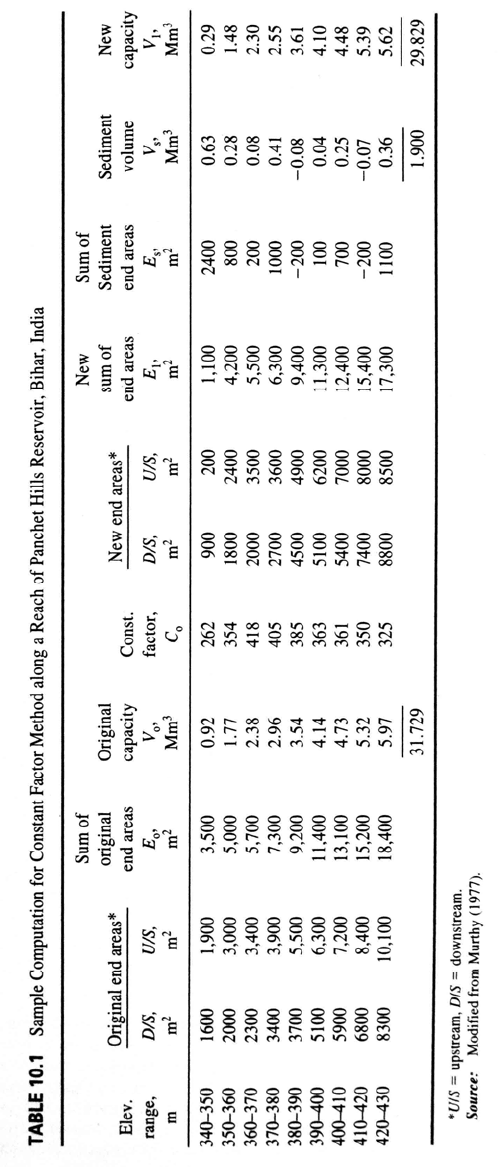
5.1.1. Constant Factor Method
The constant factor method (Burrell, 1951; Borland, 1971) is a range method in which a
constant factor relationship is computed between the range end areas and the total volume
in each segment. It has the advantage of a high level of precision for computing the incremental
volume loss due to sedimentation, and also computes volume loss as a function of
elevation, allowing the stage-capacity relationship to be revised.
To apply this procedure first compute the volume and range end areas for each reach
and for each elevation increment, based on a contour survey. The constant factor is then
computed as
C
0
V
0
E
0
(10.9)
where V
0
= original capacity of the reach, E
0
= original sum of end areas for the reach,
and C
0
= constant factor. In practice, this means that once the volume of a segment has
been computed, the new volume may be computed following sedimentation by applying
the constant factor to the new end areas computed within each elevation increment. The
change in the volume of sediment deposits is determined by applying the constant factor
to the change in the end area between surveys:

SEDIMENT DEPOSITS IN RESERVOIRS 10.24
V
s
C
0
E
s
C
0
(
E
0
E
1
) (10.10
where E
0
is the sum of end areas from the original survey and E
1
is from the subsequent
survey, V
s
= sediment volume deposited in (or scoured from) the reach, and E
S
= (E
0
- E
1
)
= sum of sediment end areas bounding the reach.
This method is illustrated with computations for the reach between ranges 3 and 4 at
Panchet Hill Reservoir in India (Table 10.1). Columns 1 to 5 are data from the original
survey, and the constant factor C
0
in column 6 is computed from C
0
= V
o
/E
0
using data in
columns 4 and 5 for each depth increment. The new end areas are obtained from subsequent
survey and are summed in column 9. The sum of sediment end areas is computed as E
S
= E
0
- E
1
,
the sediment volume is computed as V
S
= C
0
E
S,
and the new capacity is computed as V
1
=
V
0
– V
S
.
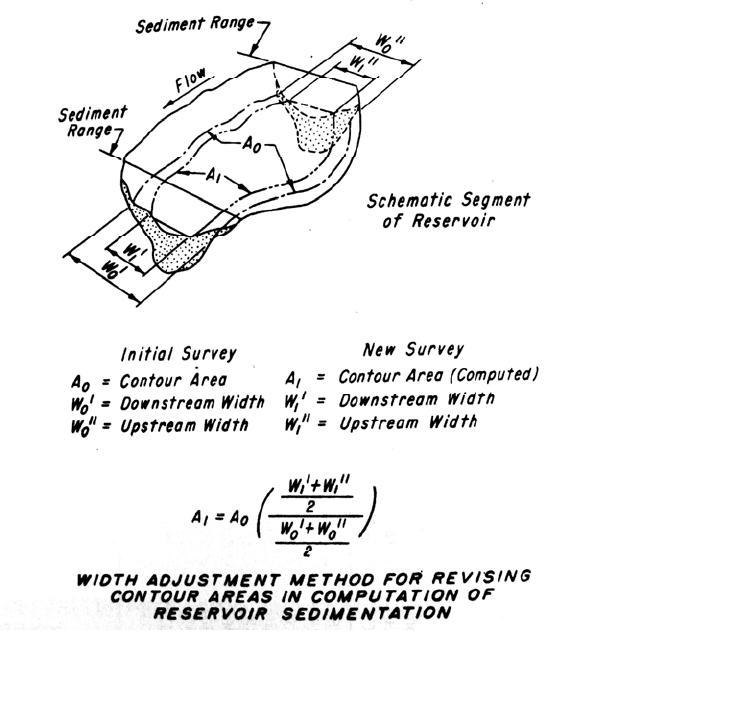
10.7.7 Width Adjustment Method
The width adjustment method (Blanton, 1982) is a range method used by the Bureau of
Reclamation and derived from the constant factor described previously. To use the
constant width method the new contour area A
1
between two ranges is computed for an
adjustment factor defined as the ratio of the new average width to the original average width
for both the upstream and downstream ranges at the specified contour. The computational basis
for a single depth increment within a reach is illustrated in Fig. 10.14. After all reaches have
been computed, the reach areas at each contour elevation are summed across the entire
reservoir to construct the revised stage-area curve.
10.8 RESERVOIR RELEASING AND TRAPPING
EFFICIENCY
The sediment release efficiency of a reservoir is the mass ratio of the released sediment to
the total sediment inflow over a specified time period. It is the complement of trap efficiency:
Release efficiency = 1 - trap efficiency (10.11)
Churchill (1948) based his empirical relationship on the concept of sediment releasing.
whereas Brune (1953) used the concept of sediment trapping which has come into more common
use. However, sediment release efficiency is the more useful concept from the sediment
management standpoint because it can also be used to evaluate flushing, which can release
more sediment than enters, thereby producing a release efficiency in excess of 100 percent.
If expressed in terms of trap efficiency, this would yield a negative value, which is
difficult to conceptualize. Because the sediment trapping or releasing efficiency can be
computed over any time period (e.g., single-event or long-term) the time frame should be
specified.
Both Brune's and Churchill's empirical relationships have been widely used and found
to provide reasonable estimates of long-term releasing or trapping efficiency, but Borland
(1971) notes that the Churchill method is more applicable for estimating sediment retention
in desilting and semidry reservoirs. Both methods are based on the ratio of volume to inflow,
and neither method specifically considers features such as the grain size of the inflowing
sediment load and the outlet configuration. The effects of reservoir operation are included only
to the extent that they are reflected in the selection of the operation are included only to the
extent that they are reflected in the selection of the pool volume use in the computations.
Judgment is required to adjust these methods to specific conditions. For instance, Frenete
SEDIMENT DEPOSITS IN RESERVOIRS 10.25
SEDIMENT DEPOSITS IN RESERVOIRS 10.26
and Julien (1986) used Brune's, Churchill's, and other empirical methods at Peligre
Reservoir in Haiti and found all methods significantly overestimated release efficiency.
This was attributed to the high rate of clay flocculation, producing sediment fall rates
much higher than the rate for discrete particles. A predominance of slowly settling fine
sediment and a low-level outlet will tend to increase sediment release, while
predominantly coarse inflow will reduce sediment release compared to "average"
reservoirs. Empirical methods incorporating particle size as a variable have also been
developed (Borland, 1971; Chen, 1975). Mathematical modeling is required to provide
information on single-event behavior and the effect of changed operating rules on
sediment release.
FIGURE 10.14 Width adjustment method used
by U.S. Bureau of Reclamation for revising contou
r
area (Blanton, 1982).
10.8.1 Brune Curve
Brune (1953) developed an empirical relationship for estimating long-term trap
efficiency in normally impounded reservoirs based on the correlation between the
capacity to inflow ratio (C:I) and trap efficiency observed in Tennessee Valley Authority
reservoirs in the southeastern United States (Fig. 10.15). This is probably the most
widely used method for estimating the sediment retention in reservoirs, and gives
reasonable results from very limited data: storage volume and average annual inflow. As
a limitation, the method is applicable only to long-term average conditions. Brune
noted that significant departures can occur as a result of changes in the operating rule.
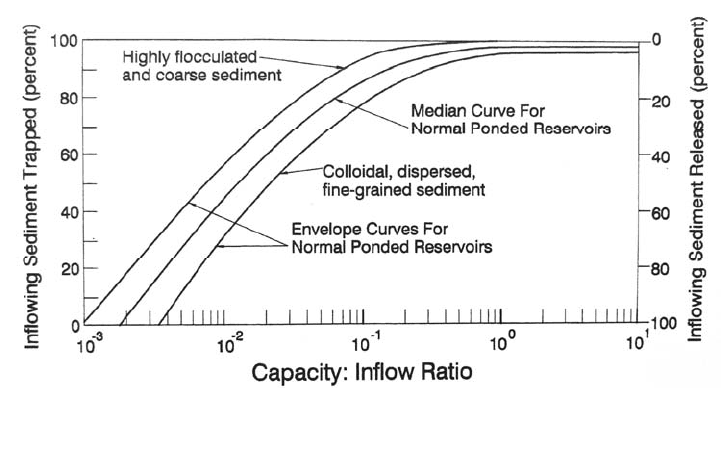
SEDIMENT DEPOSITS IN RESERVOIRS 10.27
FIGURE 10.15 Brune curve for estimating sediment trapping or release efficiency in
conventional impounding reservoirs (adapted from Brune. 1953).
N
ormally dry reservoirs tend to be less efficient at trapping sediment, and shallow
sediment-retention basins designed for the express purpose of trapping sediment can
operate much more efficiently than indicated by the curve. For instance, the All-
American Canal desilting basins in Arizona would have negligible sediment trapping
efficiency based on their C:I ratio, but the basins operate at a trapping efficiency of 91.7
percent. Trapping efficiency also depends on the actual storage level at which the
reservoir is held during flood periods (as opposed to its nominal storage capacity), and
the placement of outlets.
10.8.2 Churchill Method
Churchill (1948) developed a relationship between the sediment release efficiency and
the sedimentation index of the reservoir, defined as the ratio of the retention period to the
mean flow velocity through the reservoir (Fig. 10.16). The minimum data required to use
this method are storage volume, annual inflow, and reservoir length. In applying the
Churchill method, the following definitions are used:
Capacity. Reservoir capacity at the mean operating pool level for the analysis period
(m
3
or ft
3
)
Inflow. Average daily inflow rate during study period (m
3
/s or ft
3
/s)
Retention period. Capacity divided by inflow rate (s)
Length. Reservoir length at mean operating pool level (m or ft)
Velocity. Mean velocity computed by dividing inflow by average cross-sectional
area. The average cross-sectional area can be determined by dividing reservoir
capacity by length (m/s or ft/s).
Sedimentation index. Retention period divided by velocity.
The sedimentation index computed form the above definitions is applied to the curve in
Fig. 10.16 to estimate the sediment release efficiency. Churchill’s method can be used to
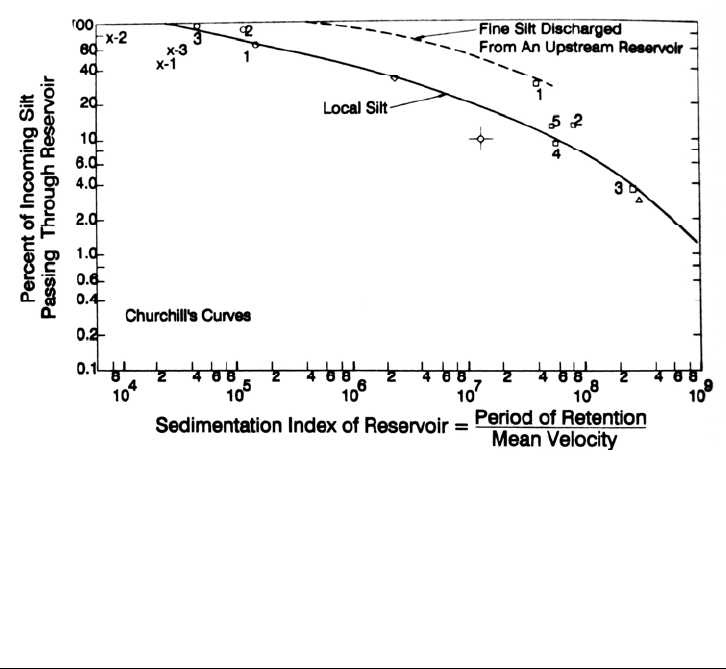
SEDIMENT DEPOSITS IN RESERVOIRS 10.28
FIGURE 10.16 Churchill curve for estimating sediment release efficiency (adapted from
Churchill, 1948).
esti
mate the release efficiency in settling basins, small reservoirs, flood retarding
structures, semidry reservoirs, or reservoirs that are continuously sluiced.
10.9 SPECIFIC WEIGHT OF RESERVOIR DEPOSITS
Inflowing sediment load is measured in terms of its mass. To predict storage depletion,
sediment mass is converted to volume based on deposit density. Conversely, the volume
of surveyed sediments in an existing reservoir must be converted into mass to estimate
sediment yield from the watershed. The dry weight of a deposit per unit volume is
variously called the specific weight, unit weight, or dry bulk density. The specific
weight of a sediment deposit is estimated in a two-step process: (1) the initial specific
weight is estimated and (2) the effect of consolidation with time is computed. Methods
for converting the volume of deposited sediment to units of mass have been reviewed by
Heinemann and Rausch (1971).
10.9.1 Compaction Processes
Specific weight is determined by grain size, deposit thickness, and whether the deposit
has been exposed to the air and allowed to dry. Consolidation is a time-dependent
process which increases specific weight, and reservoir sediments may consolidate for
decades because of self-weight plus overburden from additional sedimentation.
Coarse sediment particles rest directly against one another as soon as they are
deposited, and the void spaces between coarse grains are large enough that water can freely
escape from between the grains. As a result, coarse sediments achieve essentially ultimate
density as soon as they are deposited, and the subsequent rearrangement of the grains and
compression of the deposit is negligible. Silts and clays, however, typically settle initially

SEDIMENT DEPOSITS IN RESERVOIRS 10.29
into a loose matrix with interparticle bridging, resulting in a large volume of small water-filled
voids. As additional layers of sediment are deposited, additional pressure is applied and the
interparticle bridges can collapse, compressing the sediment. However, the overlaying sediments
which compress the deposit also act as a confining layer which retards the vertical migration and
escape of the void water, thereby impeding and prolonging the compaction process. The poor
permeability of clay can also cause deposits of higher density to overlay layers of lower density,
causing a reversal of the vertical density gradient, as reported for deposits in Lake Mead (Fig.
10.17). Grain size variation will also cause bulk density to vary with depth. Bulk density is highest
in the coarse materials deposited in the delta region of the reservoir, and decreases in the direction
of the dam as the deposits become finer-grained.
10.9.2 Range of Unit Weights
The density of solid sediment particles is approximately 2.65 t/m
3
. The dry bulk densities
for 1129 samples of reservoir sediments reported by Lara and Pemberton (1963) ranged
from 1.8 to 3.0 t/m
3
. The guidelines in Table 10.2 developed by the U.S. Natural Resources
Conservation Service illustrate the range of specific weights commonly encountered in
reservoirs as a function of both grain size and consolidation processes.
10.9.3 Lara-Pemberton Method for Initial Bulk Density
Lara and Pemberton (1963) developed an empirical method for estimating the initial specific
weight of sediment deposits based on the analysis of some 1300 sediment samples from
Figure 10.17 change in dry bulk density of reservoir sediment as a function of depth, sampling
location 5, Lake Mead (Standard and Pemberton, 1987).

SEDIMENT DEPOSITS IN RESERVOIRS 10.30
Table 10.2 Typical Specific Weights for Reservoir Deposits
Dom
inant grain size Always submerged Aerated
Metric (t/m
3
or g/cm
3
):
Clay 0.64 to 0.96 0.96 to 1.28
Silt 0.88 to 1.20 1.20 to 1.36
Clay-silt mixture 0.64 to 1.04 1.04 to 1.36
Sand-silt mixture 1.20 to 1.52 1.52 to 1.76
Sand 1.36 to 1.60 1.36 to 1.60
Gravel 1.36 to 2.00 1.36 to 2.00
Poorly sorted sand and gravel 1.52 to 2.08 1.52 to 2.08
U.S. Customary Units (1h/ft
3
):
Clay 40 to 60 60 to 80
Silt 55 to 75 75 to 85
Clay-silt mixture 40 to 65 65 to 85
Sand-silt mixture 75 to 95 95 to 110
Clay-silt-sand mixture 50 to 80 80 to 100
Sand 85 to 100 85 to 100
Gravel 85 to 125 85 to 125
Poorly sorted sand and gravel 95 to 130 95 to 130
Source: Geiger (1963).
reservoirs and rivers. This method incorporates the two primary factors influencing bulk
density: (1) grain size distribution taking into account the presence of clays and (2)
reservoir operation which affects consolidation and air drying. Data on the bulk density
of deposits from subsequent reservoir resurveys by the Bureau of Reclamation have
supported the Lara and Pemberton equation (Strand and Pemberton, 1987).
To estimate initial specific weight, classify the reservoir operation into one of four
categories: (1) sediment always submerged or nearly submerged, (2) normally
moderate to considerable drawdown, (3) normally empty reservoir, and (4) riverbed
sediments. The sediment composition must also be divided among the sand, silt, and
clay fractions. The specific weight computation is performed by using the values in
Table 10.3 and the following equation:
W
W
c
P
c
W
M
P
M
W
S
P
S
(10.12)
where W = is the deposit specific weight (kg/m
3
or lb/ft
3
); P
C
, P
M
, P
S
= the percentages
of clay, silt, and sand, respectively, for the deposited sediment; and W
C
, W
M
, W
S
= the
TABLE 10.3 Coefficient Values for Lara-Pemberton Equation
Initial weight kg/m
3
(lb/ft
3
)
W
C
W
M
W
S
Operational Condition
Continuously submerged 416 (26) 1120 (70) 1154 (97)
Periodic drawdown 561 (35) 1140 (71) 1154 (97)
N
ormally empty reservoir 641 (40) 1150 (72) 1154 (97)
Riverbed sediment 961 (60) 1170 (73) 1554 (97)
