Morris & Fan. Reservoir Sedimentation Handbook
Подождите немного. Документ загружается.

APPENDIXES A.4
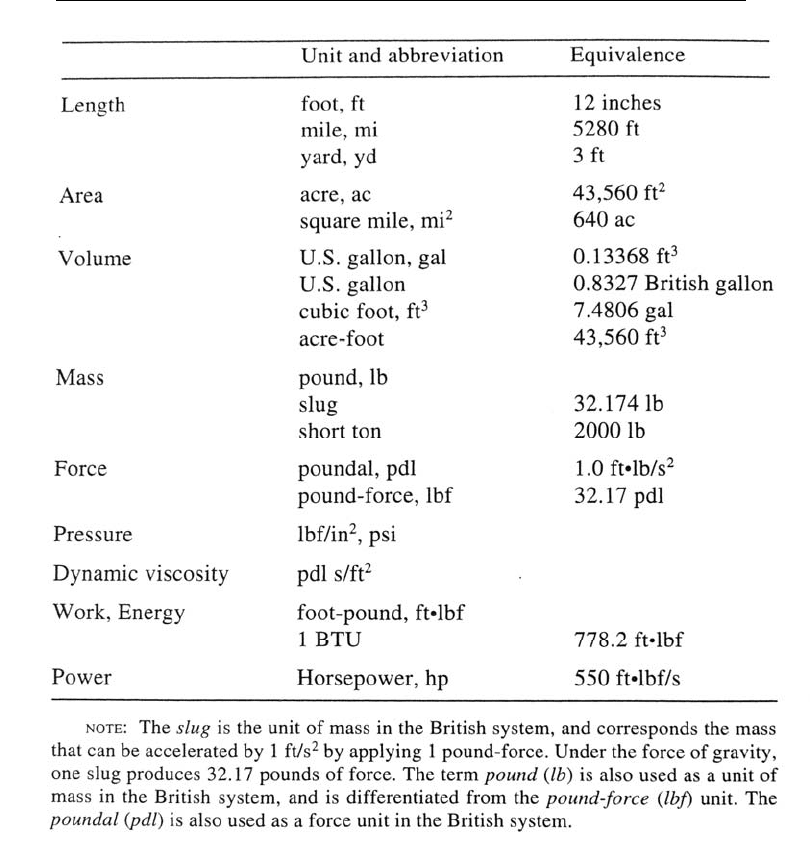
G. UNITS OF MEASURE IN BRITISH OR U.S.
CONVENTIONAL UNITS (FPS SYSTEM)
APPENDIXES A.5
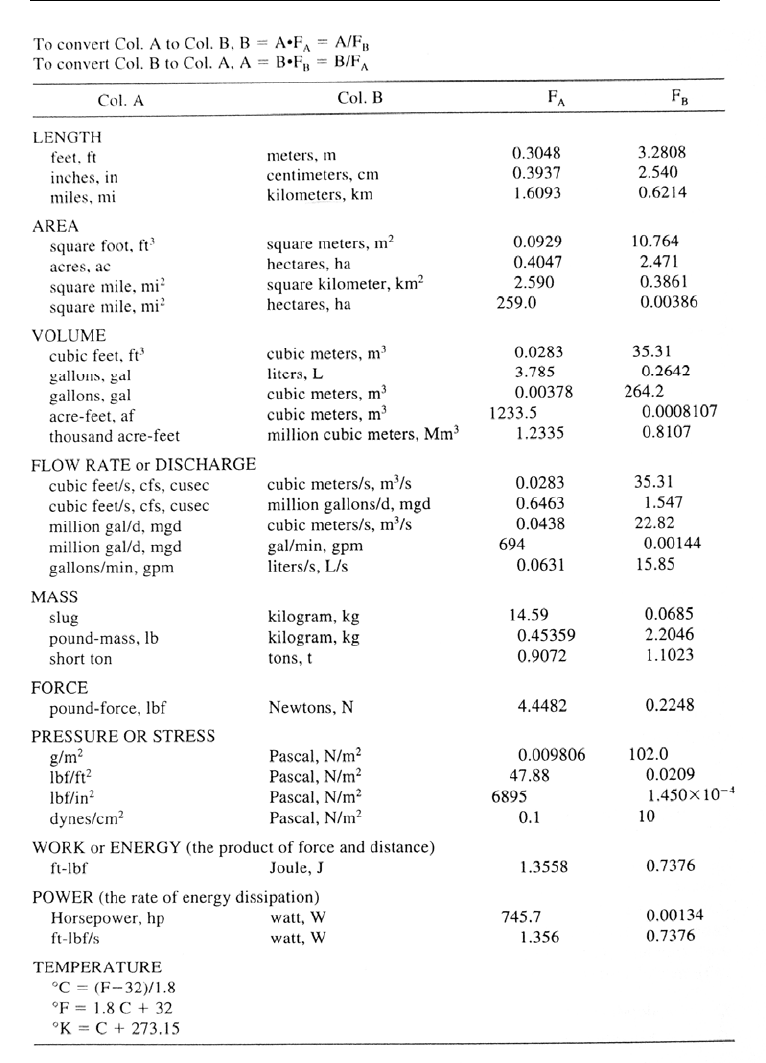
H. TABLE OF UNIT CONVERSION FACTORS
APPENDIXES A.6
I. DENSITY OF WATER OR WATER-SEDIMENT
MIXTURES
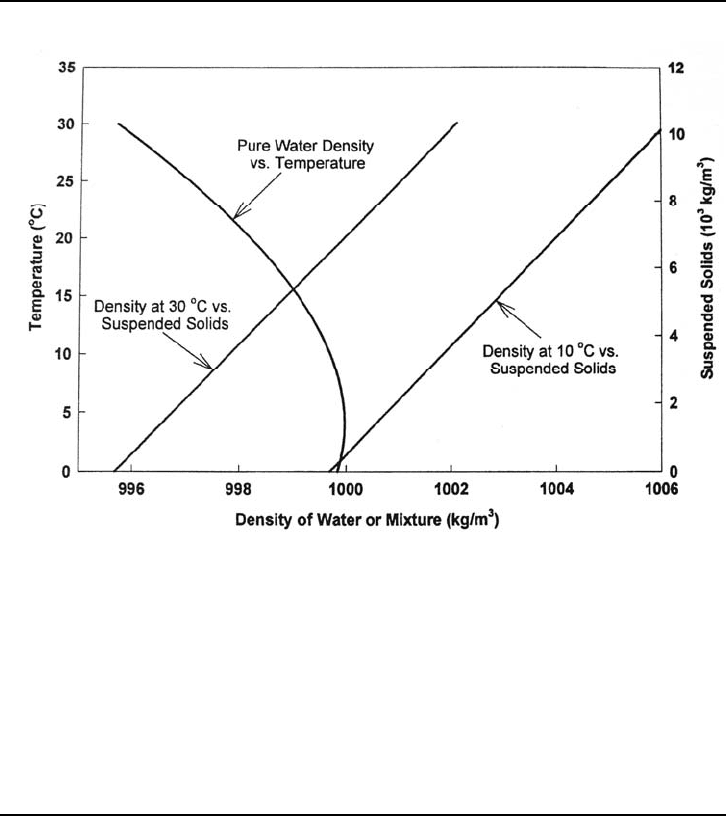
FIGURE App I.1 Density of pure water vs. temperature, and of water-sediment
mixtures as a function of suspended solids concentration at two different water
temperatures. These graphs are computed from the equations given in Section 4.2.3.
J. STREAM POWER
Stream power is an important concept in sediment transport, and is the basis for several
methods to estimate the initiation of erosion, to determine the rate of transport of bed
material load (see Secs. 9.7.5, 9.7.6, and 9.9.3). Chang (1988) has hypothesized that the
geomorphic form of alluvial channels evolves in a way that minimizes stream power
per unit channel length. This appendix presents basic stream power relations, using the
definitions and units presented below and in Sec. 9.1.
D = depth (m)
B = breadth (width) of channel (m)
∆E = change in energy grade line (m)
APPENDIXES A.7
L = channel length (m)
P = total power expenditure or rate or energy dissipation (W)
Q = DBV = total discharge (m
3
/s)
q = Q/B = discharge per unit channel width (m
3
/s m = m
2
/s)
S = slope of energy grade line (dimensionless)
V = velocity (m/s)
γ = gamma, the unit weight of fluid (N/m
3
)
τ = γDS = bed shear stress
Units are given in brackets [W = watts].
The rate of energy dissipation or the power of expenditure by flowing water is given
by:
P = γ Q ∆E [W] (J.1)
Thus, the total power expended by 1 m
3
/s of water dropping through a 0.5 m vertical
fall may be computed as:
P = (9810 N/m
3
)(1 m
3
/s)(0.5 m) 4905 W
For flow along a channel let ∆E = SL and Q = DBV so that:
P = γQSL = γ(DBV)(SL) [W] (J.2)
Although in streams the bed slope or the water surface slope can be used to approximate
the energy slope, this approximation may lead to unacceptable error when varying
flow is being analyzed.
The rate of energy dissipation per unit of channel length may be computed by re-
arranging Eq. (J.2)
P / L = γQS = [W/m] (J.3)
The rate of energy dissipation may be computed per unit area of channel bed by
rearranging Eq. (J.2) and substituting
τ =
γDS
P/(BL) = γDSV = τV [W/m
2
] (J.4)
The rate of energy dissipation per unit weight of water is termed unit stream power and
may also be obtained by rearranging Eq. (J.2)
P / (γDBL) = VS [m/s] (J.5)
