Mench M.M. Fuel Cell Engines
Подождите немного. Документ загружается.


c04 JWPR067-Mench January 28, 2008 17:28 Char Count=
140 Performance Characterization of Fuel Cell Systems
match this current as long there is enough voltage potential available. If not, the limiting
current is reached and the current level is not possible.
In general,
i
cell
= i
o
C
s
C
∗
γ
exp
α
a
F
R
u
T
η
−exp
−α
c
F
R
u
T
η
(4.35)
III
where Eq. (4.35) can be used for net oxidation or reduction reactions, with I representing
the oxidation branch of the electrode reaction and II representing the reduction branch.
This is our standard model of BV kinetics and can be used to solve for the activation
and mass transfer polarization at each electrode. Some points to remember:
Ĺ I in Eq. (4.35) is the oxidation reaction at the particular electrode.
Ĺ II in Eq. (4.35) is the reduction reaction at the particular electrode.
Ĺ i
cell
is the fuel cell total current density (the same for both electrodes by conservation
of charge).
Ĺ i
o
is the exchange current density of the electrode of interest and is a function of
reaction concentration, temperature, catalyst, age, and other factors. It is different
for anode and cathode reactions on an electrode: i
o,c
= i
o,a
.
Ĺ C
s
is the electrode reactant concentration at the catalyst surface.
Ĺ C
*
is the reference concentration of the reactant at STP conditions.
Ĺ α
a
is the anodic charge transfer coefficient, the fraction of the activation polarization
energy of reaction that goes toward enhancing the oxidation branch of the reaction at
a given electrode. The parameter α
a,a
refers to the anodic charge transfer coefficient
at the anode, and α
a,c
refers to the cathodic charge transfer coefficient at the anode.
Ĺ α
c
is the fraction of the additional activation polarization energy of the reaction that
goes into enhancing the reduction branch of the equilibrium; α
c
+ α
a
= n, where n
is the number of electrons transferred in the elementary reaction step for the electron
transfer. Experimentally, this value is often found to be a noninteger between 1 and
2 due to multiple charge transfer reactions.
Ĺ γ is the reaction order for the elementary charge transfer step, which can vary for
different electrodes and reactants and is typically determined experimentally.
Ĺ η is the activation overpotential at the given electrode and is in units of volts.
Exchange Current Density Exchange current density i
o
is a very important parameter that
has a dominating influence on the kinetic losses. It appears from Eq. (4.35) that activation
polarization should increase with temperature. However, i
o
is a highly nonlinear function of
the kinetic rate constant of reaction and the local reactant concentration and can be modeled
with an Arrhenius form as
i
o
(T , C
R
) = i
o,ref
exp
−
E
a
R
u
T
C
R
C
R,ref
γ
(4.36)
where R refers to the reactant at that electrode and i
o,ref
is the reference exchange current
density with no concentration loss and at a reference temperature. It is important to note
that the exchange current density is not an intrinsic function of a catalyst, although it is
c04 JWPR067-Mench January 28, 2008 17:28 Char Count=
4.2 Region I: Activation Polarization 141
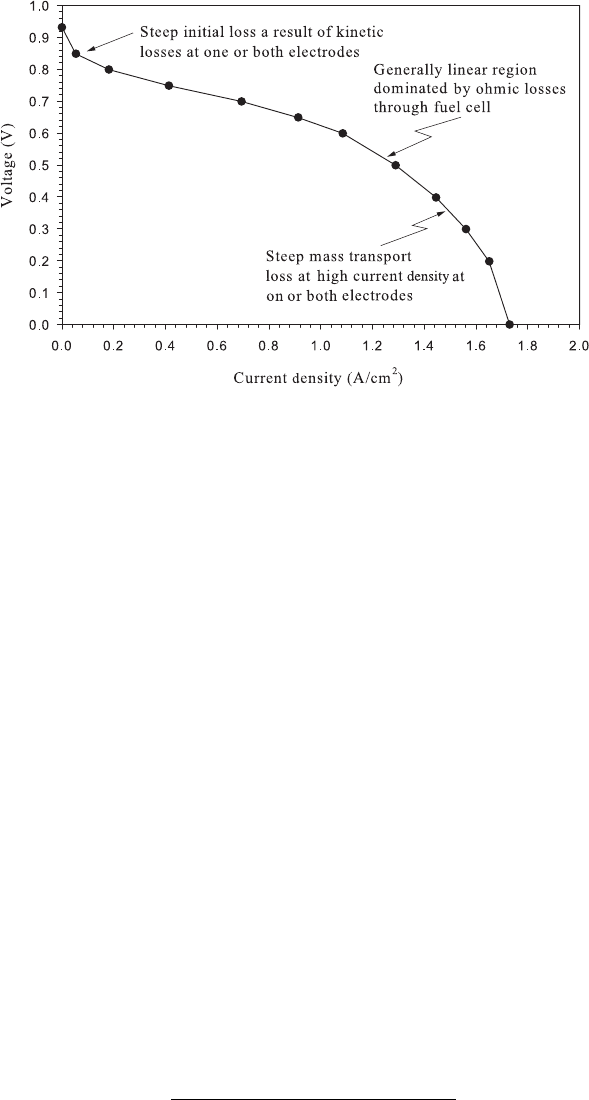
Figure 4.18 Typical polarization curve for low-temperature PEFC. Despite the use of an expensive
platinum catalyst, there is still significant activation polarization. The fuel cell is operating at 65
◦
C,
with zero back pressure, 100% RH on anode and cathode, anode stoichiometry of 1.5, and cathode
stoichiometry of 2.0.
most strongly related to the catalyst choice. It is also a function of electrode morphology,
catalyst type, reactant, temperature, pressure, age, and other factors. Two electrodes with
the same catalyst density can have different exchange current density values. Another
point concerning the exchange current density is as follows: In general, the more complex
the reactant molecule, the lower the exchange current density. Therefore, we expect the
hydrogen oxidation exchange current density for a given electrode to be high relative to a
methanol oxidation exchange current density for the same electrode.
Thus, i
o
is an exponentially increasing function of temperature and the net effect
of increasing temperature is to significantly decrease activation polarization in a highly
nonlinear fashion. For this reason, high-temperature fuel cells such as SOFC or MCFC
typically have very low activation polarization and can use less exotic catalyst materials.
Accordingly, the effect of an increase in electrode temperature is to decrease the voltage
drop within the activation polarization region. Typical polarization curves from a low-
temperature PEFC and a high-temperature SOFC are shown in Figures 4.18 and 4.19,
respectively. Within the various fuel cell systems, however, the operating temperature range
is typically dictated by the electrolyte and material properties, so that temperature cannot
be arbitrarily increased to reduce activation losses. For example, the operating temperature
of a conventional PEFC is limited to <120
◦
C due to electrolyte material limitations.
Roughness Factor In some cases, the ratio of the actual electrochemically active catalyst
surface area to the planform geometric surface area is used as a separate parameter to
delineate morphology effects, called the roughness factor a:
a =
actual electrochemically active area
planform geometric area
(4.37)
c04 JWPR067-Mench January 28, 2008 17:28 Char Count=
142 Performance Characterization of Fuel Cell Systems
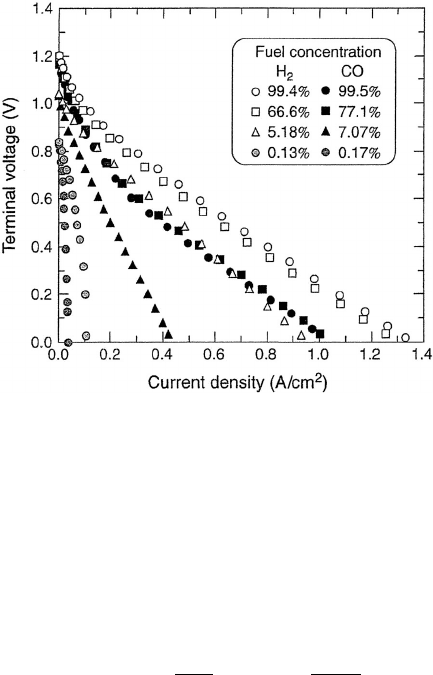
Figure 4.19 Typical polarization curves for high-temperature SOFC in different gas environments
at 1000
◦
C. The high operating temperature enables the use of low-cost catalyst materials such as nickel
(anode) and strontium-doped lanthanum manganite (cathode), with very low kinetic polarization
losses. (Reproduced with permission from [5].)
Roughness factors for carbon-supported platinum electrodes are typically between 600
and 2000 [6], and can decrease with age and operation due to catalyst sintering and
morphological changes due to stresses in the catalyst layer. When the roughness factor is
used as a separate parameter, the BV expression is then shown as
i
cell
= ai
o
exp
α
a
F
R
u
T
η
− exp
−α
c
F
R
u
T
η
(4.38)
and thus some inclusion of catalyst morphological effects can be included in the formulation.
The exchange current density is the dominant parameter in the BV equation and has
a strong effect on the activation loss. Typical values for the exchange current density
for various reactions with an acid electrolyte are given in Table 4.1. Exchange current
densities for alkaline electrolytes are given in Table 4.2. Keep in mind that the exchange
current density is a strong function of temperature, electrode active catalyst area and
morphology, concentration, and other factors. Nevertheless, the relative orders of magnitude
are consistent, and several trends can be noted
1. The HOR for both low- and high-temperature fuel cells in acid (cation transfer) and
alkaline (anion transfer) electrolytes is much more facile than the ORR.
2. A key advantage of alkaline electrolytes is the relatively high exchange current
density for the ORR compared to acid electrolytes which is in general 10 to 100 times
greater than for acid-based electrolytes. Very high operating efficiencies are possible
with these systems, although other limitations certainly exist.
3. Obviously, temperature has a large effect. Comparing the same reaction in a low-
temperature PEFC (80
◦
C) to a high-temperature SOFC (1000
◦
C), i
o
for the HOR

c04 JWPR067-Mench January 28, 2008 17:28 Char Count=
4.2 Region I: Activation Polarization 143
Table 4.1 Selected Typical Exchange Current Densities for Different Reactions and Smooth Elec-
trodes in an Acid Electrolyte at 300 K, 1 atm.
Electrode Reaction Catalyst Value
*
(A/cm
2
)
HOR Pt 1 × 10
−3
HOR Pd 1 × 10
−4
HOR Ni 1 × 10
−5
ORR Pt 1 × 10
−9
ORR Pd 1 × 10
−10
ORR Rh 1 × 10
−11
ORR-PEFC Pt-C 3 × 10
−9
ORR-PEFC PtCr-C 9 × 10
−9
ORR-PEFC PtNi-C 5 × 10
−9
ORR-PEFC PtFe-C 7 × 10
−9
∗
Value given is for smooth electrode surfaces. The roughness factors in fuel cells can range from 600 to 2000,
however, increasing the effective exchange current density.
Source: Data adopted from [7].
and the ORR is orders of magnitude higher for the SOFC, even using inexpensive
nonnoble catalysts that would be entirely ineffective at PEFC temperatures.
4. Surface roughness also plays a key role. The more electrode area is available for
reaction, the higher the effective exchange current density (ai
o
) can be.
4.2.2 Butler–Volmer Simplifications
Considering the electrode polarization–current relationship, there are three regions, as
shown in Figure 4.20:
1. A low-overpotential region where kinetics are facile and relatively low losses
occur
2. A higher overpotential region, where losses become much more significant
3. A very high current region where mass transport losses dominate
The BV equation is a very common model for electrode kinetics applied in most studies of
fuel cells but does not provide an explicit analytical solution for the electrode overpotential
η. Although we can still solve the full BV equation computationally, we would still prefer
an explicit solution for η to enable more simplified calculation and decreased computational
Table 4.2 Selected Typical Exchange Current Densities for Different Reactions and Smooth Elec-
trodes in an Alkaline Electrolyte at 300 K, 1 atm.
Electrode Reaction Catalyst Value
*
(A/cm
2
)
HOR Pt 1 × 10
−4
HOR Pd 1 × 10
−4
HOR Ni 1 × 10
−4
∗
Value given is for smooth electrode surfaces. The roughness factors in fuel cells can range from 600 to 2000,
however, increasing the effective exchange current density.
Source: Data adopted from [7].

c04 JWPR067-Mench January 28, 2008 17:28 Char Count=
144 Performance Characterization of Fuel Cell Systems
Linearized
Kinetics
Region
Tafel Kinetics
Region
Slope breakoff
due to mass
transfer effects
logi
η
activation
i
o
Region 1
Region 2
Region 3
Figure 4.20 Schematic of activation polarization behavior at an electrode.
complexity. Based on the mathematical form of the BV equation, there are several common
simplifications that can be made to achieve this goal.
Simplified Butler–Volmer Equation 1: Facile Kinetics—Linearized Butler–Volmer Model
Whenever the exchange current density is very high and the current is low, a special form
of the BV model can be applied. One has to be careful in its use, however, because the
exchange current density is rarely large enough to justify its use for appreciable operating
current densities. Nevertheless, in situations where the electrode polarization is very low
(see Example 4.1), it is applicable. One example where this linearized version has been
applied is for a pure hydrogen oxidation at high temperatures. The exchange current
density for this reaction can reach 0.5 A/cm
2
[8], which actually covers much of the normal
operating range. Even the SOFC cathode exchange current density can reach 0.2 A/cm
2
at
this elevated temperature.
From the BV equation
i
cell
= i
o
exp
α
a
F
R
u
T
η
− exp
−α
c
F
R
u
T
η
(4.39)
Let x = α
i
Fη/R
u
T. Using a power series expansion to describe e
x
and eliminating the higher
order terms for a numerically small values of x, ∼0 for small x,
x = 1 + x +
x
2
2!
+
x
3
3!
+
...
(4.40)
i
cell
= i
o
α
a
F
R
u
T
η + 1
−
−α
c
F
R
u
T
η + 1
(4.41)
With algebra we can show the desired result, an explicit expression for the activation polar-
ization at the electrode as a function of current density. Keep in mind that this expression
is only valid in the low-loss region circled in Figure 4.21.
η =
i
i
o
R
u
T
(
α
a
+ α
c
)
nF
=
i
i
o
R
u
T
nF
(4.42)

c04 JWPR067-Mench January 28, 2008 17:28 Char Count=
4.2 Region I: Activation Polarization 145
Linearized
kinetics
region
Tafel kinetics
region
Slope breakoff
due to mass
transfer effects
logi
η
activation
i
o
Figure 4.21 Region of applicability for linearized Butler–Volmer model.
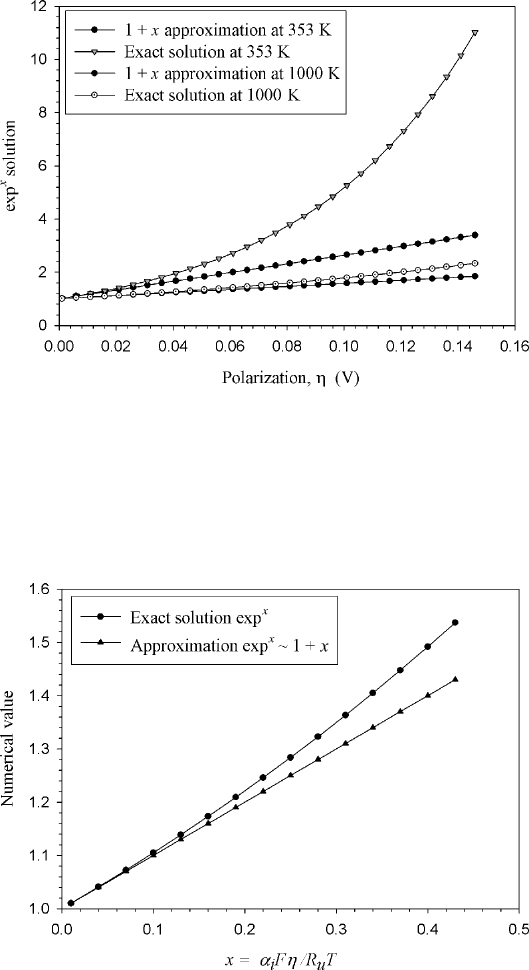
Example 4.1 How Applicable Is the Linearized Assumption? We should always desire
to understand the limitations of our approximations. In this case we would like to examine
the applicability of our assumption that, for values of low polarization, we can assume that,
mathematically,
e
x
≈ 1 + x
(a) Calculate the activation polarization η where the linearized assumption is ap-
propriate for a low-temperature PEFC at 80
◦
C and a high-temperature SOFC at
1000
◦
C.
(b) Calculate the value of x = α
i
Fη/R
u
T where the linearization is appropriate.
SOLUTION (a) To approximate the BV equation as linear, we assume e
x
≈ 1 + x, where
x =
α
i
Fη
R
u
T
Assuming α
a
∼ α
c
∼ 0.5 as a reasonable value with a single electron transfer (n = 1), we
can show that
x =
0.5 × 96,485η
8.314T
C
mol
J
C
mol
J
Rearranging for η,
x = 5802
η
T
If we compare e
x
to the approximation e
x
≈ 1 + x at 353 and 1000 K, we can plot the
following figure:
c04 JWPR067-Mench January 28, 2008 17:28 Char Count=
146 Performance Characterization of Fuel Cell Systems
So for 353 K, as long as the polarization is less than around 40 mV, the linearized approxi-
mation is fairly precise. For the higher temperature SOFC case, the approximate and exact
solutions diverge significantly around 0.1 V.
(b) In this case, we simply plot the exact versus approximate solution for different
values of x. Divergence from the exact solution indicates the imprecision in the linearization
approximation.
From this plot, we can see that the linearized approximation is effective until x = α
i
Fη/R
u
T
> ∼ 0.15. At x = 0.15, the error is only a little over 1%.
COMMENTS: This result shows another way to check the accuracy of the linearized BV
approximation. Although the simplifications to the BV are convenient, it is important to be

c04 JWPR067-Mench January 28, 2008 17:28 Char Count=
4.2 Region I: Activation Polarization 147
Linearized
kinetics
region
Tafel kinetics
region
Slope breakoff
dueto mass
transfer effects
log i
η
activation
i
o
Figure 4.22 Region of applicability for Tafel kinetics model.
sure they are applied correctly. In this case, if x = α
i
Fη/R
u
T < 0.15, the linearized BV is
an acceptable approximation.
Simplified Butler–Volmer Equation 2: High-Electrode-Loss Region of Butler–Volmer
Model—Tafel Kinetics Whenever the exchange current density is very low or the polar-
ization is significant, a special form of the BV model can be applied that applies to the
high-loss region of the electrode polarization curve circled in Figure 4.22. In most cases,
the Tafel kinetics can be applied with little error, since only the small region where the
linearized kinetics are valid is ignored (this region is actually quite smaller in most cases
than Figure 4.22). Examples where Tafel kinetics are applicable include almost every fuel
cell reaction besides pure hydrogen oxidation at high temperature.
From the BV equation, we see that for high polarization one of the branches will
dominate. For an anode reaction with positive η, the anodic branch will exponentially
increase, while the cathodic branch will be a diminishing function. For a cathodic reaction
with negative η, the cathodic branch will exponentially increase, while the anodic branch
will be a diminishing function:
i
cell
= i
o
exp
α
a
F
R
u
T
η −exp
One of the branches will dominated for hi
g
h η
−α
c
F
R
u
T
η
(4.43)
Therefore, with high losses at a given electrode, one of the branches can be neglected,
leaving the Tafel kinetics model. This can be rearranged to provide an explicit expression
for η as a function of cell current:
i
cell
= i
o
±exp
±α
j
F
R
u
T
η
⇒ η =±
R
u
T
α
j
F
ln
i
i
o
(4.44)
where the subscript j is used to indicate the value is related to the dominant branch of the
electrode. Since the ± is awkward to include, it is omitted in the rest of the book. However,
cathode polarization is negative with reference to the SHE and anode polarization is positive
with reference to the SHE, as shown in Figure 4.5.
c04 JWPR067-Mench January 28, 2008 17:28 Char Count=
148 Performance Characterization of Fuel Cell Systems
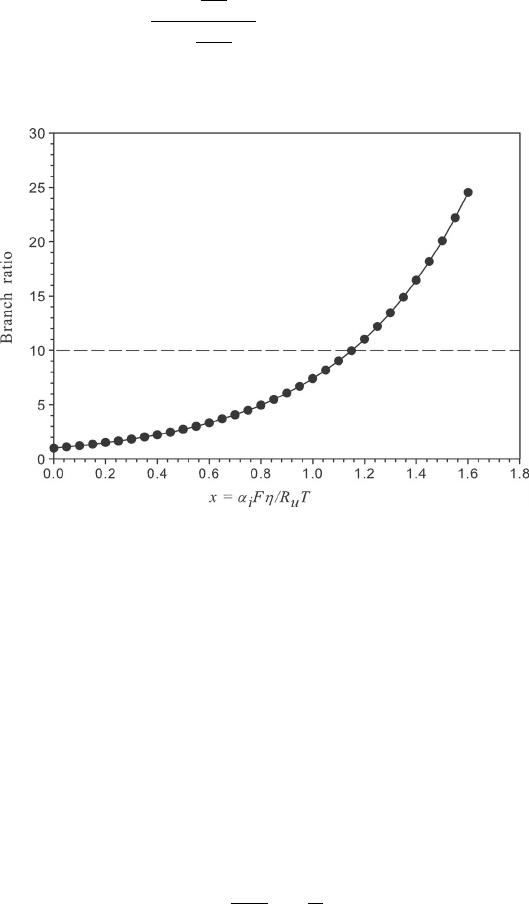
Example 4.2 How Applicable Is the Tafel Assumption? Here we wish to evaluate the
range of validity of the Tafel kinetics assumption. As in Example 4.1, we wish to evaluate
a general criterion for the range where x = α
i
Fη/R
u
T results in one of the branches of the
BV becoming negligible.
SOLUTION To solve, rearrange the two branch terms from the BV equation to form a
ratio of the anodic to cathodic branch:
exp
α
a
F
R
u
T
η
exp
−α
c
F
R
u
T
η
= branch ratio
Assuming positive polarization (net anodic current), we can plot the following:
When x > 1.2, the branch ratio is approximately 10 : 1, and we can apply Tafel kinetics.
COMMENTS: This represents a general criterion for the applicability of the Tafel kinetics
assumption with low error. Before detailed calculations are made, one should always check
the applicability of the assumptions and simplifications made.
Example 4.3 Calculation of Expected Polarization with Tafel Kinetics Plot the activa-
tion polarization as a function of current density for an electrode ignoring all other losses
for an initial OCV of 1.2 V. Use the following values for the parameters: i
o
= 0.3 to × 10
−4
A/cm
2
. Assume the reaction is occurring at 353 K and α
a
= α
c
= 0.5.
SOLUTION From the last example we know Tafel kinetics will be valid when x =
α
i
Fη/R
u
T > 1.2, which occurs for η>0.07 V in this case. With the Tafel approximation,
we have to evaluate
η =
R
u
T
α
j
F
ln
i
i
o
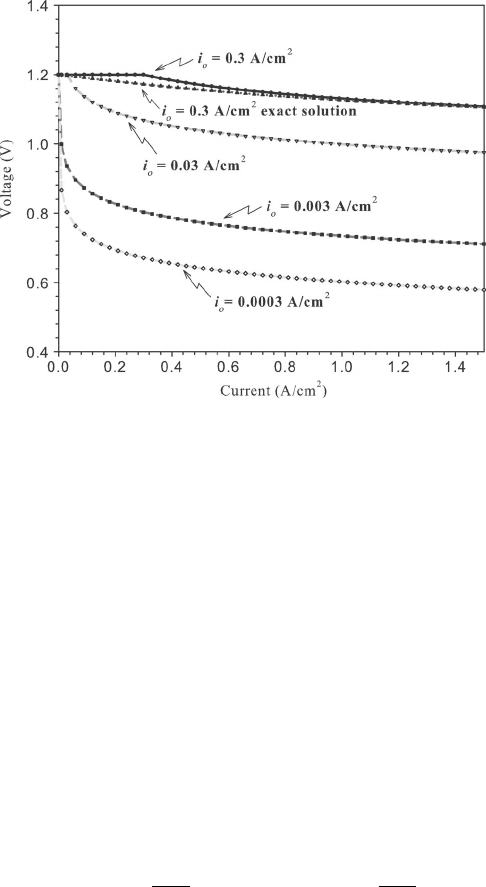
c04 JWPR067-Mench January 28, 2008 17:28 Char Count=
4.2 Region I: Activation Polarization 149
The plot is shown below:
These polarization curves were deduced using Tafel kinetics at a single electrode only and
no other losses. Note that the Tafel expression results in zero losses (mathematically, it will
actually predict negative polarization, which is physically unrealistic) until i > i
o
. The exact
solution shown for i
o
= 0.3 A/cm
2
shows the error associated with the Tafel approximation
with a higher exchange current density.
COMMENTS: Notice the strong effect of the exchange current density near the open
circuit. There are still activation polarization losses accumulating throughout the entire
polarization curve, but the effect is most dramatic at low current density.
Determination of Exchange Current Density In 1905, based on experimental observa-
tion, Tafel proposed the following relationship between electrode overpotential and current
density [10]:
η = a + b log i (4.45)
Using the Tafel approximation from the BV equation (4.35), we can show that
a = 2.303
R
u
T
α
j
F
log i
o
b =−2.303
R
u
T
α
j
F
(4.46)
where b is the Tafel slope. Experimentally, the exchange current density and charge transfer
coefficient are found with a Tafel plot, which is a plot of the log of current density versus
overpotential for a given reaction. From the slope of a semilog plot of voltage versus
current, the charge transfer coefficient can be determined, and from the intercept the
exchange current density can be found. Figure 4.23 is a Tafel plot for a hydrogen PEFC.
Near zero overpotential, these curves deviate from linearity on a log scale (Not shown in
