McMahon D. String Theory Demystified
Подождите немного. Документ загружается.


46
String Theory Demystifi ed
This can only be true if
sin k
π
= 0
, which means that k must be an integer. Denoting
it by n, a simple exercise shows that Eq. (2.47) can be written as
Xx p i
n
en
s
s
n
n
in
µµ µ
µ
τ
τ
α
σ
=+ +
≠
−
∑
C
C
2
0
2
cos( )
(2.53)
Closed Strings
In the case of closed strings, the boundary condition becomes one of periodicity,
namely
XX
µµ
τσ τσ π
(, ) (, )=+2
(2.54)
This condition restricts the solutions to those for which the wave number k takes on
integral values. Hence,
X
x
pi
n
e
L
ss
n
in
µ
µ
µ
µ
τ
τσ τ σ
α
(, ) ( )
(
=+ ++
−
22
2
2
CC
++
≠
∑
σ
)
n 0
(2.55)
X
x
pi
n
e
R
ss
n
in
n
µ
µ
µ
µ
τσ
τσ τ σ
α
(, ) ( )
()
=+ −+
−−
22
2
2
CC
≠≠
∑
0
(2.56)
where n is an integer. In addition, periodicity enforces the condition that
pp
µµ
=
(2.57)
for closed strings. We saw that if this condition was satisfi ed in the case of open
strings, there was no winding of the string permitted. In the case of closed strings,
however, the situation is a little bit more involved if we allow for the possibility
where the ambient space-time includes a compact extra dimension (then
pp
µ
µ
=
does not hold). Then, we can consider, for example, the situation where the closed
string is compactifi ed on a circle of radius R.
Using Eq. (2.57), we sum Eqs. (2.55) and (2.56) to obtain the complete solution,
focusing on the momentum term–and imposing the periodicity condition of Eq. (2.54).
This gives the total solution which can be written as
XRX RW
µµ
τσ π τσ π
(, ) (, )+= +22
(2.58)
CHAPTER 2 Equations of Motion
47
We call W the winding number, which literally tells us how many times the string
has wound around the compact dimension (so W must be an integer). As we let
σ
σπ
→+2
, notice that the momentum terms change as
CC CC
ss ss
pp p
22 22
222
µµµ
τσπ τσπ τσ
()()()++ + −− = + +
222
2
ppp
s
µµµ
τσ
π
() ( )−+ −
C
That is, the total solution changes as
XX pp
s
µµ µµ
τσ π τσ
π
(, ) (, ) ( )+= + −
C
2
2
(2.59)
Therefore, we call
(
)
pp
µµ
−
the winding contribution.
Finally, we consider open strings with fi xed endpoints. The boundary condition is
X
µ
σ
=
=
0
0
(2.60)
(Dirichlet boundary condition). Using Eqs. (2.48) and (2.49), we have
CC
Xpe
L
ss
k
ik
k
µµµτ
τσ α
(, )== +
−
≠
∑
0
2
2
2
0
(2.61)
CC
Xpe
R
ss
k
ik
k
µµµτ
τσ α
(, )== +
−
≠
∑
0
2
2
2
0
(2.62)
So, Eq. (2.60) implies that
pp
pp
µµ
µµ
+=
⇒=−
0
(2.63)
and
αα
µµ
kk
+=0
(2.64)
If a dimension is noncompact for an open string, then
pp
µµ
=
. To simultaneously
satisfy Eq. (2.63), the total momentum of the string must vanish. In the next
example, we consider the case where both endpoints are fi xed.
Open Strings with Fixed Endpoints

48
String Theory Demystifi ed
EXAMPLE 2.4
What is the length of a string that has both endpoints fi xed?
SOLUTION
If both endpoints are fi xed, then we must also satisfy the boundary condition
XX
LR
µµ
τσ π τσ π
(, ) (, )=+ ==0
In this case, we have
CC
Xpe
L
ss
k
ik
k
µµµτπ
τσ π α
(, )
()
== +
−+
≠
∑
2
0
2
2
CC
Xpe
R
ss
k
ik
k
µµµτπ
τσ π α
(, )
()
== +
−−
≠
∑
2
0
2
2
The boundary condition can only be satisfi ed if k is an integer. The overall solution
in this case can be written as
Xx p
n
en
ss
n
n
in
µµ µ
µ
τ
σ
α
σ
=+ −
≠
−
∑
CC
2
0
2sin()
(2.65)
Here we applied the conditions in Eqs. (2.63) and (2.64). This expression includes
the winding term
wp
s
= C
2
µ
(2.66)
Now let’s compute the string coordinates at the endpoints. We have
Xx
Xxw
µµ
µµ
τ
τπ π
(,)
(, )
0 =
=+
Hence, the length of the string is
XXw
µµ
τπ τ π
(, ) (,)−=0
CHAPTER 2 Equations of Motion
49
In going from ordinary classical mechanics to quantum theory, we follow Dirac and
use the correspondence between the Poisson brackets and commutators. In string
theory, we consider equal
τ
Poisson brackets as our starting point when we quantize
the modes on the strings. Later, we will discuss hamiltonians and the Virasoro algebra,
an important concept in string theory, but for now we simply introduce the important
Poisson brackets which will allow us to quantize the string. First we note that
{(,),(,)} ( )XX
T
µν
µν
τσ τσ δσ σ η
′
=−
′
1
(2.67)
or, in terms of momentum
{(,), (,)} ( )PX
µν
µν
τσ τσ δσ σ η
′
=−
′
(2.68)
The Fourier expansion can be used to derive Poisson brackets for the modes. These
are
αα δ η
µν
σµν
mn mn
im,
,
{}
=−
+
(2.69)
The Poisson brackets will be the starting point to quantize the theory.
Quiz
1. Consider the lagrangian given in the Eq. (2.10) which includes the auxiliary
fi eld. Use the Euler-Lagrange equations to derive the equation of motion.
2. Start with the Nambu-Goto lagrangian
L
XX X X=
′
−⋅
′
22 2
()
and consider
the gauge choice which gives the fl at metric
h
α
β
. Using the constraints
XX XX
22
00+
′
=⋅
′
=
,
, fi nd the equations of motion.
3. Consider the Polyakov action. A Weyl transformation is one of the form
heh
αβ
φτσ
αβ
→
(,)
and
δ
µ
X = 0
. Determine the form of the Polyakov action
under a Weyl transformation [hint:
heh
αβ φ τ σ α
β
→
− (,)
].
4. Using the Polyakov action, defi ne the energy-momentum tensor on the
worldsheet by varying the action with respect to the intrinsic metric
T
T
h
S
h
P
αβ
αβ
δ
δ
=−
−
21
Poisson Brackets
50
String Theory Demystifi ed
Using the fact that
δ
δ
αβ
α
β
−=− −hhhh
1
2
fi nd an expression for the
induced metric so that it can be eliminated from the action. This should
allow you to recover the Nambu-Goto action.
5. Consider an open string with free endpoints. Find the variation of the center
of mass position of the string with
τ
by calculating
1
0
π
στσ
π
µ
dX
∫
(, )
6. Find the conserved momentum of the open string with free endpoints by
calculating
PTdX
µ
π
µ
στσ
=
∫
0
(, )
where the dot represents differentiation with respect to
τ
.
The Classical String II:
Symmetries and
Worldsheet Currents
In the last chapter we introduced some basic notions of classical string theory,
including the equations of motion and boundary conditions. In this chapter we
will expand our discussion of the classical string, discussing symmetries and
introducing the energy-momentum tensor and conserved currents. This will fi nish
the groundwork we need for the classical string, and in the next chapter we will
quantize the string.
CHAPTER 3
Copyright © 2009 by The McGraw-Hill Companies, Inc. Click here for terms of use.
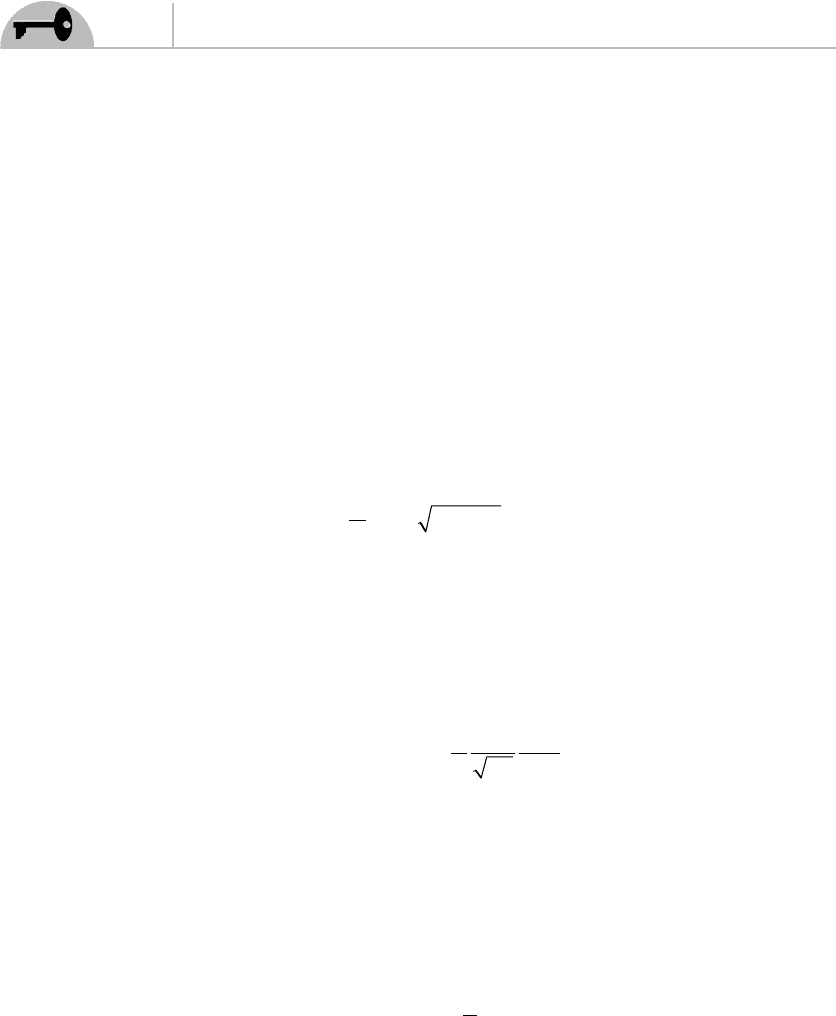
52
String Theory Demystifi ed
The Energy-Momentum Tensor
Let’s quickly review a few things before getting started. Recall that the intrinsic
distance on the worldsheet can be determined using the induced metric
h
αβ
. This is
given by
ds h d d
2
=
αβ
αβ
σσ
(3.1)
where
στσσ
01
==,
are the coordinates which parameterize points on the
worldsheet. A set of functions
X
µ
στ
(, )
describe the shape of the worldsheet and
the motion of the string with respect to the background space-time, where
µ
=−01
1
, , ..., D
for a D-dimensional space-time. To fi nd the dynamics of the
string, we can minimize the Polyakov action [Eq. (2.27)]:
S
T
dhhXX
P
=− − ∂ ∂
∫
2
2
ση
αβ
α
µ
β
ν
µν
det( )
(3.2)
Minimizing
S
P
(by minimizing the area of the worldsheet) gives us the equations
of motion for the
X
µ
στ
(, )
, and hence the dynamics of the string. In the quiz at the
end of Chap. 2 in Prob. 4, you were invited to show that the Polyakov and Nambu-
Goto actions were equivalent by considering the energy-momentum or stress-energy
tensor
T
αβ
which is given by
T
Th
S
h
P
αβ
αβ
δ
δ
=−
−
21
(3.3)
In this book we’ll go mostly by the name energy-momentum tensor. In a nutshell,
the energy-momentum tensor describes the density and fl ux of energy and
momentum in space-time. You should be familiar with the basics of what
T
αβ
is
from some exposure to or study of quantum fi eld theory, so we’re just going to go
with that and describe how it works in string theory. When working out the solution
to Prob. 4 in Chap. 2, you should have found that
TXX hhXX
αβ α
µ
β
ν
µν αβ
ρσ
ρ
µ
σ
ν
µν
ηη
=∂ ∂ − ∂ ∂
()
1
2
(3.4)
The fi rst property that we will establish for the energy-momentum tensor is that it
has zero trace. We can calculate the trace using the induced metric:
Tr T T h T()
αβ
α
α
αβ
αβ
==

CHAPTER 3 Symmetries and Worldsheet Currents
53
The trace is easy to calculate:
hT h X X hh h X X
αβ
αβ
αβ
α
µ
β
ν
µν
αβ
αβ
ρσ
ρ
µ
σ
ν
µ
ηη
=∂∂ − ∂∂
1
2
νν
αβ
α
µ
β
ν
µν
ρσ
ρ
µ
σ
ν
µν
ηη
()
=∂∂ −∂∂hXX hXX (because hh
hXX hXX
αβ
αβ α
α
αβ
α
µ
β
ν
µν
αβ
α
µ
β
ν
δ
η
==
=∂∂ −∂∂
2)
ηηρασβ
µν
(relabel dummy indices, →→
=
,)
0
So we’ve established our fi rst fact to fi le away about the energy-momentum tensor
for the string. It’s traceless:
T
α
α
= 0
(3.5)
In a moment we will learn the physical reason why the energy-momentum tensor is
traceless.
In this section we will list some of the symmetries of the Polyakov action (and
hence of the bosonic string in Minkowski space-time). There are three symmetry
groups of the Polyakov action. These include
• Poincaré transformations
• Reparameterizations of the worldsheet coordinates
• Weyl transformations
The concept of symmetries is so important we will take a momentary digression
to discuss the topic before describing the symmetries of the Polyakov action.
Symmetries in physics can be global symmetries or local symmetries. These are
defi ned as follows:
• A global symmetry is one that holds at all points in space-time. The
parameters of the transformation will not depend on space-time.
• A local symmetry is one that acts differently at different space-time points.
In this case, the parameters of the transformation will be functions of the
space-time coordinates.
You should recall from your studies of classical mechanics and quantum fi eld
theory that a symmetry in physics leads to a conservation law. The formal statement
Symmetries of the Polyakov Action

54
String Theory Demystifi ed
of this fact is called Noether’s theorem. Let’s quickly review the most famous
example of a conserved quantity, the conservation of electric charge.
The electromagnetic fi eld tensor
F
µν
is defi ned in terms of the 4-vector
potential via
FAA
µν µ ν ν µ
=∂ −∂
The Maxwell equations with source terms are written as
∂=
µ
µν ν
FJ
Now it follows from the defi nition of
F
µν
that
∂=
ν
ν
J 0
because
∂=∂∂−∂∂⇒∂∂
=∂∂∂ −∂∂∂
µ
µν
µ
µν
µ
νµ
νµ
µν
νµ
µν
νµ
FAAF
A
ννµ
νµ
µν
µν
νµ
A
AA=∂∂ ∂ −∂ ∂ ∂ (partial derivatives commute)
(relabel dumm=∂∂∂ −∂∂∂
νµ
µν
νµ
µν
AA yy indices)
= 0
Hence
J
µ
is a conserved quantity. This is a fact expressed in the famous continuity
equation which tells us that
∂
∂
+∇⋅ =
ρ
t
J
I
I
0
where
ρ
is the charge density and
I
J
is the current density. It follows that charge is
conserved. The charge Q is of course defi ned using
Qdx=
∫
3
ρ
Using the continuity equation and taking the surface of integration S to be at infi nity
we get
dQ
dt
dx
t
dx J J dA
S
=
∂
∂
=− ∇⋅ =− ⋅ =
∫∫ ∫
33
0
ρ
I
III
x
Hence, charge is conserved.
We demonstrated that charge is conserved starting with the equation of motion
for the electromagnetic fi eld. More formally, we can determine what the conserved

CHAPTER 3 Symmetries and Worldsheet Currents
55
quantities are and relate them to symmetries by looking at the action S, or more
particularly the lagrangian. This is where Noether’s theorem comes into play—
symmetries in the lagrangian lead to conserved quantities.
You can understand Noether’s theorem with a simple one-dimensional example.
Consider a particle whose motion is described by a lagrangian
L
qq
(,
)
where
q
dq
dt
=
The momentum of the particle is given by
p
L
q
=
∂
∂
The Euler-Lagrange equations are the equations of motion for this system:
d
dt
L
q
L
q
∂
∂
−
∂
∂
=
0
Now suppose that the lagrangian is invariant under a symmetry. That is, the form
of the lagrangian does not change under a one parameter coordinate transformation
tst→ (
)
:
qt qs() ()→
Saying that the lagrangian is invariant under this symmetry means that
d
ds
Lqs qs[(), ()]
= 0
The symmetry of the lagrangian can be written out explicitly using the chain rule as
d
ds
Lqs qs
L
q
dq
ds
L
q
dq
ds
[(), ()]
=
∂
∂
+
∂
∂
= 0
Now let’s get to the central idea. Noether’s theorem tells us that
Qp
dq
ds
=
is a conserved quantity, that is,
dQ
dt
= 0
