Mattingly J.D., Heiser W.H., Pratt D.T. Aircraft Engine Design
Подождите немного. Документ загружается.

60 AIRCRAFT ENGINE DESIGN
number and altitude, 8) loiter, 9) warm-up, 10) takeoff rotation, and 11) constant
energy height maneuver.
For this type of flight, the required thrust may not be known in advance. The
thrust is modulated or throttled so that T = (D + R), or u = 1 by Eq. (3.5), and
dZe
= 0 by Eq. (3.4).
Using Eq. (3.3), it follows immediately that
dW___W TSFC(-~)dt
(3.10)
It is often found that the quantity
{TSFC(D + R)/W}
remains relatively unchanged
over the flight leg, and Eq. (3.10) can be integrated to yield
Wfwi = exP l_TsFC(_~__R)At }
(3.11)
where At is the total flight time. If desired, the required thrust may be computed
after the fact. Otherwise, the integration of Eq. (3.10) can be accomplished by
breaking the leg into several smaller intervals.
TSFC behavior.
The engine installed thrust specific fuel consumption that
appears in Eq. (3.3) is a complex function of the combination of the instantaneous
altitude, speed, and throttle setting, especially if the engine has the option of after-
burning. A satisfactory starting point with sufficient accuracy for this stage
of analysis is the approximation that (see Sec. 3.3.2 and Appendix D)
TSFC = (C1 + C2M)~/-O
(3.12)
where C1
and
C 2 are
constants that are known in advance for each type and
operation of turbine engine cycle.
It should be emphasized that approximations for
TSFC
different from Eq. (3.12)
could be made and the weight fraction analysis continued to completion, but our
experience is that this model strikes an excellent balance between accuracy and
ease of solution for turbojet, turbofan, and turboprop engines, with or without
afterbuming.
dW
W
where
Summary of weight fraction equations.
Combining Eqs. (3.6), (3.7), (3.10),
(3.11), and (3.12) as appropriate, the weight fraction analysis results may be sum-
marized as follows:
Type A ( Ps > O, T = ~ TsL
given):
_ (C1/M+C2) ( V 2)
asta(1 ~-~ d h + ~go
(Instantaneously exact) (3.13)
D+R
b[ -- --
T
WI
exp {Exact when (3.14)
[(CI/M +
C2)/(1 - u)] is constant.}
MISSION ANALYSIS 61
Please note that type A weight fraction analysis is independent of altitude.
Type B (Ps = O, T = D +
R):
W - -(C1 + C2M)~/O T dt
(Instantaneously exact)
(3.15)
Wfwi
=exp{-(Cl
+C2M),v/O(~-)At}
[Exactwhen (3.16)
(C1 + C2M)VrO(D + R)/W
is constant.]
Please note that type B weight fraction analysis depends on altitude through 4'0.
A large number and variety of special cases of Eqs. (3.13-3.16) shall now be
developed in order both to illustrate their behavior and to provide more specific
design tools for weight fraction analysis. Close attention should be paid to the
fact that the formulas to follow can be used in two separate ways. First, they are
imbedded in the Mission Analysis subroutines of AEDsys as the basis for the fuel
consumption computations for legs bearing the same titles. Second, they can be
used as they stand to estimate weight fractions or carry out mission analysis by
hand.
In all the example cases that follow, it is assumed that the ot of Eq. (2.3), the fl
of Eq. (2.4), the K1, K2, and
Coo
of Eq. (2.9), and the C1 and C2 of Eq. (3.12) are
known. It may also be helpful to note in the following developments that n = 1
and L = W except for Cases 4, 6 (n > 1), 9, and 10.
3.2.1 Case 1: Constant Speed Climb ( Ps =
dh/dt)
Given:
dV/dt
= 0, n = 1 (L = W), full thrust (T
=
oITsL)
and values
ofhinitial,
hfinal , and V.
Under these conditions
/gm
so that Eq. (3.14) becomes
CD -~- CDR ~ ( WTo ~
c~ ~ \-T-S/
Wf=
exp {
We
cxj ÷c2 [ I/
ast d 1
-- [(CD ~- CDR)/CL](t~/OI)(WTo/TsL)
where the level flight relationships
D+R D+R
W L
have been used, and
(3.17)
q(CD
q- CDR)S CD -~- fOR
- (3.18)
qCLS CL
Ah = h~n~l - hinitial
Appropriate average values of or, fi, O, M, and
(CD +
CDR)/CL
for the altitude
interval should be used, unless the quantity
(C1/M +
C2)/(1 - u) varies too much
for acceptable accuracy. Then the integration is accomplished by breaking the
62 AIRCRAFT ENGINE DESIGN
climb into several smaller intervals and applying Eq. (3.17) to each. The overall
Wf/W~
is then the product of the separate results.
Please note that, as stated earlier, the term [(CD +
CDR)/CL](/3/ot) (Wro/TsL)
represents the fraction of the engine thrust work that is dissipated, while
{1 - [(CD +
CDIO/CL](/3/~)(Wro/TsD}
is the fraction invested in potential en-
ergy. Low drag and high thrust are obviously conducive to efficient energy conver-
sion, a theme that recurs in all type A flight. This highlights one path to efficient
acceleration and/or climb.
3.2.2 Case 2: Horizontal Acceleration (Ps = Vd V/go
dt)
Given:
dh/dt
= O, n = 1 (L = W), full thrust (T =
otTsL)
and values of h,
Vinitial,
and
Vfinal.
Under these conditions
so that Eq. (3.14) becomes
CD ~- CDR /3 ( WTo ~
Wi ast d 1 -- [(C D -[- CDR)/CL](fl/Ol)(WTo/TsL)J
(3.19)
where Eq. (3.18) has been used, and
A V 2 V~na I 2
= -- ginitia I
Appropriate average values of or,/3, 0, V, and
(CD + Czm)/CL
for the speed
interval should be used, unless the quantity
(C1/M +
C2)/(1 - u) varies too much
for acceptable accuracy. Then the integration is accomplished by breaking the
acceleration into several smaller intervals and applying Eq. (3.19) to each. The
overall
Wf/Wi
is then the product of the separate results.
Please note that, as stated earlier, the term
(CD/CL)(/3/Ot) (Wvo/TsD
represents
the fraction of the engine thrust work that is dissipated, while {1-
(CD/CL)(/3/oO
× (Wro/TsD}
is the fraction invested in kinetic energy. Low drag and high thrust
are again the ingredients of efficient energy conversion.
3.2.3 Case 3: Climb and Acceleration ( Ps = dh/dt + Vd V/go
dt)
Given: n = 1, (L = W), full thrust (T =
otTsL),
and values of
hinitial, hfinal ,
and
Wfinal .
This solution combines those of Cases 1 and 2, with the same qualifying remarks.
The result is
W~ =exp{ (C]/M
JvC2)[, A(h +___ V2/2go)
]/
Wi astd 1 -- [(CD + CDR)/CL](fl/ot)(Wro/TsL) J ]
(3.20)
Special care must be taken in the application of Eq. (3.20) because h and V are
truly independent of each other until the flight trajectory is chosen. The Ps diagram

MISSION ANALYSIS 63
(e.g., Fig. 2.E5) can provide excellent guidance in the selection of the h vs V
path, especially if a maximum Ps trajectory is desired. Also, as already noted,
the term
[(Co + COR)/CL](~/Ot)(Wro/TSL)
represents the fraction of the engine
thrust work that is dissipated, and {1 - [(Co +
CDn)/CL](~/oO(Wro/Tsl.)}
is the
fraction that is invested in mechanical energy (i.e., potential plus kinetic) or energy
height.
3.2.4 Case 4: Takeoff Acceleration (Ps = Vd V/go
dt)
Given:
dh/dt
= 0, full thrust (T =
aTsi~)
and values of
P, D, CLmax, kro,
and R.
Under these conditions Eq. (3.14) becomes
/ I
-- go \~/
where u can be obtained from Eq. (2.21) as
{ (q)( S ) }fl(WTo~
u = Cro ~ Wro +/Zro -or \-~-sL ] (3.22)
and
Fro
is given by Eq. (2.20) and M is an average value during takeoff.
3.2.5 Case 5: Constant Altitude~Speed Cruise (Ps = O)
Given:
dh/dt
= 0,
dV/dt
= 0, n = 1 (L = W), and values of h, V, and cruise
range As.
Under these conditions, and replacing At with
As~V,
Eqs. (3.16) and (3.18)
become
Wf
exp{
(C1/M+C2)(CD+CDR~Asl
-~i = a,ta
C-[ ,] l (3.23)
where CL is obtained by setting lift equal to weight and
Co
is computed from
Eq. (2.9). Because
(Co + CoR)/CL
will vary as fuel is consumed and the weight
of the aircraft decreases, this result is not, strictly speaking, exact. Hence, it might
become advisable to break the cruise into several intervals and apply Eq. (3.23) to
each. The type B Cases 6-10 that follow are, however, exact integrals.
Equation (3.23) and the variants that follow in Case 7 are close relatives of
the familiar Breguet range formula) The reciprocal of the collection of terms
preceding the range As in the exponent is customarily referred to as the range
factor (RF). Thus, for a given weight fraction, the range is directly proportional
to the
RF.
The endurance factor (EF) of Case 8 belongs to the same family and
enjoys qualities quite similar to those of the
RF.
3.2.6 Case 6: Constant Altitude~Speed Turn ( Ps = O)
Given:
dh/dt
= 0,
dV/dt
= 0, and values of h, V, n >1, and number of
turns N.
64 AIRCRAFT ENGINE DESIGN
This situation can be treated much the same as Case 5, except that L = n W.
The duration of the turning can be shown, with the help of Eq. (2.17), to equal
2Jr Rc N 27r N V
- (3.24)
At -- V g0~/-~ " - 1
Under these conditions Eqs. (3.16) and (3.24) become
go n 2~"-nff-Z-]-- 1 ]
where CL is obtained from the expression
q\S]
and CD is computed from Eq. (2.9). Because the duration of the turn is usually a
small fraction of the mission, the values in Eq. (3.25) may be regarded as constant.
3.2.7 Case 7: Best Subsonic Cruise Mach Number and Altitude
(BCM/BCA) (Ps = O)
Given: dh/dt = O, dV/dt = 0, n = 1 (L = W), and the value of cruise range
AS.
Subsonic cruise is usually the most important portion of any mission because
it uses the largest amount of the onboard fuel. Hence, the analysis must be as
accurate as possible and the results applied to minimize fuel usage. Under these
conditions, and replacing dt with ds/V, Eq. (3.15) becomes
dW (C1/M +
C2) (C
D -~- CDR
W = astd , -C-L Ids (3.26)
where the level flight relationship Eq. (3.18) has been used.
From Eq. (3.26), it is obvious that aircraft weight reduction and fuel consump-
tion are minimized by operating at the lowest possible value of (C1/M + C2) ×
[(Co + CoR)/CL]. This is known as the "best" cruise condition and the indi-
vidual parameters associated with it are referred to as CL*, Co*, and M*. Finding
them for a given aircraft is not difficult, as the following will demonstrate for one
of the common type represented by Fig. 2.Ela.
First, note that below the critical drag rise Mach number Mcnff, neither CL nor
Co depend on M. In this range,
CD "~- CDR KIC~ -4- K2CL -1- CDO -f- fOR
CL CL
A minimum for (CD +
CDR)/CL
can
be found by differentiating the preceding
equation with respect to CL and setting the result equal to zero. This leads to
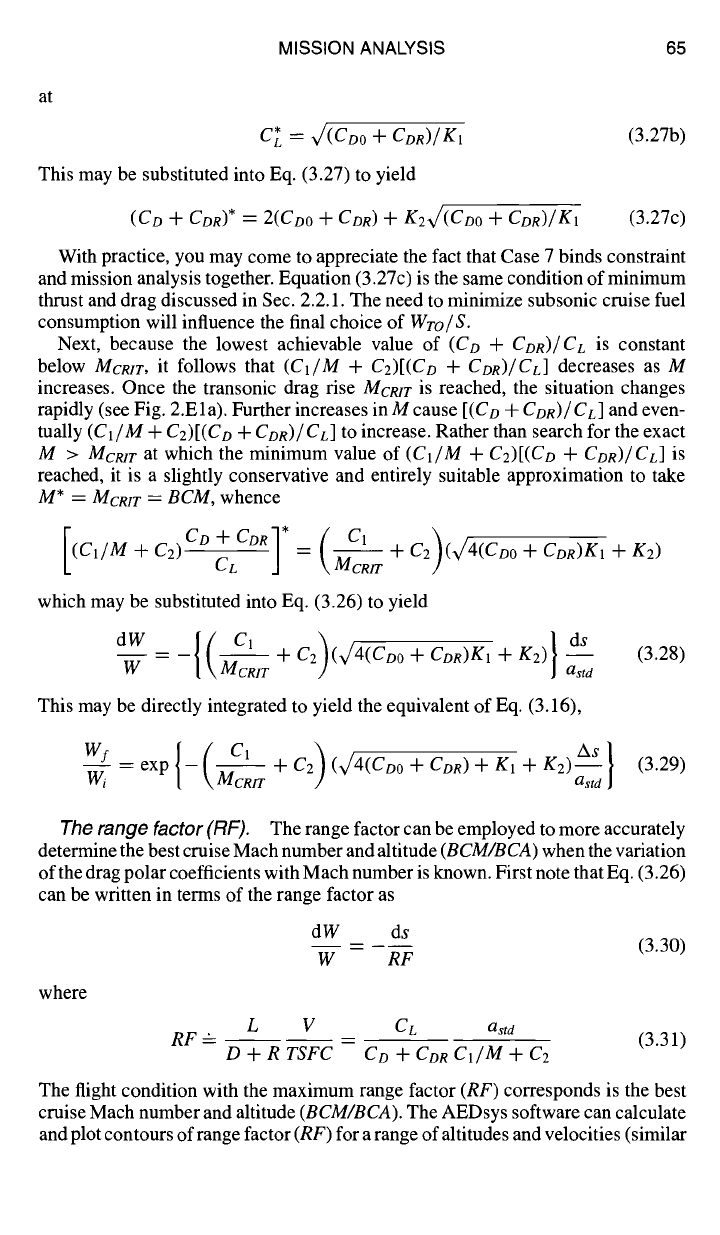
CD CDR ~* ~ "~ CDR)KI q- K2
(3.27a)
+
MISSION ANALYSIS 65
at
C* L = ~/(CDo + CDR)/K1
(3.27b)
This may be substituted into Eq. (3.27) to yield
(CD ÷ CDR)* = 2(CD0 ÷ CDR) + K2~/(CDo + CDR)/KI
(3.27c)
With practice, you may come to appreciate the fact that Case 7 binds constraint
and mission analysis together. Equation (3.27c) is the same condition of minimum
thrust and drag discussed in Sec. 2.2.1. The need to minimize subsonic cruise fuel
consumption will influence the final choice of
Wro/S.
Next, because the lowest achievable value of
(CD + CDR)/CL
is constant
below MCRIT,
it follows that
(CI/M + C2)[(CD ÷ CDR)/CL]
decreases as M
increases. Once the transonic drag rise
MCRIT
is reached, the situation changes
rapidly (see Fig. 2.E 1 a). Further increases in M cause
[(CD + CoR)/CL]
and even-
tually
(C1/M + C2)[(CD + CDR)/CL] to
increase. Rather than search for the exact
M > Mcmr
at which the minimum value of
(C1/M + C2)[(CD + CDR)/CL]
is
reached, it is a slightly conservative and entirely suitable approximation to take
M* = MCRlr = BCM,
whence
÷CDRI*= (M@RIT÷C2)(~/4(CDo÷CDR)KI÷K2)
[
(C~/M +
c2)C° c[ J
which may be substituted into Eq. (3.26) to yield
I / as
W ~ - + C2 G/4(Co0 +
ColdKl +
K2) (3.28)
astd
This may be directly integrated to yield the equivalent of Eq. (3.16),
I + /
C2
(x/4(CDo
CDR) ÷ gl ÷ K2) AS
(3.29)
astd
The range factor (RF).
The range factor can be employed to more accurately
determine the best cruise Mach number and altitude
(BCM/BCA)
when the variation
of the drag polar coefficients with Mach number is known. First note that Eq. (3.26)
can be written in terms of the range factor as
where
dW ds
- (3.30)
W RF
L V CL astd
RE "-- -- -- --
(3.31)
D + R TSFC Co + Cole C1/M + C2
The flight condition with the maximum range factor
(RF)
corresponds is the best
cruise Mach number and altitude
(BCM/BCA).
The AEDsys software can calculate
and plot contours of range factor
(RF)
for a range of altitudes and velocities (similar
66 AIRCRAFT ENGINE DESIGN
to the Ps contours of Fig. 2.E5), making it easy for the user to determine the best
cruise conditions. For cruises where the range factor
(RF)
is essentially constant,
Eq. (3.30) can be integrated to obtain
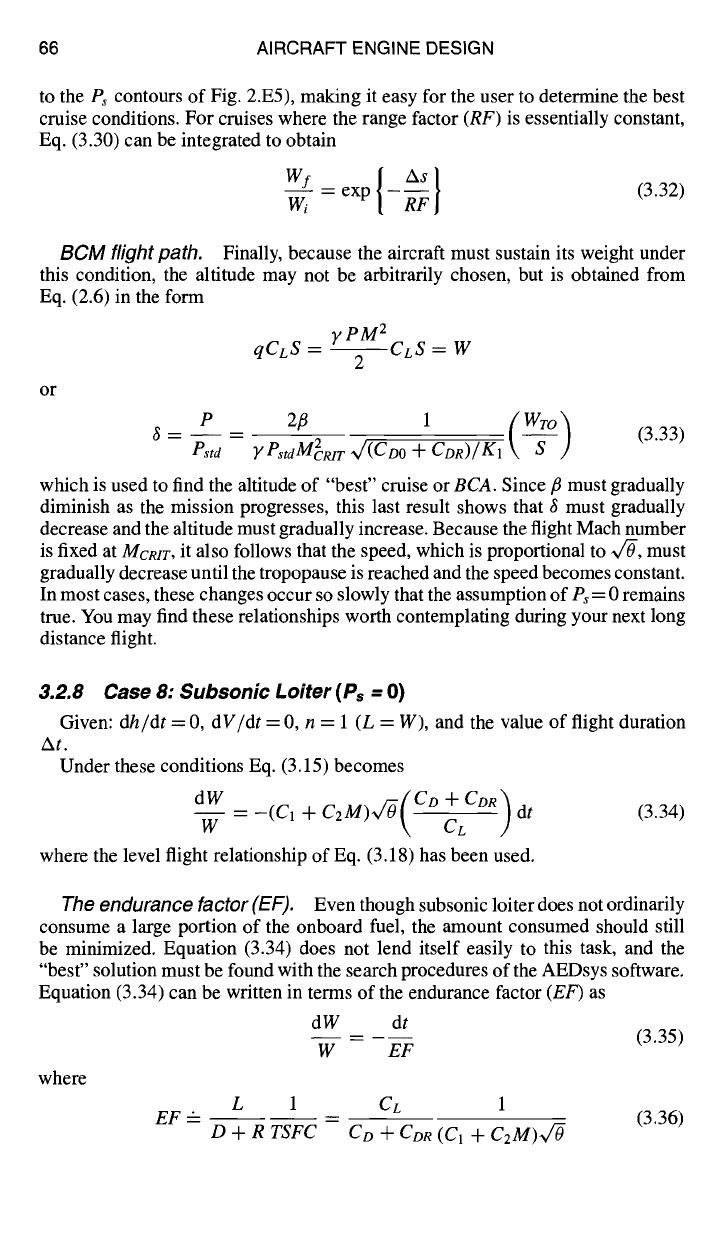
exp{ "s /
~- = -~-~ (3.32)
BCM flight path.
Finally, because the aircraft must sustain its weight under
this condition, the altitude may not be arbitrarily chosen, but is obtained from
Eq. (2.6) in the form
yPM 2
qCLS - --CLS : W
2
or
1
-- Ps~d -- Y PstdM2RIT ~/(CDo Jc CDR)/K1 --
(3.33)
which is used to find the altitude of "best" cruise or
BCA.
Since/3 must gradually
diminish as the mission progresses, this last result shows that 3 must gradually
decrease and the altitude must gradually increase. Because the flight Mach number
is fixed at
MCRIT,
it also follows that the speed, which is proportional to ~, must
gradually decrease until the tropopause is reached and the speed becomes constant.
In most cases, these changes occur so slowly that the assumption of
Ps
= 0 remains
true. You may find these relationships worth contemplating during your next long
distance flight.
3.2.8 Case 8: Subsonic Loiter (Ps = O)
Given:
dh/dt = O, dV/dt = O, n = 1 (L = W),
and the value of flight duration
At.
Under these conditions Eq. (3.15) becomes
w (c~ +
c~
CD
+
-- - ~-~ / dt (3.34)
where the level flight relationship of Eq. (3.18) has been used.
The endurance factor (EF).
Even though subsonic loiter does not ordinarily
consume a large portion of the onboard fuel, the amount consumed should still
be minimized. Equation (3.34) does not lend itself easily to this task, and the
"best" solution must be found with the search procedures of the AEDsys software.
Equation (3.34) can be written in terms of the endurance factor (EF) as
dW dt
- (3.35)
W EF
where
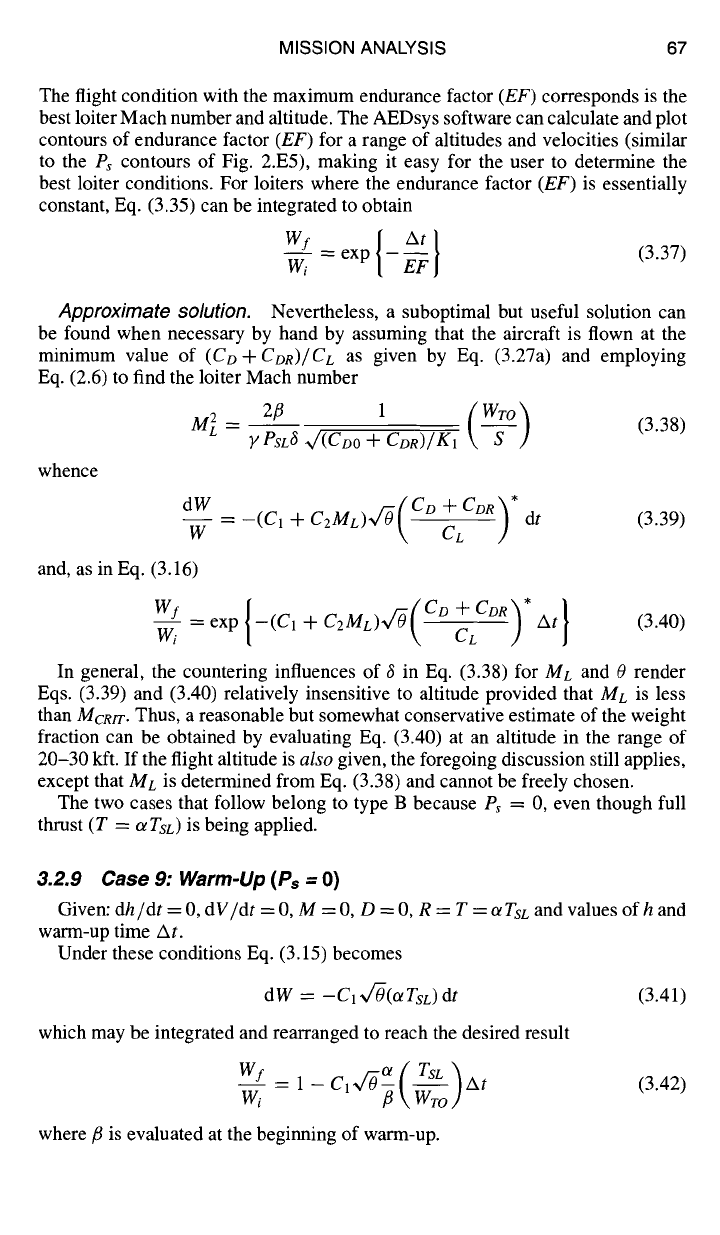
L 1 CL 1
EF -- D +~ TSF~ -- CD + CDR
(C 1 -q-
C2M)~q/-O
(3.36)
MISSION ANALYSIS 67
The flight condition with the maximum endurance factor
(EF)
corresponds is the
best loiter Mach number and altitude. The AEDsys software can calculate and plot
contours of endurance factor
(EF)
for a range of altitudes and velocities (similar
to the
Ps
contours of Fig. 2.E5), making it easy for the user to determine the
best loiter conditions. For loiters where the endurance factor
(EF)
is essentially
constant, Eq. (3.35) can be integrated to obtain
exp{
Wi
- ~-~ (3.37)
Approximate solution.
Nevertheless, a suboptimal but useful solution can
be found when necessary by hand by assuming that the aircraft is flown at the
minimum value of
(Co + CoR)/CL
as given by Eq. (3.27a) and employing
Eq. (2.6) to find the loiter Mach number
2fl
1 (~o)
M~ -- Y PSL~--~ ~/(CDo + CDR)/K1 --
(3.38)
whence
dW
W
and, as in Eq. (3.16)
(el +
C2ML)x/O( CD -~ + CDR)*,] dt
(3.39)
{ (
)'1
Wf
= exp -(C1 +
C2ML)v/O Co + CoR At
(3.40)
W; C£
In general, the countering influences of ~ in Eq. (3.38) for
My
and 0 render
Eqs. (3.39) and (3.40) relatively insensitive to altitude provided that ML is less
than
MCRIT.
Thus, a reasonable but somewhat conservative estimate of the weight
fraction can be obtained by evaluating Eq. (3.40) at an altitude in the range of
20-30 kft. If the flight altitude is
also
given, the foregoing discussion still applies,
except that ML is determined from Eq. (3.38) and cannot be freely chosen.
The two cases that follow belong to type B because Ps = 0, even though full
thrust (T
=
ctTsL)
is being applied.
3.2.9 Case 9: Warm-Up (Ps = O)
Given:
dh/dt
= 0,
dV/dt
= 0, M = 0, D = 0, R = T =
ctTsL
and values ofh and
warm-up time At.
Under these conditions Eq. (3.15) becomes
dW = --C1 v/-O(c~ TsL) dt
(3.41)
which may be integrated and rearranged to reach the desired result
Wf = I--CI~/ro%( TSL ~At
w;
where fl is evaluated at the beginning of warm-up.
(3.42)

68 AIRCRAFT ENGINE DESIGN
3.2.10 Case 10: Takeoff Rotation (Ps = O)
Given:
dh/dt
=0,
dV/dt
=0,
(D + R) = T =otTsc
and values of h,
Mro, and
rotation time
tR.
Under these conditions Eq. (3.15) becomes
dW = -(C1 +
c2gro)v/O(~TsL) dt
(3.43)
which may be integrated and rearranged to reach the desired result
Wf =
-(Cl
+ C2Mro)V/-O~( ~-~LTo)tR
(3.44)
where/~ is evaluated at the beginning of rotation.
3.2.11 Case 11: Constant Energy Height Maneuver(Ps =
O)
Given:
AZ e =
0, n = 1 (L = W), and the values
ofhinitial, hfinal, Vinitial,
and
Vfinal.
This special case corresponds to the situation in which the potential energy is
being exchanged in a climb or a dive for an essentially equal amount of kinetic
energy. The required thrust work of the engine is only that necessary to balance
the energy dissipated by the aerodynamic drag along the path. Because
Wf
is not
much different from
Wi
for such flight, it is not necessary to perform an elaborate
evaluation of relevant parameters along the complete trajectory. Instead, a common
sense estimate of the average values of M, 0, and
(Co + CDR)/CL
will suffice.
Under these conditions Eqs. (3.16) and (3.18) become
W fwi
= exp {-(C1
+CzM)~(CD+C°R~Atlc-L
,/ | (3.45)
where
CL
is obtained by setting lift equal to weight and
CD
is computed from
Eq. (2.9). All terms in Eq. (3.45) are calculated at the average altitude
(havg)
and initial energy height
(Zei).
The associated velocity is designated as
Vmia
and
calculated from the kinetic energy at the average energy state using
Vmid = v/2go(Zei
--
havg)
The maneuver time interval At is best obtained by recognizing that the maneuver
vertical distance is
hfinal
- hinitial
and assuming that the vertical speed is some
fraction of the midspeed (Vmid).
3,2.12
General Determination of
I/~ITo
Equation (3.1) is the most fruitful starting point for evaluating
WTO,
as well as
for understanding the factors that control
Wro.
Regarding the latter, it is useful
and illuminating to rewrite Eq. (3.1) in the form
WTo = Wp/(1 wToWF ~TO)
(3.1a)
This relationship reveals that
Wro
is directly proportional to the
We
(as required
by the customer), and inversely proportional to the difference between one and the
MISSION ANALYSIS 69
fuel weight fraction (based on aerodynamic and propulsion technologies) and the
structural weight fraction (based on material and structural technologies).
This is a scenario rich with heroes and villains, real and imaginary. To increase
your appreciation of the stakes, consider the typical situation where the fuel and
structural weight fractions add up to about 0.9, and Wro is therefore about 10 Wp.
On the one hand, if the fuel plus structural weight fraction turned out to be only
5% higher than expected, Wro would be more than 18 Wp. On the other hand, if
the fuel plus structural weight fraction could be made 5% lower than expected,
Wro would be less than 7 Wp. Thus, this highly nonlinear equation magnifies both
the cost of failure and the reward of technological advance.
This relationship also explains the attraction of novel aircraft concepts. The
unpiloted air vehicle (UAV), for example, has less payload (e.g., no pilot plus
equipment) and a smaller structural weight fraction (e.g., no cockpit, canopy, or
environmental and protective gear) than its piloted counterpart. The result is a
dramatically smaller, lighter, and less expensive vehicle for the same mission.
The Mission Analysis subroutine of AEDsys allows Wro to be determined by
an iterative process, as follows. For the given mission profile, Wp, an initial esti-
mate of Wro, and the corresponding allowable We/Wro (e.g., Figs. 3.1 and 3.2
or Sec. 3.3.1), the vehicle is flown from takeoff to landing. Any remaining pay-
load or reserve fuel is then removed in order to determine the remaining weight,
which equals We, and allows the actual WE/Wro to be calculated. If the latter
value exceeds the allowable We/Wro, a higher value of Wro must be selected
and the process repeated, and vice versa. This process continues until a satis-
factory level of convergence is reached. Since We/Wro is weakly dependent
upon Wro (see Sec. 3.3.1), unexpectedly large changes of Wro are generally re-
quired to correct for relatively small differences in WE/Wro. This is, of course,
very good news when the latter is smaller than allowable, but very bad news
otherwise.
3.2.13
Algebraic Calculation of
WTO
Equation (3.1) may also be used to calculate the takeoff weight algebraically
(i.e., by hand). It will be convenient in this derivation to use the terminology
Wf = 1-I -< 1 (3.46)
Wi if
for the weight ratio of mission legs where only fuel is consumed. For example,
if the consecutive junctions of the mission are labeled with sequential cardinal
numbers, such as those of the AAF RFP in Chapter 1, it follows that
__ W5
w, - w3 × _ = lq i-i i-i = i- I
W2 W2 W3 W4 23 34 45 25
For the special case where the expendable payload is delivered at some point
"j" in the mission, the terminology Wf/Wi or IF] is not used. Write instead
W~ - Wpe Wee
- 1 - -- (3.47)
w: wj
