Massoud M. Engineering Thermofluids: Thermodynamics, Fluid Mechanics, and Heat Transfer
Подождите немного. Документ загружается.


542 IVb. Heat Transfer: Forced Convection
Equation IVb.2.1 and obtain a relation for V
y
. [Hint: Use the equation for conti-
nuity given by Equation IIIa.3.13-1].
4. Find the Pr number of a fluid having v = 0.001 ft
2
/s, k = 0.08 Btu/ft h F, c
p
=
0.45 Btu/lbm
⋅F, and
ρ
= 58 lbm/ft
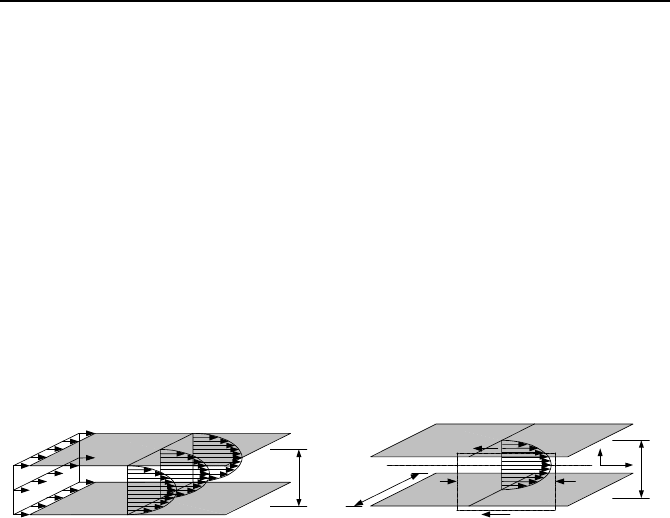
3
. [Ans.: 1175].
5. Consider a flat plate 15.3 cm long. Find the free stream velocity, V
f
so that the
flow regime remains laminar for such fluids as water, air, and helium at 1 atm and
20 C. [Ans.: 3.3, 51, and 387 m/s].
6. Air at atmospheric pressure and room temperature (27 C) flows over a flat plate
at a speed of 2 m/s. The length of the plate is 0.5 m. Find the thickness of the
boundary layer at the middle of the plate (x = 25 cm) and at the trailing edge (x =
L). [Ans. 7 mm and 1 cm].
7. A flat plate has a length of 40 cm and width of 1 m. Air, at pressure of 2 atm,
temperature of 57 C, and velocity of 3 m/s flows over the plate. Find the thickness
of the boundary layer at x = L.
8. Use the result of Problem 3 and find the y-component of velocity over the plate
of Problem 6 at the outer edge of the boundary layer for two locations; a) the mid-
dle of the plate and b) the trailing edge of the plate.
9. Solve Problem 3 using the velocity profile of Problem 2. Use the result and
find the y-component of velocity over the plate of Problem 6. Calculate numerical
values for V
y
at the outer edge of the boundary layer for two locations; a) the mid-
dle of the plate and b) the trailing edge of the plate.
10. Plot the thickness of the boundary condition as a function of the length of a
flat plate (
δ
= Cx
0.5
), starting from the leading and ending at the trailing edge. For
this purpose consider the flow of air at a pressure of 2 atm and temperature of 27
C over the flat plate. Find
δ
at x = 0.45 m.
11. Start with Equation IVb.2.5 and obtain Equation IVb.2.6. [Hint: By assum-
ing constant pressure throughout the flow, the left hand side is simplified as dP/dx
= 0. To simplify the right hand side, first substitute for the last term from the inte-
gration-by-part technique. Now write the Bernoulli equation and conclude that
d(V
x
)/dx is also zero.]
12. Start with Equation IVb.2.8 and obtain Equation IVb.2.9. For this purpose,
first ignore the non-linear term compared with the two dominant terms. Then sub-
stitute for the velocity and temperature profiles. To develop the integral, consider
a case where the hydrodynamic boundary layer is thicker than the thermal bound-
ary layer (thus the integral is zero for y >
δ’). Arrange the result in terms of ȗ =
δ’/δ and ignore ȗ
4
.
13. Air at a pressure of 1 atm and temperature of 30 C flows over a flat plate. The
plate has a length of 120 cm, a width of 200 cm and a temperature of 10 C.
Questions and Problems 543
14. To derive an analytical solution for the thickness of the thermal boundary
layer, Equation IVb.2.9, it was qualitatively argued that the net viscous work term
is generally negligible compared with the more dominant terms. The goal of this
problem is to quantify the above argument for fluids having low, medium, and
high Prandtl numbers. Assume constant fluid properties and equal hydrodynamic
and thermal boundary layer thicknesses to determine cases that the above ap-
proximation is valid. [Hint: Define a figure of merit being the ratio of the viscous
dissipation to the surface conduction. Use an order of magnitude analysis to relate
this ratio to the Prandtl number multiplied by the Eckert number].
15. Equation IVb.2.9 was derived by using the cubic-parabola profiles for veloc-
ity and temperature as given in Equations IVb.2.1 and IVb.2.2. Use the same cu-
bic-parabola profile for temperature but a linear velocity profile, as for Problem 2,
and obtain the Nu number in terms of he Re and Pr numbers.
16. Consider the flow of a fluid between two large parallel flat plates located at a
distance of 2
∆ apart.
2∆
2∆
x
y
b
Find the governing momentum equation for the flow between the plates for the
condition that the velocity profile is fully developed. [Hint: In this case, the net
momentum flux is zero].
17. Use the momentum equation of Problem 16 and obtain the velocity profile in
terms of y,
∆, and the maximum velocity at y = 0. [Ans.: V
x
= (1 – y
2
/∆
2
)/(V
x
)
0
].
18. Find
ξ
= δ’/δ for the fluids of Table IVb.1.1 at P = 1 at and T = 20 C.. [Ans.:
ξ
Water
= 0.5].
19. Consider a heat exchanger made of sheets of parallel flat plates. Water at 25
C, 1 atm, and 4 m/s flows over a plate. Find the water flow rate in the boundary
layer of one plate at 10 cm from the leading edge.
20. Air is flowing at 1atm, 130 C, and 20 m/s over a flat plate. Find the thickness
of the boundary layer at 5 cm from the leading edge and the air flow rate in the
boundary layer at this location.
21. A heated flat plate is exposed to the colder air flowing over the plate at a ve-
locity of 35 ft/s. The plate is at a temperature of 200 F while air is at atmospheric
pressure and 70 F. Find the rate of heat transfer if the plate is a) heated at the
leading edge and b) at a distance of 3 in from the leading edge.

544 IVb. Heat Transfer: Forced Convection
22. Two parallel flat plates are placed 2 cm apart. Water, at 20 C, flows between
these plates at a velocity of 1.6 m/s. Find: a) if the flow becomes fully developed
and b) the distance from the leading edge if it does.
23. A flat plate having a length of 75 cm and width of 50 cm is maintained at
85 C. Cold air at 10 kPa and 30 C flows over the plate at 10 m/s. Find the total
rate of heat loss from the plate.
24. Water at 80 F is flowing over a flat plate with a velocity of 8 ft/s. The plate is
5 ft long. Find and plot the heat transfer coefficient as a function of the plate
length.
25. Air at a temperature of 95 F and a velocity of 20 ft/s flows over a hot flat
plate, maintained at 482 F. Find the rate of heat transfer form this 1ft by 1 ft
square plate. [Ans. ~ 423 Btu/hr].
26. In Problem 25, find the distance from the surface of the plate where flow ve-
locity becomes 75% of the free stream velocity at x = L/2.
27. A plate is heated over its entire length and maintained at 140 F. Air at atmos-
pheric pressure and 80 F is flowing over the plate at velocity of 6.6 ft/s. Find the
heat transfer coefficient and the rate of heat transfer from at a distance of 1.6 ft
from the leading edge. The depth of plate is 1 ft. [Ans. ~392 Btu/hr].
28. For flow over a flat plate, the average temperature difference (i.e.,
fs
TT − )
when the plate temperature is kept constant is readily calculated. This is not the
case if a constant heat flux to or from the surface is imposed. Use the averaging
scheme over the plate length as given by:
()
³
³
L
L
fs
fs
dx
dxTT
TT
0
0
−
=−
to find the average temperature difference. [Hint: Substitute for ∆T in the nu-
merator from the Nu number and for Nu number from Equation IVb.2.11 and in-
tegrate].
29. Obtain the average temperature difference for the laminar flow of fluids over
a heated plate (L × b), for constant heat flux boundary condition, in terms of the
Re and the Pr numbers. For this purpose, use the result of Problem 28 and the lo-
cal Nu
x
number for constant heat flux boundary condition given as:
3/12/1
PrRe453.0Nu
xx
= ( constant=
′′
s
q
)
where in this relation properties are developed at the film temperature.
[Ans.:
)/(PrRe47.1
3/12/1
kLqTT
sLfs
′′
=−
−−
].

Questions and Problems 545
30. A heated flat plate is exposed to the flow of cold air. Find h
x
at x = L/2 and
the total rate of heat transfer for two types of boundary conditions: a) isothermal
plate and b) constant heat flux. Data: plate dimensions are 50 cm × 50 cm, T
air
=
10 C, P
air
= 0.5 atm, V
air
= 4 m/s,
plate
T = 100 C. [Ans.: 157 W, 70 W].
31. A uniformly heated square plate of 2 ft × 2 ft is exposed to cold air at 80 F.
The heater output remains at a constant value of 1 kW. Air, at atmospheric pres-
sure, flows over the plate at a velocity of 16.5 ft/s. Find the average temperature
of this plate. [Ans.: ~ 513 F].
32. A flat square plate is uniformly heated over its entire surface. One side of the
plate is insulated and the other is exposed to cold air flowing over the plate. Find
the plate average temperature. Data: L = 35 cm, T
air
= 20 C, V
air
= 5 m/s, P
air
= 1
atm,
q
′′
= 900 W/m
2
. [Ans.: 80 F].
33. Water at 25 C flows in a smooth tube, having a diameter of 5 cm, at a rate of 1
kg/s. The tube in unheated and the temperature difference between the tube wall
and the water is negligible. Determine the flow regime.
34. Show that the mass flow rate of water in a pipe or tube of diameter D is given
by
m
=
π
D
µ
Re/4.
35. Water enters a heated tube of 2 mm diameter at 30 C and leaves the tube at
60 C. Find the water mass flow for the average Reynolds number of 1000. [Ans.:
0.00564 kg/s].
36. Air at 10 atm and 77 C flows in a duct of rectangular cross section, 20 cm by
10 cm, at a rate of 0.001 kg/s. Find a) the flow regime and b) the heat transfer co-
efficient. The duct is also at 77 C.
37. We want to establish fully turbulent flow in a smooth circular tube carrying
water at a velocity of 5.75 ft/s. Find the maximum tube diameter that still ensures
the flow is fully turbulent. What size tube should be used if air instead of water is
flowing in the tube? Both fluids are at 1 atm and 68 F. What conclusion you
draw from your solution?
38. Water is flowing at a rate of
m
in a smooth pipe of diameter D. A section of
this pipe having a length of L is heated. Water at the inlet to the heated section
has a temperature of T
f1
. Water temperature at the exit of the heated section is T
f2
.
The heated section of the pipe wall is maintained at T
s
. Show that for turbulent
flow in the pipe and a specified pipe diameter, the required heated length is given
by:
()
¸
¸
¹
·
¨
¨
©
§
∆
∆
¸
¸
¹
·
¨
¨
©
§
=
s
f
T
T
mDL
2.08.0
2.0
6.0
Pr
407.11
µ
546 IVb. Heat Transfer: Forced Convection
where ∆T
f
= T
f2
– T
f1
, ∆T
s
= T
s
–
f
T , and
f
T = (T
f1
+ T
f2
)/2. [Hint: Use Newton’s
law of cooling in conjunction with Equations IVb.2.20 and IVb.3.4].
39. Water at a rate of 5 kg/s is flowing inside a heated tube. The tube has a di-
ameter of 6 cm and its wall is maintained at 85 C. Find the required tube length so
that the water can be heated from 10 to 20 C.
40. Water is flowing at a rate of
m
in a smooth pipe of diameter D. A section of
this pipe having a length of L is heated. Water at the inlet to the heated section
has a temperature of T
f1
. Water temperature at the exit of the the heated section is
T
f2
. The heated section of the pipe wall is maintained at T
s
. Show that for turbu-
lent flow in the pipe and a specified heated length, the pipe diameter is given by:
25.1
4.0
6.02.0
0477.0
»
»
¼
º
«
«
¬
ª
¸
¸
¹
·
¨
¨
©
§
∆
∆
¸
¸
¹
·
¨
¨
©
§
=
f
s
p
T
T
k
cm
L
D
µ
41. Water flows in a heated round tube at a rate of 3 kg/s. The heated section of
the tube is 4 m long. The tube wall is maintained at 90 C. Water enters the tube at
35 C and leaves the heated section at 45 C. Find the tube diameter. [Ans.: 10.3
cm].
42. Water is flowing in a smooth pipe of diameter D. A section of this pipe hav-
ing a length of L is heated. Water at the inlet to the heated section has a tempera-
ture of T
f1
. Water temperature at the exit of the heated section is T
f2
. The heated
section of the pipe wall is maintained at T
s
. Show that for turbulent flow in the
pipe and a specified heated length and pipe diameter, pressure drop in the heated
section is given by:
9.0
54.038.0
52.5
9.1
Pr
0159.0
¸
¸
¹
·
¨
¨
©
§
∆
∆
¸
¸
¹
·
¨
¨
©
§
¸
¸
¹
·
¨
¨
©
§
=∆
f
s
T
T
D
L
P
ρ
µ
[Hint: Use Newton’s law of cooling in conjunction with Equations IIIb.3.6,
IIIb.3.7, and IVb.2.20].
43. As described in Chapter VIb, one way to measure the mass flow rate is to use
a heated duct. Consider the flow of air in a duct. To measure the air flow rate, we
first raise the temperature of a segment of this duct and maintain the wall tempera-
ture at a desired value. We then measure the air pressure and temperature at the
inlet and exit of the heated segment. Use this technique and the given data to find
the air flow rate in the duct. Data: Air pressure: 17 psia, air temperature at the
inlet: 65 F, air temperature at the exit: 125 F, duct wall temperature 230 F, duct
cross section is 1.5 ft by 1 ft, and the length of the heated segment is: 8 ft.
44. Consider a small double pipe heat exchanger. Hot air flows in the inner and
cold water in the outer pipe. The heat exchanger is well insulated. The inner pipe

Questions and Problems 547
is thin and made of copper tubing. Use the given data to find the required heat ex-
changer length. Data: Inner pipe diameter: 3 cm, wall thickness: 2 mm, outer
pipe diameter: 6 cm, P
air
= 1 atm,
air
m
= 1 kg/s,
water
m
= 2 kg/s, total rate of
heat transfer: 20 kW, average air temperature: T
air
= 450 C, average water tem-
perature: T
water
= 160 C, water pressure 5 atm.
45. Water flows in a heated tube. The constant heat flux of 2 MW/m
2
is applied
to the tube wall. Water enters the tube at a rate of 1 kg/s and an inlet temperature
of 35 C. Find the water exit temperature. The tube inside diameter is 2 cm and
the tube length is 1 m. [Ans.: 65 C].
46. Consider the flow of water in a heat flux controlled channel. The channel
length and diameter are D and L, respectively. The heat flux at the wall varies
linearly along the channel. Show that the water temperature at the exit of the
channel is given by:
()
2
L
qq
cm
D
TT
exitinlet
p
inletexit
′′
+
′′
+=
π
47. Consider the flow of water in a heat flux controlled channel. The wall heat
flux varies linearly. Find the water temperature at the exit of the channel. Data:
D = 3 cm, L = 1.5 m,
water
m
= 1 kg/s,
inlet
q
′′
= 1 MW/m
2
,
outlet
q
′′
= 2 MW/m
2
, T
in
= 30 C. [Ans.: 81 C].
48. Water is flowing in a smooth pipe of diameter D. A section of this pipe hav-
ing a length of L is heated. Water at the inlet to the heated section has a tempera-
ture of T
f1
. Water temperature at the exit of the heated section is T
f2
. The heated
section of the pipe wall is maintained at a constant heat flux so that a constant
temperature difference of
∆T
s
= T
s
–
f
T exists between the wall and the bulk wa-
ter temperature. Show that for turbulent flow in the pipe and a specified heated
length and pipe diameter, water temperature at the exit of the heated section is
given by:
¸
¸
¹
·
¨
¨
©
§
∆
¸
¸
¹
·
¨
¨
©
§
¸
¸
¹
·
¨
¨
©
§
+=
2.06.0
2.0
8.0
2
Pr
0876.0
1
m
T
D
L
TT
s
ff
µ
49. Water flows in a heated tube at a velocity of 2 m/s. The tube length and di-
ameter are 20 cm and 6 mm, respectively. A constant heat flux is imposed on the
tube wall to maintain a constant temperature difference of 8 C between the tube
wall temperature and the bulk water temperature. For a water temperature of 40 C
at the inlet, find the water temperature at the outlet of the tube. [Ans.:
§ 44 C].
50. The surface of a cylinder, having a diameter of 25 cm, is maintained at 140 C.
Air flows over the cylinder at a steady state velocity of 50 m/s, a temperature of
35 C, and a pressure of 1 atm. Assuming the surface emissivity is very low, find

548 IVb. Heat Transfer: Forced Convection
the rate of heat transfer to the cylinder to make up the loss by convection to the
cross flow of air and maintain its temperature at the specified value.
51. The surface of a cylinder, having a diameter of 6 in and a length of 2 ft, is
maintained at 300 F. The cylinder is exposed to the cross flow of carbon dioxide
flowing at a steady state velocity of 150 ft/s, a temperature of 70 F, and a pressure
of 1 atm. Assuming the surface emissivity is very low, find the rate of heat trans-
fer to the cylinder to make up the heat loss by convection to the cross flow of air
and to maintain its temperature at the specified value.
52. A sphere made of copper, having a diameter of 2 cm, is heated to 50 C. We
now place this sphere in air flowing over the sphere at a velocity of 15 m/s, a tem-
perature of 17 C, and a pressure of 1 atm. Find the time the sphere temperature
drops to 25 C.
53. A steel pellet is heated up to 400 F and placed in air, flowing at a velocity of
155 ft/s over the pellet. The palette has a diameter of 0.5 in. We want to estimate
the cooldown rate of the pellet by using a lumped capacitance method. Plot the
pellet temperature versus time for 30 minutes. Data:
ε
= 0.8, T
air
= 100 F and P
air
= 1 atm,
ρ
= 488 lbm/ft
3
, c = 0.1 Btu/lbm·F.
54. A cross flow heat exchanger consists of a cluster of 25 tubes, arranged in a
staggered square array. Thus, there are 5 rows, each consists of 5 tubes. The tube
diameter, the longitudinal pitch, and the transverse pitch are 15 mm, 35 mm, and
32 mm, respectively. Air flows over the tube bank at a velocity of 8 m/s and an
inlet temperature of 27 C. The tube surface temperature is maintained at 77 C.
Find the rate of heat transfer and the air exit temperature.

1. Definition of Free Convection Terms 549
IVc
.
. Free Convection
Free or natural convection is that mode of heat transfer where fluid flows only due
to the presence of buoyancy forces. This in turn is the result of the action of body
forces, most notably gravity, in the presence of density gradient, generally due to a
temperature gradient. Although the rate of heat transfer by free convection is gen-
erally smaller than that of forced convection, the most notable advantage associ-
ated with free convection is in its passive nature, which in turn increases system
reliability. Since the lower rate of heat transfer results in higher thermal resis-
tance, making the application of this mode of heat transfer essential in enhancing
insulation. Free convection in flow loops results in the circulation of the working
fluid, referred to as natural circulation, which plays a major role in nuclear plants
during shutdown. Free convection does not always lead to natural circulation as
the latter requires the heat source to be located at a lower elevation than the heat
sink. Even in such case, the buoyancy force must be sufficient to overcome the
friction force caused by the fluid shear stresses. As shown in Section 2 of this
chapter, the interesting feature of free convection heat transfer is the fact that the
thermal and the hydrodynamic aspects are intertwined.
1. Definition of Free Convection Terms
Volumetric expansion coefficient (
β
) for a fluid, as defined in Chapter II, is
the change of fluid volume with temperature at constant pressure. The volumetric
expansion coefficient is given by
β
= (∂V/∂T)
P
/V and has units of inverse tem-
perature. We may approximately express the volumetric expansion coefficient as:
β
≅ [(V
1
− V
2
)/(T
1
− T
2
)]/V
1
= [(
ρ
1
−
ρ
2
)/(T
1
− T
2
)]/
ρ
1
. For ideal gases,
β
= 1/T
where T is the gas absolute temperature in degrees K or R.
Characteristic length is the length over which free convection is established.
For vertical flat plates and cylinders, this is the height of the plate. For horizontal
cylinders and sphere, this is the diameter. Finally, for horizontal plates, L = A
s
/P
where A
s
and P are the plate surface area and perimeter, respectively.
Grashof number, after Franz Grashof, is a measure of buoyancy as compared
with the viscous forces in the hydrodynamic boundary layer. Due to the appear-
ance of the buoyancy forces, the Grashof number plays an important role in heat
transfer by free convection. For flow of fluid over a plate, the Grashof number is:
Gr = g
β
(T
s
– T
f
)x
3
/v
2
The Gr number is used to determine relative importance of the modes of heat
transfer by convection. If Gr << Re
2
, then the forced convection mode is domi-
nant. For Gr >> Re
2
, the free convection mode is dominant.
Mixed Convection is that mode of convection heat transfer for which Gr
≈ Re
2
.
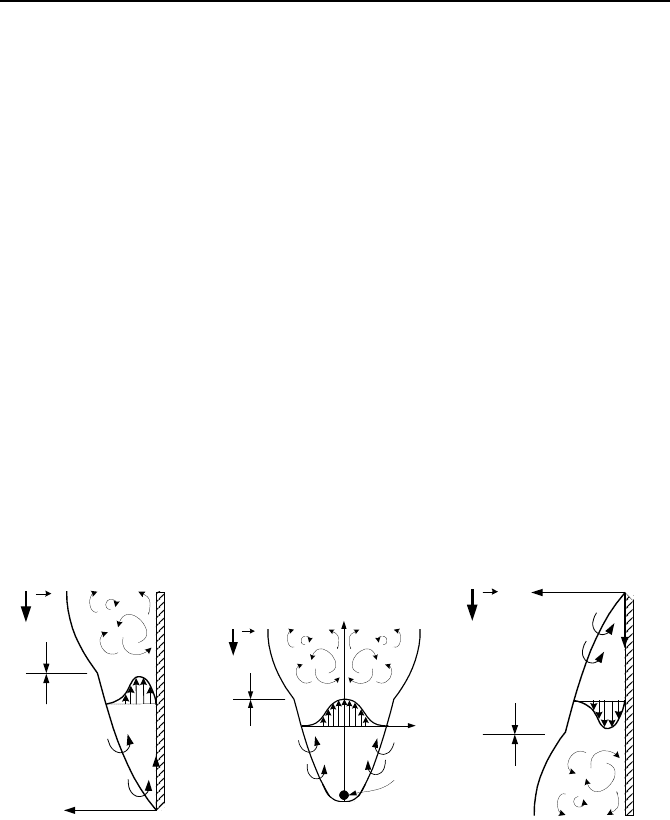
550 IVc. Heat Transfer: Free Convection
Modified Grashof Number (Gr*) is the product of Gr and Nu numbers, Gr* =
GrNu =
)/(
24
kvxQg
s
β
Rayleigh number (Ra) is the product of the Grashof and the Prandtl numbers,
Ra = GrPr.
2. Analytical Solution
Figure IVc.1 shows the free convection boundary layers for a hot vertical plate, a
hot horizontal wire and a cold vertical plate. Note that outside the boundary layer,
fluid is quiescent and V
f
= 0 since fluid away from the surface is stagnant. To
show the significance of the Grashof number, we obtain the kinetic energy of the
fluid in the boundary layer from an energy balance. Ignoring the frictional losses
on the wall we find that:
(
ρ
f
−
ρ
s
) gx/2 =
ρ
f
V
2
/2
where we have approximated the average density difference in the boundary layer
as (
ρ
f
−
ρ
s
)/2. For the flow in the boundary layer, we can find Re
2
= (Vx/v)
2
. Sub-
stituting for V
2
from the energy balance, we find Re
2
= [(
ρ
f
−
ρ
s
)gx/
ρ
f
](x/v)
2
, which
is an alternative way to represent the Gr number. If we substitute from the defini-
tion of the volumetric expansion coefficient, we find Gr = g
β
(
Τ
s
−
Τ
f
)x
3
/v
2
.
x
y
Quiescent Fluid
Laminar
Turbulent
g
T
f
,
ρ
f
T
s
T
s
>T
f
x
y
Quiescent Fluid
Laminar
Turbulent
g
T
f
,
ρ
f
T
s
T
s
>T
f
y
Quiescent Fluid
Laminar
Turbulent
g
T
f
,
ρ
f
x
T
s
T
s
< T
f
Figure IVc.1.1. Free convection boundary layers
2.1. External Laminar Flow
Consider the steady laminar flow of an incompressible fluid over a vertical flat
plate. Similar assumptions used in the forced convection analysis are applicable
here.
2. Analytical Solution 551
Determination of Velocity and Temperature Profiles
The governing equation for the hydrodynamic boundary layer is Equa-
tion III.3.20-1 repeated here as:
X
y
V
v
x
P
y
V
V
x
V
V
xx
y
x
x
ρρ
11
2
2
+
∂
∂
+
∂
∂
−=
∂
∂
+
∂
∂
IIIa.3.20-1
where body force is now accounted for. Since
∂P/∂x = −
ρ
f
g and X = −
ρ
g, the
momentum equation becomes:
2
2
2
2
)(
y
V
vTTg
y
V
vg
y
V
V
x
V
V
x
f
x
f
x
y
x
x
∂
∂
+−=
∂
∂
+
¸
¸
¹
·
¨
¨
©
§
−
=
∂
∂
+
∂
∂
β
ρ
ρρ
IVc.2.1
The temperature boundary layer is given by Equation III.3.23-1. Similar to the
forced convection case, we may find the velocity and the temperature profile in
the boundary layer from the boundary conditions:
Profile y = 0
δδ
Temperature T = T
s
T = T
f
∂T/∂y =0
Velocity V
x
= 0 V
x
= 0 ∂V
x
/∂y =0
For temperature profile we then find:
2
1
¸
¹
·
¨
©
§
−=
−
−
δ
y
TT
TT
fs
f
For the velocity profile at y = 0 from Equation IVc.2.1 we find an additional con-
dition:
vTTg
y
V
f
x
/)(
2
2
−−=
∂
∂
β
Hence, the velocity profile becomes:
2
1
¸
¹
·
¨
©
§
−=
δ
y
V
V
xo
x
where V
xo
is an assumed velocity since in free convection, V
f
= 0. Note that the
velocity profile also satisfies the continuity Equation IIIa.3.13-1 as it should. To
be able to solve the governing equations analytically, similar to forced convection,
we seek to convert the partial differential equations to ordinary differential equa-
tions. Ostrach used a change of variables from x and y to
ξ
so that
ξ
= (Gr
x
/4)
1/4
y/x
and a stream function
ψ given by ψ(x, y) = 4v(x/y)
ξ
f(
ξ
) = 4v(Gr
x
/4)
1/4
f(
ξ
) where
f(
ξ
) is a function to be determined. Having the stream function, V
x
and V
y
are
found in terms of
ξ
. For V
x
, we take the derivative of ψ with respect to y:
