Lyons W.C. (ed.). Standard handbook of petroleum and natural gas engineering.2001- Volume 1
Подождите немного. Документ загружается.

Differential and Integral Calculus
45
and the process may be repeated, as in
I {I [I
f(x)dxldxldx
+
ISlf(x)dx.9
The double integral of a function with two independent variables is of the
Definite multiple integrals are solved from the inner integral to the outer.
form
SI
f(x,y)dy dx
=
I
[I
f(x,y)dyIdx
where the limits of the inner integral are functions of x
or
constants as in the
definite integral
where a and b may be
f,(x)
and f,(x).
Second Fundamental Theorem
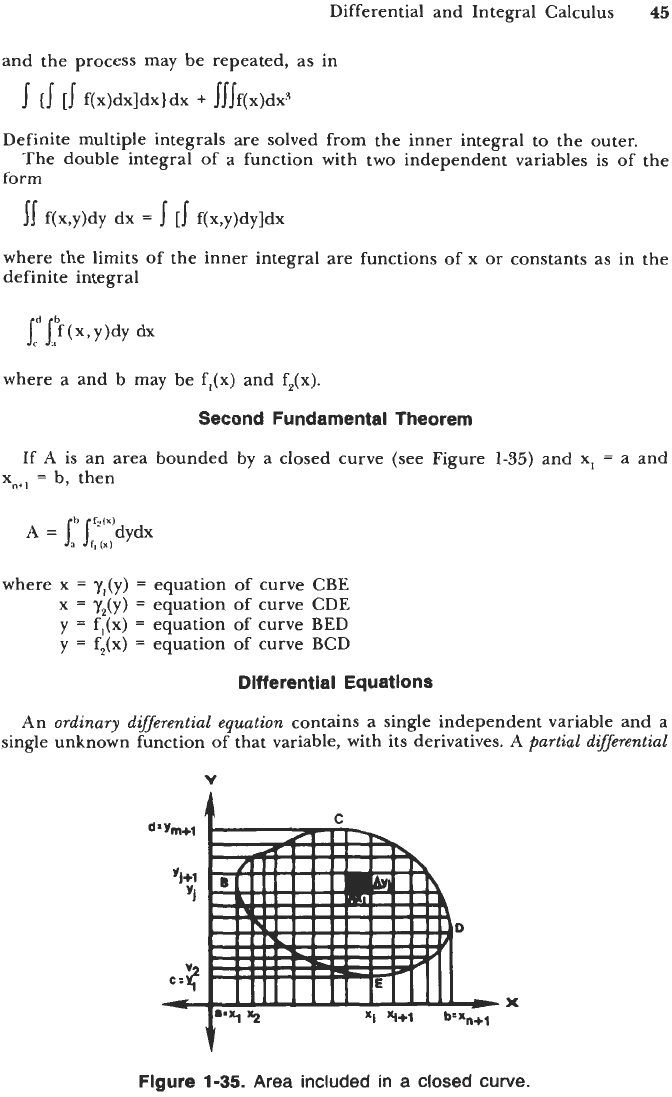
If A is an area bounded by a closed curve (see Figure
1-35)
and
x,
=
a and
x”+~
=
b, then
where
x
=
y,(y)
=
equation of curve CBE
x
=
y,(y)
=
equation
of
curve CDE
y
=
f,(x)
=
equation of curve BED
y
=
f,(x)
=
equation of curve BCD
Differential Equations
An
ordinary differential equation
contains a single independent variable and a
single unknown function
of
that variable, with its derivatives.
A
partial differential
V
X
Figure
1-35.
Area included
in
a
closed curve.

46
Mathematics
equation
involves an unknown function of two or more independent variables,
and its partial derivatives. The general solution of
a
differential equation of
order n is the set of all functions that possess at least n derivatives and satisfy
the equation, as well as any auxiliary conditions.
Methods
of
Solving Ordinary Differential Equations
For
first-order equations,
if possible, separate the variables, integrate both sides,
and add the constant of integration, C. If the equation is
homogeneous
in x and
y,
the value of dy/dx in terms of x and
y
is of the form dy/dx
=
f(y/x) and the
variables may be separated by introducing new independent variable
v
=
y/x
and then
dv
dx
x-+v
=
f(v)
The expression f(x,y)dx
+
F(x,y)dy is an
exact difj-erential
if
Then, the solution
of
f(x,y)dx
+
F(x,y)dy
=
0
is
jf(x,y)dx
+
[F(x,y)
-
IP
dxldy
=
C
or
hx,y)dy
+
[f(x,y)
-
h'
dyldx
=
C
A
linear differential equation
of the first order such as
dy/dx
+
f(x)
y
=
F(x)
has the solution
y
=
e-'[ jePF(x)dx
+
C] where
P
=
If(x)dx
In the class of nonlinear equations known as
Bernoulli's equations,
where
dy/dx
+
f(x)
y
=
F(x) y"
substituting y'-"
=
v
gives
dv/dx
+
(1
-
n)f(x)
v
=
(1
-
n)F(x)
[n
#
0 or 11
which is linear in
v
and x. In
Clairaut's equations
y
=
xp
+
f(p)
where p
=
dy/dx,
the solution consists of the set of lines given by
y
=
Cx
+
f(C), where
C
is any
constant, and the curve obtained by eliminating p between the original equation
and x
+
f'(p)
=
0
[l].

Differential and Integral Calculus
47
Some differential equations of the
second
order
and their solutions follow:
For d2y/dX2
=
-n2y
y
=
C,sin(nx
+
C,)
=
C,sin nx
+
C,cos nx
For d2y/dx2
=
+
n2
Y
y
=
C,sinh(nx
+
C,)
=
C enx
+
Cqe-"x
For d2y/dx2
=
f(y)
where P
=
jf(y)dy
For d2y/dx2
=
f(x)
y
=
jPdx
+
C,x
+
C, where
P
=
jf(x)dx
=
XP
-
jxf(x)dx
+
C,x
+
C,
For d2y/dx2
=
f(dy/dx), setting dy/dx
=
z
and dP/dx2
=
dz/dx
x
=
jdz/f(z)
+
C, and
y
=
jzdz/f(z)
+
C,, then eliminating
z
For d2y/dx2
+
Pb(dy/dx)
+
a2y
=
0
(the equation for damped vibration)
If a2
-
b2
>
0,
then m
=
Ja2
-
b2
y
=
C,e-bxsin(mx
+
C,)
=
e-bx[C,sin(mx)
+
C,cos(mx)]
If a2
-
b2
=
0,
If a,
-
b2
<
0,
y
=
e-bx(C,
+
C,x)
then n
=
db2
-
a' and
y
=
C,e-bxsinh(nx
+
C
)
=
C
e-(b+n)x
+
C
e-(b-&
For d2y/dx2
+
2b(dy/dx)
+
a2y
=
c
y
=
c/a2
+
y,
where
y,
is the solution of the previous equation with second term zero.
The preceding two equations are examples of linear differential equations with
constant coefficients and their solutions are often found most simply by the use
of Laplace transforms
[
13.
For the linear equation of the nrh order
An(x)d"y/dx"
+
An-,(x)d"-'y/dxn-'
+
.
.
.
+
A,(x)dy/dx
+
A,(x)y
=
E(x)
48
Mathematics
the general solution is
y
=
u
+
c,u,
+
C,U,
+ . .
.
+
C"U",
where u is any solution of the given equation and ul, uq,
. .
.,
un form a
fundamental system
of solutions to the homogeneous equation [E(x)
t
zero].
A
set of functions has linear independence if its Wronskian determinant, W(x),
#
0,
where
UI
up
...
u,
u, up
...
U"
. . ... .
W(x)
=
u;" u;
...
u:
and m
=
n
-
lLh
derivative. (In certain cases, a set of functions may be linearly
independent when W(x)
=
0.)
The Laplace Transformation
The
Laplace transformation
is based upon the Laplace integral which transforms
a differential equation expressed in terms of time to an equation expressed in
terms of
a
complex variable
B
+
jw.
The new equation may be manipulated
algebraically to solve for the desired quantity as an explicit function of the
complex variable.
Essentially three reasons exist for the use of the Laplace transformation:
1.
The ability to use algebraic manipulation to solve high-order differential
2.
Easy handling of boundary conditions
3.
The method is suited to the complex-variable theory associated with the
equations
Nyquist stability criterion
[
11.
In Laplace-transformation mathematics, the following symbols and variables
are used:
f(t)
=
a function of time
F(s)
=
the Laplace transform of
f,
expressed in
s,
resulting from operating on
s
=
a complex variable of the form
(O
+
jw)
f(t)
with the Laplace integral.
6:
=
the Laplace operational symbol, i.e.,
F(s)
=
S[f(t)].
The Laplace integral is defined as
6:
=
jo-e-"dt and
so
6:[f(t)]
=
re-'"f(t)dt
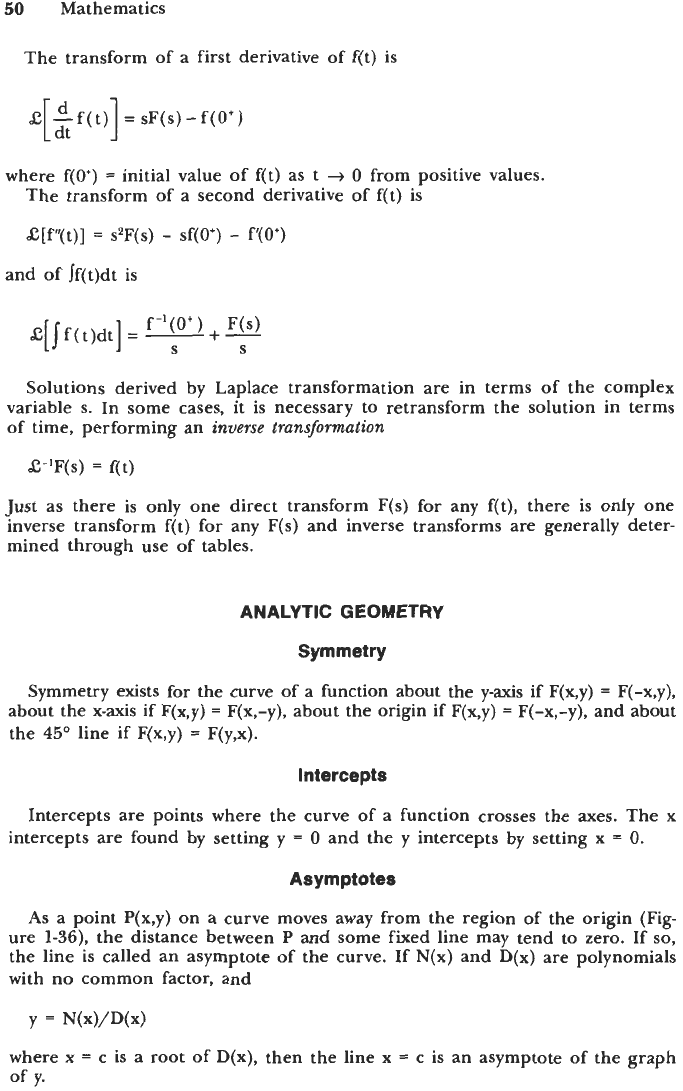
Table
1-8
lists the transforms of some common time-variable expressions.

Differential and Integral Calculus
49
Table
1-8
Laplace Transforms
Ae"'
sin
Pt
S
s2
+
p'
cos
pt
1
-
sin
pt
P
Ae-"
-
Be-w
C
A=a-a
where
B
=
a
-
p
C=P-a
e-&
ABC
-
e-a
+
-
+
-
A
=
(p
-
a)(6
-
a)
where
B
=
(a
-
p)(6
-
P)
c
=
(a
-
S)(P
-
6)
tn
d/dt[f(t)]
d2/dt2[f(t)]
n!
gt'
-
sF(s)
-
f(0')
df
dt
s2F(s)-sf(0+)--(0+)
df d2f
dt dt2
s3F(s)
-
s2f(0')
-
s-(O+)
-
-(O+)
d3/dt3[f(t)]
~[F(s)
+
jf(t)dt
I
of]
hdt
S
1
-sinh
at
a
cosh
at
1
s2
-
a2
s2
-
a2
S
50
Mathematics
The transform of a first derivative of f(t) is
S[$f(t)]
=
sF(s)-f(0')
where f(0')
=
initial value of f(t) as t
+
0
from positive values.
The transform of a second derivative of f(t) is
S[f"(t)]
=
s2F(s)
-
sf(0')
-
f'(0')
and of jf(t)dt is
f-'(O')
+
F(s)
S[jf(t)dt]
=
7
-
S
Solutions derived by Laplace transformation are in terms of the complex
variable
s.
In
some cases, it is necessary to retransform the solution in terms
of time, performing an
inverse transformation
S-'F(s)
=
f(t)
Just as there is only one direct transform F(s) for any f(t), there
is
only one
inverse transform
f(
t) for any F(s) and inverse transforms are generally deter-
mined through use of tables.
ANALYTIC GEOMETRY
Symmetry
Symmetry exists for the curve of a function about the y-axis if F(x,y)
=
F(-x,y),
about the x-axis if F(x,y)
=
F(x,-y), about the origin if F(x,y)
=
F(-x,-y), and about
the
45"
line
if
F(x,y)
=
F(y,x).
Intercepts
Intercepts are points where the curve of a function crosses the axes. The x
intercepts are found by setting
y
=
0
and the
y
intercepts by setting x
=
0.
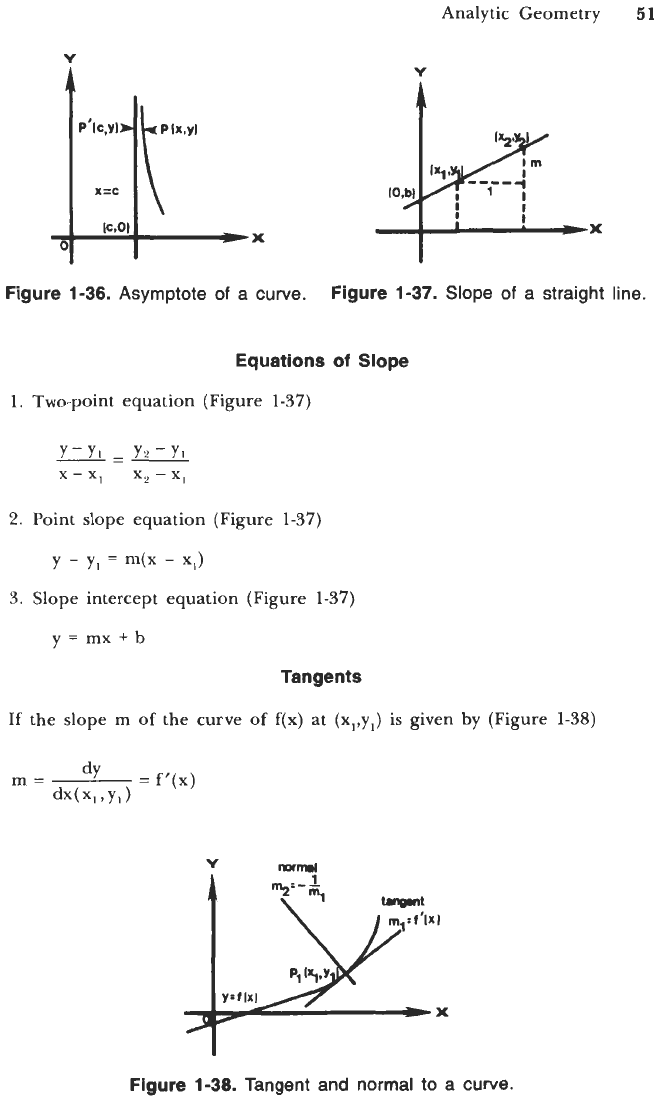
Asymptotes
As
a point P(x,y) on a curve moves away from the region of the origin (Fig-
ure
1-36),
the distance between P and some fixed line may tend to zero. If
so,
the line is called an asymptote
of
the curve. If N(x) and
D(x)
are polynomials
with no common factor, and
y
=
N(x)/D(x)
where x
=
c is a root of D(x), then the line x
=
c is an asymptote of the graph
of
y.
Analytic Geometry
51
V
V
I
X
Figure 1-36.
Asymptote
of
a
curve.
Figure 1-37.
Slope
of
a
straight line.
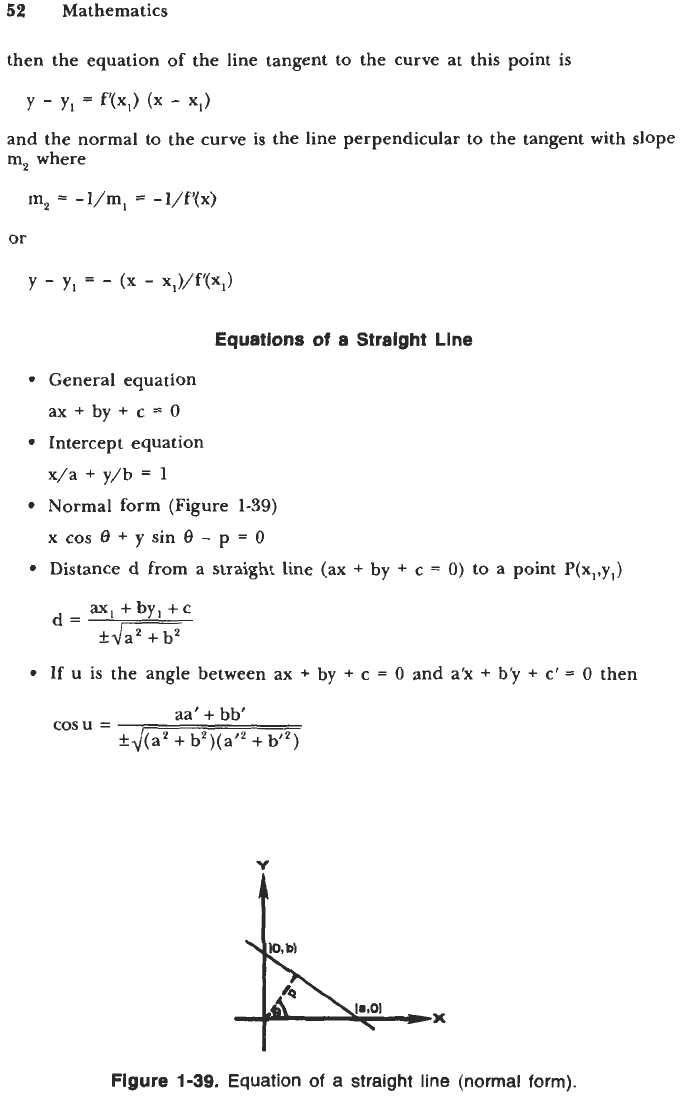
Equations
of
Slope
1.
Two-point equation (Figure 1-37)
Y
-Y1
=
Y'L-Y1
--
x-XI
X2-X1
2.
Point slope equation (Figure 1-37)
y
-
YI
=
m(x
-
XI)
3.
Slope intercept equation (Figure 1-37)
y=mx+b
Tangents
If
the slope m
of
the curve
of
f(x) at (xI,yI) is given by (Figure 1-38)
V
norm
Figure 1-38.
Tangent and
normal
to
a curve.
52
Mathematics
then the equation of the line tangent to the curve at this point
is
and the normal to the curve is the line perpendicular to the tangent with slope
m2 where
m2
=
-I/m,
=
-I/f'(x)
or
Equations
of
a
Straight Line
General equation
ax
+
by
+
c
-
0
9
Intercept equation
x/a
+
y/b
=
1
Normal form (Figure
1-39)
x cos
8
+
y
sin
8
-
p
=
0
9
Distance d from a straight line (ax
+
by
+
c
=
0)
to a point P(x,,y,)
ax,
+by,
-+
c
d=
kdPTi7
If
u
is the angle between ax
+
by
+
c
=
0
and a'x
+
b'y
+
c'
=
0
then
aa'
+
bb'
kd(a2
+
bZ)(a''
+
b'*)
COSU
=
V
Figure
1-39.
Equation
of
a
straight line (normal
form).

Analytic Geometry
53
Equations
of
a Circle (Center
(h,k))
(x
-
h)2
+
(y
-
k)2
=
r2
Origin at center
x2
+
y2
=
rz
General equation
x2
+
y2
+
Dx
+
Ey
+
F
=
0
where center
=
(-D/2,
-E/2)
radius
=
d(
D/2)'
+
(E/2)'
-
F
Tangent to circle at (x,,y,)
1 1
2
2
Parametric form, replacing x and
y
by
x
=
a cos
u
and
y
=
a sin
u
X,X
+
yIy
+
-D(x
+
x,)+
-
E(y
+
y,)
+
F
=
0
Equations
of
a Parabola (Figure 1-40)
A
parabola is the set of points that are equidistant from a given fixed point
(the focus) and from a given fixed line (the directrix) in the plane. The key
feature
of
a parabola is that it
is
quadrilateral in one of its coordinates and
linear in the other.
(y
-
k)'
=
~P(X
-
h)
Coordinates of the vertex V(h,k) and of the focus F(h
+
p,k)
Y
Figure 1-40.
Equation
of
a
parabola.

54
Mathematics
Origin at vertex
y2
=
4px
Equation of the directrix
x=h-p
Length of latus rectum
LL'
=
4p
Polar equation (focus as origin)
r
=
p/(1
-
cos
0)
Equation
of
the tangent to
y2
=
2
px at (xI,yI)
y,y
=
P(X
+
XI)
Equations
of
an Ellipse
of
Eccentricity
e
(Figure 1-41)
(x
-
h)'
(y
-
k)?
0-
+-=1
a2
b2
Coordinates
of
center C(h,k), of vertices V(h
+
a,k) and V'(h
-
a,k), and
of
foci
F(h
+
ae,k) and F'(h
-
ae,k)
Center at origin
x2/a2
+
y2/b2
=
1
Equation
of
the directrices
x
=
h
f
a/e
Equation
of
the eccentricity
Length of the latus rectum
LL'
=
2b2/a
Parametric form, replacing
x
and
y
by
x
=
a
cos
u
and
y
=
b
sin
u
Y
Figure 1-41.
Ellipse
of
eccentricity e.
