Lyons W.C. (ed.). Standard handbook of petroleum and natural gas engineering.2001- Volume 1
Подождите немного. Документ загружается.


Algebra
25
and the roots
-b
+
db2
-
4ac
2a
1.
and
-b
-
.\/b2
-
4ac
2a
2.
The sum of the roots is -b/a and their product is c/a.
after division by the coefficient of the highest-order term,
Third-degree equations
(cubic equations),
in the general case, have the form,
xJ
+
ax2
+
bx
+
c
=
0
with the solution
x:
=
Ax,
+
B
where x,
=
x
-
a/3
A
=
3(a/3)2
-
b
B
=
-2(a/3)3
+
b(a/3)
-
c
Exponential equations
are of the form
ax
=
b
with the solution x
=
(log b)/(log a) and the root (log b)/(log a). The complete
logarithm must be taken, not only the mantissa.
Trigonometric equations
are of the form
a cos x
*
b sin x
If an acute angle u is found, where
tan
u
=
b/a
and an angle
v
(0'
<
v
<
180')
is found, where
cos2
v
=
c2/(az
+
b2)
the solution is x
=
f(u
f
v)
and the roots are +(u
+
v)
and +(u
-
v), depending
on the sign of b.
Solution
of
Systems
of
Simultaneous Equations
A set of
simultaneous equations
is a system of n equations in n unknowns. The
solutions (if any) are the sets
of
values for the unknowns which will satisfy all
the equations in the system.

26
Mathematics
First-degree equations in
2
unknowns are of the form
alxl
+
b,x,
=
c1
a2xl
+
b,x,
=
c2
The solution is found by multiplication of Equations a and b by some factors
that will produce one term in each that will, upon addition of Equations a and
b, become zero. The resulting equation may then be rearranged to solve for
the remaining unknown. For example, by multiplying Equation a by a2 and
Equation b by -al, adding Equation a and Equation b and rearranging their sum
and by substitution in Equation a:
A
set of
n first-degree equations in n unknowns
is
solved in a similar fashion by
multiplication and addition to eliminate n
-
1
unknowns and then back substitu-
tion.
Second-degree eyuations in
2
unknowns
may be solved in the same way when
two of the following are given: the product of the unknowns, their
sum
or dif-
ference, the sum of their squares. For further solutions, see “Numerical Methods.”
Determinants
Determinants of the second order are of the following form and are evalu-
ated as
and of the third order as
and of higher orders, by the general rule as follows.
To
evaluate a determinant
of the nth order, take the eiements of the first column with alternate
plus
and
minus signs and form the sum of the products obtained by multiplying each of
these elements by its corresponding
minor.
The minor corresponding to any
element en is the determinant (of the next lowest order) obtained by striking
out from the given determinant the row and column containing en.
Some of the general properties of determinants are:
1.
Columns may be changed to rows and rows to columns.
2.
Interchanging two adjacent columns changes the sign of the result.

Trigonometry
27
3.
If two columns are equal or if one is a multiple of the other, the deter-
4.
To
multiply a determinant by any number m, multiply all elements of any
Systems of simultaneous equations may be solved by the use
of
determinants
in the following manner. Although the example is a third-order system, larger
systems may be solved by this method. If
minant is zero.
one column by m.
a,x
+
b,y
+
c,z
=
p,
a,x
+
b,y
+
c?z
=
p2
agx
+
b,y
+
clz
=
p1
and
if
then
x
=
D,/D
y
=
D,/D
z
=
DJD
where
TRIGONOMETRY
Directed
Angles
If AB and AB' are
<BAB' is the ordered
the terminal side. <BAB'
#
<B'AB and any directed angle may be
I
0"
or
2
180".
same end point A, the directed
is the initial side of <BAB' and A
28
Mathematics
A
directe
figure.
If
A
about its end point
A
to form
<BAB',
A
angle may be thought of as an amount of rotation rather than a
is considered the initial
PO
ition of the ray, which is then rotated
3
-4
'
is its terminal position.
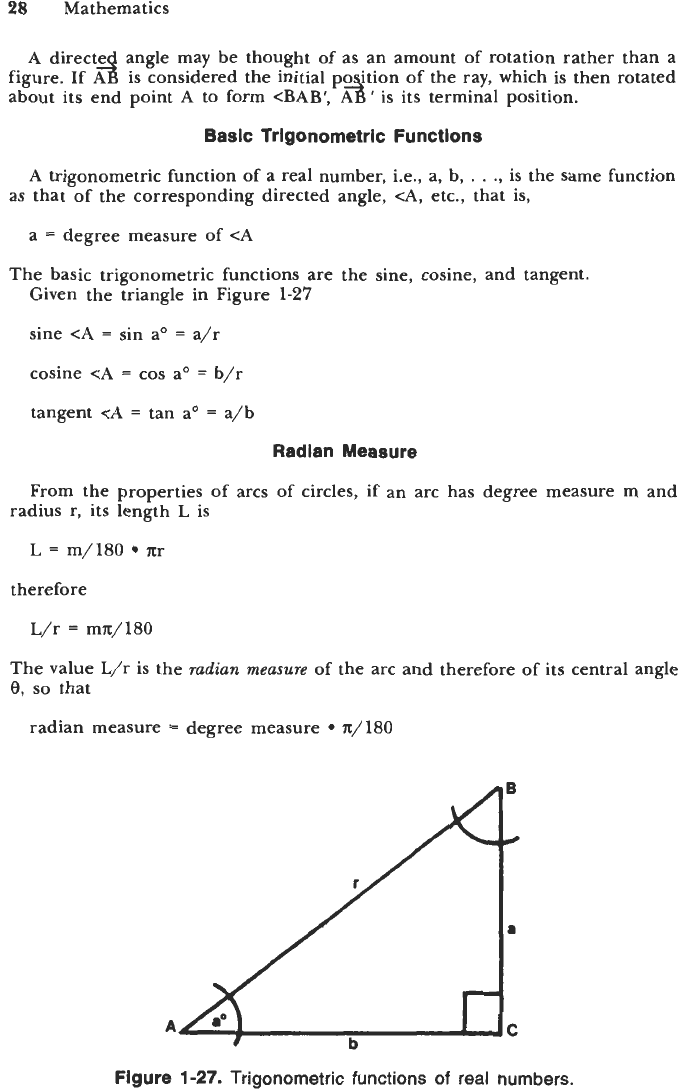
Basic Trigonometric Functions
A
trigonometric function of a real number, i.e., a, b,
. .
.,
is the same function
as that of the corresponding directed angle,
<A,
etc., that is,
a
=
degree measure of
<A
The basic trigonometric functions are the sine, cosine, and tangent.
Given the triangle in Figure
1-27
sine
<A
=
sin a'
=
a/r
cosine
<A
=
cos aQ
=
b/r
tangent
<A
=
tan a'
=
a/b
Radian Measure
From the properties of arcs of circles, if an arc has degree measure m and
radius r, its length
L
is
L
=
m/lSO 5cr
therefore
L/r
=
mn/180
The value L/r is the
radian
measure
of the arc and therefore
of
its central angle
8,
so
that
radian measure
=
degree measure
n/180
Figure
1-27.
Trigonometric functions
of
real numbers.

Trigonometry
29
Trigonometric Properties
For any angle
8
as in Figure 1-28
sin
0
=
opposite side/hypotenuse
=
s,/h
cos
0
=
adjacent side/hypotenuse
=
sJh
tan
8
=
opposite side/adjacent side
=
sI/s2
=
sin 8/cos
8
and the reciprocals of the basic functions (where the function
#
0)
cotangent
8
=
cot
8
=
l/tan
8
=
SJS,
secant
0
=
sec
8
=
l/cos
8
=
h/s2
cosecant
8
=
csc
8
=
l/sin
8
=
h/s,
The functions versed sine, coversed sine, and exterior secant are defined as
vers
0
=
1
-
cos
8
covers
8
=
1
-
sin
8
exsec
0
=
sec
0
-
1
To
reduce an angle
to
the first quadrant of the unit circle, that is, to a degree
measure between
0"
and
90",
see Table
1-1.
For function values at major angle
values, see Tables
1-2
and
1-3.
Relations between functions and the sum/
difference
of
two functions are given in Table 1-4. Generally, there will be
two
angles between
0"
and
360"
that correspond to the value of a function.
Figure
1-28.
Trigonometric functions
of
angles.
(text
continued
on
page
32)
30
Mathematics
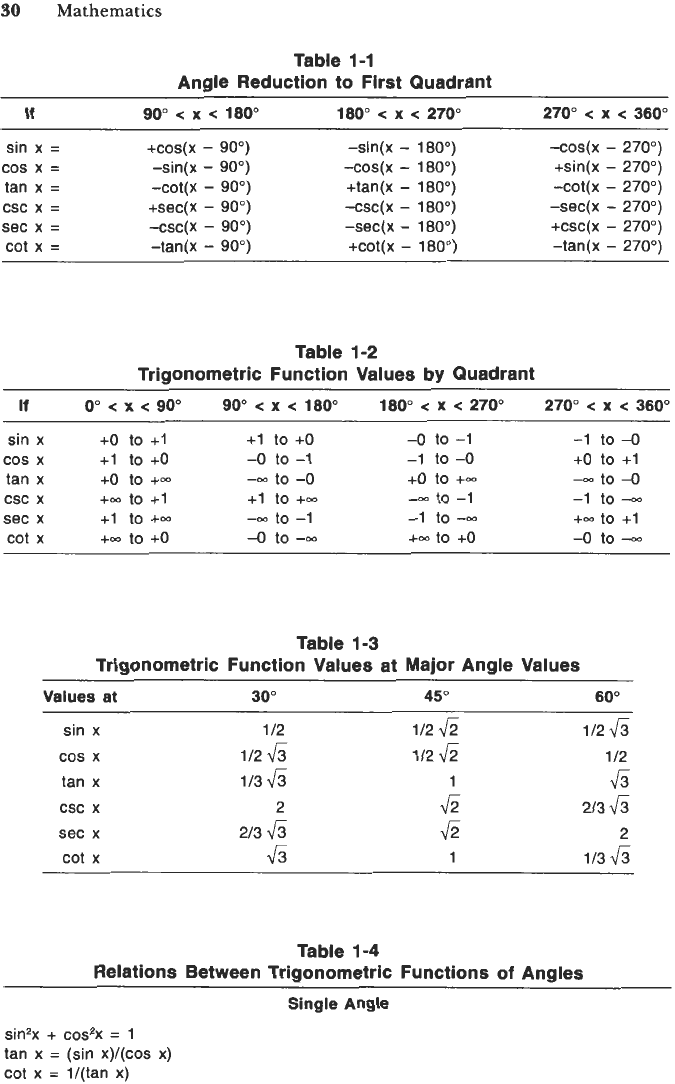
Table
1-1
Angle Reduction to First Quadrant
If
90"
<
x
<
180"
180"
<
x
<
270" 270"
x
<
360"
sin x
=
+cos(x
-
900)
-sin(x
-
180O) -cos(x
-
270")
tan x
=
-cot(x
-
900)
+tan(x
-
180") -COt(X
-
270")
cos x
=
-sin(x
-
90")
-cos(x
-
180') +sin(x
-
270")
csc x
=
+sec(x
-
90°)
-CSC(X
-
180") -sec(x
-
270")
cot x
=
-tan(x
-
90")
+COt(x
-
180") -tan(x
-
270')
sec x
=
-csc(x
-
900)
-sec(x
-
180")
+CSC(X
-
270")
Table
1-2
Trigonometric Function Values by Quadrant
If
0"
<
x
<
90" 90"
<
x
<
180"
180"
<
x
<
270" 270"
<
x
<
360"
sin x
+o
to +1 +1
to
+o
-0
to -1
-1
to
-0
cos
x
+1
to
+o
-0
to -1 -1 to
-0
+o
to +1
tan x
+o
to
+-
--
to
-0
+o
to
+-
--
to
-0
csc x
+-
to +1 +1 to
+-
--
to -1 -1
to
-m
see
x
+1 to
+-
--
to -1
-1
to
--
+-
to
+1
cot x
+-
to
+o
-0
to
--
+-
to
+o
-0
to
--
Table
1-3
Trigonometric Function Values at Major Angle Values
Values at 30"
45"
60"
sin x 112 112
v5
112
6
cos x 112
&
112
v5
112
tan x 113
&
csc x
sec x
2
213
&
1
&
213
6
2
cot x
6
1 113
&
Table
1-4
Relations Between Trigonometric Functions
of
Angles
Single Angle
sin'x
+
cos2x
=
1
tan x
=
(sin x)/(cos x)
cot x
=
l/(tan x)

Trigonometry
3
1
1
+
tan2x
=
sec2x
1
+
cotzx
=
CSCZX
sin(-x)
=
-sin x, cos(-x)
=
cos x, tan(-x)
=
-tan
x
Two
Angles
sin(x
+
y)
=
sin x cos y
+
cos x sin y
sin(x
-
y)
=
sin x cos y
-
cos x sin y
cos(x
+
y)
=
cos
x
cos y
-
sin
x
sin y
cos(x
-
y)
=
cos x cos y
+
sin
x
sin y
tan(x
+
y)
=
(tan x
+
tan y)/(l
-
tan x tan y)
tan(x
-
y)
=
(tan
x
-
tan y)/(l
+
tan x tan y)
cot(x
+
y)
=
(cot x cot y
-
l)/(cot y
+
cot x)
cot(x
-
y)
=
(cot x cot
y
+
l)/(cot y
-
cot x)
sin x
+
sin y
=
2 sin[l/2(x
+
y)] cos[l/2(x
-
y)]
sin
x
-
sin y
=
2 cos[l/2(x
+
y)] sin[l/2(x
-
y)]
cos x
+
cos y
=
2 cos[l/2(x
+
y)] cos[l/2(x
-
y)]
cos x
-
cos y
=
-
2 sin[l/2(x
+
y)] sin[l/2(x
-
y)]
tan x
+
tan y
=
[sin(x
+
y)]/[cos x cos y]
tan x
-
tan y
=
[sin(x
-
y)]/[cos x cos y]
cot x
+
cot y
=
[sin(x
+
y)]/[sin x sin y]
cot x
-
cot y
=
[sin(y
-
x)]/[sin x sin y]
sin2x
-
sin2y
=
cos2y
-
cos2x
cos2x
-
sin2y
=
cos2y
-
sin2x
sin(45"
+
x)
=
cos(45"
-
x), tan(45"
+
x)
=
cot(45"
-
x)
sin(45"
-
x)
=
cos(45"
+
x), tan(45"
-
x)
=
cot(45"
+
x)
Multlple and
Half
Angles
=
sin(x
+
y) sin(x
-
y)
=
cos(x
+
y) cos(x
-
y)
tan 2x
=
(2 tan x)/(l
-
tan2x)
sin(nx)
=
n sin x cosn-'x
-
(n) ,sin3x
COS~X
+
(n)5sin5x cosW5x
-
cos(nx)
=
cosnx
-
(n),sin2x cosw2x
+
(n),sin4x
COS~X
-
.
. .
(Note: (n)z,
.
. .
are the binomial coefficients)
sin(x/2)
=
i
J1/2o
cot 2x
=
(COt2X
-
1)/(2 cot x)
COS(x/2)
=
f
Jl2
(1
+
cos
x)
tan(x/2)
=
(sin x)/(l
+
cos x)
=
f
J(1-
COS
x)/(I
+
COS
x)
Three
Angles Whose
Sum
=
180"
sin
A
+
sin B
+
sin C
=
4 cos(N2) cos(B/2) cos(CI2)
cos
A
+
cos B
+
cos C
=
4 sin(N2) sin(B/2) sin(C/2)
+
1
sin A
+
sin B
-
sin
C
=
4 sin(N2) sin(B/2) cos(C/2)
cos A
+
cos B
-
cos C
=
4 cos(N2) cos(B/2) sin(CI2)
-
1
sin2A
+
sin2B
+
sin%
=
2 cos
A
cos B cos C
+
2
sinZA
+
sin2B
-
sin%
=
2 sin A sin B cos C
tan A
+
tan B
+
tan C
=
tan A tan B tan C
sin 2A
+
sin 28
+
sin 2C
=
4 sin A sin B sin C
sin 2A
+
sin 28
-
sin 2C
=
4
cos
A cos B sin C
cot(N2)
+
cOt(B/2)
+
cot(C/2)
=
cot(N2) cot(B/2) cot(C/2)
32
Mathematics
(text continued
from
page
29)
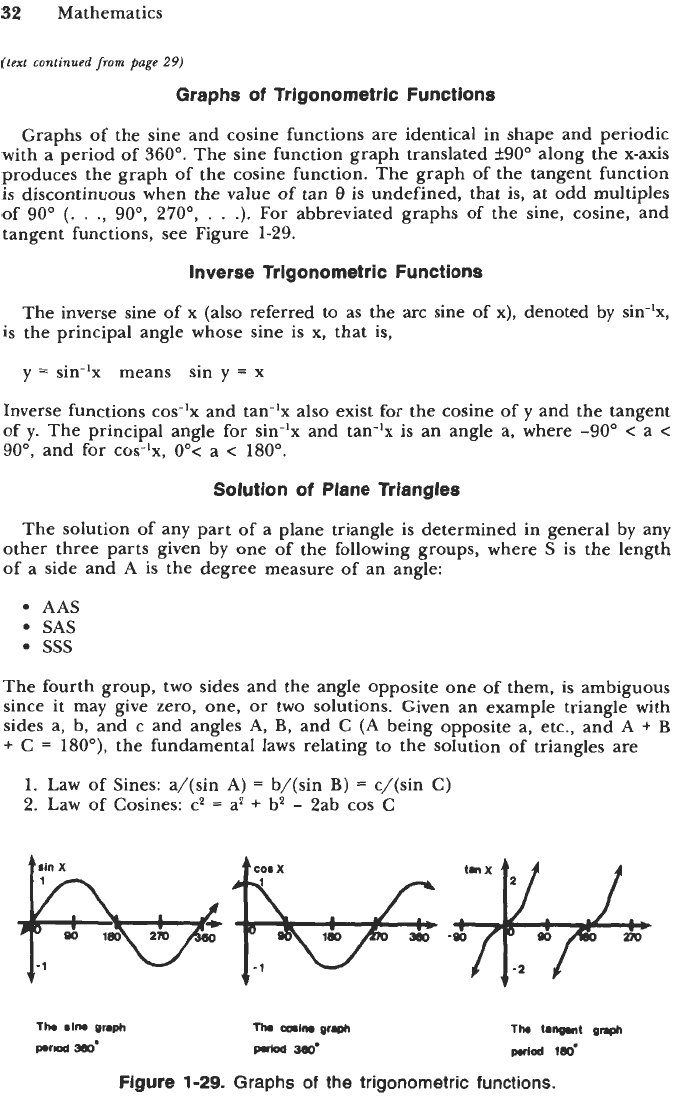
Graphs
of
Trigonometric Functions
Graphs
of
the sine and cosine functions are identical in shape and periodic
with a period of
360".
The sine function graph translated
f90"
along the x-axis
produces the graph of the cosine function. The graph of the tangent function
is discontinuous when the value
of
tan
9
is undefined, that is, at odd multiples
of
90"
(.
.
.,
go", 270",
.
.
.).
For abbreviated graphs of the sine, cosine, and
tangent functions, see Figure 1-29.
Inverse Trigonometric Functions
The inverse sine of x (also referred to as the
arc
sine of x), denoted by sin-'x,
is
the principal angle whose sine is x, that is,
y
=
sin-'x means sin
y
=
x
Inverse functions
COS-'^
and tan-'x also exist for the cosine of
y
and the tangent
of
y. The principal angle for sin-'x and tan-'x is an angle a, where
-90"
<
a
<
go", and for cos-'x,
O"<
a
<
180".
Solution
of
Plane Triangles
The solution of any part of a plane triangle is determined in general by any
other three parts given by one of the following groups, where
S
is the length
of a side and
A
is the degree measure of an angle:
AAS
SAS
sss
The fourth group, two sides and the angle opposite one
of
them, is ambiguous
since
it
may give zero, one,
or
two solutions. Given an example triangle with
sides a, b, and c and angles
A,
B,
and C
(A
being opposite a, etc., and
A
+
B
+
C
=
180"), the fundamental laws relating to the solution of triangles are
1. Law of Sines: a/(sin
A)
=
b/(sin
B)
=
c/(sin C)
2. Law of Cosines':
2
=
4*i"
x
..
ai
+
b2
1
2ab cos
C
Figure
1-29.
Graphs of the trigonometric functions.
Trigonometry
33
Hyperbolic Functions
The
hyperbolic sine, hyperbolic cosine,
etc. of any number x are functions related
to
the exponential function
ex.
Their definitions and properties are very similar
to the trigonometric functions and are given in Table
1-5.
The
inverse hyperbolic functions,
sinh-’x, etc., are related to the logarithmic
functions and are particularly useful in integral calculus. These relationships may
be defined for real numbers x and
y
as
sinh-’ (x/y)
=
In( x
+
Jx*
+
y2
)
-
In
y
ash-’ (x/y)
=
In( x
+
Jx*
-
y2
)
-
In
y
tanh-’(x/y)
=
1/2
In[(y
+
x)/(y
-
x)]
coth-’(x/y)
=
1/2
In[(x
+
y)/(x
-
y)]
Table
1-5
Hyperbolic Functions
sinh
x
=
1/2(ex
-
e-.)
cosh x
=
1/2(eK
+
e-.)
tanh
x
=
sinh x/cosh
x
csch
x
=
l/sinh x
sech
x
=
llcosh x
coth
x
=
l/tanh
x
sinh(-x)
=
-sinh
x
tanh(-x)
=
-tanh
x
cosh’x
-
sinh’x
=
1
1
-
tanh‘x
=
sech’x
cosh(-x)
=
COSh
x
1
-
COth’X
=
-
CSCh’X
sinh(x
f
y)
=
sinh
x
cosh y
f
cosh
x
sinh
y
cosh(x
f
y)
=
cosh
x
cosh y
f
sinh x sinh y
tanh(x
f
y)
=
(tanh x
f
tanh y)/(l
f
tanh
x
tanh y)
sinh 2x
=
2 sinh
x
cosh
x
cosh 2x
=
cosh2x
+
sinhzx
tanh 2x
=
(2
tanh x)/(l
+
tanh2x)
sinh(d2)
=
.\11/2(cosh
x
-
1)
~0~h(x/2)
=
,/1/2(cosh
x
+
1)
tanh(d2)
=
(cosh
x
-
l)/(sinh x)
=
(sinh x)/(cosh
x
+
1)

34
Mathematics
Polar Coordinate System
The
polar
coordinate
system
describes the location of a point (denoted as [r,81)
in a plane by specifying a distance r and an angle
8
from the origin
of
the
system. There are several relationships between polar and rectangular coordinates,
diagrammed in Figure
1-30.
From the Pythagorean Theorem
Also
sin
8
=
y/r or
y
=
r sin
8
cos
8
=
x/r or x
=
r cos
8
tan
8
=
y/x or
8
=
tan-'(y/x)
To
convert rectangular coordinates to polar coordinates, given the point (x,y),
using the Pythagorean Theorem and the preceding equations.
[r,~]
=
[J-,tan-'(y/x)]
To convert polar to rectangular coordinates, given the point [r,eI:
(x,y)
=
[r cos
8,
r sin
81
For graphic purposes, the polar plane is usually drawn as a series of con-
centric circles with the center at the origin and radii
1,
2,
3,
.
.
..
Rays from
the center are drawn at
0",
15", 30°,
. .
.,
360'
or
0,
7t/12, x/6,
n/4,
.
.
.,
27t
V
Figure
1-30.
Polar
coordinates.
