Lyons W.C. (ed.). Standard handbook of petroleum and natural gas engineering.2001- Volume 1
Подождите немного. Документ загружается.

Differential and Integral Calculus
35
Figure
1-31.
The
polar
plane.
radians. The origin is called the
pole,
and points [r,0] are plotted by moving
a positive or negative distance r horizontally from the pole, and through an
angle
0
from the horizontal. See Figure
1-31
with
0
given in radians as used in
calculus. Also note that
[r,
01
=
[-r,e
+
n]
DIFFERENTIAL AND INTEGRAL CALCULUS
See References
1
and
5-8
for additional information.
Derivatives
Geometrically, the derivative of
y
=
f(x) at any value xn is the slope of a
tangent line T intersecting the curve at the point P(x,y).
Two
conditions applying
to differentiation (the process of determining the derivatives of a function) are:
1.
The primary (necessary and sufficient) condition is that
lim
AY
AXXOO,
exists and is independent of the way in which Ax+O
2.
A
secondary (necessary, not sufficient) condition is that
lim
~
Of(x+Ax)
=
f(x)
A
short table of derivatives will be found in Table
1-6.

36
Mathematics
Table
1-6
Table
of
Derivatives*
d
-(XI
=
1
dx
d
-(a)
=
0
dx
d du dv
dx dx dx
-(UfVf
.....)
=
--f--f
.....
d du
-(au) =a-
dx dx
d dv du
dx dx dx
-(uv)
=
u-+v-
du dv
-
dx dx
du
v--u-
dx v
V2
d du
dx dx
d log, e du
dx u dx
d 1 du
dx u dx
d du
dx dx
d du
dx dx
d du dv
dx dx dx
d. du
--mu
=
cosu-
dx dx
d du
-cosu
=
-sinu-
dx dx
d du
-
tanu
=
sec2 u-
dx dx
d du
-cotu
=
-csc2u-
dx dx
d
du
-secu
=
secutanu-
dx
dx
d du
-cscu
=
-cscucotu-
dx
dx
d du
-
vers u
=
sin u
-
dx dx
-(us)
=
nu"-'
-
-log,u
=
-1ogu
=
--
-a"
=
a"*loga*-
-
eu
=
e'
-
-
u"
=
vu"-'
-
+
u" logu
-
d 1 du
-tan-' u
=
-
-
dx l+uz dx
1
du -cot-'u d
=
dx 1+u2 dx
-
d vers-' u
=
-
1
-
du
-sinhu d.
=
coshu- du
dx dx
-coshu d
=
sinhu- du
dx dx
-
d tanh u
=
sech2 u
-
du
dx dx
-
d COth
u
=
-
CSCh2
u
-
du
dx dx
-
d sech u
=
-
sech u tanh u
-
du
dx dx
-CSChu d
=
-csChUCOthu- du
dx dx
dx
,In
dx
1 du
-
d c0th-l u
=
-
-
-
dx u2
-
1 dx
'Note:
u
and
v
represent funcitons
of
x.
All
angles are
in
radians.

Differential and Integral Calculus
37
Higher-Order Derivatives
The
second derivative
of
a
function
y
=
f(x), denoted f"(x) or dzy/dx2 is the
derivative of f'(x) and the
third derivative,
f"'(x) is the derivative of f"(x).
Geometrically, in terms of f(x): if f"(x)
0
then f(x) is concave upwardly, if f"(x)
<
0 then f(x) is concave downwardly.
Partial Derivatives
If u
=
f(x,y,
.
.
.)
is a function of two
or
more variables, the
partial derivative
of u with respect to x, fx(x,y,
.
.
.)
or &/ax, may be formed by assuming x to
be the independent variable and holding (y,
. .
.)
as constants. In a similar
manner, fy(x,y,
. .
.)
or au/ay may be formed by holding (x,
. .
.)
as constants.
Second-order partial derivatives of f(x,y) are denoted by the manner of their
formation as fm,
f,
(equal to f,,),
f,
or as a2u/ax2, a2u/axay, a2u/ay2, and the
higher-order partia! derivatives are likewise formed.
Implicit functions,
i.e., f(x,y)
=
0,
may be solved by the formula
at the point in question.
Maxima and Minima
A
critical point
on a curve
y
=
f(x) is a point where
y'
=
0,
that is, where the
tangent to the curve is horizontal. A critical value of x, therefore, is a value
such that f'(x)
=
0.
All roots of the equation f'(x)
=
0
are critical values of x,
and the corresponding values of
y
are the critical values of the function.
A function f(x) has a
relative maximum
at x
=
a if f(x)
<
f(a) for all values of
x (except a) in some open interval containing a and a
relative minimum
at x
=
b
if f(x)
>
f(b) for all
x
(except b) in the interval containing b. At the relative
maximum a of f(x), f'(a)
=
0,
i.e., slope
=
0,
and f"(a)
<
0, Le., the curve is
downwardly concave at this point, and at the relative minimum b, f'(b)
=
0 and
f"(b)
>
0
(upward concavity). In Figure
1-32,
A,
B,
C,
and
D
are critical points
Figure
1-32.
Maxima and minima.
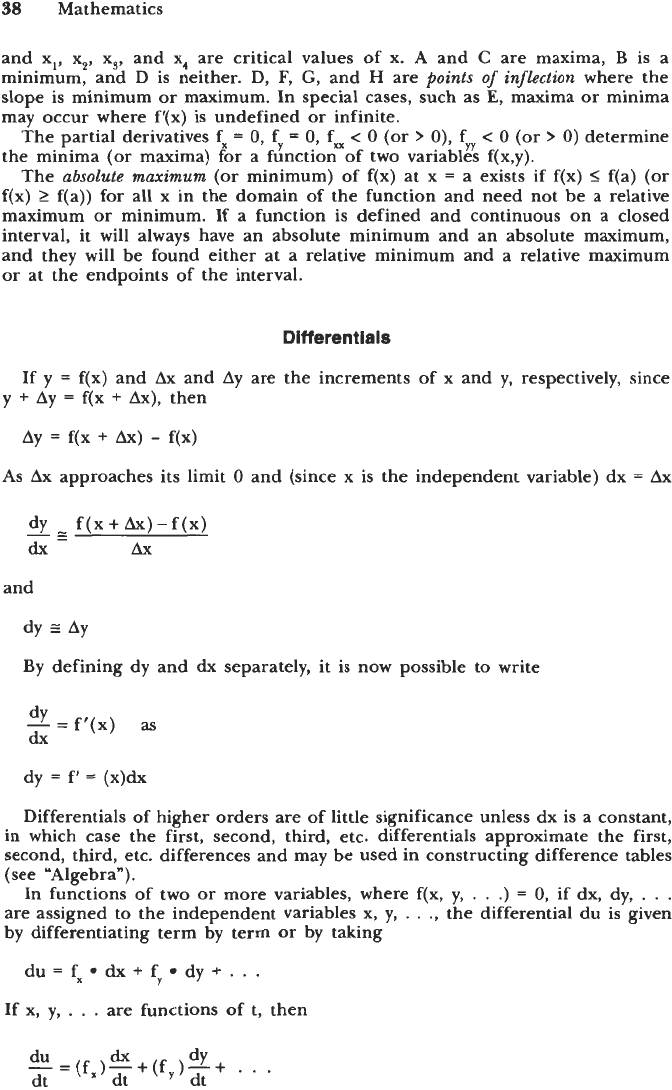
38
Mathematics
and xl, xp, x3, and x4 are critical values of x. A and
C
are maxima,
B
is a
minimum, and D is neither. D,
F,
G,
and
H
are
points of inflection
where the
slope is minimum or maximum. In special cases, such as
E,
maxima
or
minima
may occur where f'(x) is undefined or infinite.
The partial derivatives f,
=
0,
f,
=
0,
f,
<
0
(or
0),
f,,
<
0
(or
>
0)
determine
the minima (or maxima) for a function of two variables f(x,y).
The
absolute maximum
(or minimum) of f(x) at x
=
a exists if f(x)
5
f(a) (or
f(x)
2
f(a)) for all x in the domain
of
the function and need not be a relative
maximum or minimum. If a function is defined and continuous on a closed
interval, it will always have an absolute minimum and an absolute maximum,
and they will be found either at a relative minimum and a relative maximum
or at the endpoints of the interval.
Differentials
If
y
=
f(x) and Ax and
Ay
are the increments of x and
y,
respectively, since
y
+
Ay
=
f(x
+
Ax),
then
Ay
=
f(x
+
AX)
-
f(X)
As Ax approaches its limit
0
and (since x is the independent variable) dx
=
Ax
and
dy
5
Ay
By defining dy and
dx
separately, it is now possible to write
dY
-
=
f'(x)
dx
dy
=
f'
=
(x)dx
Differentials of higher orders are of little significance unless dx
is
a constant,
in which case the first, second, third, etc. differentials approximate the first,
second, third, etc. differences and may be used in constructing difference tables
(see "Algebra").
In functions
of
two or more variables, where f(x, y,
. .
.)
=
0,
if dx, dy,
. .
.
are assigned to the independent variables x,
y,
.
.
.,
the differential du is given
by differentiating term by term or by taking
du=fx*dx+fy*dy+.
. .
If x, y,
. .
.
are functions
of
t, then
_-
du
dx
dY
-(f,)z+(f
)-+
. .
.
dt dt
Differential and Integral Calculus
39
expresses the rate of change of
u
with respect to t, in terms of the separate
rates of change of x, y,
. . .
with respect to t.
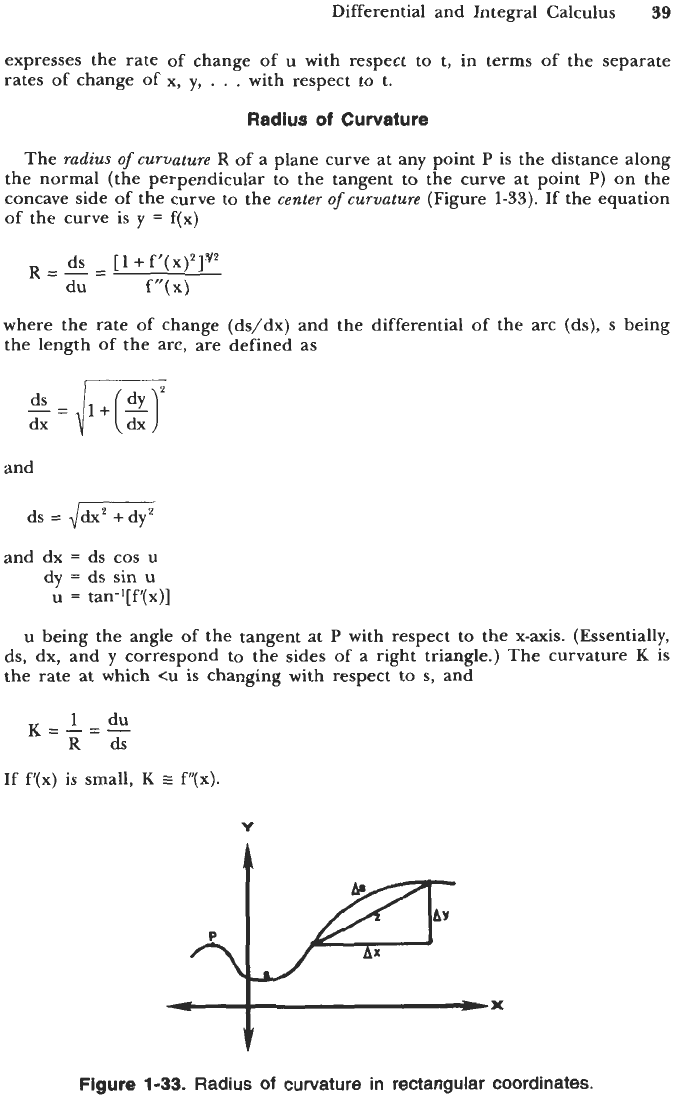
Radius
of
Curvature
The
radius
of
curvature
R
of a plane curve at any point
P
is the distance along
the normal (the perpendicular to the tangent to the curve at point
P)
on the
concave side of the curve to the
center
of
curvature
(Figure
1-33).
If the equation
of the curve is
y
=
f(x)
where the rate of change (ds/dx) and the differential of the arc (ds),
s
being
the length of the arc, are defined as
and
ds
=
Jdx'
+
dy2
and dx
=
ds cos
u
dy
=
ds sin
u
u
=
tan-'[f'(x)]
u
being the angle of the tangent at
P
with respect to the x-axis. (Essentially,
ds,
dx, and
y
correspond to the sides
of
a right triangle.) The curvature
K
is
the rate at which
<u
is changing with respect
to
s,
and
1
du
R
ds
K=-=-
If f'(x) is small,
K
s
f"(x).
Figure
1-33.
Radius
of
curvature in rectangular coordinates.
40
Mathematics
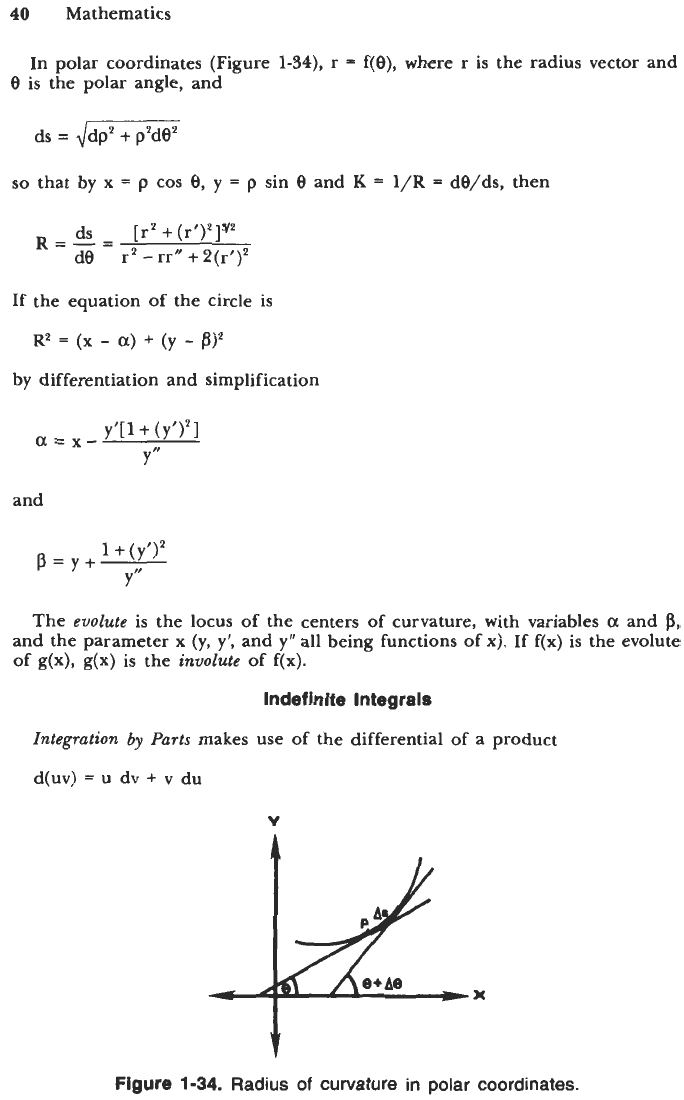
In polar coordinates (Figure
1-34),
r
=
f(8), where r is the radius vector and
8
is the polar angle, and
so
that by x
=
p
cos
8,
y
=
p
sin
8
and
K
=
1/R
=
de/ds, then
ds [r2 +(r’)2]9YS
de
R=-=
r2
-
rr”
+
2(r’)‘
If the equation of the circle
is
R2
=
(x
-
a)
+
(y
-
p)‘
by differentiation and simplification
Y’C1+
(Y’)‘]
Y”
a=x-
and
The
evolute
is the locus of the centers of curvature, with variables
a
and
p,
and the parameter x
(y,
y’, and
y”
all being functions of x). If f(x) is the evolute
of
g(x),
g(x) is the
involute
of f(x).
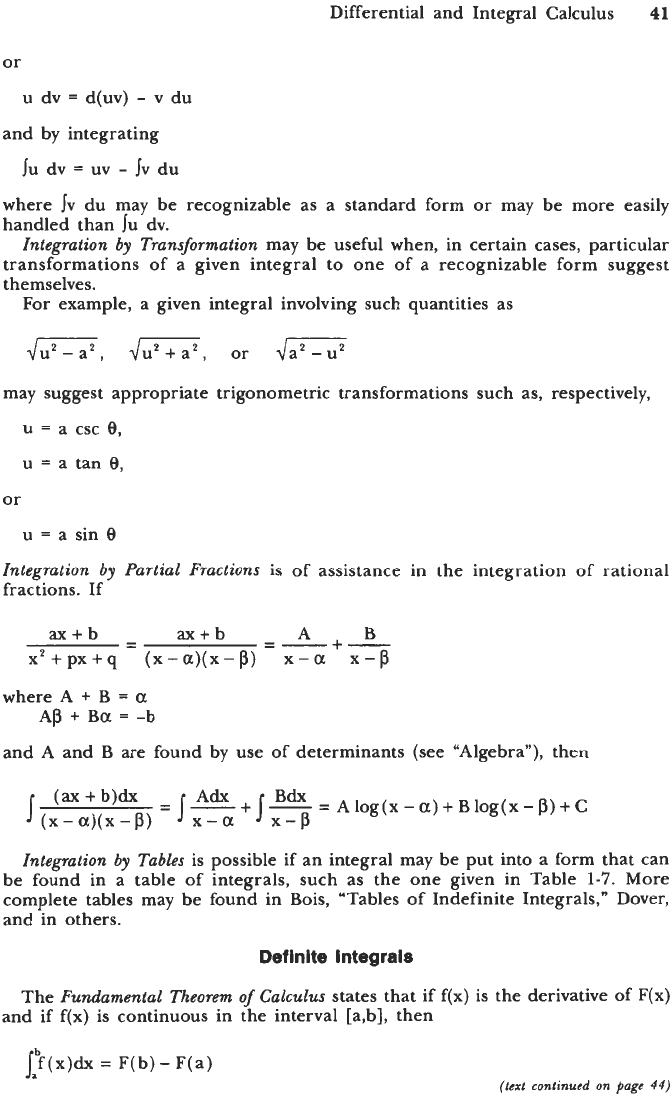
Indefinite Integrals
Integration
by
Parts
makes use of the differential of a product
d(uv)
=
u
dv
+
v
du
V
Figure
1-34.
Radius
of
curvature in polar coordinates.
Differential and Integral Calculus
41
or
u
dv
=
d(uv)
-
v
du
and by integrating
ju dv
=
uv
-
jv du
where
Iv
du may be recognizable as a standard form
or
may be more easily
handled than ju dv.
Integration by Transformation
may be useful when, in certain cases, particular
transformations of a given integral to one of a recognizable form suggest
themselves.
For example, a given integral involving such quantities as
Ju2_a2,
JiFT-2,
or
J2TF
may suggest appropriate trigonometric transformations such as, respectively,
u
=
a csc
8,
u
=
a tan
8,
or
u
=
a sin
8
Integration by Partial Fractions
is of assistance in the integration of rational
fractions. If
A B
ax+b
=-+-
ax+b
-
-
x2+px+q (x-a)(x-P) x--01 x-P
where A
+
B
=
a
AP
+
Ba
=
-b
and
A
and B are found by use of determinants (see “Algebra”), then
Alog(x-a)+Blog(x-P)+C
(ax
+
b)dx
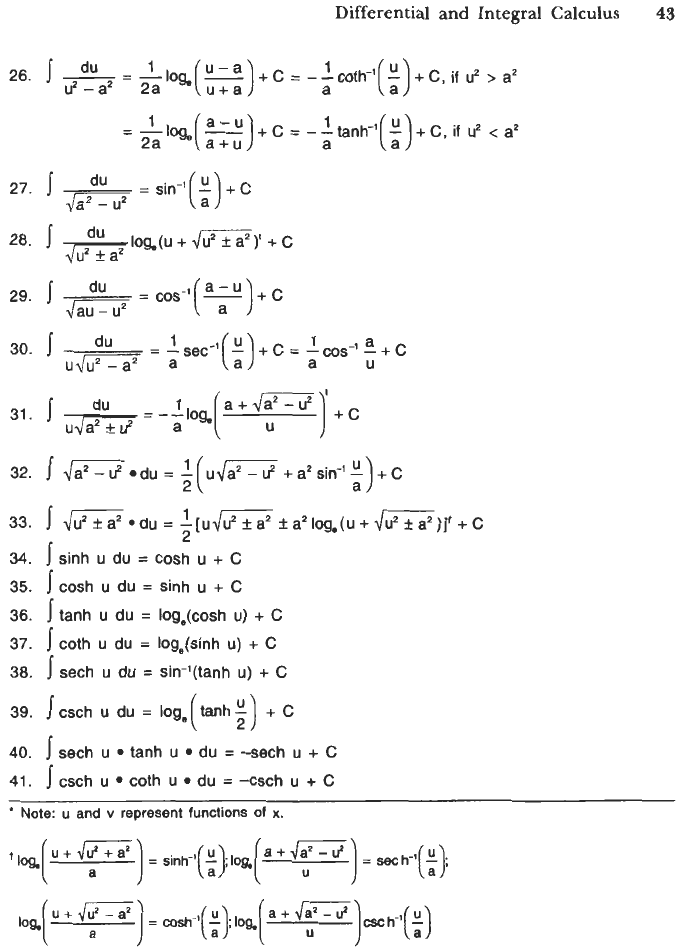
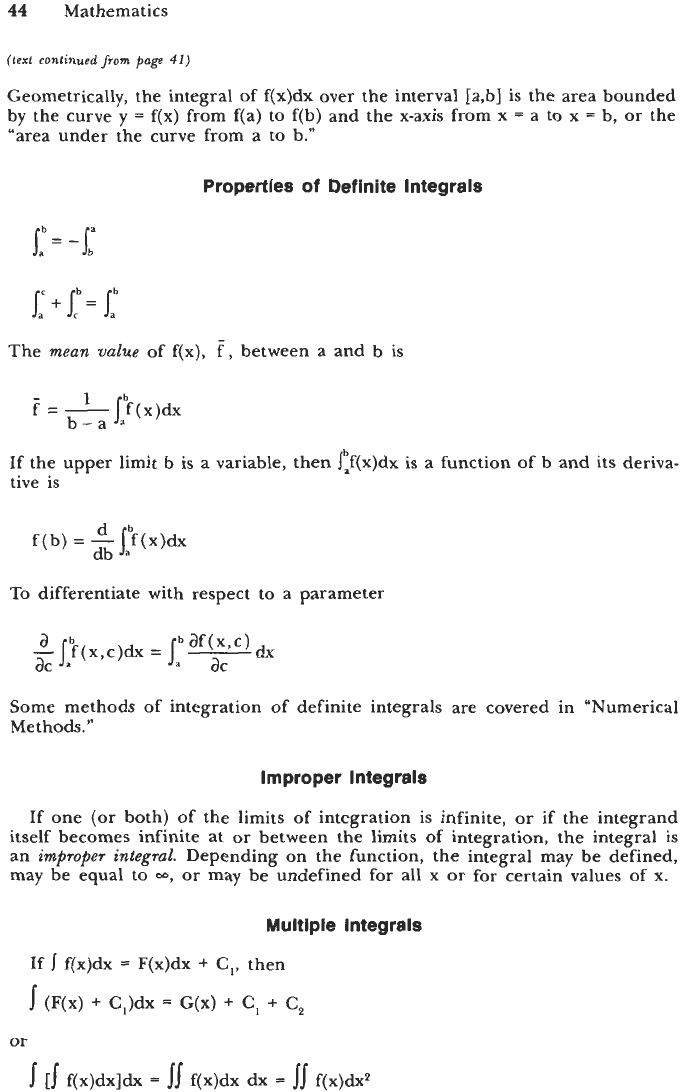
Integration by Tables
is possible if an integral may be put into a form that can
be found in a table of integrals, such as the one given in Table
1-7.
More
complete tables may be found in Bois, “Tables of Indefinite Integrals,” Dover,
and in others.
Definite integrals
The
Fundamental Theorem
of
Calculus
states that if f(x)
is
the derivative of F(x)
and if f(x)
is
continuous in the interval [a,b], then
ja?(x)dx
=
F(b)-F(a)
(fext continued on
page
44)

42
Mathematics
Table
1-7
Table
of
Integrals'
1.
1
df(x)
=
f(x)
+
C
2.
d f(x)dx
=
f(x)dx
3. jO*dx=C
4.
af(x)dx
=
a
f(x)dx
5. (u f v)dx
=
udx f vdx
6.
udv
=
uv
-
vdu
udv du
7.
dx
dx=uv-jv dx
8. f(y)dx
=
1
f(y)dy
3-
dx
U"+l
9.
u"du
=
n+l
+
C,
n
f
-1
10.
j
$
=
log$
+
c
11.
eUdu
=
e"
+
C
12. b"du
=
b"
+c
13.
./sin
u du
=
-cos
u
+
c
14.
jcos
u du
=
sin
u
+
c
15.
tan
u du
=
log, sec
u
+
C
=
-log, cos
u
+
c
16.
1
cot
u du
=
log, sin
u
+
C
=
-log, csc
u
+
c
17.
sec
u du
=
log,(sec
u
+
tan
u)
+
C
=
log, tan
[
+
t)
+
C
U
18.
csc
u du
=
log,(csc
u
-
cot
u)
+
C
=
log, tan
-
2
+
C
1 1
2
19.
sinz
u du
=
-
u
-
5
sin
u
cos
u
+
c
1 1
2
20.
cos2
u du
=
-
u
+
5
sin
u
cos
u
+
c
21.
22.
23.
24.
j
ctn2
u du
=
-cot
u
-
u
+
c
sec2
u du
=
tan
u
+
c
cscz
u du
=
-cot
u
+
C
tanz
u du
=
tan
u
-
u
+
C
du 1
U
u
+a
a
25.
j
=
2
tan-'
-
+
c
Differential and Integral Calculus
43
a
du
u-a
27.
A
=
sin-'
28.
du
lo&(u+dm)'+C
29.
j
=
cos-'(~]+C
30.
@=iF
7T=i-F
VGiTF
u~FT
a
=
-sec-'(:]+~
1
=
-cos-'a+~ 1
a
U
du
31.
du
=
-;IO&(
U
u a'
f
uz
dG
du
=
1
(
u~G
+
a'
sin-'
32.
33.
dG*du
=
1[udG*a210g,(u+dm)]'+C
34.
sinh
u du
=
cosh
u
+
C
35. jcosh
u du
=
sinh
u
+
C
+
C
2 a
I
2
36. tanh
u du
=
log,(cosh
u)
+
C
37.
I
coth
u du
=
loge(sinh
u)
+
C
38.
sech
u du
=
sin-l(tanh
u)
+
C
40.
41.
sech
u
tanh
u du
=
-sech
u
+
C
csch
u
coth
u du
=
-csch
u
+
C
'
Note:
u
and
v
represent functions
of
x.
44
Mathematics
(text
continued
from
page
41)
Geometrically, the integral of f(x)dx over the interval [a,b] is the area bounded
by the curve
y
=
f(x) from f(a) to f(b) and the x-axis from
x
=
a to x
=
b, or the
“area under the curve from a to b.”
Properties
of
Definite Integrals
I:
+
Ip
=
jab
The
mean value
of f(x),
T,
between a and b is
f=-
b-a
If the upper limit b is a variable, then l:f(x)dx is a function of b and its deriva-
tive is
f(b)
=
-jf(x)dx db
db
a
To
differentiate with respect to a parameter
Some methods of integration of definite integrals are covered in “Numerical
Methods.”
Improper Integrals
If one
(or
both) of the limits of integration is infinite,
or
if the integrand
itself becomes infinite at
or
between the limits of integration, the integral
is
an
improper integral.
Depending on the function, the integral may be defined,
may be equal to
-=,
or may be undefined for all x
or
for certain values
of
x.
Multiple Integrals
If
f(x)dx
=
F(x)dx
+
C,, then
