Lyons W.C. (ed.). Standard handbook of petroleum and natural gas engineering.2001- Volume 1
Подождите немного. Документ загружается.

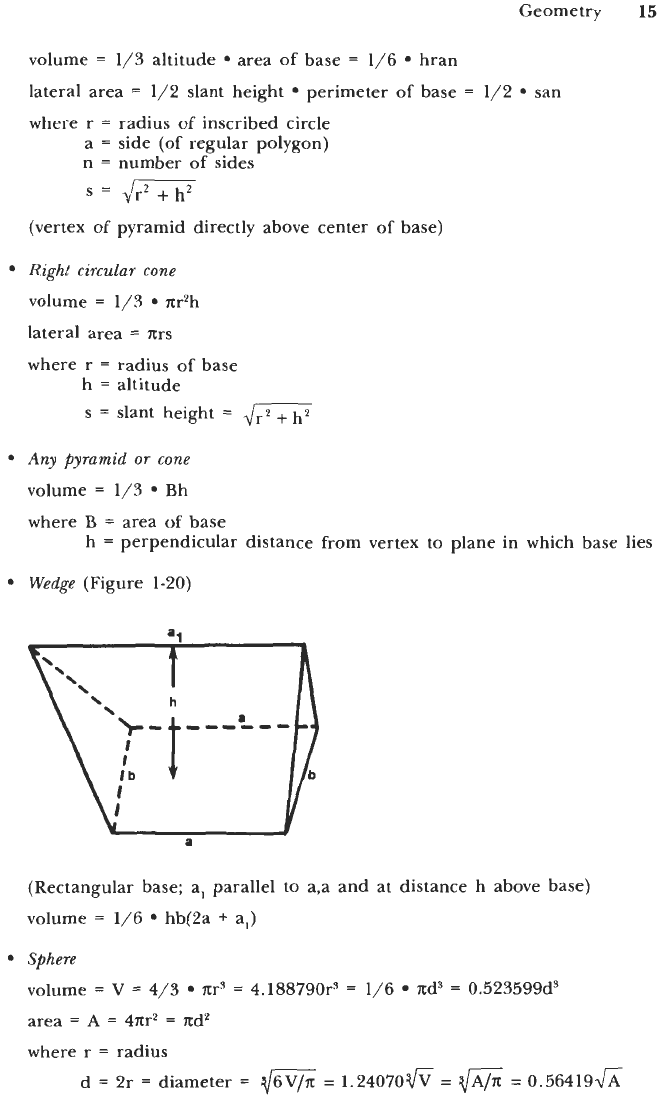
Geometry
15
volume
=
1/3
altitude area of base
=
1/6
hran
lateral area
=
1/2 slant height perimeter
of
base
=
1/2 san
where r
=
radius of inscribed circle
a
=
side
(of
regular polygon)
n
=
number of sides
s
=
JTTi7
(vertex
of
pyramid directly above center
of
base)
Right
circular cone
volume
=
1/3 zr2h
lateral area
=
zrs
where r
=
radius
of
base
h
=
altitude
s
=
slant height
=
dm
Any pyramid
or
cone
volume
=
1/3
Bh
where
B
=
area
of
base
h
=
perpendicular distance from vertex to plane in which base lies
Wedge
(Figure
1-20)
a.
(Rectangular base; a, parallel to a,a and at distance h above base)
volume
=
1/6 hb(2a
+
a,)
Sphere
volume
=
V
=
4/3 zrJ
=
4.188790r5
=
1/6 zd3
=
0.523599d’
area
=
A
=
4zr2
=
nd2
where r
=
radius
d
=
2r
=
diameter
=
=
1.240706
=
=
0.56419fi
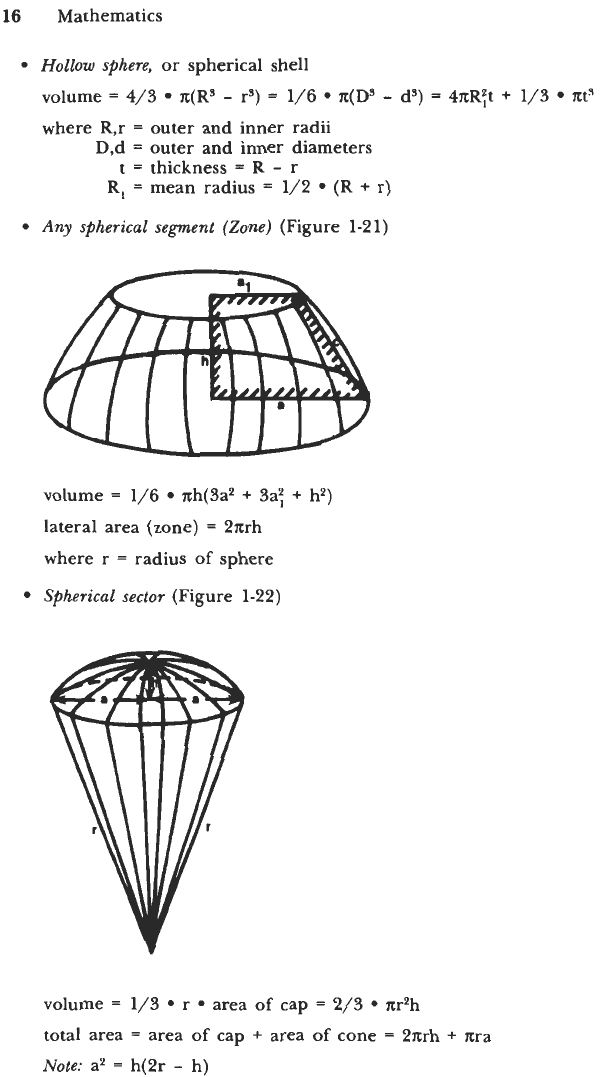
16
Mathematics
9
Hollow
sphere,
or spherical shell
volume
=
4/3
z(R3
-
r3)
=
1/6 x(D3
-
d3)
=
4xR;t
+
1/3
ztq
where R,r
=
outer and inner radii
D,d
=
outer and inner diameters
t
=
thickness
=
R
-
r
R,
=
mean radius
=
1/2
(R
+
r)
Any
spherical segment (Zone)
(Figure 1-21)
volume
=
1/6 sch(3a2
+
3a:
+
h2)
lateral area (zone)
=
2nrh
where r
=
radius
of
sphere
Spherical sector
(Figure 1-22)
volume
=
1/3 r area
of
cap
=
2/3 m2h
total area
=
area
of
cap
+
area
of
cone
=
2xrh
+
ma
Note:
as
=
h(2r
-
h)
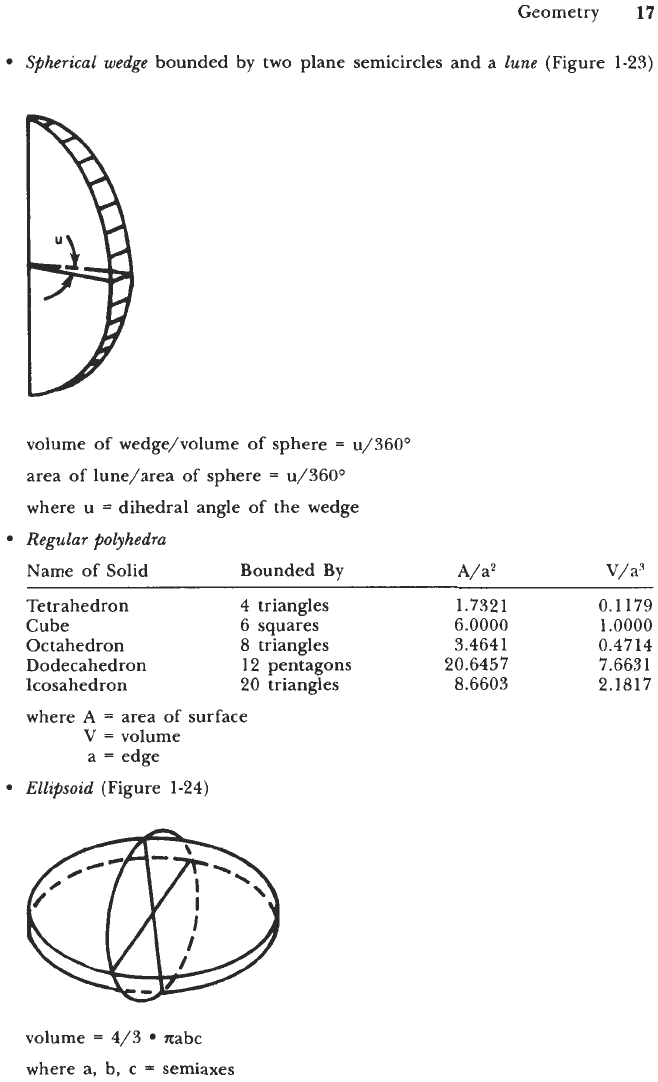
Geometry
17
Spherical wedge
bounded by two plane semicircles and a
lune
(Figure 1-23)
volume of wedge/volume of sphere
=
u/36Oo
area of lune/area of sphere
=
u/360°
where u
=
dihedral angle of the wedge
Regular polyhedra
Name of Solid
Bounded By
A/
a2
V/aS
Cube
6 squares 6.0000 1.0000
Tetrahedron 4 triangles 1.732 1 0.1179
Octahedron
8
triangles
3.4641 0.4714
Dodecahedron
12 pentagons
20.6457 7.6631
Icosahedron
20 triangles
8.6603 2.1817
where
A
=
area of surface
V
=
volume
a
=
edge
Ellipsoid
(Figure 1-24)
volume
=
4/3 xabc
where a, b, c
*
semiaxes

18
Mathematics
Spheroid
(or
ellipsoid of revolution)
By
the prismoidal formula:
volume
=
1/6
h(A
+
B
+
4M)
where h
=
altitude
A
and
B
=
areas of bases
M
=
area
of
a plane section midway between the bases
Paraboloid
of
revolution
(Figure 1-25)
0
#--
7--,
/
\
#
volume
=
1/2 nr2h
=
1/2 volume of circumscribed cylinder
Torus,
or
anchor
ring
(Figure 1-26)
volume
=
2n2cr2
area
=
4nr2cr (proof by theorems of Pappus)
ALGEBRA
See References
1
and 4 for additional information.
Operator Precedence and Notation
Operations in a given equation are performed in decreasing order of prece-
dence as follows:
Algebra
19
1.
Exponentiation
2.
Multiplication or division
3. Addition or subtraction
from left to right unless this order is changed by the insertion of parentheses.
For example:
a
+
b c
-
d3/e
will be operated upon (calculated) as if it were written
a
+
(b c)
-
[(ds)/e]
The symbol
I
a
I
means “the absolute value of a,”
or
the numerical value
of
a
regardless of sign,
so
that
1-21
=
121
=
2
n! means “n factorial” (where n is a whole number) and is the product
of
the whole numbers
1
to n inclusive,
so
that
4!
=
1
2
3
4
=
24
The notation for the sum of any real numbers a,, a2,
.
. .
,
an
is
and for their product
The notation
‘‘x
=
y”
is read
“x
varies directly with
y”
or
‘‘x
is
directly
proportional to y,” meaning x
=
ky
where k is some constant.
If
x
=
l/y, then
x
is inversely proportional to
y
and x
=
k/y.
Rules
of
Addition
a+b=b+a
(a
+
b)
+
c
=
a
+
(b
+
c)
a
-
(-b)
=
a
+
b and
a
-
(x
-
y
+
z)
=a
-
x
+
y
-
z
(i.e., a minus sign preceding a pair
of
parentheses operates to reverse the signs
of
each term within, if the parentheses are removed)

20
Mathematics
Rules
of
Multiplication and Simple Factoring
a*b=b*a
(ab)c
=
a(bc)
a(b
+
c)
=
ab
+
ac
a(-b)
=
-ab and -a(- b)
=
ab
(a
+
b)(a
-
b)
=
a2
-
b2
(a
+
b)2
=
a2
+
2ab
+
b2
and
(a
-
b)2
=
a2
-
2ab
+
b2
(a
+
b)s
=
a3
+
3a2
+
3ab2
+
bS
and
(a
-
b)s
=
a3
-
3a2
+
3ab2
-
bs
(For higher order polynomials see the section “Binomial Theorem”)
a”
+
b” is factorable by (a
+
b) if n is odd, thus
a3
+
b”
=
(a
+
b)(a2
-
ab
+
b2)
and a”
-
b”
is
factorable by (a
-
b), thus
a”
-
b”
=
(a
-
b)(a”-’
+
a”- 2b
+
. .
.
+
abn-2
+
bn-1
1
Fractions
The numerator and denominator of a fraction may be multiplied or divided
by any quantity (other than zero) without altering the value of the fraction, so
that,
if
m
#
0,
ma+mb+mc
-
a+b+c
mx+my
X+Y
-
To add fractions, transform each to a common denominator and add the
numerators (b,y
#
0):
To multiply fractions (denominators
#
0):

Algebra
21
a
ax
-ox=-
b b
axc axc
b
y
z
byz
-*-*-=-
To
divide one fraction by another, invert the divisor and multiply:
Exponents
a"
=
1
(a
#
0)
and a'
=
a
a-"'
=
l/am
avn
=
and amIn
=
(ab)"
=
a"b"
(a/b)"
=
a"/b"
Except in simple cases (square and cube roots) radical signs are replaced by
fractional exponents.
If
n is odd,
but
if
n is even, the nth root of -a is imaginary.
Logarithms
The logarithm
of
a positive number
N
is the power to which the base (10
or
e) must be raised to produce
N.
So,
x
=
logeN means that ex
=
N,
and x
=
log,,N
means that
10"
=
N.
Logarithms to the base
10,
frequently used in numerical
computation, are called
common
or
denary logarithms,
and those
to
base e, used
in theoretical work, are called
natural logarithms
and frequently notated as
In.
In either case,
log(ab)
=
log a
+
log
b

22
Mathematics
log(a/b)
=
log a
-
log b
log(l/n)
=
-log n
log(a")
=
n log a
log,(b)
=
1,
where b is either 10 or e
log
0
=
-03
log 1
=
0
log,,e
=
M
=
0.4342944819
. .
.
,
so
for conversion
loglox
=
0.4343 logex
and since 1/M
=
2.302585, for conversion (In
=
loge)
In x
=
2.3026 loglox
Binomial Theorem
Let
n,
=
n
n(n
-
1)
n2
=
-
2!
n(n
-
l)(n
-
2)
3!
n3
=
etc. Then for any n, 1x1
<
1,
(1
+
x)"
=
1
+
n,x
+
n2x2
+
ngx3
+
. . .
If n
is
a positive integer, the system is valid without restriction on x and
completes with the term n,x".
Some of the more useful special cases are the following
[l]:
...
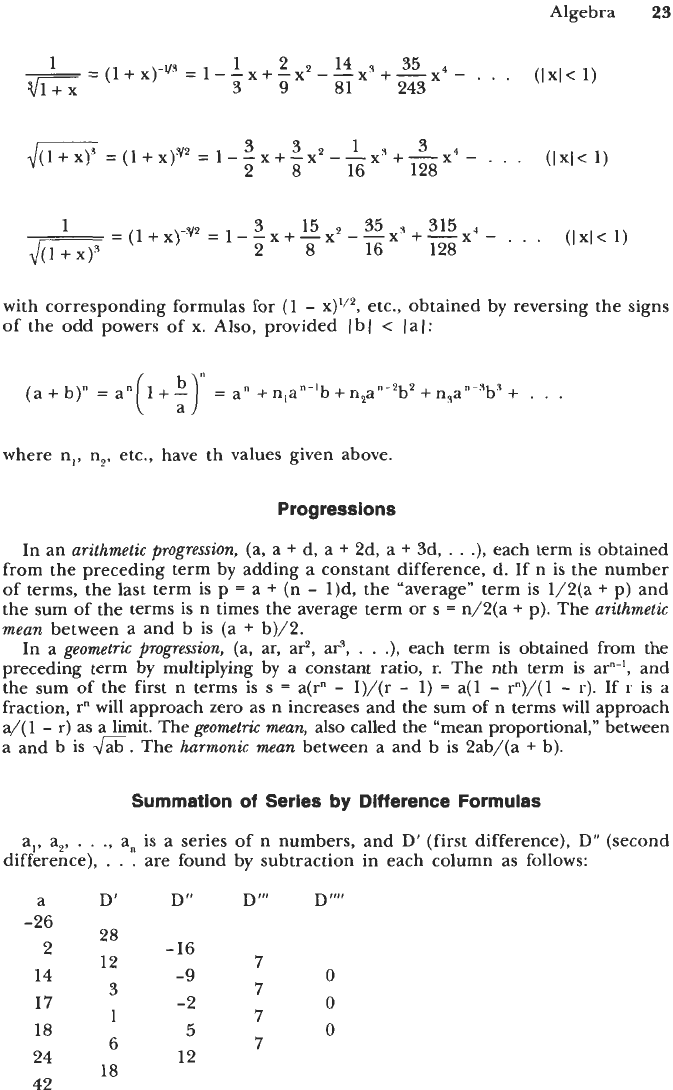
(Ixl<
1)
11
1
5
2 8 16 128
Jl+X=(l+x)'/Z =1+-x--x~+-xxJ--x4+
...
(Ixl< 1)
G'Y
=
(1
+
x)q3
=
1
+-x--x2
--x3
--x4
+
11
5
10
3 9 81 243
(Ixl<
1)
--
-(1+x)-'=1-x+x2-xxJ+x4-
. .
.
l+x
Algebra
23
. . .
(Ixl<
1)
1 (l+x)-Y* =1--x+-x2--xxJ+-x4 1 2 14 35
-
G=
3
9
81 243
33 1
3
~~=(1+X)"=l--x+-x~--xxJ+-x4-.
2 8 16 128
. .
(Ixl< 1)
with corresponding formulas for (1
-
x)'", etc., obtained by reversing the signs
of the odd powers of x.
Also,
provided lbl
<
(a(:
(a+b)" =an(l+$)"
=a"+nla"-'b+n2a"-2b2+n,a"-'bb"+
.
..
where nl, n2, etc., have th values given above.
Progressions
In an
arithmetic progression,
(a, a
+
d, a
+
2d, a
+
3d,
. .
.),
each term is obtained
from the preceding term by adding a constant difference, d. If n is the number
of terms, the last term is p
=
a
+
(n
-
l)d, the "average" term is 1/2(a
+
p) and
the sum of the terms is n times the average term
or
s
=
n/2(a
+
p). The
arithmetic
mean
between a and b is (a
+
b)/2.
In a
geometric progression,
(a, ar, ar2,
ar',
. .
.),
each term is obtained from the
preceding term by multiplying
by
a constant ratio, r. The nth term
is
a?', and
the sum of the first n terms is
s
=
a(r"
-
l)/(r
-
1)
=
a(l
-
rn)/(l
-
r). If
r
is a
fraction,
r"
will approach zero as n increases and the sum of
n
terms will approach
a/( 1
-
r)
as a limit. The
geometric mean,
also called the "mean proportional," between
a and b is
Jab.
The
harmonic mean
between a and b is 2ab/(a
+
b).
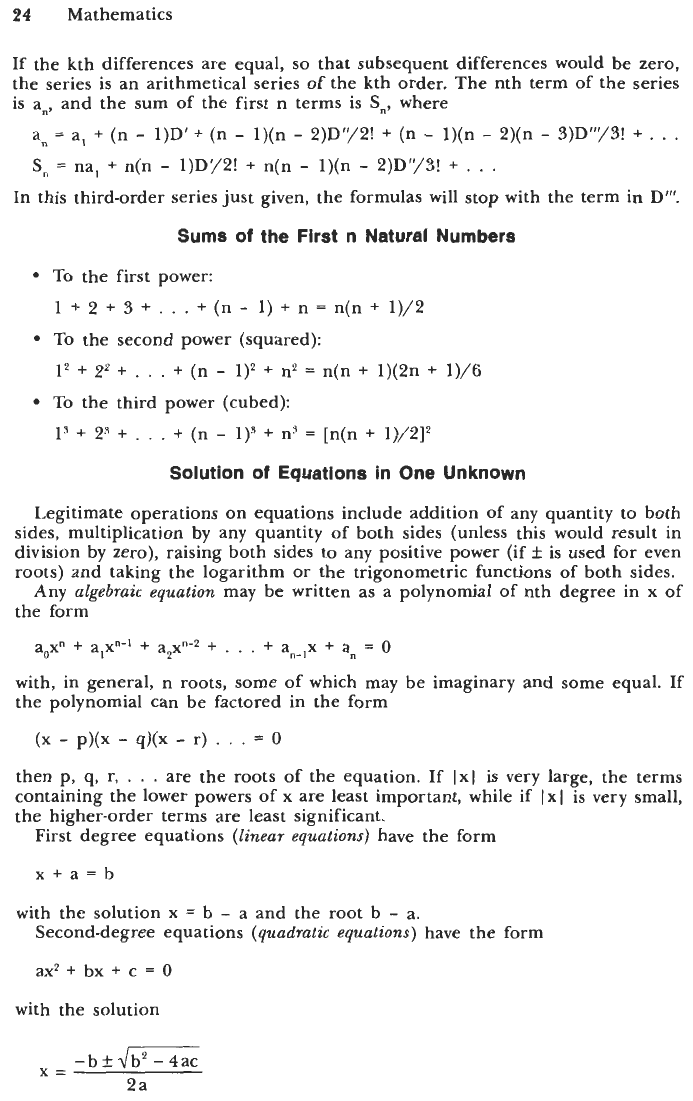
Summation of Series
by
Difference Formulas
a,, a2,
. .
.,
an is a series of n numbers, and
D'
(first difference),
D"
(second
difference),
. . .
are found by subtraction in each column as follows:
a
D'
D"
D"' D""
-26
2
14
17
18
24
42
28
12
3
1
6
18
-16
-9
-2
5
12
0
0
0
24
Mathematics
If the kth differences are equal,
so
that subsequent differences would be zero,
the series is an arithmetical series of the kth order. The nth term of the series
is an, and the sum of the first n terms is
Sn,
where
an
=
a,
+
(n
-
1)D'
+
(n
-
l)(n
-
2)D'y2!
+
(n
-
l)(n
-
2)(n
-
3)D'"/3!
+
. . .
Sn
=
na,
+
n(n
-
1)D'/2!
+
n(n
-
l)(n
-
2)DfY3!
+
. . .
In
this third-order series just given, the formulas will stop with the term in D"'.
Sums of the First n Natural Numbers
To
the first power:
1
+
2
+
3
+.
. .
+
(n
-
1)
+
n
=
n(n
+
1)/2
To
the second power (squared):
l2
+
22
+
. . . +
(n
-
1)*
+
n2
=
n(n
+
1)(2n
+
1)/6
To
the third power (cubed):
1'
+
2'
+ .
.
. +
(n
-
1)'
+
nJ
=
[n(n
+
1)/212
Solution
of
Equations in One Unknown
Legitimate operations on equations include addition of any quantity to both
sides, multiplication by any quantity of both sides (unless this would result in
division by zero), raising both sides to any positive power
(if
k
is used for even
roots) and taking the logarithm or the trigonometric functions of both sides.
Any
algebraic equation
may be written as a polynomial of nth degree in x of
the form
aOxn
+
a,x""
+
a2xn-2
+ . . .
+
an_,x
+
an
=
0
with, in general, n roots, some of which may be imaginary and some equal. If
the polynomial can be factored in the form
(x
-
p)(x
-
q)(x
-
r)
.
. .
=
0
then p,
q,
r,
. . .
are the roots of the equation. If 1x1 is very large, the terms
containing the lower powers of x are least important, while
if
1x1 is very small,
the higher-order terms are least significant.
First degree equations
(linear equations)
have the form
x+a=b
with the solution x
=
b
-
a and the root b
-
a.
Second-degree equations
(quadratic equations)
have the form
ax2
+
bx
+
c
=
0
with the solution
-b+Jb2-4ac
2a
x=
