Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.


best.
Pontgryagin
[1966]
is
also
a
very
good
book.
Helgason
[1962]
is
a
detailed
treatment
of
the
differential
geometry
of
the
group
spaces.
A
topological
group
G
is
a
group
which
is
also
endowed
with
the
structure
of
a
topological
space
and
the
map
~
:
GxG
into
G
defined
by
~(x,y)=
xy"
1
is
continuous.
Clearly,
the
multiplication
and
inverse
maps
defined
by
(x,y)
~
xy
and
x
~
x·
1
are
both
continuous.
For
example,
the
additive
group
of
real
numbers
is
a
topological
group,
and
the
group
of
invertible
nxn
real
matrices
is
also
a
topological
group.
Let
G
be
a
topological
group
and
X a
topological
space.
G
acts
on
X
to
the
left
if
there
is
a
continuous
map
~
: G x
X~
X
and
we
write
~(g,x)=
gx
such
that:
(i)
~(g,
~(h,x))=
~(gh,x)
or
(gh)x
=
g(hx)
for
all
g,h
€
G,
x € X
and
(ii)
if
e € G
is
the
identity
element
in
G,
and
x €
X,
~(e,x)
=
ex
=
x.
Sometimes
G
is
called
a
left
transformation
group.
Note,
a
right
action
would
be
defined
by
a map T : X x G
~
X
with
the
appropriate
properties.
Given
an
action
~
: G x X
~
X
and
a
set
S
!:::
X,
then
G
5
=
{g
€
Gl
~(g,y)=
y
for
ally
€
S}.
If
Gx
=
{e),
i.e.,
only
the
identity
element
leaves
X
fixed,
we
say
the
action
is
effective.
If
x,
y £
X,
there
is
some g £ G
~
~(g,x)
=
y,
then
we
say
the
action
is
transitive.
A
trivial
example
of
a
transitive
action
which
is
not
effective,
is
when G
is
nontrivial
but
x
is
a
single
point.
Of
course,
if
G =
{e),
then
G
is
effective
on
any
X.
Given
an
action
~
: G x X
~
X
and
a
subgroup
H
!:::
G,
then
there
is
an
induced
action
~"
: H x X
~
X
defined
by
~(h,x)
where
h €
H.
If
~
is
effective,
so
is
~"'
and
if
~"
is
transitive,
so
is
~·
Let
X
be
a
Hausdorff
space,
~
: G x X
~
X a
transitive
action.
Fix
some X
0
€
X,
and
let
H = {g €
Gl
~(g,x
0
)
= X
0
},
the
isotropy
group
of
x
0
,
then
H
is
a
closed
subgroup
of
G.
The
coset
space,
G/H,
is
defined
to
be
the
quotient
space
of
G
by
the
equivalence
relation
g . h
iff
g"
1
h £
H.
G/H
is
Hausdorff
because
the
mapping
~
: G
~
G/H
is
continuous
and
74

open.
If
G/H
is
compact,
then
the
map G/H
~
X
is
a
homeomorphism.
If
H
is
a
closed
subgroup
of
G,
then
G
acts
transitively
on
G/H. A
space
with
a
transitive
group
of
operators
is
called
homogeneous,
such
as
G/H
for
H
being
a
closed
subgroup
of
G.
For
example,
the
action
of
O(n),
the
group
of
nxn
orthogonal
matrices,
on
sn·l.
The
subgroup
of
O(n)
which
leaves
a
unit
vector
v =
(1,0,
••
,0)
fixed
is
isomorphic
to
O(n-1).
The
coset
space
is
O(n)/O(n-1)
= sn·l
which
is
a
homogeneous
space.
More
generally,
a
Stiefel
manifold
vm,k
is
defined
to
be
O(m)/O(k).
Thus,
V.,.
1
,m
=
sm
[Chevalley
1946,
Steenrod
1951].
A
Lie
group
G
is
a
differentiable
(or
analytic)
manifold
and
also
endowed
with
a
group
structure
such
that
~
G X G
~
G
defined
by
(g,h)
~
gh"
1
,
where
g,h
e
G,
is
c!"
(or
analytic).
For
example:
(i)
Rn
is
a
Lie
group
under
vector
addition.
(ii)
The
manifold
GL(n,R)
of
all
nxn
non-singular
real
matrices
is
a
Lie
group
under
matrix
multiplication.
(iii)
The
product
G X H
of
two
Lie
groups
is
itself
a
Lie
group
with
the
product
manifold
structure
and
the
direct
product
group
structure,
i.e.,
(g
1
,h
1
)
(g
2
,h
2
)
=
(g
1
g
2
,
(h
1
h
2
) "
1
)
where
g
1
,g
2
e
G,
h
1
,h
2
e
H.
(iv)
The
unit
circle
s
1
is
a
Lie
group
with
the
addition
of
angles.
(v)
The
n-torus
~
(n
an
integer
> 0)
is
the
Lie
group
which
is
the
product
of
the
Lie
group
s
1
with
itself
n
times.
If
f
is
c!",
then
we
may
be
able
to
extend
Taylor's
formula
into
a
convergent
series.
If
we
can,
then
f
is
said
to
be
analytic.
A
standard
example
of
a
c!"
function
which
is
not
analytic
is
the
following.
exp
{-1/(1-
lxl•)),
~(X)
= I
0,
75
lxl
lxl
<
1,
~
1.

-1
Let
g £ G.
Left
translation
by
g
and
right
translation
~
are
the
diffeomorphisms
1
9
and
r
9
of
G
defined
by
1
9
(h)
=
gh,
r
9
(h)
=
hg
for
all
h £ G.
If
U
is
a
subset
of
G,
we
denote
1
9
(U)
and
r
9
(U)
by
gU
and
Ug
respectively.
A
vector
field
X
on
G
is
called
left
invariant
if
for
each
g £ G, X
is
1
9
-related
to
itself,
i.e.,
if
1
9
:
G
~
G
dl
9
:
Gh
~
G
9
h,
the
tangent
space
of
G
dl
9
• X = X · 1
9
•
Clearly,
the
translation
map
gives
a
prescribed
way
of
translating
the
tangent
space
at
one
point
to
the
tangent
space
at
another
point
on
a
Lie
group
G.
We
shall
come
back
to
this
later
after
we
have
introduced
the
concept
of
tangent
bundles
and
fiber
bundles.
Recall
that
we
have
defined
the
tangent
vector
fields
as
derivatives
and
the
bracket
operation
of
two
vector
fields
earlier,
now
we
shall
use
these
concepts
to
define
the
Lie
algebra.
A
Lie
algebra
g
over
R
is
a
real
vector
space
g
together
with
a
bilinear
bracket
operator
[ , ] : g x q
~
q
such
that
for
all
X, Y, z £
q,
(i)
the
bracket
is
anti-commuting,
i.e.,
[X,Y]
-[Y,X],
(ii)
the
Jacobian
identity
is
satisfied,
i.e.,
[[X,Y],Z]
+
[[Y,Z],X]
+
[[Z,X],Y]
=
0.
The
importance
of
the
concept
of
Lie
algebra
is
its
intimate
association
with
a
Lie
group.
For
instance,
the
connected,
simply
connected
Lie
group
are
completely
determined
(up
to
isomorphism)
by
their
Lie
algebras.
Thus
the
study
of
these
Lie
groups
reduces
in
large
part
to
the
study
of
their
Lie
algebras.
Examples
of
Lie
algebra:
76

(i)
The
vector
space
of
all
smooth
vector
fields
on
the
manifold
M
forms
a
Lie
algebra
under
the
bracket
operation
on
vector
fields.
(ii)
Any
vector
space
becomes
a
Lie
algebra
if
all
brackets
are
set
equal
to
o.
Such
a
Lie
algebra
is
called
abelian.
(iii)
The
vector
space
gl(n,R)
of
all
nxn
matrices
form
a
Lie
algebra
if
we
set
[A,B]
=
AB
-
BA
where
A,
B e
gl(n,R).
(iv)
R
3
with
the
bilinear
operatioon
X x Y
of
the
vector
cross
product
is
a
Lie
algebra.
Theorem
2.6.1
Let
G
be
a
Lie
group
and
g
its
set
of
left
invariant
vector
fields.
Then,
(i)
g
is
a
real
vector
space,
and
the
map
a : g
~
Ge
defined
by
a(X)=
X(e)
is
an
isomorphism
of
g
with
the
tangent
space
Ge
of
G
at
the
identity.
Thus,
dim
g =
dim
Ge
dim
G.
(ii)
Left
invariant
vector
fields
are
smooth.
(iii)
The
Lie
bracket
of
two
left
invariant
vector
fields
is
itself
a
left
invariant
vector
field.
(iv)
g
forms
a
Lie
algebra
under
the
Lie
bracket
operation
on
vector
fields.
It
should
be
noted
that
the
correspondence
between
Lie
groups
and
Lie
algebras
of
the
above
theorem
is
not
unique.
For
instance,
all
1-dim
Lie
groups
such
as
s
1
or
R
1
have
the
same
Lie
algebra.
Similarly,
the
plane
R
2
,
torus
T
2
,
and
cylinder
s
1
xR
1
all
have
the
same
abelian
Lie
algebra.
It
is
not
difficult
to
see
that
two
Lie
groups
that
have
isomorphic
Lie
algebra
are
themselves
isomorphic
at
least
in
a
sufficiently
small
neighborhood
of
their
identity
elements.
Such
a
notion
can
be
formulated
more
precisely
in
terms
of
a
local
Lie
group
in
a
neighborhood
of
e.
Then
the
correspondence
between
such
local
Lie
groups
and
Lie
algebras
is
indeed
one-to-one
and
onto.
We
have
Theorem
2.6.2:
Two
Lie
groups
are
locally
isomorphic
iff
their
Lie
algebras
are
isomorphic.
Let
us
now
consider
the
subgroups
and
subalgebras
of
a
77

given
Lie
group
and
its
algebra.
Let
G
be
a
Lie
group.
A
subgroup
H
~
G
is
a
Lie
subgroup
of
G
if
the
set
of
elements
of
H
is
a
submanifold
of
the
smooth
manifold
G.
Let
g
be
a
Lie
algebra.
h
~
g
is
a
Lie
subalgebra
of
g
if
(i)
h
is
a
vector
subspace
of
g,
and
(ii)
if
X,Y
E
h,
then
[X,Y]
E
h.
A
Lie
subgroup
is
clearly
a
Lie
group
with
the
structure
inherited
from
the
bigger
group.
A
Lie
subgroup
is
also
closed.
Conversely,
it
is
known
that
a
closed
subgroup
of
a
Lie
group
is
a
Lie
subgroup.
It
is
obvious
that
if
g
is
an
abelian
Lie
algebra,
then
any
vector
subspace
of
g
is
a
Lie
subalgebra.
Clearly,
any
one-
dimensional
subspace
of
a
Lie
algebra
is
a
Lie
subalgebra.
Theorem
2.6.3
(a)
Let
G
be
a
Lie
group
and
H a
Lie
subgroup.
Then
the
Lie
algebra
of
H,
h,
is
a
subalgebra
of
the
Lie
algebra
of
G,
g.
(b)
Let
G
be
a
Lie
group
with
Lie
algebra
g.
Suppose
h
is
a
Lie
subalgebra
of
g.
Then
there
is
a
Lie
group
H
whose
Lie
algebra
is
isomorphic
to
h
and
a
1-1
map
of
H
to
G.
Nonetheless,
the
image
of
H
and
G
need
not
be
a
submanifold
of
G,
thus
H
is
not
necessarily
a
Lie
subgroup
of
G.
Theorem
2.6.4
A
closed
subgroup
of
a
Lie
group
is
a
Lie
subgroup.
From
the
idea
of
the
imbedding
theorem,
one
may
be
tempted
to
think
that
any
given
Lie
group
is
isomorphic
to
a
Lie
subgroup
of
GL(n,R)
for
sufficiently
large
n.
But
this
is
not
to
be
the
case.
Certainly,
there
are
Lie
groups
with
the
same
Lie
algebra
which
are
mapped
to
some
GL(n,R)
by
a
one-to-one
map
(Theorem
2.5.3(b)),
moreover,
the
image
need
not
be
a
Lie
subgroup
as
pointed
out
earlier.
Don't
despair,
as
a
corollary
of
a
famous
theorem
of
Peter
and
Weyl[1927]
for
representations
of
Lie
groups,
one
is
assured
that
a
compact
Lie
group
is
isomorphic
to
a
subgroup
of
some
GL(n,R)
for
sufficiently
large
n.
By
using
a
corollary
of
the
duality
theorem
of
Tannaka
(Chevalley
78

1946,
Hochschild
1965)
and
the
reduction
theorem
of
Lie
groups,
one
can
sharpen
the
result
by
stating
that
a
compact
Lie
group
is
isomorphic
to
some
Lie
subgroup
of
O(k)
(see
also,
Chevalley
1946,
1951,
Hochschild
1965].
Let
G
be
a
Lie
group.
A
continuous
homomorphism a : G
~
GL(n,R),
for
some
positive
integer
n,
is
called
a
representation
of
G.
a
is
trivial
if
the
image
of
a
is
constantly
the
identity
matrix
and
a
is
faithful
if
a
is
one-to-one.
Roughly
speaking,
an
application
of
the
theorem
of
Peter
and
Weyl
asserts
that
compact
Lie
groups
always
have
at
least
one
faithful
representation.
The
theory
of
representation
is
an
interesting
subfield
of
mathematics,
for
those
who
are
interested
in
this
subject,
the
theory
of
characters,
orthogonality
relations,
theorem
of
Peter
and
Weyl,
can
be
found
in
[Chevalley
1951,
Pontryagin
1966].
There
are
many
important
applications
of
representation
theory
in
physics
such
as
in
quantum
mechanics,
atomic
spectra,
solid
state
physics,
nuclear
and
particle
physics
[Bargmann
1970,
Hamermesh
1962,
Hermann
1966,
Am.
Math.
Soc.
Translation
Ser.
1,
Vol
9,
1962].
We
shall
not
go
into
this
any
further.
Let
us
take
a
closer
look
at
closed
subgroups
of
Lie
groups.
As a
preliminary,
we
shall
discuss
the
exponential
map
which
is
a
generalization
of
the
power
series
for
ex
where
x
is
a
matrix.
Let
G
be
a
Lie
group,
and
let
g
be
its
Lie
algebra.
A
homomorphism
¢:
R
~
G
is
called
a
one-parameter
subarouo
of
g.
Let
X £
g,
then
ldjdt
~
lX
is
a homomorphism
of
the
Lie
algebra
of
R
into
g.
Since
the
real
line
is
simply-connected,
then
there
exists
a
unique
one-parameter
subgroup
expx : R
~
G
such
that
d
expx(ldjdt)
=
lX.
That
is,
t
~
expx(t)
is
the
unique
one-parameter
subgroup
of
G
whose
tangent
vector
at
0
is
X(e).
The
exponential
map
is
defined
as
exp:
g
~
G
by
setting
exp(X)
=
expx(1).
We
shall
show
that
the
exponential
map
for
the
GL(n,R)
is
actually
given
by
exponentiation
of
matrices.
79
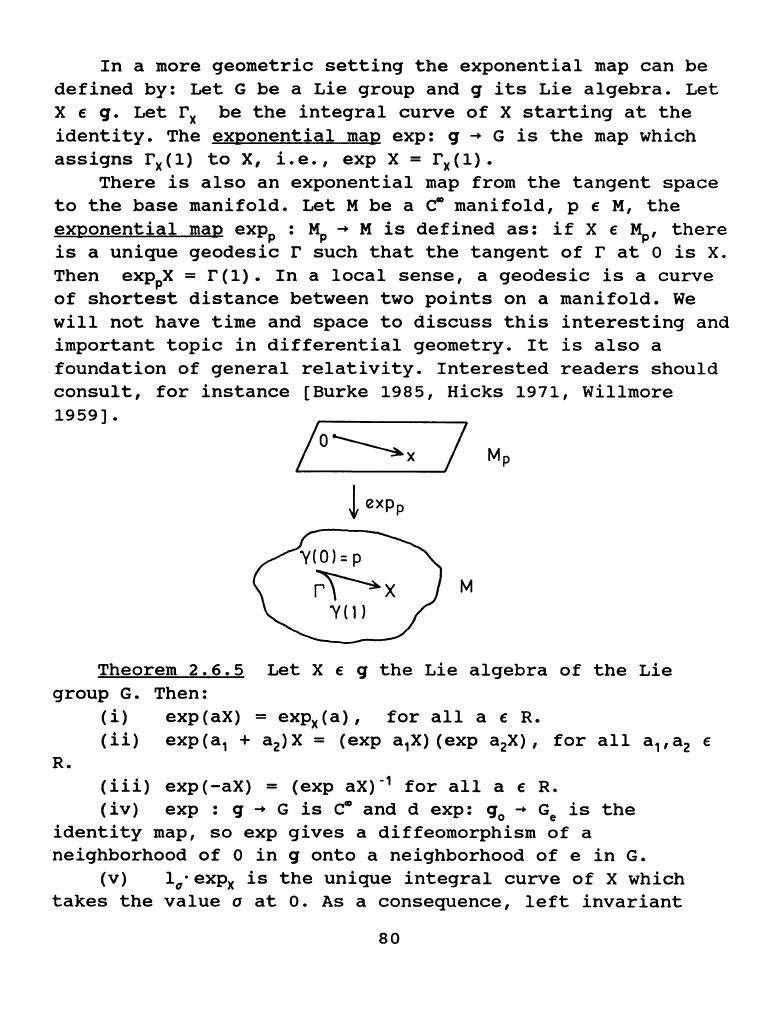
In
a
more
geometric
setting
the
exponential
map
can
be
defined
by:
Let
G
be
a
Lie
group
and
q
its
Lie
algebra.
Let
X £
q.
Let
rx
be
the
integral
curve
of
X
starting
at
the
identity.
The
exponential
map
exp:
q
~
G
is
the
map
which
assigns
rx(1)
to
X,
i.e.,
exp
X=
rx(1).
There
is
also
an
exponential
map
from
the
tangent
space
to
the
base
manifold.
Let
M
be
a
c-
manifold,
p £
M,
the
exponential
map expP :
~
~
M
is
defined
as:
if
X £
~,
there
is
a
unique
geodesic
r
such
that
the
tangent
of
r
at
0
is
X.
Then
expPX
=
r(1).
In
a
local
sense,
a
geodesic
is
a
curve
of
shortest
distance
between
two
points
on
a
manifold.
We
will
not
have
time
and
space
to
discuss
this
interesting
and
important
topic
in
differential
geometry.
It
is
also
a
foundation
of
general
relativity.
Interested
readers
should
consult,
for
instance
[Burke
1985,
Hicks
1971,
Willmore
1959].
M
Theorem
2.6.5
Let
X £ q
the
Lie
algebra
of
the
Lie
group
G.
Then:
(i)
exp(aX)
=
expx(a),
for
all
a£
R.
(ii)
exp(a
1
+ a
2
)X =
(exp
a
1
X)
(exp
a
2
X),
for
all
a
1
,a
2
£
R.
(iii)
exp(-aX)
=
(exp
aX)_,
for
all
a £ R.
(iv)
exp
: q
~
G
is
c-
and
d
exp:
q
0
~
Ge
is
the
identity
map,
so
exp
gives
a
diffeomorphism
of
a
neighborhood
of
0
in
q
onto
a
neighborhood
of
e
in
G.
(v)
lu·expx
is
the
unique
integral
curve
of
X
which
takes
the
value
a
at
o. As a
consequence,
left
invariant
80

vector
fields
are
always
complete.
(vi)
The
one-parameter
group
of
diffeomorphism
X
8
associated
with
the
left
invariant
vector
field
X
is
given
by
x.
= r exp
(8)
•
Theorem
2.6.6
Let~:
H
~
G
be
a
homomorphism,
then
the
following
diagram
commutes:
H - •
~
G
exp
t t
exp
h -
~
~
g
In
the
case
of
groups
of
matrices,
we
shall
see
that
the
exponential
map
exp
:
gl(n,R)
~
GL(n,R)
for
the
general
linear
group
is
given
by
exponentiation
of
matrices.
Let
I
(rather
than
e)
denote
the
identity
matrix
in
GL(n,R),
let
eA
=I+
A+
A
2
/2!
+
•.•
+ Ak/k! +
••..
(2.5-1)
for
A E
gl(n,R).
We
want
to
show
that
the
right
hand
side
of
the
series
converges.
In
fact,
the
series
converges
uniformly
for
A
in
a
bounded
region
of
gl(n,R).
For
a
given
bounded
region
n
of
gl(n,R),
there
is
a~>
o
such
that
for
any
matrix
A
inn,
IAiil 5
~
for
each
element
(or
component)
of
A.
It
follows
by
induction
that
I
(Ak)
ij
I 5
n<k-l>~k.
Then
by
the
Weierstrass
M-
test,
each
of
the
series
of
components
'Ek=O"'
(Ak);/k!,
(1
5
i,
j 5 n)
(2.5-2)
converges
uniformly
for
A
in
n.
Thus
the
series
in
(2.5.1)
converges
uniformly
for
A
in
n.
Let
Sk(A)
be
the
k-th
partial
sum
of
the
series
(2.5.1),
i.e.,
Sk(A)=
I+
A+
A
2
/2!
+
••••
+ Ak/k!
(2.5-3)
and
let
B,C
E
gl(n,R).
Since
c
~
BC
is
a
continuous
map
of
gl(n,R)
into
itself,
it
follows
that
B (limk..., Sk(A)) = limk...,
(BSk(A)).
In
particular,
if
B
is
non-singular,
then
B (limk..., Sk(A))
B-
1
= limk..., BSk(A)B-
1
•
Because
BAkB-
1
=
(BAB-
1
)k,
it
then
follows
that
BeAB-
1
= e
8
A
8
•
From
this,
it
is
easy
to
show
that
if
the
eigenvalues
of
A
are
A.
1
, • • •
A.n,
then
the
eigenvalues
of
eA
are
e
1
•
,
••
, e
1
'!
e
1
•
is
an
eigenvalue
of
eA
follows
easily
if
the
upper
left
81

corner
of
A
is
l
1
and
the
rest
of
the
first
column
are
zero.
Likewise
for
the
others.
The
determinant
of
eA
is
e
raised
to
the
trace
of
A,
by
observing
that
the
determinant
is
the
product
of
the
eigenvalues
and
the
trace
is
the
sum
of
the
eigenvalues.
eA
is
always
non-singular.
If
A
and
B
commute,
then
eA+B
=
eAeB.
Consider
the
map
cpA
: t
-+
tA
of
R
into
GL(n,R).
It
is
smooth
because
etA
can
be
represented
by
a
power
series
in
t
with
infinite
radii
of
convergence.
Its
tangent
vector
at
0
is
A
(simply
differentiate
the
power
series
term
by
term),
and
this
map
is
a
homomorphism,
because
cpA
(t)
= etA,
cpA(t,
+
tz)
=
e<t,•t,..JA
=
et,A+t].A
= cpA(t,)cpA(tz),
etc.
Thus
cpA
: t
-+
etA
is
the
unique
one-parameter
subgroup
of
GL(n,R)
whose
tangent
vector
at
0
is
A.
So
the
exponential
map
exp:
ql(n,R)
-+
Gl(n,R)
is
given
by
exponentiation
of
matrices:
exp(A)
=
cpA(l)
=
eA
where
A E
ql(n,R).
Thus
this
is
the
historical
justification
for
the
terminology.
It
should
be
pointed
out
that
exp
: q
-+
G
need
not
be
onto
even
when G
is
connected.
An
example
for
ql(2,R)
has
been
constructed
to
demonstrate
this
[Kahn
1980,
p.272].
2.7
Fiber
bundles
Fiber
bundles
provide
a
convenient
framework
for
discussing
the
concepts
of
relativity,
invariance,
gauge
transformations
and
group
representations.
Fiber
bundles
were
originally
introduced
in
order
to
formulate
and
solve
global
topological
problems.
Nonetheless,
the
notion
of
a
fiber
bundle
is
also
very
appropriate
for
local
problems
of
differential
geometry.
The
concept
of
induced
representation
of
Lie
groups,
which
is
important
for
particle
physics
and
field
theory,
can
be
very
easily
represented
and
explained
by
using
fiber
bundle
language.
The
canonical
formalism
of
classical
mechanics
assumes
the
cotangent
bundle
of
the
configuration
space
as
the
underlying
manifold.
Classical
electrodynamics
may
be
interpreted
as
the
theory
of
an
infinitesimal
connection
in
a
principal
fiber
bundle
with
82

structure
group
U(l).
A
similar
interpretation
can
be
given
to
the
Yang-Mills
fields,
and
in
general,
to
all
fields
resulting
from
"gauge
transformations".
Bundles
are
a
generalization
of
the
concept
of
Cartesian
product.
An
example
from
the
evolution
of
the
framework
of
physics
can
clarify
as
well
as
provide
the
need
for
such
a
generalization.
In
Aristotelian
physics,
space
and
time
are
considered
to
be
absolute,
i.e.,
every
physical
event
being
defined
by
an
instant
of
time
and
a
location
in
space.
This
is
equivalent
to
say
that
space-time
M
is
a
Cartesian
product
ofT
(time)
and
S
(space).
In
Galilean
(or
Newtonian)
physics,
the
time
remains
absolute,
but
space
is
not.
This
can
be
described
by
saying
that
there
is
a
projection
map
~
: M
~
T
which
to
any
event
p E M
associates
the
corresponding
instant
of
timet=
~(p).
Tis
called
the
base
space.
The
inverse
image
oft,
~-
1
(t),
is
called
a
fiber.
Each
fiber
is
isomorphic
to
R
3
,
is
therefore
called
a
typical
fiber.
This
is
the
usual
spatial
part
of
the
space-time,
where
the
Galilean
transformations
(translations
and
rotations)
map a
point
on
a
typical
fiber
to
another
point
on
the
same
fiber.
And
the
Galilean
invariant
quantities
make
sense.
In
special
relativity,
neither
the
space
nor
the
time
is
absolute.
Nonetheless,
the
bundle
of
linear
frames
of
the
space-time
is
a
product
bundle.
But
in
general
relativity,
it
is
so
complicated
that
only
the
principal
bundle
of
the
bundle
of
frames
of
the
space-time
is
a
product
bundle.
The
word
"global"
came
as
a
specific
mathematical
notion
when
differential
geometry
broke
away
from
its
historical
confinement
as
a
"local"
discipline.
The
contrast
between
the
local
and
global
aspects
of
differential
geometry
is
perhaps
best
illustrated
by
the
analytic
tools
that
are
being
used
for
studying
local
and
global
aspects
of
surfaces
or
manifolds
in
general.
The
analytic
machinery
of
local
differential
geometry
manifests
itself
as
a
collection
of
differential
expressions
83
