Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.


=
:Ec;
••• ; >
sign(i
1
,
••
,ir)(auCX;m»···Ca;p(X;q)).
For
instance,
if
r =
2,
a,
p e A
1
(M),
X, Y E X(M)
then
I
a
ex>
P
ex>
I
(aAP>
(X,
Y)
=a
(Y)
p
(Y)
In
general,
if
a
is
a
p-form,
P
is
a
q-form
on
M,
and
x
1
,
••
,x~q
e
X(M),
then
(a
A
P)
(X
1
,
••
,X~q)
=
:Ec;
.•.
; >
sign(i
1
••
i~q)
a(X;
,
•.
,X;
) PCX; ,
••
X;
) .
We
shall
denote
AP(M*)
to
be
the
collection
of
all
p-forms
on
M.
When
there
is
no
danger
of
ambiguity,
we
usually
will
denote
it
by
AP.
We
shall
now
introduce
a
derivative
called
an
exterior
derivative.
Let
M
be
~.
The
exterior
derivative
is
a
map:
d:
A~
A
with
the
following
properties:
(i)
For
q = o, f
is
a
~
function,
then
df
is
a
1-form;
(ii)
(iii)
(iv)
d
is
a
linear
map
such
that
d(Aq) c
A~
1
;
For
a E Aq,
{3
E A
8
d(a
A
P)
=
da
A
13
+
(-1)qa
A
dp;
d(df)
= 0
and
it
follows
that
d(da)
= 0
for
any
a.
Any
form
a
is
said
to
be
closed
if
da
= O;
if
a
is
a
p-form
and
there
is
a
(p-1)-form
p
such
that
a =
dp,
then
we
call
a
an
exact
p-form.
From
(iv)
above,
clearly
every
exact
p-form
is
also
closed.
Let
us
denote
AeP
be
the
space
of
exact
p-forms,
A/
be
the
space
of
closed
p-forms.
Clearly,
AeP
c
A/
c
AP.
oP(M) =
A//A/
is
called
the
p-dimensional
de
Rham
cohomology
group
of
M
obtained
by
using
differential
forms.
Clearly
oP
is
an
abelian
group
because
AeP
and
A/
are
themselves
abelian.
Theorem
2.8.1
Cde Rhaml
For
a
paracompact
and
T
2
differentiable
manifold
M,
DP(M)
~
HP(M,R).
Remark:
Thus
1)1'
is
a
topological
invariant.
It
also
follows
immediately
that
from
properties
(iii)
and
(iv)
94

closed
form
A
closed
form
=
closed
form,
closed
form
A
exact
form
=
exact.
Another
way
to
define
or
to
determine
whether
a
manifold
is
orientalble
or
not
is
the
following
useful
theorem.
Theorem
2.8.2
A
paracompact,
T
2
,
ca
manifold
M
of
dim
m
is
orientable
iff
M
admits
a
continuous,
nonvashing
globally
defined
n-form
(for
instance,
a
volume
element).
In
physics,
a
space-time
M
is
a
4-dim,
connected,
paracompact,
ca-manifold
with
Lorentz
signatures.
The
orientability
of
a
physical
"space-time"
is
concerning
the
consistent
assignment
of
an
"arrow
of
time"
and
of
"handedness"
throughout
the
"space-time".
The
strong
principle
of
equivalence
[Dicke
1959,
1962]
asserts
that
local
experiments
should
be
the
same
throughout
the
space-time.
From
the
strong
principle
of
equivalence,
some
local
experiments
in
particle
physics,
c,
P
(P-decay),
and
CP(KL, K
5
decays)
noninvariance
and
T
noninvariance,
and
CPT
invariance.
We
can
conclude
that
the
space-time
must
be
orientable.
Remark:
Assuming
space-time
being
orientable
is
also
convenient;
otherwise
we
can
always
find
its
universal
covering
space
which
is
orientable.
Now
we
shall
introduce
a
linear
mapping
which
is
also
an
antiderivation.
Let
X
£X(M)of
a
differentiable
manifold
M.
A
linear
mapping
(inner
product)
i(X)
: A
~
A
such
that
(i)
i(X)
:
AP
~
AP"
1
,
p
;::
1;
and
i(X)
(f)
=
O;
(ii)
i(X)
is
an
antiderivation,
i.e.,
if
a f
AP,
~
f
Aq,
then
i(X)
(a
A~)=
(i(X)a)
A~+
(-1)Pa
A
(i(X)~);
(iii)
if
a£
A
1
,
i(X)a
=
a(X).
Lie
derivative
is
a
geometric
procedure
which
is
very
useful
in
finding
symmetries,
such
as
Killing
vector
field
£xg = o.
We
shall
define
and
state
the
properties
of
Lie
derivatives.
£xY
=
[X,
Y],
also
called
the
"dragging"
of
Y
along
X
(more
appropriately,
along
the
integral
curve
of
X),
as
indicated
in
the
following
diagram.
95
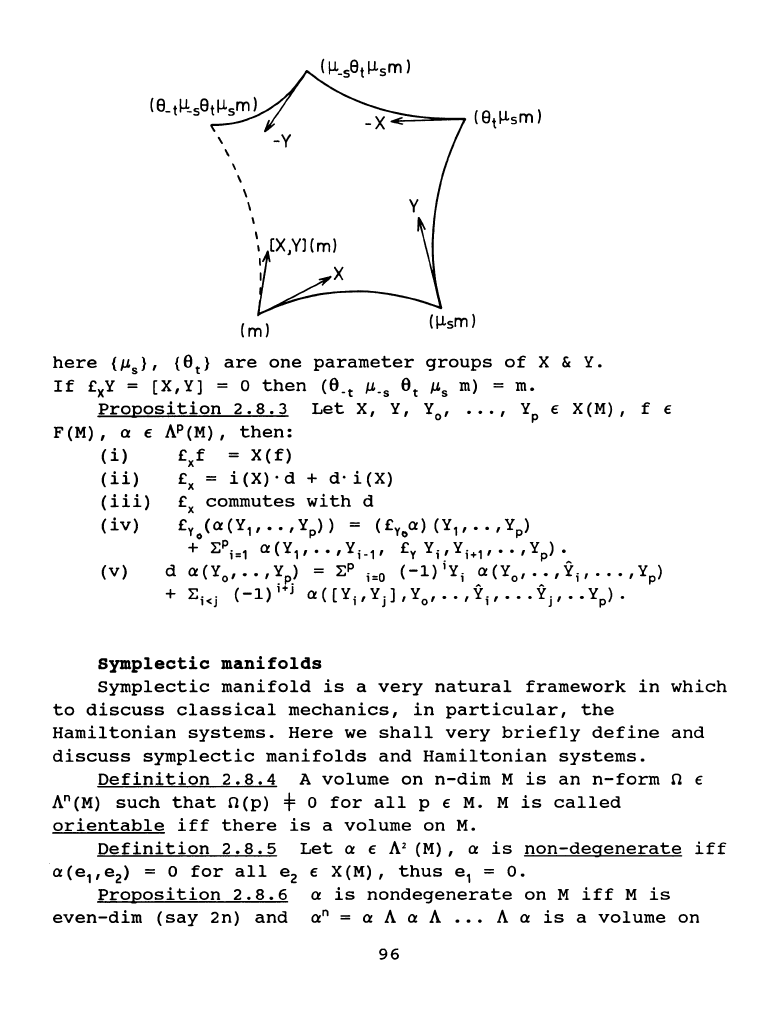
here
(~
5
},
(6t}
are
one
parameter
groups
of
X &
Y.
If
fxY
= [X,
Y]
= 0
then
(6.t
~-s
6t
~s
m)
=
m.
Proposition
2.8.3
Let
X,
Y,
Y
0
,
•••
,
YP
e X(M), f e
F(M),
a e AP(M),
then:
(i)
£xf =
X(f)
(ii)
£x=i(X)·d+d·i(X)
(iii)
£x
commutes
with
d
(iv)
£y
0
(a(Y
1
,
•.
,YP)) = (£y
0
a)(Y
1
,
..
,YP)
+
I;Pi=1
a(Y,,
••
,Yi-1'
£YYi,Yi+1'""'Yp)•
(v)
d
a(Y
0
,
••
,YP)
=
I;P
i=O
(-l)iyi
a(Y
0
,
••
,Yp••••Yp)
i+j
...
...
+
:Ei<i
(-1)
a([Yi'Yi],Y
0
,
••
,Yp•••Yi,
.•
YP).
symplectic
manifolds
Symplectic
manifold
is
a
very
natural
framework
in
which
to
discuss
classical
mechanics,
in
particular,
the
Hamiltonian
systems.
Here
we
shall
very
briefly
define
and
discuss
symplectic
manifolds
and
Hamiltonian
systems.
Definition
2.8.4
A
volume
on
n-dim
M
is
an
n-form
n e
An(M)
such
that
n(p)
+ 0
for
all
p e
M.
M
is
called
orientable
iff
there
is
a
volume
on
M.
Definition
2.8.5
Let
a e A
2
(M), a
is
non-degenerate
iff
a(e
1
,e
2
)
= 0
for
all
e
2
e X(M),
thus
e
1
=
0.
Proposition
2.8.6
a
is
nondegenerate
on
M
iff
M
is
even-dim
(say
2n)
and
an=
a A a A
...
A a
is
a
volume
on
96

M.
Thus
if
a
is
nondegenerate,
then
M
is
orientable.
Definition
2.8.7
A
symplectic
form
(structure)
on
an
even-
dim
M
is
a
non-degenerate,
closed
2-form
a
on
M.
A
symplectic
manifold
(M,
a)
is
an
even-dim
M
together
with
a
symplectic
form
a.
Theorem
2.8.8:
(Darboux)
Suppose
a
is
a
non-degenerate
2-form
on
2n-dim
M.
Then
da
= 0
iff
there
is
a
chart
(U,
~)
at
each
p
EM
such
that
~(p)
= 0
and
with
~(u)
=
(x
1
(u),
•••
,
x"(u),
y
1
(u),
...
,
y"(u)),
we
have
alu
=
L"i=l
dxi
A
dyi.
Remark:
The
charts
guaranteed
by
the
theorem
are
called
symplectic
charts
and
xi,
yi
are
canonical
coordinates.
Thus,
in
a
symplectic
chart
a = L
dxi
A
dyi
and
na
=
dx
1
A
A
dx"
A
dy
1
A
...
A
dy"
is
the
volume
element.
Remark:
In
most
mechanics
problems,
the
phase
space
is
T*M
and
the
coordinates
are
(q
1
,
•••
, q", p
1
,
•••
, Pn)
then
the
canonical
form
on
T*M
is
9 =
L"i=l
P;dqi
and
a =
Ln
i=l
dqi
A
dpi.
Proposition
2.8.9
T*M
of
any
manifold
is
orientable.
Theorem
2.8.10
Lie
derivative
of
X,
restricted
to
A(M*)
is
a
derivation,
i.e.,
(£x:
AP-+
AP),
£x=
i(X)
d +
d·i(X).
Example:
In
electrodynamics,
f E A
2
(M)
and
in
the
symplectic
chart,
f =
1/2
Fuv
dxu A
dxv.
The
Maxwell
equations
can
be
written
as:
df
=
0,
in
terms
of
symplectic
coordinate
chart,
it
can
be
written
as
F,.v,a + Fva,,. +
F«~',v
=
0.
In
the
usual
vector
notation,
it
is
equivalent
to
the
following
set:
div
B = 0
and
curl
E = -
dB/dt.
Now
define
the
star
operator
* :
AP
-+
A""P,
where
dim
M = n
and
the
Hodge's
operator
6 = d
*,
then
the
other
set
of
Maxwell
equations
can
be
represented
by:
of=
d*f
=
4~
*J.
It
is
equivalent
to:
divE=
4~q
and
curl
B =
dE/dt
+
4~J.
For
source-free,
then
F"v,v = o,
i.e.,
f
is
a
harmonic
2-form
(f
is
closed
and
coclosed).
The
existence
of
vector
potential
is
equivalent
to
the
non-existence
of
a
magnetic
monopole.
Definition
2.8.11
(M,
a)
and
(N,
~)
be
symplectic.
A
~
map
F : M
-+
N
is
symplectic
(or
canonical
transformation)
iff
F.
~
= a
where
F.
is
the
pullback
of
forms,
i.e.,
F.:
97

Ak
(N)
-+
Ak
(M)
•
Theorem
2.8.12
If
F:
M-+
N
is
symplectic,
then
F
is
volume
preserving,
and
F
is
a
local
diffeomorphism.
Definition
2.8.13
(M,
a)
is
symplectic.
f,
g E
F(M).
x
1
,
X
9
E
X(M).
The
Poisson
bracket
off
and
g
is
the
function
{f,g}
=
-ixt
ix
a.
't'
1
b
Propos~
~on
2.8.14
As
a
eve:
(i)
{f,g}
= - rx+ g =
rx,
f,
(ii)
d{f,g}
=
{df,dg}
(iii)
X<f,g> =
-[X
1
,X
9
]
(iv)
The
real
vector
space
F(M)
and
the
composition
{ ,
form
a
Lie
algebra.
(v)
(U,
~)
be
a
symplectic
chart,
then
the
Poisson
bracket
has
the
usual
form,
{f,g}
=
:En
i=1
[
(c3fjc3xi)
(c3gjc3yi)
-
(c3fjc3yi)
(c3gjc3xi)].
See
for
instance,
Goldstein
[1950).
Hamiltonian
systems
Definition
2.8.15
M
be
a
manifold,
X E
X(M).
Let
a E
Ak(M).
We
call
a
an
invariant
k-form
of
X
iff
£x
a=
o.
Definition
2.8.16
Let
(M,
a)
be
symplectic
and
X E
X(M).
We
say
that
X
is
locally
Hamiltonian
iff
a
is
an
invariant
2-form
of
X,
i.e.,
rx
a=
o.
The
set
of
locally
Hamiltonian
vector
fields
on
M
is
denoted
by
XL.H.
(M).
Proposition
2.8.17
(i)
Let
X E XL.H.(M)
on
2n-dim
(M,
a).
Then
a,
a•,
••.
,an
are
invariant
forms
of
X,
(ii)
XL.
H.
(M)
is
a
Lie
subalgebra
of
X
(M)
•
Proposition
2.8.18
The
following
are
equivalent:
(i)
X E
XL.H.
(M),
(ii)
ix
a
is
closed,
(iii)
U a
neighborhood
of
p E M
and
H e
F(U)
such
that
xlu
=
xH.
Definition
2.
8.
19
Let
X E
XL.H.
(M)
• A
function
H
as
above
is
called
a
local
Hamiltonian
of
X.
Proposition
2.8.20
H a
local
Hamiltonian
of
X.
Then
H
is
constant
along
the
integral
curves
of
X
in
U
(i.e.,
His
a
constant
of
motion,
or
"energy"
is
conserved).
This
is
because
£xH
=
£x
H = {H,H} =
0.
98

Proposition
2.8.21
Let
X E xl.H.(M) I
HE
F(U)
be
a
local
Hamiltonian
on
a
symplectic
manifold
(M,
a).
Let
V c
U,
(V,
~)
be
a
symplectic
chart
with
~(V)
c R
2
",
~(v)
=
(q
1
(v),
••
,q"(v),
p
1
(v)
••
pn(v)).
Then
a
curve
c(t)
on
Vis
an
integral
curve
of
X
iff
(dq;jdt)c(t)
=
(aHjaP;)c(t),
i
l,
...
,n
and
(dp;fdt)c(t)
=
-(aH;aq;)c(t),
i
l,
.•.
,n
where
qi(t)=
q;(c(t)),
P;(t)=
P;(c(t)).
Proposition
2.8.22
X, H, (M,
a)
as
above.
Then
for
f e
F(U),
we
have
£xf
=
{f,H}
on
u.
Before
ending
this
subsection,
for
our
future
use
as
well
as
for
general
interest,
we
are
going
to
discuss
briefly
the
Kolmogorov-Arnold-Moser
theorem.
This
theorem
was
originally
proved
for
Hamiltonian
of
two
degrees
of
freedom,
and
it
was
later
extended
to
n
degrees
of
freedom
systems
with
(2n
-
2)-
dimensional
Poincare
maps.
For
simplicity,
here
we
shall
only
state
the
theorem
for
two
degrees
of
freedom.
Let
us
consider
the
Hamiltonian
H'
is
a
small
perturbation
of
an
integrable
Hamiltonian
H
0
•
For
simplicity,
let
us
assume
that
the
perturbed
Hamiltonian
has
the
following
form:
H'(q,p,6,I)
=
F(q,p)
+
G(I)
+ eH
1
(q,p,6,I),
where
H
1
is
2~
periodic
in
6
and
the
unperturbed
system
H
0
(q,p,6)
=
F(q,p)
+
G(I)
decouples
directly
into
two
independent
systems
with
integrals
F
and
G.
Furthermore,
we
assume
nondegeneracy,
i.e.,
dG/di
+ o.
This
implies
that
for
e
small,
H'
is
invertible
and
can
be
solved
for
I.
By
transforming
(q,p)
to
a
second
set
of
action
angle
variables
J
and
~.
and
the
unperturbed
system
H
0
becomes
dJ/dt
=
0,
d~/dt
=
2~T,
and
it
associates
with
the
Poincare
map P
0
•
And
the
perturbed
system
modifies
the
Poincare
map
to
P,.
The
KAM
theorem
asserts
that
for
sufficiently
small
e,
most
of
the
closed
curves
J =
constant
of
P
0
are
preseved
for
P,.
In
other
words,
the
Poincare
map
under
consideration
is
an
area
preserving
diffeomorphism.
Theorem
(KAM)
If
an
unperturbed
Hamiltonian
system
is
99

nondegenerate
and
e
is
sufficiently
small,
then
the
perturbed
map P,
has
a
set
of
invariant
closed
curves
of
positive
Lebesgue
measure
~(E)
close
to
the
original
set
J
Ja~
moreover,
~(E)/~(J)
~
1
as
e
~
o.
The
surviving
invariant
closed
curves
are
filled
with
dense
irrational
orbits.
Instead
of
these
technical
versions,
we
can
put
the
KAM
theorem
in
the
following
words:
Theorem
(KAM)
If
an
uperturbed
system
is
nondegenerate,
then
for
sufficiently
small
conservative
Hamiltonian
perturbations,
most
non-resonant
invariant
tori
do
not
vanish,
but
are
only
slightly
deformed,
so
that
in
the
phase
space
of
the
perturbed
system,
there
are
invariant
tori
densely
filled
with
phase
curves
winding
around
them
conditionally
periodically
too,
with
the
number
of
independent
frequencies
equal
to
the
number
of
degrees
of
freedom.
These
invariant
tori
form
a
majority
in
the
sense
that
the
measure
of
the
complement
of
their
union
is
small
when
the
perturbation
is
small.
As
we
shall
see,
the
major
part
of
these
lectures
will
be
concerned
with
dissipative
dynamical
systems
and
with
the
structure
of
the
nonwandering
and
attracting
sets
occuring
in
such
systems.
Nonetheless,
it
is
often
very
useful
to
consider
such
systems
as
perturbations
of
Hamiltonian
systems,
this
is
because
the
existence
of
energy
integrals
or
other
constants
of
motion
enables
us
to
obtain
global
information
on
the
structure
of
solutions.
Thus,
the
KAM
theorem
will
be
utilized
many
times
in
later
discussions
either
explicitly
or
implicitly.
Recently,
transport
in
three-dimensional
nonintegrable
Hamiltonian
flows
is
studied
and
the
destruction
of
KAM
barriers
in
the
presence
of
stochastic
perturbations
are
described
[Gyorgyi
and
Tishby
1989].
They
further
extended
the
action
principle
to
Hamiltonians
with
small
noise,
which
provided
a
framework
to
determine
universal
scaling
of
characteristic
times
as
a
function
of
the
noise.
For
the
example
of
a
Hamiltonian
system
with
two
degrees
100
of
freedom,
e.g.,
two
coupled
nonlinear
oscillators,
Greene
[1979]
developed
a
method
for
deciding
the
existence
of
any
given
KAM
surface
computationally.
One
finds,
when
given
that
KAM
orbits
exist,
that
the
guiding
hypothesis
is
that
the
disapearance
of
a
KAM
surface
is
associated
with
a
sudden
change
from
stability
to
instability
of
nearby
periodic
orbits.
The
relation
between
KAM
surfaces
and
periodic
orbits
has
been
explored
extensively
in
this
paper
by
the
numerical
computation
of
a
particular
mapping.
Lagrangian
system
We
will
be
concerned
with
an
alternative
description
of
classical
mechanics
on
another
phase
space,
the
tangent
bundle
of
the
configuration
space
M
of
the
system,
TM.
We
consider
a
function
L
on
TM
and
solutions
to
a
certain
second-order
equation.
From L
we
can
derive
an
energy
function
E
on
TM
which,
when
translated
to
T*M
by
means
of
the
"fiber
derivative"
FL:
TM
-+
T*M
(
usually
called
the
Legendre
transformation),
the
derivative
of
Lin
each
fiber
of
TM
yeilds
a
suitable
Hamiltonian.
Then
the
solution
curves
in
T*M
(i.e.,
solutions
of
Hamiltonian
equations)
and
in
TM
(i.e.,
solutions
of
Lagrangian
equations)
will
coincide
when
projected
to
M.
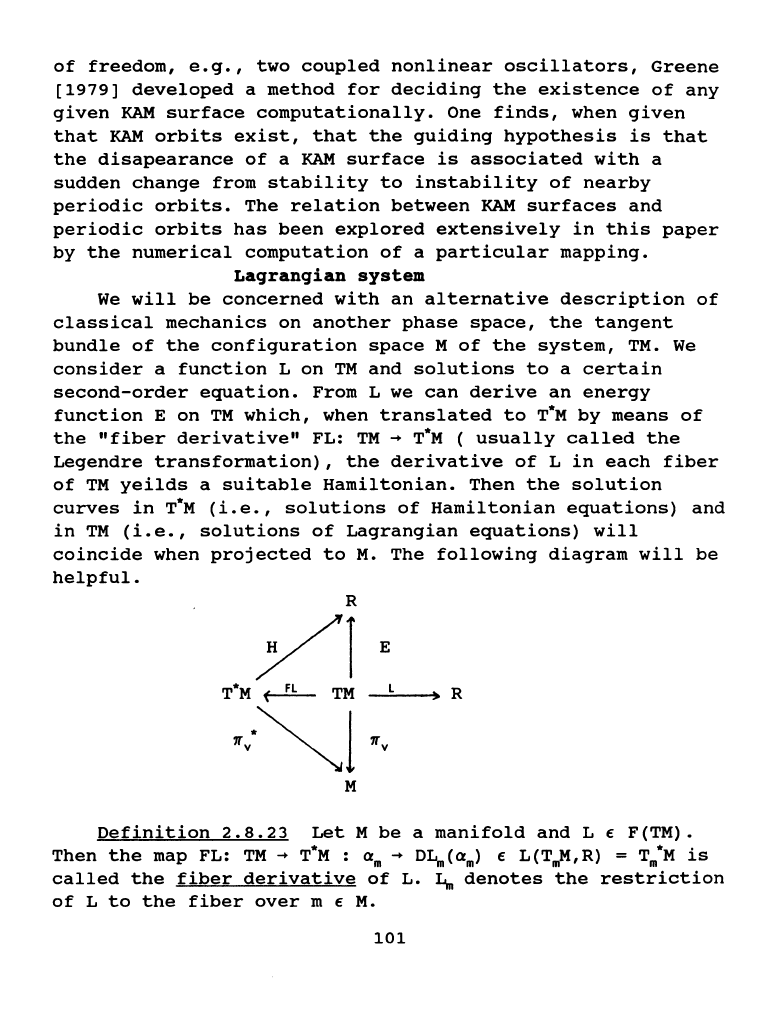
The
following
diagram
will
be
helpful.
R
/1
E
T*M
t---f,L-
TM
R
·:~1
7rv
M
Definition
2.8.23
Let
M
be
a
manifold
and
L e F(TM).
Then
the
map FL:
TM
-+
T*M
:
am
-+
DL.n(am)
e
L(T...M,R)
=
Tm*M
is
called
the
fiber
derivative
of
L.
r....
denotes
the
restriction
of
L
to
the
fiber
over
m e
M.
101

Proposition
2.8.24
FL:
TM
~
T*M
is
a
fiber
preserving
map.
It
should
be
noted
that
FL
is
not
necessarily
a
vector
bundle
mapping.
For
further
detail
of
"geometric
theory
of
classical
mechanics",
Abraham
and
Marsden
[1978]
and
Arnold
[1978]
are
highly
recommended.
2.9
vector
bundles
and
tubular
neighborhoods
As
we
have
mentioned
earlier,
a
vector
bundle
can
be
thought
of
as
a
family
of
disjoint
vector
spaces
{Vx>x•M
parameterized
by
the
base
space
M.
The
union
of
these
vector
spaces
is
a
space
B,
and
the
map
~
: B
~
M,
~(Vx)
= x
is
continuous.
Furthermore,
~
is
locally
trivial
in
the
sense
that
locally
B
looks
like
a
product
of
B
with
R";
and
there
are
open
sets
U
covering
M
and
homeomorphisms
~-
1
(U)
~
U x
R",
mapping
each
fiber
Vx
linearly
onto
{x}xR". A
morphism
from
one
vector
bundle
to
another
is
a map
taking
fibers
linearly
into
fibers.
A
vector
bundle
is
similar
to
a
manifold
in
that
both
are
building
up
from
elementary
objects
glued
together
by
special
maps.
For
manifolds,
the
elementary
objects
are
open
subsets
of
R",
the
gluing
maps
are
diffeomorphisms;
for
vector
bundles
the
elementary
objects
are
local
"trivial
bundles"
UxR",
and
the
gluing
maps
are
morphisms
UxR"
-+
UxR"
of
the
form
(x,y)-+
(x,g(x)y)
where
g:
U-+
GL(n,R).
In
both
manifolds
and
vector
bundles,
linear
maps
play
a
central
role.
Linear
maps
enter
into
manifolds
in
a
subtle
way
as
derivatives,
the
linearity
in
vector
bundle
is
more
explicit.
This
makes
the
category
of
vector
bundles
more
flexible
and
easier
to
analyze
than
that
of
manifolds.
In
fact,
many
natural
constructions
with
vector
bundles,
such
as
direct
sum,
quotients,
and
pullbacks,
are
impossible
to
make
for
manifolds.
By
introducing
tubular
neighborhoods,
a new
connection
between
vector
bundles
and
the
topology
of
manifolds
is
102

established.
If
M c N
is
a
submanifold
and
M
has
a
certain
neighborhood
in
N
which
looks
like
a
normal
vector
bundle
of
M
in
N.
Furthermore,
such
neighborhoods
are
essentially
unique.
Consequently,
the
study
of
the
kinds
of
neighborhoods
that
M
can
have
as
a
submanifold
of
a
larger
manifold
is
reduced
to
the
classification
of
vector
bundles
over
M.
For
example,
the
question
whether
the
inclusion
map
M
c~
N
can
be
approximated
by
imbedding
is
tantamount
to
whether
the
normal
bundle
of
M
in
N
has
a
nonvanshing
section.
Let
F
be
a
field
(can
be
either
real
R,
complex
c
or
quaternion
Q).
A
k-dimension
vector
bundle
n
over
F
is
a
bundle
(B,M,~)
together
with
the
structure
of
a
k-dimensional
vector
space
over
F
on
each
fiber
~-
1
(p)
such
that
the
local
triviality
condition
is
satisfied:
For
each
point
of
M
ther
is
an
open
neighborhood
U
and
an
isomorphism
i:
UxFk
~
~-
1
(U)
such
that
the
restriction
pxFk
~
~-
1
(p)
is
a
vector
space
isomorphism
for
each
p e u.
If
F = R
it
is
a
real
vector
bundle,
F = C a
complex
vector
bundle,
F = Q a
quaternionic
vector
bundle.
And
the
isomorphism
i:
UxFk
~
~-
1
(U)
is
a
local
coordinate
chart
of
TJ.
One
can
also
define
vector
bundles
as
a
special
case
of
a
fiber
bundle,
or
a
special
principal
bundle.
For
instance,
a
real
vector
bundle
is
a
fiber
bundle
where
the
fiber
is
a
real
vector
space
vk
and
the
structure
group
is
GL(k,R).
It
is
helpful
to
introduce
the
notion
of
an
exact
sequence
of
vector
bundles
morphisms
in
just
the
way
as
an
exact
sequence
of
groups
introduced
earlier,
i.e.,
a
finite
or
infinite
sequence
••••
~
Tl;-1 f
~
T/;
f
~
T/;+1
~
•••
of
morphisms
such
that
for
each
p e M
we
have
Im(f;.
1
) P
=
Ker(f;)p
for
all
i.
In
particular,
we
are
interested
in
the
short
exact
sequence
0
~
a
1
~
~
s
~
~
~
0
where
o
denotes
a
0-dim
bundle
over
M.
such
an
exact
sequence
means
that
f
is
one-to-one,
onto,
and
Im f =
Ker
g.
One
can
show
that
the
short
sequence
is
unique
up
to
isomorphism.
103
