Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.

the
k-jet
bundle
of
M
and
N,
be
the
disjoint
union
of
Jk(M,N)p,q'
i.e.,
Jk(M,N) =
U<p,qlrMxN
Jk(M,N)p,q• Any
element
h £ Jk(M,N)
is
a
k-jet
of
mappings
(or
just
k-jetl
from
M
to
N.
(v)
Let
h £ Jk(M,N)
be
a
k-
jet,
then
there
exist
p
in
M
and
q
in
N
such
that
h £
Jk(M,N)p,q•
Then
p
is
the
source
of
h
and
q
is
the
target
of
h·
The
mapping
a : Jk(M,N)
~
M,
defined
by
h
~
source
of
h,
is
the
source
map
and
the
mapping
p : Jk(M,N)
~
N,
defined
by
h
~
target
of
h,
is
the
target
map.
For
a
given
smooth
map f:M
~
N
and
for
every
p £
M,
there
is
a
canonically
defined
mapping
jkf
: M
~
Jk(M,N)
called
the
k-
jet
of
f
defined
by
jkf(p)
=
equivalence
class
of
f
in
Jk(M,N)p,f<pl"
Thus,
jkf
at
p
is
a
local
lifting
from
M
to
Jk(M,N)p,f<pl'
i.e.,
jkf(p)
is
just
an
invariant
way
of
describing
the
Taylor
expansion
of
f
at
p
up
to
order
k.
One
can
also
show
that
jkf
is
a
smooth
mapping.
Since
J
0
(M,N) = M x
N,
so
f
has
-
0
contact
with
g
at
p
iff
f(p)
=
g(p),
and
j
0
f(p)
=
(p,f(p))
is
just
the
graph
of
f!
Since
any
given
map
determines
a
k-jet
at
each
point
of
its
domain,
thus
if
f:M
~
N
is
smooth,
the
k-jet
extension
of
f
is
a map
jkf:
U
~
Jk(M,N)
by
x
~
j/f,
where
U
is
the
domain
of
f.
Clearly,
the
k-jet
extension
of
f
is
a
cross-section
of
the
source
map
a,
i.e.,
a·jkf
=
idu.
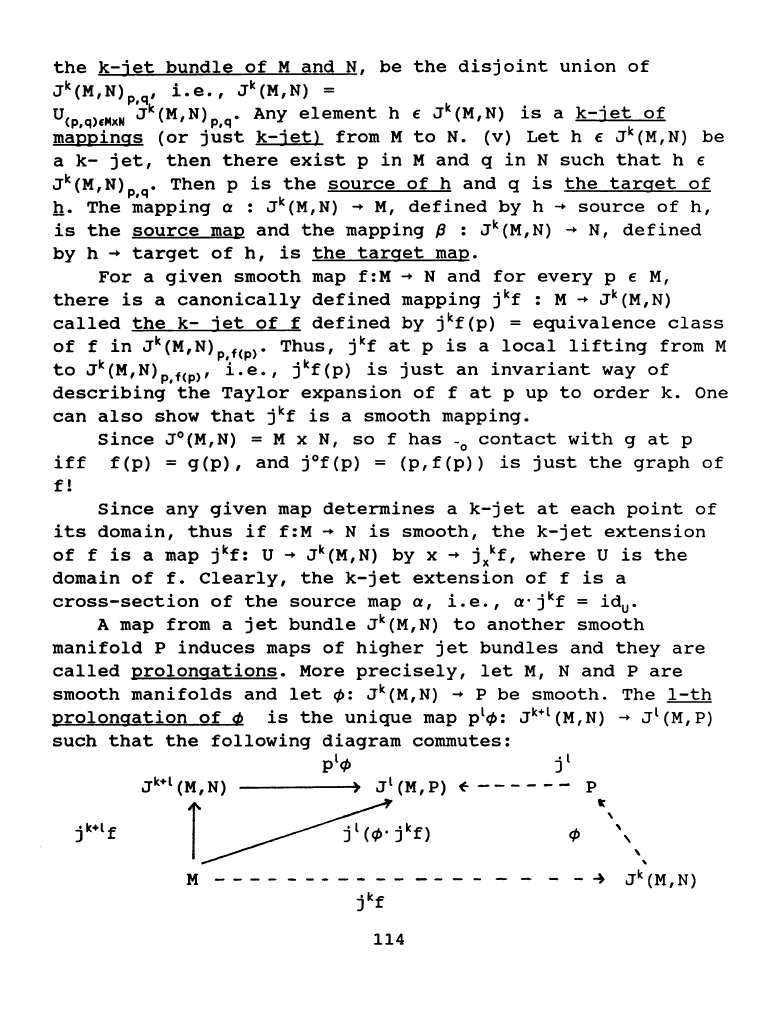
A map
from
a
jet
bundle
Jk(M,N)
to
another
smooth
manifold
P
induces
maps
of
higher
jet
bundles
and
they
are
called
prolongations.
More
precisely,
let
M,
N
and
P
are
smooth
manifolds
and
let
~=
Jk(M,N)
~
P
be
smooth.
The
1-th
prolongation
of
d>
is
the
unique
map
p
1
~:
Jk+l
(M,N)
~
J
1
(M,
P)
such
that
the
following
diagram
commutes:
pl~
jl
Jk+l
(M,N)
Jl
(M,
P)
~------
p
r~j.f}
M
------------------
--+
114
'
'

In
appropriate
local
coordinates
on
M,
N,
and
P,
roughly
speaking,
the
prolongations
amount
to
the
taking
of
total
derivatives
of
¢.
We
shall
not
go
into
any
details.
For
this,
one
may
want
to
consult
[Pirani,
Robinson
and
Shadwick
1979].
Jet
bundles
not
only
are
important
in
discussing
global
analysis
and
nonlinear
differential
operators
on
which
we
shall
embark
briefly,
but
they
are
also
important
in
describing
higher
order
vector
bundles
on
smooth
manifolds
[see
e.g.,
Yano
and
Ishihara
1973].
over
the
past
couple
of
decades,
a
class
of
transformations
discovered
by
Backlund
over
a
century
ago
has
emerged
as
an
important
tool
in
the
study
of
a
wide
range
of
nonlinear
evolution
equations
in
theoretical
physics
and
continuum
mechanics.
Backlund
transformations
are
significant
because
the
invariance
under
Backlund
transformations
can
be
utilized
to
generate
an
infinite
sequence
of
solutions
of
certain
nonlinear
evolution
equations
by
purely
algebraic
procedures.
current
work
on
Backlund
transformations
are
mainly
concerned
with
extending
their
applications
to
other
nonlinear
partial
differential
equations
of
physical
interest
and
with
encompassing
the
known
results
in
a
unified
theory
so
that
generalizations
to
higher
dimensions
can
be
made.
Jet-bundle
formulation
of
Backlund
transformations
seems
to
provide
an
appropriate
geometric
setting
for
the
main
concerns
of
Backlund
transformations
described
above
and
their
connection
with
the
inverse
scattering
formalism,
prolongation
structures,
and
symmetries.
For
those
readers
who
are
interested
in
these
areas
of
research,
please
consult
Pirani,
Robinson
and
Schadwick
[1979],
Rogers
and
Schadwick
[1982],
Hermann
[1976,
1977],
Estabrook
and
Walquist
[1975].
Lemma
3.2.1
Let
U c
R"
be
open,
and
p e U.
Let
f,g:
u
~
Rm
be
smooth
mappings.
Then
f
-k
g
at
p
iff
(D'"fi)
(p)
=
(D'"gi)
(p)
for
lal
S
k,
1
SiS
m
where
a=
(a
1
,
••
,an)
are
n-tuples
of
non-negative
integers,
I a I = a
1
+ a
2
+
•...
+ an,
o«
=
al«l;c3x
1
'"~
••
c3xn«':
and
fi
and
gi
are
the
coordinate
functions
determined
by
f
and
g
respectively.
115

This
lemma
sometimes
is
used
to
define
the
equivalence
relation
"-k".
As we
have
pointed
out
earlier,
the
following
corollary
follows
immediately.
Corollary
3.
2.
2 f
and
g U
-+
Rm
such
that
f
-k
g
at
p
iff
the
Taylor
expansions
of
f
and
g
up
to
and
including
order
k
are
identical
at
p.
The
following
lemma
which
concerns
the
equivalence
relation
between
a
pair
of
composite
maps
is
intuitively
clear
and
can
be
proven
by
induction.
Lemma
3.2.3
Let
U c
Rn
and
V c
Rm
are
open
subsets.
Let
f
1
,
f
2
:
u
-+
v
and
g,
g
2
:
V
-+
R
1
be
smooth
maps
such
that
g
1
•
f
1
and
g
2
•
f
2
make
sense.
Let
p e U
and
suppose
f
1
-k
f
2
at
p
and
g
1
-k
g
2
at
f
1
(p)
= f
2
(p)
=
q.
Then
g
1
• f
1
-k
g
2
• f
2
at
p.
Theorem
3.2.4
Let
X,
Y,
Z
and
W
be
smooth
manifolds.
(i)
Let
h:
Y-+ z
be
smooth,
then
h
induces
a map
h.:
Jk(X,Y)
-+
Jk(X,Z)
defined
by:
let
a e Jk(X,Y)p,q
and
let
f:
X-+ Y
represent
a.
Then
h.(a)
=the
equivalence
class
of
h·f
in
Jk(X,Z)p,h<q>'
(ii)
Let
a:
z-+
w
be
smooth.
Then
a.·h.
=
(a·h).
as
mappings
of
Jk(X,Y)
-+
Jk(X,W)
and
(idy)•
=
idJ
<x,n·
Thus
if
his
a
diffeomorphism,
h.
is
a
bijection
(one-to-one,
onto).
(iii)
Let
g : Z
-+
X
be
a
diffeomorphism,
then
g
induces
a
map g*:
Jk(X,Y)
-+
Jk(Z,Y)
defined
as
follows:
let
a e
Jk(X,Y)p,q
and
let
f:X-+
Y
represent
a.
Then
g*(a)=
the
equivalence
class
of
f·g
in
Jk(Z,Y)
9
"1<p>,q'
(iv)
Let
b:
W-+
z
be
a
diffeomorphism.
Then
the
induced
composite
mappings
b*·g* =
(g·b)*
are
mappings
of
Jk(X,Y)-+
Jk(W,Y)
and
(idx)*
=
id}'<x.n
so
that
g*
is
also
a
bijection.
Let
~k
be
the
vector
space
of
polynomials
in
n-variables
of
degree
S k
with
their
constant
term
equal
to
zero.
Choose
the
coefficients
of
the
polynomials
as
coordinates
of
An
k,
then
An
k
is
isomorphic
to
some
Euclidean
space
and
thus
a
smooth
manifold.
Let
Bn,mk
=
E9
i=lm
Ank·
Here
E9
is
the
Whitney
sum.
Then
Bnmk
is
also
a
smooth
manifold.
Theorem
3.2.5
Let
X
and
'y
be
smooth
manifolds
and
dim
X
=
n,
dim
Y =
m.
(i)
Jk(X,Y)
is
a
smooth
manifold,
and
dim
Jk(X,Y)
= m + n +
dim(Bn,mk).
116

(ii)
The
source
map a : Jk(X,Y)
~X
and
the
target
map p
Jk(X,Y)
~
Y,
and
a x p : Jk(X,Y)
~
X x Y
are
submersions.
(iii)
If
h : Y
~
Z
is
smooth,
then
h.
: Jk(X,Y)
~
Jk(X,Z)
is
smooth.
If
g : Z
~X
is
a
diffeomorphism,
then
g*
:
Jk(X,Y)
~
Jk(z,Y)
is
a
diffeomorphism.
(iv)
If
h :
X~
Y
is
smooth,
then
Jkh :
X~
Jk(X,Y)
is
smooth.
[Golubitsky
&
Guillemin
1973,
p.40].
Note
that
in
general
Jk(X,Y)
is
not
a
vector
bundle
because
there
is
no
natural
addition
in
Jk(X,
Y)p,q"
But
if
Y
=
Rm,
then
Jk(X,Rm)
is
indeed
a
vector
bundle
over
X x
Rm
where
the
addition
of
jets
is
given
by
the
addition
of
functions
representing
these
jets
in
Jk (X,
Rm)
p,q.
Although
Jk(X,Y)
is
not
a
vector
bundle
in
general,
it
is
more
than
just
a
manifold,
it
is
a
fiber
bundle.
In
fact,
if
k > 1
and
ignoring
all
derivatives
above
the
1-th
yields
the
natural
projection
'lrk,l
: Jk(X,Y)
~
J
1
(X,Y)
by
j\f
~
j
1
xf.
Thus,
Jk(X,Y)
is
a
fiber
bundle
over
J
1
(X,Y)
with
projection
'lrk,l"
In
particular,
J
0
(X,Y) may
be
identified
with
X x Y
and
J
1
(X,Y) may
be
thought
of
as
a
vector
bundle
over
X x
Y.
Furthermore,
7ri
1
is
understood
as
the
identity
map
of
l •
J
(X,
Y) •
As
we
have
seen
in
this
section,
jet
bundle
is
the
proper
language
to
describe
relations
between
smooth
maps
among
smooth
manifolds.
Thus,
it
will
be
very
important
in
discussing
stable
maps,
their
singularities,
and
bifurcations.
Of
course,
it
will
also
be
very
important
to
describe
global
stability
properties.
Indeed,
it
is
one
of
the
mathematical
techniques
frequently
used
in
much
current
literature
on
the
theoretical
dynamical
systems.
There
is
no
single
volume
which
discusses
jet
bundles
and
their
appliations.
Jet
bundles
can
be
found
in
many
books
discussing
global
analysis.
The
following
books
cover
some
discussions
of
jet
bundles:
Pirani,
Robinson
and
Schadwick
[1979],
Golubitsky
and
Guillemin
[1973],
and
of
course,
Palais
[1968].
117

3.3
Whitney
c-
topology
As we
have
mentioned
earlier,
in
order
that
any
physical
fields,
be
they
scalar,
vector,
or
tensor
fields,
have
physical
significance,
they
must
have
some
form
of
stability
under
"perturbation".
In
other
words,
"nearby"
fields
should
have
the
same
properties
and
physical
significance.
But
in
order
to
give
a
precise
meaning
of
"nearby",
one
has
to
define
a
topology
on
the
set
of
fields.
There
are
various
ways
in
which
this
can
be
defined
depending
on
whether
one
requires
a
"nearby"
field
to
be
nearby
in
just
its
values
(C
0
topology)
or
also
in
its
derivatives
up
to
the
kth
order
(ck
topology)
and
whether
one
requires
it
to
be
nearby
everywhere
(open
topology)
or
only
on
compact
sets
(compact
open
topology).
Let
M
and
N
be
smooth
manifolds
and
~(M,N)
be
the
set
of
all
smooth
mappings
from
M
to
N.
For
a
fixed
non-negative
integer
k,
let
U
be
a
subset
of
Jk(M,N).
Let
8(U)
denote
the
set
{f
e
~(M,N)
jjkf(M)
c U}.
Also
note
that
8(U)n8(V)
=
8(UnV).
This
family
of
sets
{8(U)}
forms
a
basis
for
the
Whitney
ck
topology.
Let
Wk
denote
the
set
of
open
subsets
of
~(M,N)
in
the
Whitney
ck
topology.
Thus
the
Whitney
C~
topology
on
~(M,N)
is
the
topology
whose
basis
is
the
union
of
all
Wk's
where
k=
O,
••
,oo,
i.e.,
W =
Uk=O~
Wk.
This
basis
is
a
well-defined
one
because
wk
c w
1
for
k S
1.
In
usual
literature,
the
Whitney
ck
topology
is
also
called
the
strong
or
fine
topology
on
ck(M,N)
sometimes
denoted
by
C
8
k(M,N),
and
the
compact-open
topology
on
ck(M,N)
is
also
called
the
weak
topology
on
ck(M,N),
denoted
by
cwk(M,N).
If
M
is
compact,
the
weak
topology
is
the
same
as
the
strong
topology.
Thus
the
strong
topology
is
more
useful.
But
it
is
only
fair
to
point
out
that
weak
topology
has
very
nice
features:
it
has
a
countable
basis
(we
shall
point
this
out
for
strong
topology
with
compact
M), a
complete
metric,
and
for
compact
M,
cwk(M,R")
is
a
Banach
space.
Moreover,
the
Whitney
imbedding
theorem
(Sect.2.4)
can
be
stated
in
relation
to
the
weak
topology:
Let
M
be
a
compact
cr
manifold,
2 S r S
oo
•
Then
imbeddings
are
dense
118

in
cwr(M,Rq)
if
q > 2m,
while
immersions
are
dense
if
q
~
2m,
m =
dim
M.
Let
us
describe
a
neighborhood
basis
in
the
Whitney
ck
topology
for
a
function
fin
c-(M,N).
Since
all
manifolds
are
metrizable
[Kelley
1955],
one
can
choose
a
metric
on
Jk(M,N)
compatible
with
the
ck
topology.
Let
B
6
(f)
=
{g
€
c-cM,N) I
for
all
p €
M,
d(jkf(p),jkg(p))
<
o(p)},
where
o:
M
~
R+
is
C
0
•
Thus
one
can
consider
B
6
(f)
as
the
set
of
smooth
mappings
of
M
~
N
whose
partial
derivatives
up
to
the
k-th
order
are
o-close
to
f's.
Let
u
be
an
open
neighborhood
of
f
in
c-(M,N),
one
can
show
that
B
6
(f)
c u,
and
indeed,
B
6
(f)
is
open
for
every
o.
If
M
is
compact,
c-(M,N)
satisfies
the
first
axiom
of
countability.
This
is
because
one
can
find
a
countable
neighborhood
basis
of
f
by
B
6
(f)
where
on(P) =
1/n
for
all
p
€
M,
thus
o
is
bounded
below
for
large
n.
One
can
easily
prove
that:
a
sequence
of
functions
fn
in
c-(M,N)
converges
to
f
(in
the
ck
topology)
iff
jkfn
converges
uniformly
to
jkf.
Clearly,
in
the
local
situation,
fn
and
all
of
the
partial
derivatives
of
fn
of
order
S k
converge
uniformly
to
f's.
For
noncompact
manifolds,
the
concept
of
the
convergence
of
fn
to
f
is
stronger
than
uniform
convergence
and
the
weak
topology
does
not
control
the
behavior
of
a map
at
"infinity"
very
well.
Thus
the
statement
for
compact
manifolds
has
to
be
modified.
Since
the
manifolds
are
paracompact,
then
it
is
locally
compact
(Sect.2.4).
Thus
one
can
extend
the
result
of
a
compact
manifold
to
a
compact
subset
of
the
noncompact
manifold.
More
precisely;
the
sequence
of
mappings
fn
converge
to
f
(in
the
ck
topology)
iff
there
is
a
compact
subset
C
of
M
such
that
jkfn
converges
uniformly
to
jkf
on
c
and
all
but
a
finite
number
of
the
fn's
equal
to
f
outside
c.
In
fact,
for
a
noncompact
M,
c-(M,N)
in
the
Whitney
ck
topology
does
not
satisfy
the
first
axiom
of
countability.
Nonetheless,
the
Whitney
(strong)
ck-
topology
is
very
important
for
differential
topology
for
the
fact
that
many
interesting
and
important
119

subsets
are
open.
For
example:
let
Immr(M,N)
be
the
set
of
cr
immersions
of
M
in
N, Imbr(M,N)
be
the
set
of
cr
imbeddings
of
M
in
N,
Diffr(M,N)
be
the
set
of
cr
diffeomorphisms
from
M
onto
N,
and
Propr(M,N)
be
the
set
of
proper
cr
maps M
-+
N
where
f:M
-+
N
is
proper
if
f-
1
takes
compact
sets
to
compact
sets.
Then
we
have
the
following
important
theorem.
Theorem
3.3.1
Immr(M,N)
and
Imbr(M,N)
are
open
in
C
5
r(M,N)
(Whitney
ck
toplogy)
for
r
~
1;
Propr(M,N)
is
open
in
C
5
r
(M,
N)
for
r
~
0;
if
M
and
N
are
cr
manifolds
without
boundary,
then
Diffr(M,N)
is
open
in
C
5
r(M,N)
for
r
~
1;
and
if
M
and
N
are
manifolds
without
boundary
and
f:M
...
N
is
a
homeomorphism,
then
f
has
a
neighborhood
of
onto
maps
in
C
5
°(M,N).
A
very
basic
approximation
theorem
for
a
manifold
without
boundary
is:
Theorem
3.3.2
Let
M
and
N
be
C
8
manifold
without
boundary,
1 S s S
~.Then
C
8
(M,N)
is
dense
in
C
8
r(M,N),
0 S
r S
s.
For
its
many
applications
in
differential
topology,
see
e.g.,
(Hirsch
1976,
Chapter
2,
section
2].
For
example,
from
the
above
theorem
and
the
openness
theorem
and
a
theorem
on
diffeomorphisms,
one
has:
Theorem
3.3.3
Let
1 S r S
~
,
every
cr
manifold
is
cr
diffeomorphic
to
a
c-
manifold.
(This
is
the
theorem
due
to
Whitney
we
quoted
at
the
begining
of
Chapter
2).
The
following
definitions
and
propositions
are
of
technical
nature
and
they
are
useful
in
describing
the
generalized
transversality
theorem,
or
in
proving
some
results.
The
reader
may
skip
the
following
five
propositions
without
loss
of
continuity.
Let
X
be
a
topological
space,
then:
(i)
A
subset
Y
of
X
is
residual
if
it
is
the
countable
intersection
of
open
dense
subsets
of
X.
(ii)
X
is
a
Baire
space
if
every
residual
set
is
dense.
Proposition
3.3.4
Let
M
and
N
be
smooth
manifolds,
then
c-(M,N)
is
a
Baire
space
in
the
Whitney
c-
topology.
120

[Goluoitsky
&
Guillemin
1973,
p.
44].
Let
us
list
some
properties
of
~(M,N)
which
will
be
used
later.
Proposition
3.3.5
Let
M
and
N
be
smooth
manifolds.
Then
the
mapping
jk:
~(M,N)
~
~(M,Jk(M,N))
defined
by
f
~
jkf
is
continuous
in
the
Whitney
~
topology.
Proposition
3.3.6
Let
M,
N
and
P
be
smooth
manifolds
and
let
~:
N
~
P
be
smooth.
Then
the
induced
map
~.:
c~(M,N)
~
~(M,P)
defined
by
f
~ ~·f
is
a
continuous
mapping
in
the
Whitney
~
topology.
Proposition
3.3.7
Let
M,
N
and
P
be
smooth
manifolds.
Then
~(M,N)x~(M,P)
is
homeomorphic
to
~(M,NxP)
in
the
C~
topology
by
the
standard
identification
(f,g)
~
f x g
where
f £
~(M,N),
g £
~(M,P),
p £ M
and
(f
X
g)
(p)
=
(f(p)
,g(p)).
Proposition
3.3.8
Let
M,
N
and
P
be
smooth
manifolds,
and
in
addition,
M
is
compact,
then
the
mapping
of
~(M,N)x~(N,P)
~
~(M,P)
defined
by
(f,g)
~
g·f
is
continuous,
where
f £
~(M,N),
g £
~(N,P).
Note
that
this
proposition
will
not
be
true
if
M
is
not
compact.
Nonetheless,
if
we
replace
~(M,N)
by
its
open
subset
of
proper
mappings
f
of
M
into
N
(recall,
f
is
proper
if
f'
1
maps
compact
sets
to
compact
sets),
then
the
same
conclusion
is
valid.
Note
also
that
the
induced
mapping
f*:
~(N,P)
~
~(M,P)
defined
by
g
~
g·f
is
(not)
continuous
if
f
is
(not)
a
proper
mapping.
In
particular,
if
M
is
an
open
subset
of
N
and
f
is
just
the
inclusion
map,
then
the
restriction
map
of
~(N,P)
~
~(M,P)
given
by
g
~
giM
is
not
continuous.
We
now
state
Them's
transversality
theorem
in
a
more
general
form
and
show
that
Theorem
2.4.27
is
a
corollary.
Theorem
3.3.9
(Them's
transversality
theorem)
Let
M
and
N
be
smooth
manifolds
and
w a
submanifold
of
Jk(M,N).
Let
Tw
=
{f
£
~(M,N)
I
jkf
if'
W}.
Then
Tw
is
a
residual
subset
of
~(M,N)
in
the
Whitney
~
topology.
Since
J
0
(M,N) = M x
Nand
j
0
f(p)
(p,f(p)),
and
the
target
map
p:
M x N
~
N
is
onto,
so
p·
1
(W)
is
a
submanifold
121

of
M x N.
If
j
0
f
.Ji
p·
1
(W)
at
p
then
clearly
f
~
W
at
p.
[If
j
0
f(p)
f
p-
1
(W)
then,
f(p)
E
W.
But
if
j
0
f(p)
E
p-
1
(W)
and
(tJ"
1
(W))Cp,f(p))
+
(dj
0
f)MP
=
(MxN)Cp,fCp»"
Then
apply
dtf
one
obtains
WfCp>
+
(df)~
=
NfCp>"
Thus
f m W
at
p).
Since
the
set
of
all
maps
which
are
transverse
to
W
contains
the
set
{ f E cf"
(M,
N)
I j
0
f
,f.
p-
1
(W)
} ,
which
is
dense
by
Theorem
3. 3.
9.
Thus
it
is
established
that
Theorem
2.4.27
is
a
special
case
of
Theorem
3.3.9.
Note
that
usually
Them's
transversality
theorem
is
stated
as
a
combination
of
Theorems
2.4.26
and
2.4.27.
Sometimes
Theorems
2.4.26
and
2.4.27
are
called
the
elementary
transversality
theorem.
Let
us
describe
an
"obvious"
generalization.
Let
M
and
N
be
smooth
manifolds
and
define~=
Mx
.•.
xM
(s
times)
and
xes>
= {
(x
1
,
••
xs) E
~
I
X;
+ xi
for
1 S i S j S
s}.
Let
a
be
the
source
map,
i.e.,
a:
Jk(M,N)
~
M.
Now
define
as:
Jk(M,N)s
~
M
8
in
an
obvious
way.
Then
J
8
k(M,N) = (as) "
1
(Xes>)
is
the
s-fold
k-jet
bundle.
A
multijet
bundle
is
some
s-fold
k-jet
bundle.
And
xes>
is
a
manifold
since
it
is
an
open
subset
of
M
8
•
Thus
J
8
k(M,N)
is
an
open
subset
of
Jk(M,N)s
and
is
also
a
smooth
manifold.
Now
let
f:
M
~
N
be
smooth.
Then
define
j
5
kf:
xes>
~
J
8
k(M,N)
in
the
natural
way,
i.e.,
j
5
kf(x
1
,
••
,xs)
=
(jkf
(x
1
),
••
,
jkf
(X
8
))
•
Theorem
3.3.10
(multijet
transversality
theorem)
Let
M
and
N
be
smooth
manifolds
and
w a
submanifold
of
J
8
k(M,N).
Let
Tw
=
{f
E cf"(M,N)
li
8
kf
.,fi
W}.
Then
Tw
is
a
residual
subset
of
cf"(M,N).
Furthermore,
if
W
is
compact,
then
Tw
is
open
[G
& G
1973,
p.57].
3.4
Infinite
dimensional
manifolds
Differentiable
manifolds
are
natural
generalization
of
Euclidean
spaces
by
building
up
from
Euclidean
spaces
and
gluing
together
by
some
overlapping
maps
and
charts.
Infinite
dimensional
manifolds
can
also
be
viewed
in
a
similar
way.
For
instance,
starting
with
some
fixed,
infinite
dimensional
linear
vector
spaces,
such
as
a
Hilbert
space
or
a
Banach
space,
one
can
form
a
manifold
by
gluing
122

together
open
sets
by
nice
overlapping
functions.
The
theory
of
infinite
dimensional
manifolds
can
provide
the
proper
framework
for
certain
analyses
and
various
connections
between
geometry
and
analysis,
particularly
the
operator
theory.
But
the
need
for
infinite
dimensional
manifolds
also
arises
in
another
vein.
For
instance,
let
M
and
N
be
two
differentiable
manifolds,
there
is
great
interest
in
the
space
of
continuous
maps
from
M
to
N
and
its
subspaces
of
various
degrees
of
differentiability
for
these
maps.
We
shall
state
that
under
certain
conditions,
this
space
of
continuous
maps
is
an
infinite
dimensional
manifold.
First,
we
shall
review
some
definitions
and
properties
of
Banach
and
Hilbert
spaces,
then
an
infinite
dimensional
manifold
on
a
given
topological
vector
space
will
be
defined
and
facts
recalled.
The
spaces
of
maps
between
manifolds
will
be
defined
and
some
results
stated.
Let
V
be
a
vector
space
over
the
field
F
(either
R
or
C).
Vis
a
topological
vector
space
if
there
is
a
topology
on
V
such
that
rp:
V x V-+ V: ( rp(v
1
,v
2
)
= v
1
+ v
2
)
and
q,: F x V
-+
V: (
rp(a,v)
=
av)
are
continuous.
Let
V
be
a
vector
space,
a norm
on
V
is
a
function
v
-+
R, llxll,
for
each
x E V,
such
that:
(i)
llxll
~
o,
llxll
= o
iff
x =
o:
(ii)
llaxll =
lal
llxll,
for
x E V, a E
F:
(iii)
llx +
Yll
:S
llxll
+
IIYII
,
for
any
x,
y E
v.
With
the
given
norm,
one
can
define
a
metric
on
v
by
e(x,y)
= llx - Yll.
Then
one
can
define
a
topology
on
V
by
taking
the
open
ball,
B,(x)=
{Y
E
vle(x,y)
< E
},
as
a
basis.
A
topological
vector
space
whose
topology
arises
from
the
norm,
such
as
the
one
given
above,
is
a
Banach
space
provided
that
it
is
complete
with
respect
to
the
norm
or
the
metric
(this
is
the
cauchy
completion).
Let
B
1
and
B
2
be
Banach
spaces
and
let
f
be
a
linear
map
f:
B
1
-+
B
2
•
Suppose
f
is
bounded,
i.e.,
there
is
k > 0
such
that
llf(x)
II
S kllxll
for
all
x E B
1
•
Then
one
can
show
that
f
is
continuous.
123
